Abstract
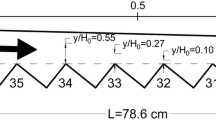
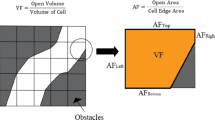
We present and discuss the results of a comprehensive study addressing the non-aerated region of the skimming flow in steep stepped spillways. Although flows in stepped spillways are usually characterized by high air concentrations concomitant with high rates of energy dissipation, the non-aerated region becomes important in small dams and/or spillways with high specific discharges. A relatively large physical model of such spillway was used to acquire data on flow velocities and water levels and, then, well-resolved numerical simulations were performed with a commercial code to reproduce those experimental conditions. The numerical runs benefited from the ability of using multi-block grids in a Cartesian coordinate system, from capturing the free surface with the TruVOF method embedded in the code, and from the use of two turbulence models: the \({k{-}\varepsilon}\) and the RNG\({k{-}\varepsilon}\) models. Numerical results are in good agreement with the experimental data corresponding to three volumetric flow rates in terms of the time-averaged velocities measured at diverse steps in the spillway, and they are in very satisfactory agreement for water levels along the spillway. In addition, the numerical results provide information on the turbulence statistics of the flow. This work also discusses important aspects of the flow, such as the values of the exponents of the power-law velocity profiles, and the characteristics of the development of the boundary layer in the spillway.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Abad JD, Rhoads BL, Guneralp I, García MH (2008) Flow structure at different stages in a meander bend with bendway weirs. J Hydraul Eng ASCE 134(8): 1052–1063
Amador A (2005) Comportamiento Hidráulico de los Aliviaderos Escalonados en Presas de Hormigón Compactado. Ph.D. thesis, UPC, Barcelona, Spain (in Spanish)
Amador A, Sanchez-Juni M, Dolz J (2006) Characterization of the non-aerated flow region in a stepped spillway by PIV. J Fluid Eng ASME 138(6): 1266–1273
André S (2004) High velocity aerated flows over stepped chutes with macro-roughness elements. Ph.D. thesis, EPFL, Lausanne, Switzerland
Arantes EJ (2007) Caracterização do Escoamento sobre Vertedouros em Degraus via CFD. Ph.D. thesis, EESC/USP, São Carlos, Brazil (in Portuguese)
Barenblatt GI (1996) Scaling, self-similarity, and intermediate asymptotics. Cambridge University Press, Cambridge
Barkhudarov MR (2004) Multi-block gridding technique for Flow-3D. Flow Science Technical Notes, Flow Science, Inc, TN59, available online
Bhuiyan ABM, Hey R (2007) Computation of three-dimensional flow field created by weir-type structures. Eng Appl Comput Fluid Mech 1(4): 350–360
Boes RM, Hager WH (2003) Two phase flow characteristics of stepped spillways. J Hydraul Eng ASCE 129(9): 661–670
Boes RM, Hager WH (2003) Hydraulic design of stepped spillways. J Hydraul Eng ASCE 129(9): 671–679
Bombardelli FA (2003) Characterization of coherent structures from parallel, LES computations of wandering effects in bubble plumes. In: Bizier P, DeBarry P (eds) Proceedings of the 2003 world water and environmental resources congress. Environmental & Water Resources Institute (EWRI), ASCE, Philadelphia (in CD)
Bombardelli FA (2004) Turbulence in multiphase models for aeration bubble plumes. Ph.D. thesis, University of Illinois at Urbana-Champaign
Bombardelli FA (2010) Water distribution systems. In: Fernando HJ (ed) Chapter 41 in Environmental fluid mechanics (in press)
Bombardelli FA, Jha SK (2009) Hierarchical modeling of dilute, suspended-sediment transport in open channels. Environ Fluid Mech 9(2): 207–230
Bombardelli FA, García MH, Caisley ME (2000) 2-D and 3-D numerical simulation of abrupt transitions in open-channel flows. Application to the design of canoe chutes. In: Proceedings of the 4th international conference on hydroinformatics IAHR, Iowa City, IA, USA (in CD)
Bombardelli FA, Hirt CW, García MH (2001) Discussion on computations of curved free surface water flow on spiral concentrators by Matthews et al. J Hydraul Eng ASCE 127(7): 629–631
Bombardelli FA, Buscaglia GC, Rehmann CR, Rincón LE, García MH (2007) Modeling and scaling of aeration bubble plumes: a two-phase flow analysis. J Hydraul Res IAHR 45(5): 617–630
Bombardelli FA, Cantero MI, García MH, Buscaglia GC (2009) Numerical aspects of the simulation of discontinuous saline underflows: the lock-exchange problem. J Hydraul Res IAHR 47(6): 777–789
Burden RL, Faires JD (2004) Numerical analysis, 8th ed. Brooks-Cole Publishing, Pacific Grove
Buscaglia GC, Bombardelli FA, García MH (2002) Numerical modeling of large scale bubble plumes accounting for mass transfer effects. Int J Multiph Flow 28: 1763–1785
Cain P, Wood IR (1981) Measurements of self-aerated flow on a spillway. J Hydraul Eng ASCE 107(HY11): 1407–1424
Caisley ME, Bombardelli FA, García MH (1999) Hydraulic model study of a canoe chute for low-head dams in Illinois. Civil Engineering Studies, Hydraulic Engineering Series No-63, University of Illinois at Urbana-Champaign
Carvalho R, Amador A (2008) Physical and numerical investigation of the skimming flow over a stepped spillway. In: Proceedings of the 3rd IAHR international symposium on hydraulic structures, Nanjing, China, pp 1767–1772
Chamani MR, Rajaratnam N (1999) Characteristics of skimming flow over stepped spillways. J Hydraul Eng ASCE 125(4): 361–368
Chanson H (2001) Hydraulic design of stepped spillways and downstream energy dissipators. Dam Eng 11(4): 205–242
Chanson H (2002) The hydraulics of stepped chutes and spillways. Balkema, Lisse
Chanson H (2009) Turbulent air-water flows in hydraulic structures: dynamic similarity and scale effects. Environ Fluid Mech 9(2): 125–142
Chatila J, Tabbara M (2004) Computational modeling of flow over an ogee spillway. Comput Struct 82: 1805–1812
Chen Q, Dai G, Liu H (2002) Volume of fluid model for turbulence numerical simulation of stepped spillway overflow. J Hydraul Eng ASCE 128(7): 683–688
Cheng X, Luo L, Zhao W (2004a) Study of aeration in the water flow over stepped spillway. In: Proceedings of the world water congress 2004, ASCE, Salt Lake City, UT, USA
Cheng X, Luo L, Zhao W, Li R (2004b) Two-phase flow simulation of aeration on stepped spillway. Prog Nat Sci 14(7): 626–630
Chow VT (1959) Open-channel hydraulics. McGraw-Hill, New York
Chung TJ (2006) Computational fluid dynamics. Cambridge University Press, New York
Crowe C, Sommerfeld M, Tsuji Y (1998) Multiphase flows with droplets and particles. CRC Press, Boca Raton
Dargahi B (2006) Experimental study and 3D numerical simulations for a free-overflow spillway. J Hydraul Eng ASCE 132(9): 899–907
Drew DA, Passman SL (1999) Theory of multicomponent fluids. Volume 135 of Applied mathematical sciences. Springer, New York
Felder S, Chanson H (2009) Energy dissipation, flow resistance, and gas liquid interfacial area in skimming flows on moderate-slope stepped spillways. Environ Fluid Mech 9(4): 427–441
Ferziger JH, Peric M (2002) Computational methods for fluid dynamics. Springer, Berlin
Flow Science, Inc (2008) FLOW-3D user’s manual, version 9.3. Flow Science, Inc, Los Alamos
Gioia G, Bombardelli FA (2002) Scaling and similarity in rough channel flows. Phys Rev Lett 88(1): 014501
Gomes JF (2006) Campo de Pressões: Condições deIncipiência à Cavitação em Vertedouros em Degraus com Declividade 1V:0,75H. Ph.D. thesis, UFRGS, Porto Alegre, Brazil (in Portuguese)
Gonzalez C (2005) An experimental study of free-surface aeration on embankment stepped chutes. Ph.D. thesis, University of Queensland, Brisbane, Australia
Gonzalez C, Chanson H (2007) Hydraulic design of stepped spillways and downstream energy dissipators for embankment dams. Dam Eng XVII(4): 223–244
Gonzalez C, Chanson H (2008) Turbulence and cavity recirculation in air-water skimming flows on a stepped spillway. J Hydraul Res IAHR 46(1): 65–72
Hager W, Boes RM (2000) Backwater and drawdown curves in stepped spillway flow. In: Proceedings of the 1st international workshop on hydraulics of stepped spillway, Zurich, Switzerland. A.A. Balkema Publisher, Rotterdam, pp 129–136
Higgs J, Frizell KW (2004) Investigation of the lake plant pump station—Lower Colorado River Authority. Hydraulic Laboratory Report HL-2004-02, Denver Technical Center, Bureau of Reclamation, United States Department of the Interior, Denver, CO, December, 2004
Hirt CW (2003) Modeling turbulent entrainment of air at a free surface. Technical Note 61, Flow Science, Inc (FSi-03-TN61)
Hirt CW, Nichols BD (1981) Volume of fluid (VOF) method for the dynamics of free boundaries. J Comput Phys 39: 201–225
Hirt CW, Sicilian JM (1985) A porosity technique for the definition of obstacles in rectangular cell meshes. In: Proceedings of the 4th international conference on ship hydro-dynamics. National Academy of Science, Washington, DC
Ho DKH, Cooper BW, Riddette KM, Donohoo SM et al (2006) Application of numerical modelling to spillways in Australia. In: Berga L (eds) Dams and reservoirs, societies and environment in the 21st century. Taylor & Francis Group, London
Hrenya CM, Sinclair JL (1997) Effects of particle-phase turbulence in gas-solid flows. AIChE J 43(4): 853–869
Jha SK, Bombardelli FA (2010) Toward two-phase flow modeling of nondilute sediment transport in open channels. J Geophys Res 115: F03015
Jiménez J (2004) Turbulent flows over rough walls. Annu Rev Fluid Mech 36: 173–196
Johnson MC, Savage BM (2006) Physical and numerical comparison of flow over ogee spillway in the presence of tailwater. J Hydraul Eng ASCE 132(12): 1353–1357
Launder BE, Spalding DB (1972) Lectures in mathematical models of turbulence. Academic Press, London
Matos J (2000) Hydraulic design of stepped spillways over RCC dams. In: Proceedings of the 1st international workshop on hydraulics of stepped spillway, Zurich, Switzerland. Balkema AA Publisher, Rotterdam, pp 187–194
Matos J, Frizell KW (1997) Air concentration measurements in highly turbulent aerated flow. In: Wang SSY, Carstens T (eds) Proceedings of the 27th IAHR congress, Theme D, vol 1. San Francisco, USA, pp 149–154
Matos J, Frizell KW (2000) Air concentration and velocity measurements on self-aerated flow down stepped chutes. In: Proceedings of the ASCE 2000 conference, Minneapolis, USA
Matos J, Sanchez-Juny M, Quintela A, Dolz J (1999) Characteristic depth and pressure profiles in skimming flow over stepped spillways. In: Proceedings of the 29th IAHR congress, Graz, Austria
Matos J, Frizell KH, André S, Frizell KW (2002) On the performance of velocity measurement techniques in air-water flows. In: Proceedings of the EWRI/IAHR joint conference on hydraulic measurements & experimental methods ASCE, Estes Park, USA
Matthews BW, Fletcher CAJ, Partridge AC, Vasquez S (1999) Computations of curved free surface water flow on spiral concentrators. J Hydraul Eng ASCE 125(11): 1126–1139
Matthews BW, Fletcher CAJ, Partridge AC, Vasquez S (2001) Computations of curved free surface water flow on spiral concentrators. J Hydraul Eng ASCE 127(7): 629–631
Meireles M (2004) Emulsionamento de Ar e Dissipação de Energia do Escoamento em Descarregadores em Degraus. M.Sc. thesis, IST, Lisbon, Portugal (in Portuguese)
Meireles I, Matos J (2009) Skimming flow in the non-aerated region of stepped spillways over embankment dams. J Hydraul Eng ASCE 135(8): 685–689
Meireles I, Matos J, Melo JF (2006) Skimming flow properties upstream of air entrainment inception on steeply sloping stepped chutes. In: Proceedings of the international symposium on hydraulic structures IAHR, Ciudad Guayana, Venezuela
Ohtsu I, Yasuda Y (1997) Characteristics of flow conditions on stepped channels. In: Proceedings of the 27th IAHR congress, San Francisco, Theme D, pp 583–588
Ohtsu I, Yasuda Y, Takahashi M (2004) Flow characteristics of skimming flows in stepped channels. J Hydraul Eng ASCE 130(9): 860–869
Paxson G, Savage B (2006) Labyrinth spillways: comparison of two popular U.S.A. design methods and consideration of non-standard approach conditions and geometries. In: Matos J, Chanson H (eds) Proceedings of the international junior researcher and engineer workshop on hydraulic structures, report CH61/06, Division of Civil Engineering, The University of Queensland, Brisbane, Australia. ISBN 1864998687
Pope SB (2000) Turbulent flows. Cambridge University Press, Cambridge
Prosperetti A, Tryggvason G (2007) Computational methods for multiphase flow. Cambridge Press, Cambridge
Renna F (2004) Caratterizzazione Fenomenologica del Moto di un Fluido Bifasico Lungo Scaricatori a Gradini. Ph.D. thesis, Politecnico di Bari, Cosenza, Italy (in Italian)
Rodi W (1984) Turbulence models and their application in hydraulics. State-of-the-Art Paper, IAHR
Rodríguez JF, Bombardelli FA, García MH, Frothingham K, Rhoads BL, Abad JD (2004) High-resolution numerical simulation of flow through a highly sinuous river reach. Water Resour Manag 18: 177–199
Sánchez-Juny M (2001) Comportamiento Hidráulico de los Aliviaderos Escalonados en Presas de Hormigón Compactado. Análisis del Campo de Presiones. Ph.D. thesis, UPC, Barcelona, Spain (in Spanish)
Savage BM, Johnson MC (2001) Flow over ogee spillway: physical and numerical model case study. J Hydraul Eng ASCE 127(8): 640–649
Savage B, Frizell K, Crowder J (2004) Brains versus brawn: the changing world of hydraulic model studies. In: Proceedings of the 2004 annual conference, Association of State Dam Safety Officials (ASDSO), Phoenix, USA
Song CCS, Zhou F (1999) Simulations of free surface flow over spillway. J Hydraul Eng ASCE 125(9): 959–967
Storti MA, Nigro NM, Paz RR, Dalcín L (2008) Dynamic boundary condition in computational fluid dynamics. Comput Methods Appl Mech Eng 197(13–16): 1219–1232
Tabbara M, Chatila J, Awwad R (2005) Computational simulation of flow over stepped spillways. Comput Struct 83: 2215–2224
Unami K, Kawachi T, Babar MM, Itagaki H (1999) Two-dimensional numerical model of spillway flow. J Hydraul Eng ASCE 125(4): 369–375
Wade RJ, Rhoads BL, Rodriguez J, Daniels M, Wilson D, Herricks EE, Bombardelli F, Garcia M, Schwartz J (2002) Integrating science and technology to support stream naturalization near Chicago, Illinois. J Am Water Resour Assoc AWRA 38(4): 931–944
Wilcox DC (1993) Turbulence modeling for CFD. DCW Industries, La Canada
Yakhot V, Orszag SA (1986) Renormalization group analysis of turbulence. I. Basic theory. J Sci Comput 1(1): 3–51
Yakhot V, Smith LM (1992) The renormalization group, the E-expansion, and the derivation of turbulence model. J Sci Comput 3: 35–61
Yasuda Y, Ohtsu I (2003) Effect of step cavity area on flow characteristics of skimming flows on stepped chutes. In: Proceedings of the 30th IAHR international congress, Thessaloniki, Greece, pp 703–710
Yasuda Y, Takahashi M, Ohtsu I (2004) Discussion on ‘Volume of fluid model for turbulence numerical simulation of stepped spillway overflow,’ by Chen et al. J Hydraul Eng ASCE 130(2): 170
Ye M, Wu C, Chen Y, Zhou Q (2006) Case study of an S-shaped spillway using physical and numerical models. J Hydraul Eng ASCE 132(9): 892–898
Acknowledgments
Fabián Bombardelli gratefully acknowledges the support of the California Department of Water Resources (DWR), through Award 4600007984 TO BD01, and the California Water Resources Control Board (CAWRCB), through Award 06-447-300 TO 5. Inês Meireles was a Visiting Scholar at the University of California, Davis, during the months of September 2007–March 2008, and was financially supported by a Fulbright/FLAD Research Grant. This support is gratefully acknowledged. The financial support granted by INAG, Portuguese Water Institution (Project 2003/2029/INAG), and by the Portuguese Foundation for Science and Technology (FCT), through Project PTDC/ECM/108128/2008, is also gratefully acknowledged. Currently, Inês Meireles is supported by FCT, Grant No. SFRH/BD/38003/2007.
Open Access
This article is distributed under the terms of the Creative Commons Attribution Noncommercial License which permits any noncommercial use, distribution,and reproduction in any medium, provided the original author(s) and source are credited.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This is an open access article distributed under the terms of the Creative Commons Attribution Noncommercial License (https://creativecommons.org/licenses/by-nc/2.0), which permits any noncommercial use, distribution, and reproduction in any medium, provided the original author(s) and source are credited.
About this article
Cite this article
Bombardelli, F.A., Meireles, I. & Matos, J. Laboratory measurements and multi-block numerical simulations of the mean flow and turbulence in the non-aerated skimming flow region of steep stepped spillways. Environ Fluid Mech 11, 263–288 (2011). https://doi.org/10.1007/s10652-010-9188-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10652-010-9188-6