Skip to main content
SpringerLink
Log in

3D numerical modelling of turbidity currents

  • Original Article
  • Published:
Environmental Fluid Mechanics Aims and scope Submit manuscript

Abstract

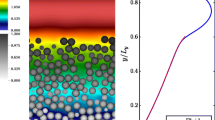
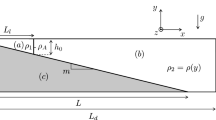
During floods, the density of river water usually increases due to a subsequent increase in the concentration of the suspended sediment that the river carries, causing the river to plunge underneath the free surface of a receiving water basin and form a turbidity current that continues to flow along the bottom. The study and understanding of such complex phenomena is of great importance, as they constitute one of the major mechanisms for suspended sediment transport from rivers into oceans, lakes or reservoirs. Unlike most of the previous numerical investigations on turbidity currents, in this paper, a 3D numerical model that simulates the dynamics and flow structure of turbidity currents, through a multiphase flow approach is proposed, using the commercial CFD code FLUENT. A series of numerical simulations that reproduce particular published laboratory flows are presented. The detailed qualitative and quantitative comparison of numerical with laboratory results indicates that apart from the global flow structure, the proposed numerical approach efficiently predicts various important aspects of turbidity current flows, such as the effect of suspended sediment mixture composition in the temporal and spatial evolution of the simulated currents, the interaction of turbidity currents with loose sediment bottom layers and the formation of internal hydraulic jumps. Furthermore, various extreme cases among the numerical runs considered are further analyzed, in order to identify the importance of various controlling flow parameters.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Mulder T, Alexander J (2001) The physical character of subaqueous sedimentary density flows and their deposits. Sedimentology 48: 269–299

    Article  Google Scholar 

  2. Janbu NE, Nemec W, Kırman E, Özaksoy V (2009) Facies anatomy of a channelized sandrich turbiditic system: the Eocene Kusuri Formation in the Sinop Basin, north-central Turkey. In: Nichols G, Paola C, Williams E, Paola C (eds) Sedimentary processes, environments and basins. Wiley, Special Publication Number 38 of the International Association of Sedimentologists, Kingston upon Thames, UK, pp 457–517

  3. Posamentier HW, Kolla V (2003) Seismic geomorphology and stratigraphy of depositional elements in deep-water settings. J Sediment Res 73(3): 367–388

    Article  Google Scholar 

  4. Saller A, Werner K, Sugiaman F, Cebastiant A, May R, Glenn D, Barker C (2008) Characteristics of Pleistocene deep-water fan lobes and their application to an upper Miocene reservoir model, offshore East Kalimantan, Indonesia. AAPG Bull 92(7): 919–949

    Article  Google Scholar 

  5. Lovell JPB (1971) Control of slope on deposition from small-scale turbidity currents : experimental results and possible geological significance. Sedimentology 17: 81–88

    Article  Google Scholar 

  6. Simpson JE, Britter RE (1979) The dynamics of the head of a gravity current advancing over a horizontal surface. J Fluid Mech 94: 477–495

    Article  CAS  Google Scholar 

  7. Britter RE, Linden PF (1980) The motion of the front of a gravity current travelling down an incline. J Fluid Mech 99: 531–543

    Article  Google Scholar 

  8. Garcia M, Parker G (1989) Experiments on hydraulic jumps in turbidity currents near a canyon-fan transition. Science. doi:10.1126/science.245.4916.393

    Google Scholar 

  9. Kneller BC, Bennett SJ, McCaffrey WD (1997) Velocity and turbulence structure of density currents and internal solitary waves: potential sediment transport and the formation of wave ripples in deep water. Sediment Geol 112: 235–250

    Article  Google Scholar 

  10. Gladstone C, Phillips JC, Sparks RSJ (1998) Experiments on bidisperse, constant-volume gravity currents: propagation and sediment deposition. Sedimentology 45: 833–843

    Article  Google Scholar 

  11. Baas JH, Van Kesteren W, Postma G (2004) Deposits of depletive, quasi-steady high density turbidity currents: a flume analogue of bed geometry, structure and texture. Sedimentology 51: 1053–1089

    Article  Google Scholar 

  12. Garcia M (1994) Depositional turbidity currents laden with poorly sorted sediment. J Hydraul Eng 120(11): 1240–1263

    Article  Google Scholar 

  13. Hartel C, Meiburg E, Necker F (2000) Analysis and direct numerical simulation of the flow at a gravity-current head: Part 1. Flow topology and front speed for slip and no-slip boundaries. J Fluid Mech 418: 189–212

    Article  Google Scholar 

  14. Kassem A, Imran J (2001) Simulation of turbid underflows generated by the plunging of a river. Geology 29(7): 655–658

    Article  Google Scholar 

  15. Heimsund S, Hansen EWM, Nemec W (2002) Computational 3D fluid-dynamics model for sediment transport, erosion and deposition by turbidity currents. In: Knoper M, Cairncross B (eds) Abstracts, international association of sedimentologists 16th international sedimentological congress. Rand Afrikaans University, Johannesburg, pp 151–152

    Google Scholar 

  16. Lavelli A, Boillat JL, De Cesare G (2002) Numerical 3D modelling of the vertical mass exchange induced by turbidity currents in Lake Lugano (Switzerland). In: Proceedings 5th international conference on hydro-science and -engineering (ICHE-2002) (Reference: LCH-CONF-2002-012 Note: [355])

  17. Necker F, Hartel C, Kleiser L, Meinburg E (2002) High-resolution simulations of particle driven gravity currents. Int J Multiph Flow 28: 279–300

    Article  CAS  Google Scholar 

  18. Cantero M, Garcia M, Buscaglia G, Bombardelli F, Dari E (2003) Multidimensional CFD simulation of a discontinuous density current. In: Proceedings of the XXX IAHR international congress, Thessaloniki, Greece, 2003

  19. Bombardelli FA, Cantero MI, Buscaglia GC, García MH (2004) Comparative study of convergence of CFD comercial codes when simulating dense underflows. In: Buscaglia G, Dari E, Zamonsky O (eds) Mec’anica Computacional, vol XXIII. Bariloche, Argentina, November 2004

  20. Imran J, Kassem A, Khan SM (2004) Three-dimensional modeling of density current. I. Flow in straight confined and unconfined channels. J Hydraul Res 42(6): 578–590

    Article  Google Scholar 

  21. Blanchette F, Strauss M, Meiburg E, Kneller B, Glinsky ME (2005) High-resolution numerical simulations of resuspending gravity currents: conditions for self-sustainment. J Geophys Res 110: C12022. doi:10.1029/2005JC002927

    Article  Google Scholar 

  22. Huang H, Imran J, Pirmez C (2005) Numerical model of turbidity currents with a deforming bottom boundary. J Hydraul Eng 131(4): 283–293

    Article  Google Scholar 

  23. Ooi SK, Constantinescu G, Weber LJ (2007) 2D large-eddy simulation of lock-exchange gravity current flows at high Grashof numbers. J Hydraul Eng 133(9): 1037–1047

    Article  Google Scholar 

  24. Mehdizadeh A, Firoozabadi B, Farhanieh B (2008) Numerical simulation of turbidity current using \({\overline{v^{2}}-f}\) turbulence model. J Appl Fluid Mech 1(2): 45–55

    Google Scholar 

  25. Cantero MI, Balachandar S, Garcia MH (2008) An Eulerian–Eulerian model for gravity currents driven by inertial paricles. Int J Multiph Flow 34: 484–501

    Article  CAS  Google Scholar 

  26. Cantero MI, Balachandar S, Garcia MH, Bock D (2008) Turbulent structures in planar gravity currents and their influence on the flow dynamics. J Geophys Res 113: C08018. doi:10.1029/2007JC004645

    Article  Google Scholar 

  27. Singh J (2008) Simulation of suspension gravity currents with different initial aspect ratio and layout of turbidity fence. Appl Math Model 32: 2329–2346

    Article  Google Scholar 

  28. ANSYS FLUENT 6.3.26 User’s Guide (2008)

  29. Hinze JO (1975) Turbulence. Mc Graw-Hill Publishing Co, New York

    Google Scholar 

  30. Bombardelli FA, Jha SK (2009) Hierarchical modeling of the dilute transport of suspended sediment in open channels. Environ Fluid Mech 9: 207–235

    Article  CAS  Google Scholar 

  31. Dey S (1999) Sediment threshold. Appl Math Model 23: 399–417

    Article  Google Scholar 

  32. Farrell GJ, Stefan HG (1986) Buoyancy induced plunging flows into reservoirs and coastal regions. Tech. Rep. No. 241, At. Anthony Falls Hydr. Lab., University of Minnesota, MN

  33. Cebeci T, Bradshaw P (1977) Momentum transfer in boundary layers. Hemisphere Publishing Corporation, New York

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Laboratory of Hydraulics and Hydraulic Structures, Department of Civil Engineering, Democritus University of Thrace, V. Sofias 12, 671 00, Xanthi, Greece

    Anastasios N. Georgoulas, Panagiotis B. Angelidis & Nikolaos E. Kotsovinos

  2. Laboratory of Fluid Mechanics, Mechanical Department, Institute of Technology, Agios Loukas, 654 04, Kavala, Greece

    Theologos G. Panagiotidis

Authors
  1. Anastasios N. Georgoulas
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Panagiotis B. Angelidis
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Theologos G. Panagiotidis
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Nikolaos E. Kotsovinos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Anastasios N. Georgoulas.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Georgoulas, A.N., Angelidis, P.B., Panagiotidis, T.G. et al. 3D numerical modelling of turbidity currents. Environ Fluid Mech 10, 603–635 (2010). https://doi.org/10.1007/s10652-010-9182-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10652-010-9182-z

Keywords

Search

Navigation