Aspects of the Segre variety ${\mathcal{S}_{1,1,1}(2)}$

Abstract

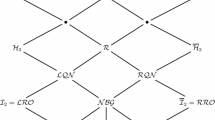
We consider various aspects of the Segre variety ${\mathcal{S}:=\mathcal{S} _{1,1,1}(2)}$ in PG(7, 2), whose stabilizer group ${\mathcal{G}_{\mathcal{S}}<{\rm GL}(8,2)}$ has the structure ${\mathcal{N}\rtimes{\rm Sym}(3),}$ where ${\mathcal{N} :={\rm GL}(2,2)\times{\rm GL}(2,2)\times{\rm GL} (2,2).}$ In particular we prove that ${\mathcal{S}}$ determines a distinguished Z ₃-subgroup ${\mathcal{Z}<{\rm GL}(8,2)}$ such that ${A\mathcal{Z}A^{-1}=\mathcal{Z},}$ for all ${A\in\mathcal{G}_{\mathcal{S}},}$ and in consequence ${\mathcal{S}}$ determines a ${\mathcal{G}_{\mathcal{S}}}$-invariant spread of 85 lines in PG(7, 2). Furthermore we see that Segre varieties ${\mathcal{S}_{1,1,1}(2)}$ in PG(7, 2) come along in triplets ${\{\mathcal{S},\mathcal{S}^{\prime},\mathcal{S}^{\prime\prime}\}}$ which share the same distinguished Z ₃-subgroup ${\mathcal{Z}<{\rm GL}(8,2).}$ We conclude by determining all fifteen ${\mathcal{G}_{\mathcal{S}}}$-invariant polynomial functions on PG(7, 2) which have degree < 8, and their relation to the five ${\mathcal{G}_{\mathcal{S}}}$-orbits of points in PG(7, 2).