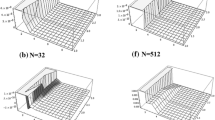
We have developed an initial-value technique for self-adjoint singularly perturbed two-point boundary value problems. The original problem is reduced to its normal form, and the reduced problem is converted into first-order initial-value problems. These initial-value problems are solved by the cubic spline method. Numerical illustrations are given at the end to demonstrate the efficiency of our method. Graphs are also depicted in support of the results.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
I. P. Boglaev, “A variational difference scheme for boundary value problems with a small parameter in the highest derivative,” USSR, Comput. Math. Math. Phys., 21, No. 4, 71–81 (1981).
E. P. Doolan, J. J. H. Miller, and W. H. A. Schilders, Uniform Numerical Methods for Problems with Initial and Boundary Layers, Boole Press, Dublin (1980).
M. G. Gasparo and M. Macconi, “Initial-value methods for second-order singularly perturbed boundary value problems,” J. Optim. Theory Appl., 66, 197–210 (1990).
M. Kumar, P. Singh, and H. K. Mishra, “An initial-value technique for singularly perturbed boundary value problems via cubic spline,” Int. J. Comput. Methods Eng. Sci. and Mech., 6, 1–9 (2007).
M. Kumar, P. Singh, and H. K. Mishra, “Numerical treatment of singularly perturbed two-point boundary value problems using initial value method,” Communicated.
J. J. H. Miller, “On the convergence uniformly in ε of difference schemes for a two-point boundary singular perturbation problem,” in: Numerical Analysis of Singular Perturbation Problems, Academic Press, New York, (1979), pp. 467–474.
K. Niijima, “On a three-point difference scheme for a singular perturbation problem without a first derivative term. II,” Mem. Numer. Math., 7, 11–27 (1980).
K. Niijima, “On a three-point difference scheme for a singular perturbation problem without a first derivative term. I,” Mem. Numer. Math., 7, 1–10 (1980).
M. H. Potter and H. F. Weinberger, Maximum Principles in Differential Equations, Prentice-Hall, Englewood Cliffs, New Jersey (1967).
M. Sakai and R. A. Usmani, “On exponential splines,” J. Approxim. Theory, 47, 122–131 (1986).
A. H. Schatz and L. B. Wahlbin, “On the finite element method for singularly perturbed reaction diffusion problems in two and one dimension,” Math. Comput., 40, 47–89 (1983).
K. Surla and V. Jerkovic, “Some possibilities of applying spline collocations to singular perturbation problems,” Numer. Methods Approxim. Theory, Vol. II, Novisad, 19–25 (1985).
V. Vukoslavcevic and K. Surla, “Finite element method for solving self-adjoint singularly perturbed boundary value problems,” Math. Montisnigri, VII, 69–86 (1996).
Author information
Authors and Affiliations
Corresponding author
Additional information
An erratum to this article can be found at http://dx.doi.org/10.1007/s10598-009-9040-3
Rights and permissions
About this article
Cite this article
Mishra, H.K., Kumar, M. & Singh, P. Initial-value technique for self-adjoint singular perturbation boundary value problems. Comput Math Model 20, 207–217 (2009). https://doi.org/10.1007/s10598-009-9029-y
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10598-009-9029-y