Distortion compensation as a shape optimisation problem for a sharp interface model

Abstract

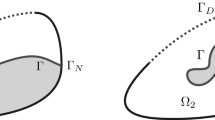
A mechanical equilibrium problem for a material consisting of two components with different densities is considered. Due to the heterogeneous material densities, the outer shape of the underlying workpiece can be changed by shifting the interface between the subdomains. In this paper, the problem is modeled as a shape design problem for optimally compensating unwanted workpiece changes. The associated control variable is the interface. Regularity results for transmission problems are employed for a rigorous derivation of suitable first-order optimality conditions based on the speed method. The paper concludes with several numerical results based on a spline approximation of the interface.

Appendix: Proof of Theorem 2.1

The result concerning higher regularity is a direct consequence of [8, Theorem 5.3.8]. Here, we show that the Eq. (2.5) indeed has a unique solution for every $\chi \in \text {X}(\text {D})$. This can be seen as follows:¹ Since $A_1, A_2$ are positive definite with coercivity constants $k_1>k_2>0$ and from Korns inequality (with constant $\theta _{K}>0$) it follows that there exist constants $C,\theta >0$, independent of $\chi$, such that for all $\varvec{\varphi }\in H^1(\text {D};{\mathbf {R}}^d)$

$$\begin{aligned} a_{\chi }(\varvec{\varphi },\varvec{\varphi })= & {} \int _{\text {D}} \chi A_1\varepsilon (\varvec{\varphi }):\varepsilon (\varvec{\varphi })\, dx+\int _{\text {D}} (1-\chi )A_2\varepsilon (\varvec{\varphi }):\varepsilon (\varvec{\varphi })\, dx\nonumber \\\ge & {} \underbrace{\int _{\text {D}} \chi (k_1-k_2)\varepsilon (\varvec{\varphi }):\varepsilon (\varvec{\varphi })\, dx}_{\ge 0}+\int _{\text {D}} k_2\varepsilon (\varvec{\varphi }):\varepsilon (\varvec{\varphi })\, dx\nonumber \\\ge & {} \theta \Vert \varvec{\varphi }\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)}^2, \end{aligned}$$

(6.1)

where $\theta :=k_2 \theta _K$ and

$$\begin{aligned} a_{\chi }(\varvec{\varphi },\varvec{\psi })\le C\Vert \varvec{\varphi }\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)}\Vert \varvec{\psi }\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)}. \end{aligned}$$

Thus the Lemma of Lax and Milgram (see [11, pp. 297–299, Theorem 1]) guarantees the unique solvability of the variational problem:

$$\begin{aligned} \text {Find }\mathbf {u}\in \mathbf {\mathcal {W}}: \quad a_{\chi }(\mathbf {u},\varvec{\varphi })=\int _{\text {D}}\beta _\chi \mathrm{\, div\,}(\varvec{\varphi })\, dx\; \text { for all } \varvec{\varphi }\in \mathbf {\mathcal {W}}. \end{aligned}$$

Since $\varvec{\varphi }\mapsto \int _{\text {D}}\beta _\chi \mathrm{\, div\,}(\varvec{\varphi })\, dx\in \mathbf {\mathcal {W}}^{-1}$ according to the Lemma of Lax and Milgram

$$\begin{aligned} \int _{\text {D}}\beta _\chi \mathrm{\, div\,}(\varvec{\varphi })\, dx\le \max \{\beta _2,\beta _1\}\sqrt{|D|}\Vert \varvec{\varphi }\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)}. \end{aligned}$$

Notice that the constants are independent of $\Omega$. In order to see this a priori bound, recall $\chi \subset \text {X}(\text {D})$ and let $\mathbf {u}_{\chi }$ denote the corresponding solution to (2.5). Using (6.2) we compute

$$\begin{aligned} \theta \Vert \mathbf {u}_n\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)}^2&\le a_{\chi _n}(\mathbf {u}_n,\mathbf {u}_n) = \int _{\text {D}} \beta _{\chi _n}(x)\mathrm{\, div\,}(\mathbf {u}_n)\, dx \le C\Vert \mathbf {u}_n\Vert _{\text {H}^1(\text {D};{\mathbf {R}}^d)} \end{aligned}$$

with $C:=\max \{\beta _1,\beta _2\}\sqrt{3|D|}/\theta$.