Skip to main content
SpringerLink
Log in

A measure space approach to optimal source placement

  • Published:
Computational Optimization and Applications Aims and scope Submit manuscript

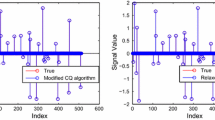
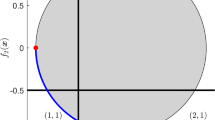
Abstract

The problem of optimal placement of point sources is formulated as a distributed optimal control problem with sparsity constraints. For practical relevance, partial observations as well as partial and non-negative controls need to be considered. Although well-posedness of this problem requires a non-reflexive Banach space setting, a primal-predual formulation of the optimality system can be approximated well by a family of semi-smooth equations, which can be solved by a superlinearly convergent semi-smooth Newton method. Numerical examples indicate the feasibility for optimal light source placement problems in diffusive photochemotherapy.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Algorithm 1
Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Attouch, H., Buttazzo, G., Michaille, G.: Variational Analysis in Sobolev and BV Spaces. Philadelphia, SIAM (2006)

    MATH  Google Scholar 

  2. Baas, P., Murrer, L., Zoetmulder, F., Stewart, F., Ris, H., van Zandwijk, N., Peterse, J., Rutgers, E.: Photodynamic therapy as adjuvant therapy in surgically treated pleural malignancies. Br. J. Cancer 76, 819–826 (1997)

    Article  Google Scholar 

  3. Bredies, K., Pikkarainen, H.: Inverse problems in spaces of measures. Tech. Rep. 2010–037, Mobis (2010). http://math.uni-graz.at/mobis/publications/SFB-Report-2010-037.pdf

  4. Brezis, H.: Functional Analysis, Sobolev Spaces and Partial Differential Equations. Springer, New York (2010)

    Book  Google Scholar 

  5. Casas, E., Herzog, R., Wachsmuth, G.: Optimality conditions and error analysis of semilinear elliptic control problems with L 1 cost functional (2011, submitted). http://www.tu-chemnitz.de/mathematik/part_dgl/publications/Casas_Herzog_Wachsmuth_Optimality_Conditions_and_Error_Analysis_of_Semilinear_Elliptic_Control_Problems_with_L1_Cost_Functional.pdf

  6. Clason, C., Kunisch, K.: A duality-based approach to elliptic control problems in non-reflexive Banach spaces. ESAIM Control Optim. Calc. Var. 17(1), 243–266 (2011). doi:10.1051/cocv/2010003

    Article  MathSciNet  MATH  Google Scholar 

  7. DiBenedetto, E.: Real Analysis. Birkhäuser, Boston (2002)

    Book  MATH  Google Scholar 

  8. Ekeland, I., Témam, R.: Convex Analysis and Variational Problems. Philadelphia, SIAM (1999)

    Book  MATH  Google Scholar 

  9. Elstrodt, J.: Maß- und Integrationstheorie, 5 edn. Springer, Berlin (2005)

    MATH  Google Scholar 

  10. Gallouet, T., Monier, A.: On the regularity of solutions to elliptic equations. Rend. Mat. Appl. 19(4), 471–488 (2000) (1999)

    MathSciNet  Google Scholar 

  11. Gilbarg, D., Trudinger, N.S.: Elliptic Partial Differential Equations of Second Order. Classics in Mathematics. Springer, Berlin (2001). Reprint of the 1998 edition

    MATH  Google Scholar 

  12. Hintermüller, M., Kunisch, K.: Total bounded variation regularization as a bilaterally constrained optimization problem. SIAM J. Appl. Math. 64(4), 1311–1333 (2004) (electronic)

    Article  MathSciNet  MATH  Google Scholar 

  13. Ito, K., Kunisch, K.: Lagrange Multiplier Approach to Variational Problems and Applications. Advances in Design and Control, vol. 15. SIAM, Philadelphia (2008)

    Book  MATH  Google Scholar 

  14. Logg, A., Wells, G.N.: DOLFIN: Automated finite element computing. ACM Trans. Math. Softw. 37(2), 20 (2010). doi:http://doi.acm.org/10.1145/1731022.1731030

    Article  MathSciNet  Google Scholar 

  15. Meyer, C., Panizzi, L., Schiela, A.: Uniqueness criteria for the adjoint equation in state-constrained elliptic optimal control. Numer. Funct. Anal. Optim. 32(9), 983–1007 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  16. Stadler, G.: Elliptic optimal control problems with L 1-control cost and applications for the placement of control devices. Comput. Optim. Appl. 44(2), 159–181 (2009). doi:10.1007/s10589-007-9150-9

    Article  MathSciNet  MATH  Google Scholar 

  17. Stampacchia, G.: Le problème de Dirichlet pour les équations elliptiques du second ordre à coefficients discontinus. Ann. Inst. Fourier 15(1), 189–258 (1965)

    Article  MathSciNet  MATH  Google Scholar 

  18. Troianiello, G.M.: Elliptic Differential Equations and Obstacle Problems. The University Series in Mathematics. Plenum Press, New York (1987)

    MATH  Google Scholar 

  19. Wachsmuth, D., Wachsmuth, G.: Convergence and regularization results for optimal control problems with sparsity functional. ESAIM Control Optim. Calc. Var. (2010). doi:10.1051/cocv/2010027

    MATH  Google Scholar 

  20. Wachsmuth, D., Wachsmuth, G.: On the regularization of optimization problems with inequality constraints. Tech. Rep. 2011-1, RICAM Report (2011). http://www.ricam.oeaw.ac.at/publications/reports/11/rep11-01.pdf

Download references

Acknowledgements

This work was supported by the Austrian Science Fund (FWF) under grant SFB F32 (SFB “Mathematical Optimization and Applications in Biomedical Sciences”). The authors wish to thank Patricia Brunner, Manuel Freiberger and Hermann Scharfetter of the Institute of Medical Engineering, Graz University of Technology, for their help on the photochemotherapy example.

Author information

Authors and Affiliations

  1. Institute for Mathematics and Scientific Computing, University of Graz, Graz, Austria

    Christian Clason & Karl Kunisch

Authors
  1. Christian Clason
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Karl Kunisch
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Christian Clason.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Clason, C., Kunisch, K. A measure space approach to optimal source placement. Comput Optim Appl 53, 155–171 (2012). https://doi.org/10.1007/s10589-011-9444-9

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10589-011-9444-9

Keywords

Search

Navigation