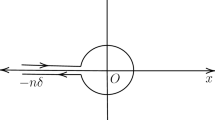
Abstract
In this paper we generalize the method used to prove the Prime Number Theorem to deal with finite fields, and prove the following theorem:
where π(x) denotes the number of monic irreducible polynomials in F q [t] with norm ⩽ x.
Similar content being viewed by others
References
M. Kruse, H. Stichtenoth: Ein Analogon zum Primzahlsatz fur algebraische Functionenkoper. Manuscripta Math. 69 (1990), 219–221. (In German.)
H. Davenport: Multiplicative Number Theory. Springer-Verlag, New York, 1980.
Author information
Authors and Affiliations
Corresponding author
Additional information
The work is supported by Grants with No. 60772131, NCET08, and the Ph.D. Programs Foundation of Ministry of Education of China 2009.
Rights and permissions
About this article
Cite this article
Wang, Q., Kan, H. Counting irreducible polynomials over finite fields. Czech Math J 60, 881–886 (2010). https://doi.org/10.1007/s10587-010-0055-x
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10587-010-0055-x