Abstract
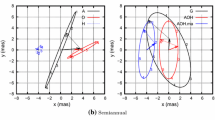
Differential equations ruling the Earth’s polar motion are slightly asymmetric with respect to the pole coordinates. This is not only associated with the lack of axial symmetry around the Earth figure axis (triaxiality) but also with the longitude dependency of the pole tide (the main contribution). We propose a consistent handling of both asymmetric contributions, formulating a unique equation in the complex equatorial plane, of which we derive a general solution. Difference with respect to the usual symmetric solution is discussed and found significant in light of the present accuracy of the observed pole coordinates. For the same geophysical excitation, the prograde Chandler wobble is accompanied by a retrograde component up to 2 milliarcseconds (mas), transforming it in a slight elliptic motion. The asymmetric contribution is relatively larger in the geodetic excitation function, for Chandler wobble excitation mixes prograde and retrograde components of comparable level (1 mas).





Similar content being viewed by others
Notes
The equatorial path of a pseudo-periodic component of the polar motion is an ellipse resulting from the combination of two opposite uniform circular motions. The corresponding ellipticity is called intrinsic when it purely results from the Earth’s property regardless of the excitation.
References
Bizouard, C.: Interprétation géophysique du mouvement du pôle de l’heure au siècle. Mémoire d’Habilitation à diriger des recherches. ftp://syrte.obspm.fr/pub/bizouard/habil.pdf, Observatoire de Paris (2012) (in press)
Bizouard, C., Seoane, L.: The atmospheric and oceanic excitation of the rapid polar motion. J. Geod. 84, 19–30 (2010)
Brzeziński, A., Capitaine, N.: The use of the precise observations of the celestial ephemeris pole in the analysis of geophysical excitation of earth rotation. J. Geophys. Res 98B4, 6667–6675 (1993)
Breziński, A., Mathews, P.M.: Recent advances in modelling the lunisolar perturbation in polar motion corresponding to high frequency nutation. Journées Systèmes de Référence spatio-temporels 2002, Proceeding, N. Capitaine (eds) (2002)
Chandler, S.C.: On the variation of latitude I. Astron. J. 11(248), 59–61 (1891)
Chen, W., Shen, W.: New estimates of the inertia tensor and rotation of the triaxial nonrigid earth. J. Geophys. Res. 115, B12419 (2010). doi:10.1029/2009JB007094
Desai, S.D.: Observing the pole tide with satellite altimetry. J. Geophys. Res. (Oceans) 107(C11), 1–7 (2002). doi:10.1029/2001JC001224
Escapa, A., Getino, J., Ferrándiz, J.M.: Indirect effect of the triaxiality in the Hamiltonian theory for the rigid Earth nutations. Astron. Astrophys. 389, 1047–1054 (2002)
Eubanks, T.M.: Variations in the orientation of the Earth. In: Smith, D.E., Turcott, D.L. (eds.) Contributions of Space Geodesy to Geodynamics: Earth Dynamics, pp. 1–54. AGU, Washington (1993)
Euler, L.: Du mouvement de rotation des corps solides autour d’un axe variable, Mém. Acad. Sci. Belles Lettres Berlin 14: 154–193 (1758), 154–193 (1765); reprinted in his Opera omniu. Ser. Secunda, Vol. 8, Orell Fussli Turici, Lausanne, 1965: 200–235 (1758/1765)
Euler, L.: Theoria motus corporum solidorum seu rigidorum: Ex primis nostrae cognitionis principiis stabilita et ad omnes motus, qui in huiusmodi corpora cadere possunt, accommodata, Rostochii et Gryphiswaldiae Ed (1765)
Folgueira, M., Souchay, J.: Free polar motion of a triaxial and elastic body in Hamiltonian formalism: Application to the Earth and Mars. Astron. Astrophys. 432, 1101–1113 (2005)
Gross, R.S.: The excitation of the Chandler wobble. Geophys. Res. Lett. 27(15), 2332–2392 (2000)
Gross, R.S.: Earth rotation variations long period. In: Herring, T.A. (ed.) Physical Geodesy Treatise on Geophysics, vol. 3. Elsevier, Oxford (2007)
IERS C04, Earth Rotation Parameters reference series downloaded from http://hpiers.obspm.fr/eop-pc (2012)
IERS Conventions (IERS Technical Note 36). Gérard Petit and Brian Luzum (eds.). http://tai.bipm.org/iers/conv2010/conv2010.html (2010)
IERS Global Geophysical Fluid Center. http://geophy.uni.lu/ (2012)
Jeffreys, H.: Causes contributory to the annual variation of latitude. Mon. Notices R. Astron. Soc. 76(6), 499–525 (1916)
Lambeck, K.: The Earth’s Variable rotation: Geophysical Causes and Consequences. Cambridge University Press, Cambridge (1980)
Liouville, J.: Développements sur un chapitre de la Mécanique de Poisson. J. de Math. Pures et Appl. Deuxième série, Tome 3, 1–25 (1858)
Markowitz, W.: Latitude and longitude and the secular motion of the pole. In: Runcorn, S.K. (ed.) Methods and Techniques in Geophysics 1, pp. 325–361. Interscience, New York (1960)
Mathews, P., Bretagnon, P.: Polar motions equivalent to high frequency nutations for a non rigid Earth with anelastic mantle. Astron. Astrophys. 400, 1113–1128 (2003)
Mathews, P., Herring, T., Buffett, B.: Modeling of nutation and precession: New nutation series from non rigid Earth and insights into the Earth interior. J. Geophys. Res. 107, B4 (2002)
Munk, W.H., MacDonald, G.: The rotation of the Earth. Cambridge University Press, Cambridge (1960)
Newcomb, S.: On the dynamics of the Earth’s rotation with respect to the periodic variations of latitude. Mon. Notices R. Astron. Soc. 248, 336–341 (1892)
Okamoto, I., Sasao, T.: On the ellipticity of the Chandler wobble. Publ. Astron. Soc. Jpn. 29, 107–127 (1977)
Seoane, L., Nastula, J., Bizouard, C., Gambis, D.: The use of gravimetric data from GRACE mission in the understanding of polar motion variations. J. Geophys. Int. 178, 614–622 (2009)
Wilson, C.: Discrete polar motion equation. Geophys. J. R. Astron. Soc. 80(2), 551–554 (1985)
Zhu, Y., Gao, B.: Dissipation and ellipticity of the Chandler wobble. Polar Motion Hist. Sci. Prob. 208, 473–479 (2000)
Zotov, L., Bizouard, C.: On modulations of the Chandler wobble excitation. J. Geodyn. (2012). doi:10.1016/j.jog.2012.03.010
Acknowledgments
The second author is supported by Chinese Academy of Sciences Fellowship for Young International Scientists and RFBR Grant 12-02-31184. We greatly thank the reviewers for their remarks and corrections. One of them, by doing a very careful review, and making us aware of the work of Okamoto and Sasao (1977), permitted us to refine this study.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Bizouard, C., Zotov, L. Asymmetric effects on Earth’s polar motion. Celest Mech Dyn Astr 116, 195–212 (2013). https://doi.org/10.1007/s10569-013-9483-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10569-013-9483-x