Skip to main content
SpringerLink
Log in

Problem Statements for k-Node Shortest Path and k-Node Shortest Cycle in a Complete Graph*

  • Published:
Cybernetics and Systems Analysis Aims and scope

Abstract

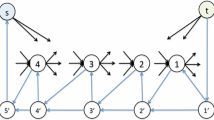
The author formulates mixed Boolean linear programming problems to find the shortest route and the shortest cycle that pass through the given number of nodes in a complete graph. Their special cases provide formulations of problems for finding the shortest Hamiltonian path and the shortest Hamiltonian cycle. The problems include no more than 2n2 variables and no more than (n + 1)2 constraints, where n is the number of nodes of the complete graph.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. N. Christofides, Graph Theory: An Algorithmic Approach, Acad. Press (1975).

  2. E. V. Alekseeva, Constructing Mathematical Models of Integer Linear Programming. Examples and Problems: A Handbook [in Russian], Novosibirsk. Gos. Univer., Novosibirsk (2012).

  3. Gurobi Optimization, Inc., Gurobi Optimizer Reference Manual (2014), http://www.gurobi.com.

Download references

Author information

Authors and Affiliations

  1. V. M. Glushkov Institute of Cybernetics, National Academy of Sciences of Ukraine, Kyiv, Ukraine

    P. I. Stetsyuk

Authors
  1. P. I. Stetsyuk
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to P. I. Stetsyuk.

Additional information

*The study was financially supported by the NAS of Ukraine (Projects No. 0113U003146 and No. 0112U002251).

Translated from Kibernetika i Sistemnyi Analiz, No. 1, January–February, 2016, pp. 78–82.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Stetsyuk, P.I. Problem Statements for k-Node Shortest Path and k-Node Shortest Cycle in a Complete Graph* . Cybern Syst Anal 52, 71–75 (2016). https://doi.org/10.1007/s10559-016-9801-x

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10559-016-9801-x

Keywords

Search

Navigation