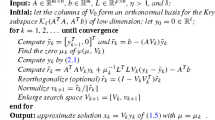Skip to main content
SpringerLink
Log in

A new zero-finder for Tikhonov regularization

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

Abstract

Tikhonov regularization with the regularization parameter determined by the discrepancy principle requires the computation of a zero of a rational function. We describe a cubically convergent zero-finder for this purpose.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. M. L. Baart, The use of auto-correlation for pseudo-rank determination in noisy ill-conditioned least-squares problems, IMA J. Numer. Anal., 2 (1982), pp. 241–247.

    Article  MATH  MathSciNet  Google Scholar 

  2. D. Calvetti and L. Reichel, Tikhonov regularization of large linear problems, BIT, 43 (2003), pp. 263–283.

    Article  MATH  MathSciNet  Google Scholar 

  3. J. E. Dennis and R. B. Schnabel, Numerical Methods for Unconstrained Optimization, SIAM, Philadelphia, 1996.

    MATH  Google Scholar 

  4. L. Eldén, Algorithms for the regularization of ill-conditioned least squares problems, BIT, 17 (1977), pp. 134–145.

    Article  MATH  Google Scholar 

  5. H. W. Engl, M. Hanke, and A. Neubauer, Regularization of Inverse Problems, Kluwer, Dordrecht, 1996.

    MATH  Google Scholar 

  6. W. Gander, On Halley’s iteration method, Am. Math. Mon., 92 (1985), pp. 131–134.

    Article  MATH  MathSciNet  Google Scholar 

  7. P. C. Hansen, Regularization tools: A MATLAB package for analysis and solution of discrete ill-posed problems, Numer. Algorithms, 6 (1994), pp. 1–35 (Software is available in Netlib at http://www.netlib.org.).

    Article  MATH  MathSciNet  Google Scholar 

  8. P. C. Hansen, Rank-Deficient and Discrete Ill-Posed Problems, SIAM, Philadelphia, 1998.

    Google Scholar 

  9. C. W. Groetsch, The Theory of Tikhonov Regularization for Fredholm Equations of the First Kind, Pitman, Boston, 1984.

    MATH  Google Scholar 

  10. J. J. Moré and D. C. Sorensen, Computing a trust-region step, SIAM J. Sci. Comput., 4 (1983), pp. 553–572.

    Article  MATH  Google Scholar 

  11. V. A. Morozov, Methods for Solving Incorrectly Posed Problems, Springer, New York, 1984.

    Google Scholar 

  12. C. H. Reinsch, Smoothing by spline functions II, Numer. Math., 16 (1971), pp. 451–454.

    Article  MathSciNet  Google Scholar 

  13. C. B. Shaw Jr., Improvements of the resolution of an instrument by numerical solution of an integral equation, J. Math. Anal. Appl., 37 (1972), pp. 83–112.

    Article  MATH  MathSciNet  Google Scholar 

  14. J. F. Traub, Iterative Methods for the Solution of Equations, Prentice-Hall, Englewood Cliffs, 1964.

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, Kent State University, Kent, OH, 44242, USA

    Lothar Reichel & Andriy Shyshkov

Authors
  1. Lothar Reichel
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Andriy Shyshkov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lothar Reichel.

Additional information

AMS subject classification (2000)

65F22, 65H05, 65R32

Rights and permissions

Reprints and permissions

About this article

Cite this article

Reichel, L., Shyshkov, A. A new zero-finder for Tikhonov regularization . Bit Numer Math 48, 627–643 (2008). https://doi.org/10.1007/s10543-008-0179-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10543-008-0179-7

Key words

Search

Navigation