Pricing mechanism of variable opaque products for dual-channel online travel agencies

Abstract

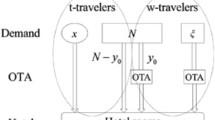
With the rapid development of e-commerce, an increasing number of online travel agencies (OTA) have combined traditional channels with opaque channels to maximise revenue, by implementing a price-discrimination strategy. Based on posted-price (PP) mechanism, this study reviews variable opaque products (VOPs) that allow travellers to change the opacity of an opaque channel to avoid unsatisfactory hotels. The purpose of the study is to determine how to optimise OTA revenue. Using a variation of the Hotelling model, this study examines the behaviour of travellers whose reservation values are relatively low. Additionally, we determine the optimal pricing strategy, which changes with the opacity of the opaque channel. The study shows that when the proportion of high-value travellers is low, OTA should decrease the price of transparent hotels; however, when the proportion is high, OTA should set a high price in the traditional channel, so that high-value travellers buy in the traditional channel, while low-value travellers buy in the opaque channel. And when the valuations differ greatly, OTA tend to increase the price of transparent hotels. Finally, when the number of travellers is constant, revenue increases when hotels increase, though there is an upper limit, and revenue decreases when the market expands. The results provide significant implications.

Appendices

Appendix A: Proofs of the piecewise function when N is large

If there are N hotels, and N is even.

For one leisure-traveller who is located at x, $0\le x\le \frac{\pi *D}{2N}$, we obtain the following if no hotels are removed:

$$\bar{V}=\frac{1}{N}\left(N{V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-1\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-1\right)\frac{\pi *D}{N}+x\right)+\left(\frac{N}{2}*\frac{\pi *D}{N}-x\right)\right)t\right)={V}_{L}-\frac{\pi *D*t}{4}$$

If the leisure-traveller removes one disliked hotel:

$$\bar{V}=\frac{1}{N-1}\left(\left(N-1\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-1\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-1\right)\frac{\pi *D}{N}+x\right)\right)t\right)={V}_{L}-\left(\frac{x}{N-1}+\frac{\left(N-2\right)*\pi *D}{4\left(N-1\right)}\right)t$$

If the leisure-traveller removes two disliked hotels:

$$\bar{V}=\frac{1}{N-2}\left(\left(N-2\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-2\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-2\right)\frac{\pi *D}{N}+x\right)+\left(\left(\frac{N}{2}-1\right)\frac{\pi *D}{N}-x\right)\right)t\right)={V}_{L}-\frac{\left(N-2\right)*\pi *D*t}{4N}$$

If the leisure-traveller removes three disliked hotels:

$$\bar{V}=\frac{1}{N-3}\left(\left(N-3\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-2\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-2\right)\frac{\pi *D}{N}+x\right)\right)t\right)={V}_{L}-\left(\frac{x}{N-3}+\frac{\left(N-2\right)\left(N-4\right)*\pi *D}{4N(N-3)}\right)t$$

If the leisure-traveller removes four disliked hotels:

$$\bar{V}=\frac{1}{N-4}\left(\left(N-4\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-3\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-3\right)\frac{\pi *D}{N}+x\right)+\left(\left(\frac{N}{2}-2\right)\frac{\pi *D}{N}-x\right)\right)t\right)={V}_{L}-\frac{\left(N-4\right)*\pi *D*t}{4N}$$

If the leisure-traveller removes five disliked hotels:

$$\bar{V}=\frac{1}{N-5}\left(\left(N-5\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-3\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-3\right)\frac{\pi *D}{N}+x\right)\right)t\right)={V}_{L}-\left(\frac{x}{N-5}+\frac{\left(N-4\right)\left(N-6\right)*\pi *D}{4N(N-5)}\right)t$$

If the leisure-traveller removes six disliked hotels:

$$\bar{V}=\frac{1}{N-6}\left(\left(N-6\right){V}_{L}-\left(x+\left(\frac{\pi *D}{N}-x\right)+\left(\frac{\pi *D}{N}+x\right)+\left(\frac{2\pi *D}{N}-x\right)+\left(\frac{2\pi *D}{N}+x\right)+\dots +\left(\left(\frac{N}{2}-4\right)\frac{\pi *D}{N}-x\right)+\left(\left(\frac{N}{2}-4\right)\frac{\pi *D}{N}+x\right)+\left(\left(\frac{N}{2}-3\right)\frac{\pi *D}{N}-x\right)\right)t\right)={V}_{L}-\frac{\left(N-6\right)*\pi *D*t}{4N}$$

In conclusion, the general formula is given by

$$\bar{V}=\left\{\begin{array}{c}{V}_{L}-\left(\frac{x}{N-i}+\frac{\left(N-\left(i-1\right)\right)\left(N-\left(i+1\right)\right)*\pi *D}{4N\left(N-i\right)}\right)t\,i \,is\, odd\\ {V}_{L}-\frac{\left(N-i\right)*\pi *D}{4N}t\,i\, is \,even\end{array}\right.$$

The calculation for an odd number of hotels is similar to when there is an even number of hotels; therefore, it is omitted here. The general formulas are symmetrical, whether the number of hotels is even or odd.□

Appendix B: Proofs of Proposition 1

Through Appendix A, we can find that the leisure-travellers’ valuation for the VOP with two hotels is ${\bar{V}}_{2}={V}_{L}-\frac{\pi {*}D}{2N}t$ $(0\le x\le \frac{\pi {*}D}{2N})$, which would not be impacted by the position of leisure-travellers in the circle city; the valuation of the VOP with three hotels is ${\bar{V}}_{3}={V}_{L}-\frac{1}{3}xt-\frac{2\pi {*}D}{3N}t$ $(0\le x\le \frac{\pi {*}D}{2N})$, which is decreasing in x. When $x=0$, ${\bar{V}}_{3}^{max}={V}_{L}-\frac{2\pi {*}D}{3N}t<{V}_{L}-\frac{\pi {*}D}{2N}t={\bar{V}}_{2}$. The derivation process for the valuation of other VOPs is similar, i.e., ${\bar{V}}_{2}>{\bar{V}}_{n}^{max}$, $n\ge 3$, that is, the leisure-travellers’ valuation of the VOP with two hotels is higher than any other type of VOPs. In order to maximize the revenue, the OTA will set the price for the VOP with two hotels as ${\bar{V}}_{2}$, and set the price of each other type of VOPs higher than the corresponding highest valuation, in order to make the leisure-travellers give up buying other types of VOPs and only buy the two hotels left on OTA. Therefore, ${p}_{2}^{{*}}={V}_{L}-\frac{\pi {*}D}{2N}t$, ${p}_{3}^{{*}}={V}_{L}-\frac{2\pi {*}D}{3N}t$, ${p}_{4}^{{*}}>{V}_{L}-\frac{\pi {*}D}{N}t$, ${p}_{5}^{{*}}={V}_{L}-\frac{6\pi {*}D}{5N}t$,${p}_{6}^{{*}}>{V}_{L}-\frac{3\pi {*}D}{2N}t$, ${p}_{7}^{{*}}={V}_{L}-\frac{12\pi {*}D}{7N}t$, ${p}_{8}^{{*}}>{V}_{L}-\frac{2\pi {*}D}{N}t$, and so on.□

Appendix C: Proofs of Proposition 2

When ${p}_{1}\in \left[{V}_{L},{V}_{H}\right]$, the optimal revenue is given by

$${{\Pi }}_{1}^{*}={D*\rho *V}_{H}+D*\left(1-\rho \right)*\left({V}_{L}-\frac{\pi *D}{2N}t\right)$$

When ${p}_{1}\in \left[{V}_{L}-\frac{\pi *D}{2N}t,{V}_{L}\right]$, the revenue is derived by

$${{\Pi }}_{2}=D*\rho *{p}_{1}+\frac{2N*D*(1-\rho )}{\pi *D}\left({p}_{1}*\frac{{V}_{L}-{p}_{1}}{t}+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\frac{{V}_{L}-{p}_{1}}{t}\right)\right)$$

The first order is as follows:

$$\frac{\partial {{\Pi }}_{2}}{\partial {p}_{1}}=0,{p}_{1}={V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t$$

The second order is as follows:

$$\frac{{\partial }^{2}{{\Pi }}_{2}}{\partial {p}_{1}^{2}}=-\frac{4N(1-\rho )}{\pi *t}<0$$

Since $\frac{{\partial }^{2}{{\Pi }}_{2}}{\partial {p}_{T}^{2}}<0$, maximum revenue can be obtained when

$${x}=\frac{{V}_{L}-{p}_{1}}{t}=\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)$$

Because $0\le x\le \frac{\pi *D}{2N}$, we can get that $V_{L} - \frac{{\pi *D}}{{2N}}t \le p_{1} \le V_{L} ,{\text{~}}0 \le \rho \le \frac{1}{2}$.

We obtain the optimal revenue

$${{\Pi }}_{2}^{*}=D*\rho {*p}_{1}+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\left({p}_{1}*\frac{{V}_{L}-{p}_{1}}{t}+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\frac{{V}_{L}-{p}_{1}}{t}\right)\right)=D*\rho *\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)+\frac{2N*D*(1-\rho )}{\pi *D}\left(\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)*\left(\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)\right)+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\left(\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)\right)\right)\right)$$

Then,${\Delta }{\Pi }={{\Pi }}_{2}^{*}-{{\Pi }}_{1}^{*}$

$${\Delta }{\Pi }=D{\rho }\left({V}_{L}-{V}_{H}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)+D\left(1-\rho \right)\left(-\frac{\pi *D*t}{8N}{\left(\frac{1-2\rho }{1-\rho }\right)}^{2}+\frac{\pi *D*t}{4N}\frac{1-2\rho }{1-\rho }\right)=D\left(\rho {V}_{L}-\rho {V}_{H}-\frac{\pi *D*t}{8N}\frac{{\left(1-2\rho \right)}^{2}}{\left(1-\rho \right)}-\frac{\rho *\pi *D*t}{4N}\frac{1-2\rho }{1-\rho }+\frac{\pi *D*t}{4N}\left(1-2\rho \right)\right)$$

Taking the derivative of ${\Delta }{\Pi }$ with respect of $\rho$

$$\frac{\partial {\Delta }{\Pi }}{\partial \rho }=N\left({V}_{L}-{V}_{H}-\frac{\pi *D*t}{8N}\frac{1-2\rho }{1-\rho }-\frac{\pi *D*t}{2N}\right)=N({V}_{L}-{V}_{H}-\frac{\pi *D*t*\left(2\rho -3\right)}{8N\left(1-\rho \right)})<0$$

We can conclude that $\Delta {\Pi }$ is a decreasing function of $\rho$, when $\rho =0,{\Delta }{\Pi }=\frac{\pi *{D}^{2}*t}{8N}>0$. When $\rho =\frac{1}{2},{\Delta }{\Pi }=\frac{D \left({V}_{L}-{V}_{H}\right)}{2}<0$.

Therefore, there must be $\bar{\rho }\in \left[0,\frac{1}{2}\right]$; when $\rho {\epsilon }\left[0,\bar{\rho }\right]$, ${\Delta }{\Pi }\ge 0$, when $\rho {\epsilon }\left[\bar{\rho },1\right]$, ${\Delta }{\Pi }\le 0$.□

Appendix D: Proofs of Corollary 4

From Case I and Case II, we can get that ${{\Pi }}_{1}^{D}={{\Pi }}_{1}^{*}={D*\rho *V}_{H}+D*\left(1-\rho \right)*\left({V}_{L}-\frac{\pi *D}{2N}t\right)$, ${{\Pi }}_{2}^{D}={{\Pi }}_{2}^{*}=D*\rho *\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)+\frac{2N*D*(1-\rho )}{\pi *D}\left(\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)*\left(\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)\right)+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\left(\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)\right)\right)\right)$.

When the OTA only adopts the traditional channel, we can get the following piecewise revenue function

:

$$\left\{\begin{array}{c}{{\Pi }}_{1}^{S}=D*\rho *{p}_{1}^{S}, if \,{{V}_{L}\le p}_{1}^{S}\le {V}_{H}\\ {{\Pi }}_{2}^{S}=D*\rho {*p}_{1}^{S}+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\frac{{V}_{L}-{p}_{1}^{S}}{t}{p}_{1}^{S}, if \,{V}_{L}\ge {p}_{1}^{S}\end{array}\right.$$

For ${{\Pi }}_{1}^{D}$ and ${{\Pi }}_{1}^{S}$, as long as ${V}_{L}-\frac{\pi *D}{2N}t\ge 0$ is satisfied, the dual channel is always better than the single channel, and the closer $\rho$ is to 1, the closer ${{\Pi }}_{1}^{S}$ is to ${{\Pi }}_{1}^{D}$.

For ${{\Pi }}_{2}^{D}$ and ${{\Pi }}_{2}^{S}$, $\frac{\partial {{\Pi }}_{2}^{S}}{\partial {p}_{1}^{S}}=D*\rho +\frac{2N\left(1-\rho \right)}{\pi *t}\left({V}_{L}-2{p}_{1}^{S}\right)=0,{p}_{1}^{S}=\frac{1}{2}{V}_{L}+\frac{D*\rho *\pi *t}{4N*(1-\rho )}$, $\frac{{\partial }^{2}{{\Pi }}_{2}^{S}}{\partial {\left({p}_{1}^{S}\right)}^{2}}<0$.

There are some leisure-travellers who will buy in the traditional channel, so ${p}_{1}^{S}<{V}_{L}$, ${p}_{1}^{D}<{V}_{L}$, $\rho <\frac{1}{2}.$ In order to introduce the opaque channel, we must ensure that ${{V}}_{{L}}-\frac{\pi *D}{2N}{t}\ge 0$.${p}_{1}^{D}-{p}_{1}^{S}={V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t-\left(\frac{1}{2}{V}_{L}+\frac{D*\rho *\pi *t}{4N*\left(1-\rho \right)}\right)=\frac{1}{2}{V}_{L}-\frac{\pi *D}{4N}t>0$, so we can get ${p}_{T}^{S}<{p}_{T}^{D}<{V}_{L}$. Because $x\le \frac{\pi *D}{2N}$, $\frac{{V}_{L}-{p}_{1}^{S}}{t}\le \frac{\pi *D}{2N}\to \frac{1}{2}{V}_{L}+\frac{D*\rho *\pi *t}{4N*\left(1-\rho \right)}\ge {V}_{L}-\frac{\pi *D}{2N}t$, so $\rho \ge 1-\frac{\pi *D*t}{2{V}_{L}*N-\pi *D*t},$ $1-\frac{\pi *D*t}{2{V}_{L}*N-\pi *D*t}<\frac{1}{2}\to {V}_{L}<\frac{3*\pi *D}{2N}t$. When ${V}_{L}<\frac{3*\pi *D}{2N}t$, the above proof results exist.□

$\Delta {\Pi }={{\Pi }}_{2}^{D}-{{\Pi }}_{2}^{S}=D*\rho *{p}_{1}^{D}+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\left({p}_{1}^{D}*\frac{{V}_{L}-{p}_{1}^{D}}{t}+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\frac{{V}_{L}-{p}_{1}^{D}}{t}\right)\right)-\left(D*\rho {*p}_{1}^{S}+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\frac{{V}_{L}-{p}_{1}^{S}}{t}{p}_{1}^{S}\right)=D*\rho *\left({p}_{1}^{D}-{p}_{1}^{S}\right)+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\left(\frac{{V}_{L}}{t}\left({p}_{1}^{D}-{p}_{1}^{S}\right)-\frac{\left({p}_{1}^{D}-{p}_{1}^{S}\right)\left({p}_{1}^{D}+{p}_{1}^{S}\right)}{t}+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\frac{{V}_{L}-{p}_{1}^{D}}{t}\right)\right)$, ${p}_{1}^{D}-{p}_{1}^{S}=\frac{1}{2}{V}_{L}-\frac{\pi *D}{4N}t$, $\Delta {\Pi }=\left({V}_{L}-\frac{\pi *D*t}{2N}\right)\left(\frac{\pi *D*t*\left(3+2\rho \right)-3\rho *t+2N*{V}_{L}*\left(\rho -1\right)}{4\pi *t}\right)$. We know that ${V}_{L}<\frac{3*\pi *D}{2N}t$ and ${V}_{L}-\frac{\pi *D}{2N}t>0$ from the above analysis, then we can derive that $\rho >\frac{2N*{V}_{L}-3\pi *D*t}{2N*{V}_{L}+2\pi *D*t-3t}$, so $\Delta {\Pi }>0$.□

Appendix E: Proofs of Proposition 3

From Appendix B we can get that ${\Delta }{\Pi }={{\Pi }}_{2}^{*}-{{\Pi }}_{1}^{*}$

$${\Delta }{\Pi }=D\left(\rho {V}_{L}-\rho {V}_{H}-\frac{\pi *D*t}{8N}\frac{{\left(1-2\rho \right)}^{2}}{\left(1-\rho \right)}-\frac{\rho *\pi *D*t}{4N}\frac{1-2\rho }{1-\rho }+\frac{\pi *D*t}{4N}\left(1-2\rho \right)\right)$$

Then making ${\Delta }{\Pi }=0$, it can be derived that $\frac{1-2\bar{\rho }}{\bar{\rho }}\left(\frac{1-2\bar{\rho }}{1-\bar{\rho }}+\frac{2\bar{\rho }}{1-\bar{\rho }}-2\right)=\frac{8N*\left({V}_{L}-{V}_{H}\right)}{\pi *D*t}$, ${\left(\bar{\rho }-\frac{1}{2}\right)}^{2}=\frac{2N\left({V}_{H}-{V}_{L}\right)}{8N\left({V}_{H}-{V}_{L}\right)+4\pi *D*t}$, for $\bar{\rho }\in \left[0,\frac{1}{2}\right]$, so $\frac{1}{2}-\bar{\rho }=\sqrt{\frac{2N\left({V}_{H}-{V}_{L}\right)}{8N\left({V}_{H}-{V}_{L}\right)+4\pi *D*t}}$, $\bar{\rho }=\frac{1}{2}-\sqrt{\frac{N\left({V}_{H}-{V}_{L}\right)}{4N\left({V}_{H}-{V}_{L}\right)+2\pi *D*t}}$.□

Appendix F: Proofs of Proposition 5

We know that ${{\Pi }}_{1}^{*}={D*\rho *V}_{H}+D*\left(1-\rho \right)*\left({V}_{L}-\frac{\pi *D}{2N}t\right)$ in Sect. 4, $\frac{\partial {{\Pi }}_{1}^{*}}{\partial D}=\rho *{V}_{H}+\left(1-\rho \right)*\left(1-\frac{\pi *D*t}{N}\right)$, when $D\le \frac{N}{\pi *t}*\left(1+\frac{\rho }{1-\rho }*{V}_{H}\right)$, $\frac{\partial {{\Pi }}_{1}^{*}}{\partial D}\ge 0$, ${{\Pi }}_{1}^{*}$ increases in D; while $D>\frac{N}{\pi *t}*\left(1+\frac{\rho }{1-\rho }*{V}_{H}\right)$, $\frac{\partial {{\Pi }}_{1}^{*}}{\partial D}<0$, ${{\Pi }}_{1}^{*}$ decreases in D, we know that the price in the opaque channel decreases in D, in order to get a positive price, we make ${p}_{2}^{*}={V}_{L}-\frac{\pi *D}{2N}t\ge 0, D\le \frac{2N*{V}_{L}}{\pi *t}.$ ${{\Pi }}_{2}^{*}=D*\rho *\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)+\frac{2N*D*\left(1-\rho \right)}{\pi *D}\left(\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)*\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)+\left({V}_{L}-\frac{\pi *D}{2N}t\right)\left(\frac{\pi *D}{2N}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)\right)\right)=D*\rho *\left({V}_{L}-\frac{\pi *D}{4N}\left(\frac{1-2\rho }{1-\rho }\right)t\right)+\left(\frac{D*\left(1-2\rho \right)}{2}*\left({V}_{L}-\frac{\pi *D*t*\left(1-2\rho \right)}{4N*\left(1-\rho \right)}\right)+\frac{D}{2}*\left({V}_{L}-\frac{\pi *D*t}{2N}\right)\right)=\frac{D}{2}*\left(2{V}_{L}-\frac{\pi *D*t*\left(2-3\rho \right)}{4N*\left(1-\rho \right)}\right)$, $\frac{\partial {{\Pi }}_{2}^{*}}{\partial D}={V}_{L}-\frac{\pi *D*t*\left(2-3\rho \right)}{4N\left(1-\rho \right)}$, when $D\le \frac{4N*{V}_{L}*\left(10\rho \right)}{\pi *t*\left(2-3\rho \right)}$, $\frac{\partial {{\Pi }}_{2}^{*}}{\partial D}\ge 0$, ${{\Pi }}_{2}^{*}$ increases in D; while $D>\frac{4N*{V}_{L}*\left(10\rho \right)}{\pi *t*\left(2-3\rho \right)}$, $\frac{\partial {{\Pi }}_{2}^{*}}{\partial D}<0$, ${{\Pi }}_{*}^{2}$ decreases in D. For positive price from both the traditional and opaque channel, we make ${p}_{1}^{*}\ge 0$ and ${p}_{2}^{*}\ge 0$, $D\le min\left(\frac{1-\rho }{1-2\rho }\frac{4N*{V}_{L}}{\pi *t}, \frac{2N*{V}_{L}}{\pi *t}\right)=\frac{2N*{V}_{L}}{\pi *t}.$□

Appendix G: Proofs of Corollary 5

As the city circle expands, the number of travellers also increases proportionally, namely, the distribution density of leisure-travellers remains unchanged. Here, only the circular arc length is calculated, which is equivalent to the increase in the number of travellers. The superscript “+” represents the situation after the city circle expansion. $\Delta {T}={\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{x}}^{+}-\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{x}=\frac{{V}_{L}-{p}_{1}^{+}}{t}-\frac{{V}_{L}-{p}_{1}}{t}=\frac{{V}_{L}-\frac{\pi *D*t*\left(1-2\rho \right)}{4N*\left(1-\rho \right)}-{V}_{L}+\frac{\pi *{D}^{+}*t*\left(1-2\rho \right)}{4N*\left(1-\rho \right)}}{t}=\frac{\pi *\left({D}^{+}-D\right)*\left(1-2\rho \right)}{4N*\left(1-\rho \right)}$, and $\Delta {O}=\frac{\pi *{D}^{+}}{2N}-\frac{\pi *D}{2N}-\left({\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{x}}^{+}-\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{x}\right)=\frac{\pi *\left({D}^{+}-D\right)}{4N}*\left(2-\frac{1-2\rho }{1-\rho }\right)=\frac{\pi *\left({D}^{+}-D\right)}{4N}*\left(\frac{1}{1-\rho }\right)$, then $\Delta {T}-\Delta {O}=\frac{\pi *\left({D}^{+}-D\right)}{4N}*\left(\frac{-2\rho }{1-\rho }\right)<0$, therefore $\Delta {T}<\Delta {O}$, and $\frac{\Delta {T}}{\Delta {O}}=1-2{\rho }$.□

Appendix H: Proofs of Proposition 6

From the above analysis, ${{\Pi }}_{2}^{*}=\frac{{D}_{T}}{2}*\left(2{V}_{L}-\frac{\pi *D*t*\left(2-3\rho \right)}{4N*\left(1-\rho \right)}\right)$, $\frac{\partial {{\Pi }}_{2}^{*}}{\partial D}=-\frac{\pi *{D}_{T}*t*\left(2-3\rho \right)}{8N*\left(1-\rho \right)}<0$, so ${{\Pi }}_{2}^{*}$ decreases in D.□