An equation for operators on varieties of completely regular semigroups

Abstract

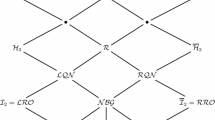
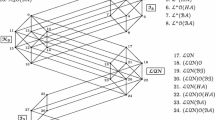
Completely regular semigroups ${\mathcal{CR}}$ with the unary operation of inversion form a variety with the lattice ${\mathcal{L(CR)}}$ of its subvarieties. The relation ${\mathbf{B}^{\wedge}}$ defined on ${\mathcal{L(CR)}}$ by ${\mathcal{U}\,\mathbf{B}^{\wedge}\,\mathcal{V}}$ if ${\mathcal{U}\,\cap\,\mathcal{B}\,=\,\mathcal{V}\cap\,\mathcal{B}}$, where ${\mathcal{B}}$ is the variety of all bands, and analogously defined ${\mathbf{B}^{\lor}}$, ${\mathbf{G}^{\wedge}}$ and ${\mathbf G^{\lor}}$, as well as the familiar relations ${\mathbf K}$,${\mathbf{T}}$, ${\mathbf{L}}$ and ${\mathbf{C}}$ on ${\mathcal{L(CR)}}$ induce decompositions of ${\mathcal{L(CR)}}$ and lower and upper operators all of which are useful in the study of the structure of ${\mathcal{L(CR)}}$. Manipulation of these operators is made effective by certain relationships among them.

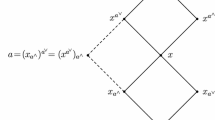
Beside possible commuting of some pairs of operators, a very useful formula is${\mathcal{V}^{X}\,=\,{(\mathcal{V}^{X})}_Y}$ for all or some ${\mathcal{V}}$ in a well defineddomain. Here the operators ${\mathcal{V}\,\mapsto\,\mathcal{V}_{X}}$ and ${\mathcal{V}\,\mapsto\,\mathcal{V}^{X}}$ are induced by an equivalence relation ${\mathbf{X}}$ on${\mathcal{L(CR)}}$ and ${[\mathcal{V}_{X},\,\mathcal{V}^{X}]}$ is an ${\mathbf{X}}$-class. We find several examples where the formula is valid on large domains and list those already known.