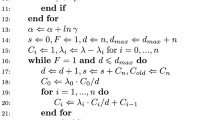Abstract
For continuous-state branching processes in Lévy random environments, the recursion of n-moments and the equivalent condition for the existence of general f-moments are established, where f is a positive continuous function satisfying some standard conditions.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Luschgy H, Pagès G. Moment estimates for Lévy processes. Electron Commun Prob, 2008, 13: 422–434
Li Z. Measure-Valued Branching Markov Processes. Heidelberg: Springer-Verlag, 2011
Gün O, König W, Sekulovió O. Moment asymptotics for branching random walks in random environment. Electron J Probab, 2013, 18(63): 1–18
Agarwal R, Hristova S, Kopanov P, O’regan D. Impulsive differential equations with Gamma distributed moments of impulses and p-moment exponential stability. Acta Mathematica Scientia, 2017, 37B(4): 985–997
Qiu D, Chen P, Andrei V. Complete moment convergence for LP-mixingales. Acta Mathematica Scientia, 2017, 37B(5): 1319–1330
Zhao Y. Asymptotic properties of the moment convergence for NA sequences. Acta Mathematica Scientia, 2014, 34B(2): 301–312
Barczy M, Li Z, Pap G. Moment formulas for multitype continuous state and continuous time branching process with immigration. J Theor Probab, 2016, 29(3): 958–995
Ji L, Li Z. Moments of continuous-state branching processes with or without immigration. 2017[2018-03-31]. https://arxiv.org/abs/1702.08698
Jirina M. Stochastic branching processes with continuous state space. Czech Math J, 1958, 8: 292–313
Bansaye V, Millan J C P, Smadi C. On the extinction of continuous state branching processes with catastrophes. Electron J Probab, 2013, 18(106): 1–31
Palau S, Pardo J C. Continuous state branching processes in random environment: the Brownian case. Stoch Process Appl, 2017, 127: 957–994
He H, Li Z, Xu W. Continuous-state branching processes in Lévy random environments. J Theor Probab, 2017[2018-03-31]. https://doi.org/10.1007/s10959-017-0765-1
Palau S, Pardo J C. Branching processes in a Lévy random environment. Acta Appl Math, 2018, 153(1): 55–79
Dawson D A, Li Z. Stochastic equations, flows and measure-valued processes. Ann Probab, 2012, 40(2): 813–857
Caballero M E, Lambert A, Uribe Bravo G. Proof(s) of the Lamperti representation of continuous-state branching processes. Probab Surv, 2009, 6: 62–89
Sato K I. Lévy Processes and Infinitely Divisible Distributions. Cambridge University Press, 1999
Athreya K B, Ney P E. Branching Processes. Berlin: Springer, 1972
Acknowledgements
Heartfelt thanks are given to Professor Zenghu Li for his careful guidance and helpful suggestions.
Author information
Authors and Affiliations
Corresponding authors
Additional information
The authors are supported by the National Natural Science Foundation of China (11531001).
Rights and permissions
About this article
Cite this article
Ji, L., Zheng, X. Moments of Continuous-State Branching Processes in Lévy Random Environments. Acta Math Sci 39, 781–796 (2019). https://doi.org/10.1007/s10473-019-0310-7
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10473-019-0310-7