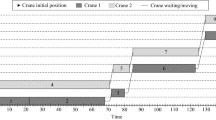
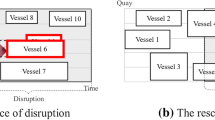
Abstract
The ever increasing demand for container transportation has led to the congestion of maritime container terminals in the world. In this work, the two interrelated problems of berth and quay crane scheduling are considered in an integrated multiobjective mathematical model. A special character of this model is that the arrival times of vessels and the failure (working) times of quay cranes are not deterministic and can vary based on some scenarios. Hence, a robust model is devised for the problem having three objectives of minimising the deviations from target berthing locations and times as well as departure delays of all vessels. This robust optimisation seeks to minimise the value of the objectives regarding all the scenarios. An exact solution approach based on the 𝜖-constraint method by the Gurobi software is applied. Moreover, regarding the complexity of the problem, two Simulated Annealing (SA) based metaheuristics, namely a Multi-Objective Simulated Annealing (MOSA) and a Pareto Simulated Annealing (PSA) approach are adapted with a novel solution encoding scheme. The three methods are compared based on some multiobjective metrics and a statistical test. The advantage of the integration of berth and quay crane scheduling is examined as well.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Gurobi optimization, https://www.gurobi.com
UNCTAD: Review of maritime transport. In: United Nations conference on trade and development, p 2018 (2020)
Amine, K.: Multiobjective simulated annealing: Principles and algorithm variants. Adv. Oper. Res. 2019, 1–13 (2019)
Ben-Tal, A., Nemirovski, A.: Robust solutions of uncertain linear programs. Oper. Res. Lett. 25(1), 1–13 (1999)
Bergmann, B., Hommel, G.: Improvements of general multiple test procedures for redundant systems of hypotheses. In: Multiple Hypothesenprüfung / Multiple Hypotheses Testing, pp. 100–115. Springer, Berlin (1988)
Bierwirth, C., Meisel, F.: A survey of berth allocation and quay crane scheduling problems in container terminals. Eur. J. Oper. Res. 202(3), 615–627 (2010)
Box, G.E.P., Draper, N.R.: Response Surfaces, Mixtures, and Ridge Analyses, 2nd edn. Wiley-Interscience, Hoboken (2007)
Buhrkal, K., Zuglian, S., Ropke, S., Larsen, J., Lusby, R.: Models for the discrete berth allocation problem: a computational comparison. Transp. Res. E Logist. Transp. Rev. 47(4), 461–473 (2011)
Cahyono, R. T., Flonk, E. J., Jayawardhana, B.: Discrete-event systems modeling and the model predictive allocation algorithm for integrated berth and quay crane allocation. IEEE Trans. Intell. Transp. Syst. 21(3), 1321–1331 (2020)
Chankong, V., Making, Y. Y. Haimes.: Multiobjective Decision Theory and Methodology. Elsevier Science, New York (1983)
Correcher, J. F., Alvarez-Valdes, R., Tamarit, J. M.: New exact methods for the time-invariant berth allocation and quay crane assignment problem. Eur. J. Oper. Res. 275(1), 80–92 (2019)
Correcher, J. F., Van den Bossche, T., Alvarez-Valdes, R., Vanden Berghe, G.: The berth allocation problem in terminals with irregular layouts. Eur. J. Oper. Res. 272(3), 1096–1108 (2019)
Cunha, M., annealing, J. Marques.: A new multiobjective simulated algorithm—MOSA-GR: Application to the optimal design of water distribution networks. Water Resour. Res. 56(3), 2019wr025852 (2020)
Derrac, J., García, S., Molina, D., Herrera, F.: A practical tutorial on the use of nonparametric statistical tests as a methodology for comparing evolutionary and swarm intelligence algorithms. Swarm Evol. Comput. 1(1), 3–18 (2011)
Elwany, M.H., Ali, I., Abouelseoud, Y.: A heuristics-based solution to the continuous berth allocation and crane assignment problem. Alexandria Eng. J. 52(4), 671–677 (2013)
Fatemi-Anaraki, S., Tavakkoli-Moghaddam, R., Abdolhamidi, D., Vahedi-Nouri, B.: Simultaneous waterway scheduling, berth allocation, and quay crane assignment: A novel matheuristic approach. Int. J. Prod. Res., 1–18 (2020)
Gutierrez, F., Lujan, E., Asmat, R., Vergara, E.: A fully fuzzy linear programming model for berth allocation and quay crane assignment. In: Advances in Artificial Intelligence - IBERAMIA 2018, pp. 302–313. Springer International Publishing (2018)
Hu, Q.-M., Hu, Z.-H., Du, Y.: Berth and quay-crane allocation problem considering fuel consumption and emissions from vessels. Comput. Ind. Eng. 70, 1–10 (2014)
Idris, N., Zainuddin, Z.M.: A simultaneous integrated model with multiobjective for continuous berth allocation and quay crane scheduling problem. In: 2016 International Conference on Industrial Engineering, Management Science and Application (ICIMSA), pp. 1–5 (2016)
Karafa, J., Golias, M.M., Ivey, S., Saharidis, G.K.D., Leonardos, N.: The berth allocation problem with stochastic vessel handling times. Int. J. Adv. Manuf. Technol. 65(1-4), 473–484 (2012)
Karam, A., Eltawil, A.B.: Functional integration approach for the berth allocation, quay crane assignment and specific quay crane assignment problems. Comput. Ind. Eng. 102, 458–466 (2016)
Kasm, O.A., Diabat, A., Cheng, T.C.E.: The integrated berth allocation, quay crane assignment and scheduling problem: mathematical formulations and a case study. Ann. Oper. Res. 291(1-2), 435–461 (2019)
Kirkpatrick, S.: Optimization by simulated annealing: Quantitative studies. J. Stat. Phys. 34, 975–986 (1984)
König, E.: A review on railway delay management. Public Transp. 12, 335–361 (2020)
Kramer, A., Lalla-Ruiz, E., Iori, M., Voß, S.: Novel formulations and modeling enhancements for the dynamic berth allocation problem. Eur. J. Oper. Res. 278(1), 170–185 (2019)
Krimi, I., Todosijeviċ, I., Benmansour, R., Ratli, M., El Cadim, A.A., Aloullal, A.: Modelling and solving the multi-quays berth allocation and crane assignment problem with availability constraints. J. Glob. Optim. (2020)
Lalla-Ruiz, E., Voß, S.: POPMUSIC as a matheuristic for the berth allocation problem. Ann. Math. Artif. Intell. 76(1-2), 173–189 (2014)
Legato, P., Mazza, R. M., Gullì, D.: Integrating tactical and operational berth allocation decisions via simulation–optimization. Comput. Ind. Eng. 78, 84–94 (2014)
Li, Y., Chu, F., Zheng, F., Liu, M.: A bi-objective optimization for integrated berth allocation and quay crane assignment with preventive maintenance activities. IEEE Trans. Intell. Transp. Syst., 1–18 (2020)
Ma, S., Li, H., Zhu, N., Fu, C.: Stochastic programming approach for unidirectional quay crane scheduling problem with uncertainty. J. Sched. (2020)
Mattila, V., Virtanen, K., Hämäläinen, R.sP.: A simulated annealing algorithm for noisy multiobjective optimization. J. Multi-Criteria Decis. Anal. 20(5-6), 255–276 (2012)
Nguyen, S., Zhang, M, Johnston, M., Tan, K.C.: Hybrid evolutionary computation methods for quay crane scheduling problems (2020)
Nourmohammadzadeh, A., Hartmann, S.: An efficient simulated annealing for the integrated problem of berth allocation and quay crane assignment in seaside container terminals. In: 7th Multidisciplinary International Conference on Scheduling: Theory and Applications (MISTA 2015), pp. 154–164 (2015)
Nourmohammadzadeh, A., Voß, S.: A pareto simulated annealing for the integrated problem of berth and quay crane scheduling at maritime container terminals with multiple objectives and stochastic arrival times of vessels. In: Kotsireas, I.S., Pardalos, P.M. (eds.) Learning and Intelligent Optimization, pp. 324–340. Springer International Publishing, Cham (2020)
R Development Core Team: R: A Language and Environment for Statistical Computing. R Foundation for Statistical Computing, Vienna. ISBN 3-900051-07-0 (2008)
Rashidi, H., Tsang, E.P.K.: Novel constraints satisfaction models for optimization problems in container terminals. Appl. Math. Model. 37(6), 3601–3634 (2013)
Riquelme, N., Von Lücken, C., Baran, B.: Performance metrics in multi-objective optimization. In: 2015 Latin American Computing Conference (CLEI), pp. 1–11 (2015)
Rodriguez-Molins, M., Salido, M.A., Barber, F.: A GRASP-based metaheuristic for the berth allocation problem and the quay crane assignment problem by managing vessel cargo holds. Appl. Intell. 40(2), 273–290 (2013)
Saha, S., Bandyopadhyay, S.: A new multiobjective simulated annealing based clustering technique using symmetry. Pattern Recogn. Lett. 30(15), 1392–1403 (2009)
Serafini, P.: Simulated annealing for multi objective optimization problems. In: Multiple Criteria Decision Making, pp. 283–292. Springer, New York (1994)
Stahlbock, R., Voß, S.: Operations research at container terminals : a literature update. OR Spectr. 30, 1–52 (2008)
Tan, C., He, J., Wang, Y.: Berth allocation and quay crane assignment under uncertainties. In: Advances in Transdisciplinary Engineering. IOS Press (2020)
Tavakkoli-Moghaddam, R., Makui, A., Salahi, S., Bazzazi, M., Taheri, F.: An efficient algorithm for solving a new mathematical model for a quay crane scheduling problem in container ports. Comput. Ind. Eng. 56(1), 241–248 (2009)
Tekinalp, O., Karsli, G.: A new multiobjective simulated annealing algorithm. J. Glob. Optim. 39(1), 49–77 (2006)
Theofanis, S., Boile, M.: Empty marine container logistics: facts, issues and management strategies. GeoJournal 74(1), 51–65 (2008)
Ting, C.-J., Wu, K.-C., Chou, H.: Particle swarm optimization algorithm for the berth allocation problem. Expert Syst. Appl. 41(4), 1543–1550 (2014)
Türkoğulları, Y.B., Taşkın, Z.C., Aras, N., Altınel, İ.K.: Optimal berth allocation, time-variant quay crane assignment and scheduling with crane setups in container terminals. Eur. J. Oper. Res. 254(3), 985–1001 (2016)
Türkoğulları, Y.B., Taşkın, Z.C., Aras, N., Altınel, İ.K.: Optimal berth allocation and time-invariant quay crane assignment in container terminals. Eur. J. Oper. Res. 235(1), 88–101 (2014)
Wang, T., Du, Y., Fang, D., Li, Z.-C.: Berth allocation and quay crane assignment for the trade-off between service efficiency and operating cost considering carbon emission taxation. Transp. Sci. 54(5), 1307–1331 (2020)
Wawrzyniak, J., Drozdowski, M., Sanlaville, É.: Selecting algorithms for large berth allocation problems. Eur. J. Oper. Res. 283(3), 844–862 (2020)
Xi, X., Liu, C., Lixin, M.: A bi-objective robust model for berth allocation scheduling under uncertainty. Transp. Res. E Logist. Transp. Rev. 106, 294–319 (2017)
Xu, D., Li, C.-L., Leung, J.Y.-T.: Berth allocation with time-dependent physical limitations on vessels. Eur. J. Oper. Res. 216(1), 47–56 (2012)
Zhen, L., Chang, D.-F.: A bi-objective model for robust berth allocation scheduling. Comput. Ind. Eng. 63(1), 262–273 (2012)
Zheng, F., Qiao, L., Liu, M.: An online model of berth and quay crane integrated allocation in container terminals. In: Combinatorial Optimization and Applications, pp. 721–730. Springer International Publishing (2015)
Zitzler, E., Thiele, L.: Multiobjective evolutionary algorithms: a comparative case study and the strength Pareto approach. IEEE Trans. Evol. Comput. 3(4), 257–271 (1999)
Funding
Open Access funding enabled and organized by Projekt DEAL.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Nourmohammadzadeh, A., Voß, S. A robust multiobjective model for the integrated berth and quay crane scheduling problem at seaside container terminals. Ann Math Artif Intell 90, 831–853 (2022). https://doi.org/10.1007/s10472-021-09743-5
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10472-021-09743-5