A Viability Analysis of Fishery Controlled by Investment Rate

Abstract

This work presents a stock/effort model describing both harvested fish population and fishing effort dynamics. The fishing effort dynamic is controlled by investment which corresponds to the revenue proportion generated by the activity. The dynamics are subject to a set of economic and biological state constraints. The analytical study focuses on the compatibility between state constraints and controlled dynamics. By using the mathematical concept of viability kernel, we reveal situations and management options that guarantee a sustainable system.

Appendix

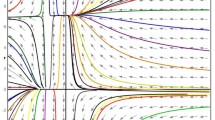
In all this section, we denote (X ₀, E ₀) an initial condition of the system (1).

1.1 Proof of Proposition 1

• 1. If (X ₀, E ₀) is in the area R4.

  • (a) If (X ₀, E ₀) is above ${{\mathcal{T}}_3}$ then, any trajectory starting at this point will decrease with respect to X and increase with respect to E for any 0 < α ≤ 1 until it crosses nullcline ${\dot{E}}=0$. Therefore, it will cross line E = E _max and then the corresponding trajectory won’t stay in the set of constraints ${{\mathcal{K}}}$, which means that we have to consider an initial condition under ${{\mathcal{T}}_3}$.

  • (b) If (X ₀, E ₀) is under ${{\mathcal{T}}_3}$ , for any 0 < α ≤ 1. The corresponding trajectory will decrease with respect to X and increase with respect to E until it crosses nullcline ${\dot{E}}=0$ and then enters in area R1.

• 2. If (X ₀, E ₀) in the area R3.

  • (a) If (X ₀, E ₀) is above $\mathcal{T}_2$, for any 0 < α ≤ 1. The corresponding trajectory will decrease with respect to X and E until it crosses line X = X _min and leaves constraint domain. Then, (X ₀,E ₀) must be choosen under ${{\mathcal{T}}_2}$.

  • (b) If (X ₀, E ₀) is under ${{\mathcal{T}}_2}$, the corresponding trajectory will decrease with respect to X and E until it crosses nullcline ${\dot{X}}=0$ and enter in area R2.

• 3. If (X ₀, E ₀) in the area R2.

  • (a) If (X ₀, E ₀) is underneath ${{\mathcal{T}}_1}$, for any 0 < α ≤ 1. The corresponding trajectory will decrease with respect to E and increase with respect to X until it crosses line X = X _min and leaves constraint domain. In this case, (X ₀, E ₀) have to be choosen under ${{\mathcal{T}}_1}$.

  • (b) If (X ₀, E ₀) is above ${{\mathcal{T}}_1}$, for any 0 < α ≤ 1. The corresponding trajectory will continue decreasing with respect to E and to X until it crosses nullcline ${\dot{E}}=0$ and enters in area R1.

• 4. Finally, if (X ₀, E ₀) is in area R1, at the top of E = E _min , for all 0 < α ≤ 1. The corresponding trajectory will increase with respect to X and E until it crosses nullcline ${\dot{X}}=0$ and enters in area R4.

1.2 Proof of Proposition 2

• 1. If (X ₀,E ₀) is above ${\dot{X}}=0$, for any −1 ≤ α < 0. The corresponding trajectory will decrease with respect to X and E. But before to reach ${\dot{X}}=0$,, depending on the initial condition position with respect to C ₂(X) , it changes direction either with respect to X or E and leaves the constraint domain.

• 2. if (X ₀, E ₀) is under ${\dot{X}}=0$ , for any −1 ≤ α < 0. The trajectory starting at (X ₀, E ₀) will increase with respect to X and E towards ${\dot{X}}=0$. As in previous case the same argument is valid by substituting C ₂(X) by C ₁(X).

• 3. If (X ₀, E ₀) on C ₁(X) , (resp. C ₂(X)) the corresponding trajectory will increase with respect to X and E (resp. decrease with respect to X and E) until it reaches nullcline ${\dot{x}}=0$.

1.3 Proof of Proposition 3

• 1. If $E_0>{\bar{E}}$ , for α = 0. The corresponding trajectory will decrease with respect to X and remain constant with respect to E until it crosses ${\dot{X}}=0$. But before it crosses line E = E _min it leaves set constraint K.

• 2. If $E_{min}\leq E_0\leq{\bar{E}}$. If (X ₀, E ₀) is over ${\dot{X}}=0$, (resp.under ${\dot{X}}=0$,) then the trajectory starting from (X ₀, E ₀) decreases (resp. increases) with respect to X and remains constant with respect to E until it reaches null-cline ${\dot{x}}=0$ and remains there.

1.4 Proof of Proposition 4

• 1. If (X ₀,E ₀) is in area A1. In this area α(t) = 0 corresponding trajectory will increase with respect to X and remain constant with respect to E until crossing nullcline ${\dot{X}}=0$ and staying there.

• 2. If (X ₀,E ₀) is in area A2. In this area −1 ≤ α(t) ≤ 1. Over C ₁ fisherman can choose to not invests and the corresponding trajectory will increase with respect to X and remain constant with respect to E until reaching nullcline ${\dot{X}}=0$. Or, he can also choose positive investment 0 < α ≤ 1, the corresponding trajectory will increase with respect to X and decrease with respect to E until reaching C ₁. Then, either he will change strategy by taking negative investment and remain on C ₁ or continue with positive investment until to enter in area A3.

• 3. If (X ₀,E ₀) is in area A3. In this area 0 ≤ α(t) ≤ 1. If fisherman chooses to not invests, then the corresponding trajectory will remain constant with respect to E and will increase or decrease with respect to X , depending on (X ₀,E ₀) position with respect to nullcline ${\dot{X}}=0$ , until reaching nullcline ${\dot{X}}=0$. If he chooses a positive investment, the corresponding trajectory will increase with respect to E and either increase or decrease with respect to X depending on (X ₀,E ₀) position with respect to nullcline ${\dot{X}}=0$ until to enter in area A6.

• 4. If (X ₀,E ₀) is in area A4. Then, in this area α = 0. We have the same case as in area A3 when fisherman chooses to not invest and the initial condition is above ${\dot{X}}=0$.

• 5. If (X ₀,E ₀) is in area A5. In this area 0 ≤ α ≤ 1. If α(t) = 0 the argument is valid as in area A4. Otherwise, if 0 < α ≤ 1, the corresponding trajectory will enter either in area A6 or A2 depending on (X ₀,E ₀) position with respect to X = X ^*.

• 6. If (X ₀,E ₀) is in area A6. In this area 0 < α ≤ 1, the corresponding trajectory will decrease with respect to X and increase or decrease with respect to E depending on (X ₀,E ₀) position and enters in area A2.

• 7. If (X ₀,E ₀) is on curve C ₂. Above ${{\mathcal{T}}_3}$ , the corresponding trajectory will decrease with respect to X and E until it reaches ${{\mathcal{T}}_3}$. Then, fisherman can choose to continue with negative investment and stays on C ₂ until to reach ${\dot{X}}=0$, or he can chooses to invest and enters in area A6.

• 8. If (X ₀,E ₀) in area A0 for any −1 ≤ α(t) ≤ 1. The corresponding trajectory starting at this point will decrease with respect to X and increases, decreases or remains constant with respect to E according to α sign, until reaching nullcline ${\dot{X}}=0$. But before that it crosses line E = E _min and leaves constraints set.