Skip to main content

Advertisement

SpringerLink
Log in

Random Modelling of Contagious Diseases

  • Regular Article
  • Published:
Acta Biotheoretica Aims and scope Submit manuscript

Abstract

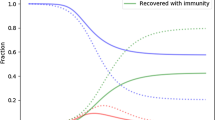
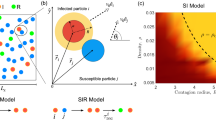
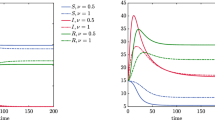
Modelling contagious diseases needs to include a mechanistic knowledge about contacts between hosts and pathogens as specific as possible, e.g., by incorporating in the model information about social networks through which the disease spreads. The unknown part concerning the contact mechanism can be modelled using a stochastic approach. For that purpose, we revisit SIR models by introducing first a microscopic stochastic version of the contacts between individuals of different populations (namely Susceptible, Infective and Recovering), then by adding a random perturbation in the vicinity of the endemic fixed point of the SIR model and eventually by introducing the definition of various types of random social networks. We propose as example of application to contagious diseases the HIV, and we show that a micro-simulation of individual based modelling (IBM) type can reproduce the current stable incidence of the HIV epidemic in a population of HIV-positive men having sex with men (MSM).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  • Allen L (2008) An introduction to stochastic epidemic models. Mathematical Epidemiology 1945:81–130

    Article  Google Scholar 

  • Amarasekare P (1998) Interactions between local dynamics and dispersal: insights from single species models. Theor Popul Biol 53(44):59

    Google Scholar 

  • Arino J, van den Driessche P (2003) The basic reproduction number in a multi-city compartmental epidemic model. LNCIS 294:135–142

    Google Scholar 

  • Artalejo AJR, Economou A, Lopez-Herrero MJ (2010) On the number of recovering individuals in the SIS and SIR stochastic epidemic models. Math Biosci 228:45–55

    Article  Google Scholar 

  • Auvert B, Taljaard D, Lagarde E, Sobngwi-Tambekou J, Sitta JR, Puren A (2005) Randomized, controlled intervention trial of male circumcision for reduction of HIV infection risk: the ANRS 1265 Trial. PLoS Med 2:e298

    Article  Google Scholar 

  • Bahar A, Mao X (2004) Stochastic delay Lotka–Volterra model. J Math Anal Appl 292:364–380

    Article  Google Scholar 

  • Bailey NTJ (1963) The simple stochastic epidemic: a complete solution in terms of known functions. Biometrika 50:235–240

    Google Scholar 

  • Bailey RC, Moses S, Parker CB, Agot K, Maclean I, Krieger JN, Williams CF, Campbell RT, Ndinya-Achola JO (2007) Male circumcision for HIV prevention in young men in Kisumu, Kenya: a randomised controlled trial. Lancet 369:643–656

    Article  Google Scholar 

  • Ball F, Neal P (2002) A general model for stochastic SIR epidemics with two levels of mixing. Math Biosci 180:73–102

    Article  Google Scholar 

  • Barreira L, Valls C (2010) Stability of delay equations via Lyapunov functions. J Math Anal Appl 365:797–805

    Article  Google Scholar 

  • Barth J (2002) What should we do about the obesity epidemic? Pract Diabetes Int 19:119–122

    Article  Google Scholar 

  • Bartholomay AF (1958a) On the linear birth and death processes of biology as Markoff chains. Bull Math Biophys 20:97–118

    Article  Google Scholar 

  • Bartholomay AF (1958b) Stochastic models for chemical reactions: I. Theory of the unimolecular reaction process. Bull. Math. Biophys. 20:175–190

    Article  Google Scholar 

  • Bartholomay AF (1959) Stochastic models for chemical reactions: II. The unimolecular rate constant. Bull Math Biophys 21:363–373

    Article  Google Scholar 

  • Beier JC (1998) Malaria parasite development in mosquitoes. Ann Rev Entomol 43:519–543

    Article  Google Scholar 

  • Ben Amor H, Demongeot J, Elena A, Sené S (2008) Structural sensitivity of neural and genetic networks. Lect Notes Comput Sci 5317:973–986

    Article  Google Scholar 

  • Beretta E, Capasso V (1986) Global stability results for a multi-group SIR epidemic model. In: Hallam TG, Gross IJ, Levin SA (eds) Mathematical ecology. World Scientific, Singapore, pp 317–342

    Google Scholar 

  • Beretta E, Hara T, Ma W, Takeuchi Y (2001) Global asymptotic stability of a SIR epidemic model with distributed time delay. Nonlinear Anal 47:4107–4115

    Article  Google Scholar 

  • Bernoulli D (1760) Essai d’une nouvelle analyse de la mortalité causée par la petite vérole, et des avantages de l’inoculation pour la prévenir. Mémoire Acad Roy Sci, Paris

  • Bochner S (1933) Abstrakte fastperiodische Funktionen. Acta Mathematica 61:150–184

    Article  Google Scholar 

  • Bouyssou A, Janier M, Dupin N, Alcaraz I, Vernay-Vaïsse C, Basselier B, Spenatto N, Dhotte P, Castano F, Semaille C, Gallay A (2011) La syphilis en France: analyse des données de surveillance sur 10 ans, Bulletin épidémiologique hebdomadaire 26-27-28:295–297

  • Bricault G (2008) Naissance d’un ordre hospitalier. Publication AFAA, Grenoble

    Google Scholar 

  • Britton T (2010) Stochastic epidemic models: a survey. Math Biosci 225:24–35

    Article  Google Scholar 

  • Brownlee J (1915) On the curve of the epidemic. Br Med J 1:799–800

    Article  Google Scholar 

  • Caputo JG, Sarels B (2011) Reaction-diffusion front crossing, a local defect. Phys Rev E 84:041108

    Article  Google Scholar 

  • Charlebois ED, Das M, Porco TC, Havlir DV (2011) The effect of expanded antiretroviral treatment strategies on the HIV epidemic among men who have sex with men in San Francisco. Clin Infect Dis 52:1046–1049

    Article  Google Scholar 

  • Christakis N, Fowler J (2006) The spread of obesity in a large social network over 32 years. N Engl J Med 355:77–82

    Google Scholar 

  • Clerc M, Gallay A, Imounga L, Le Roy C, Peuchant O, Bébéar C, Goulet V, Barbeyrac B (2011) Évolution du nombre de lymphogranulomatoses vénériennes rectales et d’infections rectales à Chlamydia trachomatis à souches non L en France entre 2002 et 2009. Bul Epidémiol Hebd 26–28:310–313

    Google Scholar 

  • Cohen-Cole E, Fletcher JM (2008) Is obesity contagious? Social networks vs. environmental factors in the obesity epidemic. J Health Econ 27:1382–1387

    Article  Google Scholar 

  • Cori A (2010) Modéliser l’hétérogénéité dans les épidémies: aspects biologiques et comporte-mentaux. PhD Thesis. University Paris VI—Pierre et Marie Curie

  • Dalal N, Greenhalgh D, Mao X (2007) A stochastic model of AIDS and condom use. J Math Anal Appl 325:36–53

    Article  Google Scholar 

  • d’Alembert J (1761) Onzième memoire: sur l’application du calcul des probabilités à l’inoculation de la petite vérole; notes sur le mémoire précédent; théorie mathématique de l’inoculation. In: Opuscules mathématiques. David, Paris, t. II, pp 26–95

  • de Saint-Pol T (2008) Obésité et milieux sociaux en France: les inégalités augmentent. Bull Epidemiol Hebdom 20:175–179

    Google Scholar 

  • Delbrück M (1940) Statistical fluctuations in autocatalytic reactions. J Chem Phys 8:120–124

    Article  Google Scholar 

  • Demongeot J (1977) A stochastic model for the cellular metabolism. In: Recent developments in statistics. North Holland, Amsterdam, pp 655–662

  • Demongeot J (1981) Existence de solutions périodiques pour une classe de systèmes différentiels gouvernant la cinétique de chaînes enzymatiques oscillantes. Lect Notes Biomath 41:40–62

    Google Scholar 

  • Demongeot J, Fricot J (1986) Random fields and renewal potentials. NATO ASI Ser F 20:71–84

    Google Scholar 

  • Demongeot J, Kellershohn N (1983) Glycolytic oscillations: an attempt to an “in vitro” reconstitution of the higher part of glycolysis. Lect Notes Biomath 49:17–31

    Article  Google Scholar 

  • Demongeot J, Laurent M (1983) Sigmoidicity in allosteric models. Math Biosci 67:1–17

    Article  Google Scholar 

  • Demongeot J, Sené S (2011) The singular power of the environment on nonlinear Hopfield networks. In: CMSB’11. ACM proceedings, New York, pp 55–64

  • Demongeot J, Waku J (2012) Robustness in genetic regulatory networks, IV. Comptes Rendus Mathématique 350:293–298

    Article  Google Scholar 

  • Demongeot J, Elena A, Sené S (2008) Robustness in neural and genetic networks. Acta Biotheor 56:27–49

    Article  Google Scholar 

  • Demongeot J, Drouet E, Moreira A, Rechoum Y, Sené S (2009) Micro-RNAs: viral genome and robustness of the genes expression in host. Phil Trans R Soc A 367:4941–4965

    Article  Google Scholar 

  • Demongeot J, Elena A, Noual M, Sené S (2011) Random Boolean Networks and Attractors of their Intersecting Circuits. In: AINA’ 11. IEEE proceedings, Piscataway, pp 483–487

  • Demongeot J, Gaudart J, Mintsa J, Rachdi M (2012a) Demography in epidemics modelling. Commun Pure Appl Anal 11:61–82

    Article  Google Scholar 

  • Demongeot J, Gaudart J, Lontos A, Promayon F, Mintsa J, Rachdi M (2012b) Least diffusion zones in morphogenesis and epidemiology. Int J Bifurcat Chaos 22:50028

    Google Scholar 

  • Dietz K (1967) Epidemics and rumours: a survey. J R Stat Soc Ser A (General) 130:505–528

    Article  Google Scholar 

  • Duchon P, Hanusse N, Lebhar E, Schabanel N (2006) Could any graph be turned into a small-world? Theor Comp Sci 355:96–103

    Article  Google Scholar 

  • Durrett RT (2010) Some features of the spread of epidemics and information on a random graph. Proc Natl Acad Sci USA 107:4491–4498

    Article  Google Scholar 

  • Eisenberg JNS, Desai MA, Levy K, Bates SJ, Liang S, Naumoff K, Scott JC (2007) Environmental determinants of infectious disease: a framework for tracking causal links and guiding public health research. Environ Health Perspect 115:1216–1223

    Article  Google Scholar 

  • Elena A (2004) Algorithme pour la simulation de la dynamique des réseaux de régulation génétique. Master Thesis. University J. Fourier, Grenoble

  • Elena A (2009) Robustesse des réseaux d’automates à seuil. University J. Fourier, Grenoble

    Google Scholar 

  • Elena A, Demongeot J (2008) Interaction motifs in regulatory networks and structural robustness. In: IEEE ARES’ 08. IEEE Press, Piscataway, pp 682–686

  • Elena A, Ben-Amor H, Glade N, Demongeot J (2008) Motifs in regulatory networks and their structural robustness. In: IEEE BIBE’ 08. IEEE Press, Piscataway, pp 234–242

  • Farr W (1866) Report on the cholera epidemic of 1866 in England. Suppl Ann Rep Reg Gen 29:1867–1868

    Google Scholar 

  • Filipe JAN, Gibson GJ (2001) Comparing approximations to spatio-temporal models for Epidemics with local Spread. Bull Math Biol 63:603–624

    Article  Google Scholar 

  • Fraser C, Hollingsworth TD, Chapman R, De Wolf F, Hanage WP (2007) Variation in HIV-1 set-point viral load: epidemiological analysis and an evolutionary hypothesis. Proc Natl Acad Sci USA 104:17441–17446

    Article  Google Scholar 

  • Gaudart J, Giorgi R, Poudiougou B, Ranque S, Doumbo OK, Demongeot J (2007) Spatial cluster detection: principle and application of different general methods. Rev Epid Santé Pub 55:297–306

    Article  Google Scholar 

  • Gaudart J, Touré O, Dessay N, Dicko AL, Ranque S, Forest L, Demongeot J, Doumbo OK (2009) Modelling malaria incidence with environmental dependency in a locality of Sudanese savannah area. Mali Malaria J 8:e61

    Article  Google Scholar 

  • Gaudart J, Ghassani M, Mintsa J, Rachdi M, Waku J, Demongeot J (2010a) Demography and diffusion in epidemics: Malaria and Black Death spread. Acta Biotheor 58:277–305

    Article  Google Scholar 

  • Gaudart J, Ghassani M, Mintsa J, Waku J, Rachdi M, Doumbo OK, Demongeot J (2010b) Demographic and spatial factors as causes of an epidemic spread, the copule approach. Application to the retro-prediction of the Black Death epidemics of 1346. In: IEEE AINA’10. IEEE Press, Piscataway, pp 751–758

  • Gibson ME (1978) Sir Ronald Ross and his contemporaries. J R Soc Med 71:611–618

    Google Scholar 

  • Gillespie DT (1970) A general method for numerically simulating the stochastic time evolution of coupled chemical reactions. J Comput Phys 22:403–434

    Article  Google Scholar 

  • Glade N, Elena A, Fanchon E, Demongeot J, Ben Amor H (2011) Determination, optimization and taxonomy of regulatory networks. The example of Arabidopsis thaliana flower morphogenesis. In: IEEE AINA’ 11. IEEE Press, Piscataway, pp 488–494

  • Graunt J (1662) Natural and political observations made upon the bills of mortality. In: J. Martin, J. Allestry and T. Dicas (eds) T. Roycroft, London

  • Gray RH, Kigozi G, Serwadda D, Makumbi F, Watya S, Nalugoda F et al (2007) Male circumcision for HIV prevention in men in Rakai, Uganda: a randomised trial. Lancet 369:657–666

    Article  Google Scholar 

  • Grinsztejn B, Ribaudo H, Cohen MS, HPTN 052 Protocol Team et al (2011) Effects of early versus delayed initiation of antiretroviral therapy (ART) on HIV clinical outcomes: results from the HPTN 052 randomized clinical trial. In: 6th IAS Conference on HIV Pathogenesis, Treatment and Prevention, Rome

  • Guo HB, Li MY, Shuai Z (2006) Global stability of the endemic equilibrium of multi-group SIR epidemic models. Can Appl Math Q 14:259–284

    Google Scholar 

  • Hallett TB, Smit C, Garnett GP, de Wolf F (2011) Estimating the risk of HIV transmission from homosexual men receiving treatment to their HIV-uninfected partners. Sex Transm Infect 87:17–21

    Article  Google Scholar 

  • Hamer WH (1906) Epidemic disease in England. Lancet 1:733–739

    Google Scholar 

  • Hethcote HW (1978) An immunization model for a heteregenous population. Theor Popul Biol 14:338–349

    Article  Google Scholar 

  • Hethcote HW, Levin SA (1995) Periodicity in Epidemiological models. In: Levin SA, Hallam TG, Gross L (eds) Applied mathematical ecology. Biomathematics, vol 18. Springer, Berlin, pp 193–211

    Chapter  Google Scholar 

  • Hethcote HW, Van den Driessche P (1995) An SIS epidemic model with variable population size and a delay. J Math Biol 34:177–194

    Article  Google Scholar 

  • Hoare MR (1970) Molecular Markov processes. Nature 226:599–603

    Article  Google Scholar 

  • Hollingsworth TD, Anderson RM, Fraser C (2008) HIV-1 transmission, by stage of infection. J Infect Dis 198:687–693

    Article  Google Scholar 

  • International Association for the Study of Obesity (2000) Obesity: preventing and managing the global epidemic. International Obesity Task Force Prevalence Data, London

    Google Scholar 

  • Ishikawa H, Ishii NagaiAN, Ohmae H, Harada M, Suguri S, Leafasia J (2008) A mathematical model for the transmission of the Plasmodium vivax malaria. Parasitol Int 52:81–93

    Article  Google Scholar 

  • Jachimowski JC, McQuarrie DA, Russell ME (1964) A stochastic approach to enzyme-substrate reactions. Biochemistry 3:1732–1736

    Article  Google Scholar 

  • Kermack WO, McKendrick AG (1927) A contribution to the mathematical theory of epidemics. Proc R Soc Lond Ser A 115:700–721

    Article  Google Scholar 

  • Kermack WO, McKendrick AG (1932) Contributions to the mathematical theory of epidemics. II. The problem of endemicity. Proc R Soc Lond Ser A 120:138–155

    Google Scholar 

  • Kermack WO, McKendrick AG (1933) Contributions to the mathematical theory of epidemics. III. Further studies of the problem of endemicity. Proc R Soc Lond Ser A 121:141–194

    Google Scholar 

  • Koella JC, Antia R (2003) Epidemiological models for the spread of anti-malaria resistance. Malaria J 2:e3

    Article  Google Scholar 

  • Koopman JS, Longini IM (1994) The ecological effects of individual exposures and nonlinear disease dynamics in populations. Am J Public Health 84:836–842

    Article  Google Scholar 

  • Korobeinikov A, Maini PK (2004) A Lyapunov function and global properties for SIR and SEIR epidemiological models with nonlinear incidence. Math Biosci Eng 1:57–60

    Article  Google Scholar 

  • Kretzschmar M (1996) Measures of concurrency in networks and the spread of infectious disease. Math Biosci 133:165–195

    Article  Google Scholar 

  • Laitinen J, Power C, Jarvelin MR (2001) Family social class, maternal body mass index, childhood body mass index, and age at menarche as predictors of adult obesity. Am J Clin Nutr 74:287–294

    Google Scholar 

  • Le Vu S, Le Strat Y, Barin F, Pillonel J, Cazein F, Bousquet V, Brunet S, Thierry D, Semaille C, Meyer L, Desenclos JC (2010) Population-based HIV-1 incidence in France, 2003–08: a modelling analysis. Lancet Infect Dis 10:682–687

    Article  Google Scholar 

  • Leclerc PM, Matthews AP, Garenne ML (2009) Fitting the HIV epidemic in Zambia: a two-sex micro-simulation model. PLoS ONE 4:e5439

    Article  Google Scholar 

  • Li MY, Wang L (1995) Global stability in some SEIR epidemic models. Math Biosci 125:155–164

    Article  Google Scholar 

  • Magal P, Ruan S (2012) SIR models revisited: from the individual level to the population level. Preprint University Bordeaux

  • Magal P, McCluskey CC, Webb GF (2010) Lyapunov functional and global asymptotic stability for an infection-age model. Appl Anal 89:1109–1140

    Article  Google Scholar 

  • Maillard G, Charles MA, Thibault N, Forhan A, Sermet C, Basdevant A, Eschwege E (1999) Trends in the prevalence of obesity in the French adult population between 1980 and 1991. Int J Obes 23:389–394

    Article  Google Scholar 

  • McQuarrie DA (1963) Kinetics of small systems. J Chem Phys 38:433–436

    Article  Google Scholar 

  • McQuarrie DA (1967) Stochastic approach to chemical kinetics. J Appl Prob 4:413–478

    Article  Google Scholar 

  • McQuarrie DA, Jachimowski CJ, Russell ME (1964) Kinetics of small systems. II. J Chem Phys 40:2914–2921

    Article  Google Scholar 

  • Melesse DY, Gumel AB (2010) Global asymptotic properties of an SEIRS model with multiple infectious stages. J Math Anal Appl 366:202–217

    Article  Google Scholar 

  • Morris M, Kretzschmar M (2000) A microsimulation study of the effect of concurrent partnerships on the spread of HIV in Uganda. Math Popul Stud 8:109–133

    Article  Google Scholar 

  • Murray JM, McDonald AM, Law MG (2009) Rapidly ageing HIV epidemic among men who have sex with men in Australia. Sex Health 6:83–86

    Article  Google Scholar 

  • Myers A, Rosen JC (1999) Obesity stigmatization and coping: relation to mental health symptoms, body image, and self-esteem. Int J Obes Relat Metab Disord 23:221–230

    Article  Google Scholar 

  • Novi Inverardi PL, Tagliani A (2006) Discrete distributions from moment generating function. Appl Math Comput 182:200–209

    Article  Google Scholar 

  • ObEpi-Roche (2009) Enquête épidémiologique nationale sur le surpoids et l’obésité. Enquête INSERM-Roche, Paris

  • Orcutt GH, Greenberger M, Korbel J, Rivlin AM (1961) Microanalysis of socioeconomic systems: a simulation study. Harper, New York

    Google Scholar 

  • Orroth KK, Freeman EE, Bakker R, Buvé A, Glynn JR, Boily MC, White RG, Habbema JDF, Hayes RJ (2007) Understanding the differences between contrasting HIV epidemics in east and west Africa: results from a simulation model of the Four Cities Study. Sex Transm Infect 83:i5

    Article  Google Scholar 

  • Pilcher CD, Tien HC, Eron JJ Jr, Vernazza PL, Leu SY, Stewart PW, Goh LE, Cohen MS (2004) Brief but efficient: acute HIV infection and the sexual transmission of HIV. J Infect Dis 189:1785–1792

    Article  Google Scholar 

  • Pilcher CD, Joaki G, Hoffman IF, Martinson FE, Mapanje C, Stewart PW, Powers KA, Galvin S, Chilongozi D, Gama S, Price MA, Fiscus SA, Cohen MS (2007) Amplified transmission of HIV-1: comparison of HIV-1 concentrations in semen and blood during acute and chronic infection. AIDS 21:1723–1730

    Article  Google Scholar 

  • Pinkerton SD, Abramson PR (1997) Effectiveness of condoms in preventing HIV transmission. Soc Sci Med 44:1303–1312

    Article  Google Scholar 

  • Porco TC, Martin JN, Page-Shafer KA, Cheng A, Charlebois E, Grant RM, Osmond DH (2004) Decline in HIV infectivity following the introduction of highly active antiretroviral therapy. AIDS 18:81–88

    Article  Google Scholar 

  • Quinn TC, Wawer MJ, Sewankambo N, Serwadda D, Li C, Wabwire-Mangen F, Meehan MO, Lutalo T, Gray RH (2000) Viral load and heterosexual transmission of human immunodeficiency virus type 1. Rakai project study group. N Engl J Med 342:921–929

    Article  Google Scholar 

  • Reynolds SJ, Makumbi F, Nakigozi G, Kagaayi J, Gray RH, Wawer M, Quinn TC, Serwadda D (2011) HIV-1 transmission among HIV-1 discordant couples before and after the introduction of antiretroviral therapy. AIDS 25:473–477

    Article  Google Scholar 

  • Rhodes CJ, Demetrius L (2010) Evolutionary entropy determines invasion success in emergent epidemics. PLoS ONE 5:e12951

    Article  Google Scholar 

  • Rogier C, Sallet G (2004) Modélisation du paludisme. Med Trop 64:89–97

    Google Scholar 

  • Ross R (1910) Prevention of Malaria. John Murray, London

    Google Scholar 

  • Ross R (1915) Some a priori pathometric équations. Br Med J 1:546–547

    Article  Google Scholar 

  • Ross R (1916) An application of the theory of probabilities to the study of a priori pathometry. Part I. Proc R Soc Lond Ser A 92:204–230

    Article  Google Scholar 

  • Ruan S, Xiao D, Beier JC (2008) On the delayed Ross–MacDonald model for Malaria transmission. Bull Math Biol 70:1098–1114

    Article  Google Scholar 

  • Sathik MM, Rasheed AA (2011) Social network analysis in an online blogosphere. Int J Eng Sci Technol 3:117–121

    Google Scholar 

  • Sawers L, Stillwaggon E (2010) Concurrent sexual partnerships do not explain the HIV epidemics in Africa: a systematic review of the evidence. J Int AIDS Soc 13:1–23

    Article  Google Scholar 

  • Scharoun-Lee M, Adair LS, Kaufman JS, Gordon-Larsen P (2009) Obesity, race/ethnicity and the multiple dimensions of socioeconomic status during the transition to adulthood: a factor analysis approach. Soc Sci Med 68:708–716

    Article  Google Scholar 

  • Seng R, Rolland M, Beck-Wirth G, Souala GF, Deveau C, Delfraissy JF, Goujard C, Meyer L (2011) Trends in unsafe sex and influence of viral load among patients followed since primary HIV infection between 2000 and 2009. AIDS 25:977–988

    Article  Google Scholar 

  • Shi R, Chen L (2007) Stage-structured impulsive model for pest management. Discrete Dyn Nat Soc 2007:97608

  • Shirreff G, Pellis L, Laeyendecker O, Fraser C (2011) Transmission selects for HIV-1 strains of intermediate virulence: a modelling approach. PLoS Comput Biol 7:e1002185

    Article  Google Scholar 

  • Taramasco C (2011) Impact de l’obésité sur les structures sociales et impact des structures sociales sur l’obésité? PhD thesis. Ecole Polytechnique, Paris

  • Taramasco C, Demongeot J (2011) Collective intelligence, social networks and propagation of a social disease, the obesity. In: EIDWT’11. IEEE Proceedings, Piscataway, pp 86–90

  • Tuckwell HC, Williams RJ (2007) Some properties of a simple stochastic epidemic model of SIR type. Math Biosci 208:76–97

    Article  Google Scholar 

  • Velter A, Enquête Presse Gay (2004) Maladies Infectieuses. Institut national de veille sanitaire, Paris

    Google Scholar 

  • Velter A, Barin F, Bouyssou A, Le Vu S, Guinard J, Pillonel J, Semaille C (2010) Prévalence du VIH et comportement de dépistage des hommes fréquentant les lieux de convivialité gay parisiens. Prevagay 2009. Bull Epidemiol Hebdom 22:464–467

    Google Scholar 

  • Wang Y, Wang J, Zhang L (2010) Cross diffusion-induced pattern in an SI model. Math Comput 217:1965–1970

    Google Scholar 

  • Wilson DP, Law MG, Grulich AE, Cooper DA, Kaldor JM (2008) Relation between HIV viral load and infectiousness: a model-based analysis. Lancet 372:314–320

    Article  Google Scholar 

  • Wilson DP, Hoare A, Regan DG, Law MG (2009) Importance of promoting HIV testing for preventing secondary transmissions: modelling the Australian HIV epidemic among men who have sex with men. Sex Health 6:19–33

    Article  Google Scholar 

  • World Health Organization (2000) Obesity: preventing and managing the global epidemic. WHO Technical report 894, Geneva Optimal contact process on complex networks

  • Xiridou M, Geskus R, de Wit J, Coutinho R, Kretzschmar M (2003) The contribution of steady and casual partnerships to the incidence of HIV infection among homosexual men in Amsterdam. AIDS 17:1029–1038

    Article  Google Scholar 

  • Xiridou M, Geskus R, de Wit J, Coutinho R, Kretzschmar M (2004) Primary HIV infection as source of HIV transmission within steady and casual partnerships among homosexual men. AIDS 18:1311–1320

    Article  Google Scholar 

  • Yang R, Zhou T, Xie YB, Lai YC, Wang BH (2008) Optimal contact process on complex networks. Phys Rev E 78:066109

    Article  Google Scholar 

  • Yongzhen P, Shaoying L, Changguo L, Lansun C (2009) The dynamics of an impulse delay model with variable coefficients. Appl Math Mod 33:2766–2776

    Article  Google Scholar 

  • Yoshida N, Hara T (2007) Global stability of a delayed SIR epidemic model with density dependent birth and death rates. J Comput Appl Math 201:339–347

    Article  Google Scholar 

  • Yu J, Jiang D, Shi N (2009) Global stability of two-group SIR model with random perturbation. J Math Anal Appl 360:235–244

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. AGIM, FRE, CNRS 3405, Faculty of Medicine of Grenoble, University J. Fourier, 38700, La Tronche, France

    J. Demongeot, O. Hansen, H. Hessami, A. S. Jannot, J. Mintsa, M. Rachdi & C. Taramasco

  2. Escuela de Ingeniería Civil Informática, Universidad de Valparaiso, Avenida Gran Bretaña 1111, Valparaiso, Chile

    C. Taramasco

  3. ISCPIF, Complex Systems Institute of Paris Ile de France, 57-59 rue Lhommond, 75005, Paris, France

    C. Taramasco

  4. Universidad de Valparaiso, Avenida Gran Bretaña 1111, Valparaiso, Chile

    J. Demongeot & C. Taramasco

  5. University Pierre Mendès-France, BP 47, 38040, Grenoble Cedex 9, France

    M. Rachdi

Authors
  1. J. Demongeot
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. O. Hansen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. H. Hessami
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. A. S. Jannot
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. J. Mintsa
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. M. Rachdi
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. C. Taramasco
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to J. Demongeot.

Annex

Annex

Fig. 12
figure 12

Diagram describing the different status an individual male single or in couple having sex with other men (MSM) could successively go through during HIV spread in a MSM population

Full size image

Rights and permissions

Reprints and permissions

About this article

Cite this article

Demongeot, J., Hansen, O., Hessami, H. et al. Random Modelling of Contagious Diseases. Acta Biotheor 61, 141–172 (2013). https://doi.org/10.1007/s10441-013-9176-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10441-013-9176-6

Keywords

Search

Navigation