Deformable Surface Model for the Evaluation of Abdominal Aortic Aneurysms Treated with an Endovascular Sealing System

Abstract

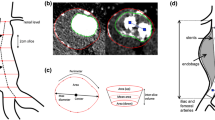
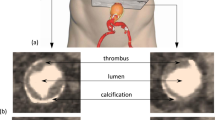
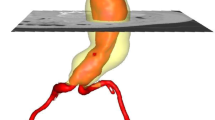
Rupture of abdominal aortic aneurysms (AAA) is responsible for 1–3% of all deaths among the elderly population in developed countries. A novel endograft proposes an endovascular aneurysm sealing (EVAS) system that isolates the aneurysm wall from blood flow using a polymer-filled endobag that surrounds two balloon-expandable stents. The volume of injected polymer is determined by monitoring the endobag pressure but the final AAA expansion remains unknown. We conceived and developed a fully deformable surface model for the comparison of pre-operative sac lumen size and final endobag size (measured using a follow-up scan) with the volume of injected polymer. Computed tomography images were acquired for eight patients. Aneurysms were manually and automatically segmented twice by the same observer. The injected polymer volume resulted 9% higher than the aneurysm pre-operative lumen size (p < 0.05), and 11% lower than the final follow-up endobag volume (p < 0.01). The automated method required minimal user interaction; it was fast and used a single set of parameters for all subjects. Intra-observer and manual vs. automated variability of measured volumes were 0.35 ± 2.11 and 0.07 ± 3.04 mL, respectively. Deformable surface models were used to quantify AAA size and showed that EVAS system devices tended to expand the sac lumen size.

Appendix

GDM Initialization

The geometrically deformed model (GDM) starts with a regular icosahedron of unit side length. Each of its triangular faces goes through two processes: projection on a sphere of radius r, and subdivision in four new faces. This process is repeated until the average side length is less than a value $d_{\text{thresh}}$, chosen in order to compromise the amount of model faces, segmentation time, and the spatial resolution of the GDM. The resulting spheroid has approximately $\frac{\pi }{\sqrt 3 }\left( {\frac{r}{{d_{\text{thresh}} }}} \right)^{2}$ initial faces.

Vertex Normal Computation

The vertex normal vector $\varvec{n}$ is the area-weighted sum of the unitary normal vectors to all faces that share the same vertex:

$$\varvec{n} = \frac{{\mathop \sum \nolimits_{j = 1}^{{N_{\varvec{F}} }} a_{j} \widehat{\varvec{n}}_{\varvec{j}}^{\varvec{F}} }}{{\mathop \sum \nolimits_{j = 1}^{{N_{\text{F}} }} a_{j} }},$$

(A1)

where $\widehat{\varvec{n}}_{\varvec{j}}^{\varvec{F}}$ is the unitary normal vector of the j-th adjacent face, and $a_{j}$ is the face area. The value of $\widehat{\varvec{n}}_{\varvec{j}}^{\varvec{F}}$ can be computed by cross-product:

$$\widehat{\varvec{n}}_{\varvec{j}}^{\varvec{F}} = \frac{{ {\Delta \varvec{x}_{1,j} } \times {\Delta \varvec{x}_{2,j} } }}{{\left| {\left| {\Delta \varvec{x}_{1,j} } \right| \times \left| {\Delta \varvec{x}_{2,j} } \right|} \right|}} = 2\frac{{ {\Delta \varvec{x}_{1,j} } \times {\Delta \varvec{x}_{2,j} } }}{{a_{j} }},$$

(A2)

where $\varvec{x} = \left( {x,y,z} \right)$ is the vertex position, and $\Delta \varvec{x}_{1,j}$ and $\Delta \varvec{x}_{2,j}$ are the vectors pointing from $\varvec{x}$ to its two neighbor vertices in the jth adjacent face.

Inflation Force over a Vertex

The inflation force $\varvec{f}^{\varvec{p}}$ on a vertex is approximated by the average force over all of its $N_{F}$ adjacent faces:

$$\varvec{f}^{\varvec{p}} = \frac{1}{{N_{F} }}\mathop \sum \limits_{j = 1}^{{N_{F} }} \varvec{f}_{\varvec{j}}^{\varvec{p}} = \frac{\Delta p}{{N_{F} }}\mathop \sum \limits_{j = 1}^{{N_{F} }} a_{j} \widehat{\varvec{n}}_{\varvec{j}}^{\varvec{F}} = \frac{2\Delta p}{{N_{F} }}\mathop \sum \limits_{j = 1}^{{N_{F} }} \varvec{n} = 2 \Delta p\varvec{n}\varvec{.}$$

(A3)

Vertex Movement Simulation

Vertex movement is simulated by the kinematic equation of force-accelerated particles (Newton’s second law):

$$\varvec{f}\left( t \right) = m\varvec{a}\left( t \right),$$

(A4)

where $m$ is the mass of the vertex, $\varvec{f}\left( t \right)$ is the total force acting on it and $\varvec{a}\left( t \right)$ is the resulting acceleration. The term $\varvec{f}\left( t \right)$ at each instant is calculated as:

$$\varvec{f}\left( t \right) = \varvec{f}^{\varvec{p}} \left( t \right) + \varvec{f}_{\varvec{s}} \left( t \right) + 0.5\varvec{f}_{\varvec{b}} \left( t \right) + \varvec{f}_{\varvec{v}} \left( t \right).$$

(A5)

The term $\varvec{f}_{\varvec{v}} \left( t \right)$ requires the prior knowledge of the vertex speed $\varvec{v}\left( t \right)$ at that moment, which approximated from the known values in a previous instant $t - dt$:

$$\varvec{v}\left( t \right) = \varvec{v}\left( {t - dt} \right) + \varvec{a}\left( {t - dt} \right).dt.$$

(A6)

The future position of the vertex, $\varvec{x}_{\varvec{f}} \left( t \right)$ is calculated for the next instant $t + dt$:

$$\varvec{x}_{\varvec{f}} \left( {t + dt} \right) = \varvec{x}\left( t \right) + \varvec{v}\left( t \right).dt + \frac{1}{2}dt^{2} \varvec{a}\left( t \right)$$

(A7)

Future position is calculated for every vertex separately, and then simultaneously updated:

$$\begin{aligned} \varvec{x}\left( {t + dt} \right)\text{ := } & \varvec{x}_{\varvec{f}} \left( {t + dt} \right) \\ t\text{ := } & t + dt. \\ \end{aligned}$$

(A8)

GDM Parameters and Selection Criteria

Mass and damping coefficient were selected after an initial calibration experiment where the spherical GDM was inflated with a constant normal force plus a random deviation of 1% of this force. After a certain time, inflation force stopped, and the mesh was allowed to relax. The quotient $m/\gamma = 2$ was found to stabilize the GDM in a number of iterations below T _sizing with little surface oscillation (i.e., behaving like an overdamped spring system) using a unitary time step. The HU thresholds resulted from a manual seed point selection, where the mean value and standard deviation of HU were computed inside an initial sphere of 20-pixel radius. Assuming Normal and $\chi^{2}$ distribution, the 95% confidence interval was calculated for mean HU ($\mu$) and standard deviation ($\sigma$), respectively. The upper limit was extended +6 times $\sigma$ above the mean and the lower limit 3 times $\sigma$ below the mean. This upper limit allowed the GDM to adequately expand through some bright structures (e.g., metallic stents or high concentrations of contrast agent). The lower limit was appropriate to constrain the GMD in the presence of dark structures surrounding the aneurysm lumen.