Skip to main content
SpringerLink
Log in

Quantitative characterization of high molecular weight polymer solutions in microfluidic hyperbolic contraction flow

  • Research Paper
  • Published:
Microfluidics and Nanofluidics Aims and scope Submit manuscript

Abstract

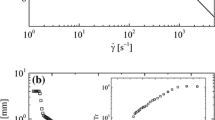
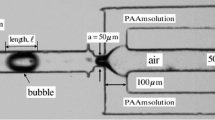
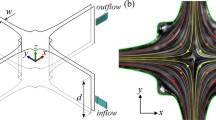
Nonlinear flows of polyacrylamide (PAAm) (\(M_{\text{w}} = 5.7 \times 10^{6}\,{\text{g}}/{\text{mol}}\)) aqueous solutions through a micro-fabricated, hyperbolic contraction geometry with high Hencky strain (\(\varepsilon_{{\text{H}}} = 3.7\)) have been characterized by micro-particle image velocimetry (\(\mu \)-PIV). Various flow dynamics regimes in a range of Weissenberg number (Wi) and Reynolds number (Re) are presented in a Wi–Re diagram. The symmetric corner vortices are only observed in the flow of low concentration PAAm solution (\(c/c^{*}=3.3\)). In a higher concentration (\(c/c^{*}=8.3\)), PAAm solution exhibits chaotic-like flow patterns in the strong nonlinear flow regime (\(Wi>350\)). Extensional deformation in nonlinear flows of Wi up to 860 has been analyzed. Furthermore, the local stretch experienced by the polymer chain in complex flow is systematically quantified and linked to the corresponding velocity vector fields, which are valuable for understanding the highly nonlinear flow phenomena.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  • Campo-Deaño L, Galindo-Rosales FJ, Pinho FT, Alves MA, Oliveira MS (2011) Flow of low viscosity Boger fluids through a microfluidic hyperbolic contraction. J Nonnewton Fluid Mech 166(21):1286–1296

    Article  MATH  Google Scholar 

  • Chan EY, Goncalves NM, Haeusler RA, Hatch AJ, Larson JW, Maletta AM, Yantz GR, Carstea ED, Fuchs M, Wong GG et al (2004) DNA mapping using microfluidic stretching and single-molecule detection of fluorescent site-specific tags. Genome Res 14(6):1137–1146

    Article  Google Scholar 

  • Cogswell F (1978) Converging flow and stretching flow: a compilation. J Nonnewton Fluid Mech 4(1):23–38

    Article  Google Scholar 

  • François J, Schwartz T, Weill G (1980) Crossover from the theta to the excluded volume single chain statistics: new experimental evidences and a modified Blob model. Macromolecules 13(3):564–570

    Article  Google Scholar 

  • Groisman A, Enzelberger M, Quake SR (2003) Microfluidic memory and control devices. Science 300(5621):955–958

    Article  Google Scholar 

  • Gulati S, Muller SJ, Liepmann D (2008) Direct measurements of viscoelastic flows of DNA in a 2: 1 abrupt planar micro-contraction. J Nonnewton Fluid Mech 155(1):51–66

    Article  Google Scholar 

  • Haward SJ, Odell JA, Li Z, Yuan X-F (2010) The rheology of polymer solution elastic strands in extensional flow. Rheol Acta 49(7):781–788

    Article  Google Scholar 

  • Hur JS, Shaqfeh ES, Babcock HP, Smith DE, Chu S (2001) Dynamics of dilute and semidilute DNA solutions in the start-up of shear flow. J Rheol (1978-present) 45(2):421–450

    Article  Google Scholar 

  • Hur JS, Shaqfeh ES, Babcock HP, Chu S (2002) Dynamics and configurational fluctuations of single DNA molecules in linear mixed flows. Phys Rev E 66(1):011915

    Article  Google Scholar 

  • James DF (1991) Flow in a converging channel at moderate Reynolds numbers. AIChE J 37(1):59–64

    Article  Google Scholar 

  • Kang K, Lee LJ, Koelling KW (2005) High shear microfluidics and its application in rheological measurement. Exp Fluids 38(2):222–232

    Article  Google Scholar 

  • Kang K, Koelling KW, Lee LJ (2006) Microdevice end pressure evaluations with Bagley correction. Microfluid Nanofluid 2(3):223–235

    Article  Google Scholar 

  • Kim L, Toh Y-C, Voldman J, Yu H (2007) A practical guide to microfluidic perfusion culture of adherent mammalian cells. Lab Chip 7(6):681–694

    Article  Google Scholar 

  • Lanzaro A, Yuan X-F (2011) Effects of contraction ratio on non-linear dynamics of semi-dilute, highly polydisperse PAAm solutions in microfluidics. J Nonnewton Fluid Mech 166(17):1064–1075

    Article  Google Scholar 

  • Lanzaro A, Yuan X-F (2014) A quantitative analysis of spatial extensional rate distribution in nonlinear viscoelastic flows. J Nonnewton Fluid Mech 207:32–41

    Article  Google Scholar 

  • Larson R, Hu H, Smith D, Chu S (1999) Brownian dynamics simulations of a DNA molecule in an extensional flow field. J Rheol (1978-present) 43(2):267–304

    Article  Google Scholar 

  • Li Z, Yuan X-F, Haward SJ, Odell JA, Yeates S (2011) Non-linear dynamics of semi-dilute polydisperse polymer solutions in microfluidics: a study of a benchmark flow problem. J Nonnewton Fluid Mech 166(16):951–963

    Article  Google Scholar 

  • Li Z, Yuan X-F, Haward SJ, Odell JA, Yeates S (2011) Non-linear dynamics of semi-dilute polydisperse polymer solutions in microfluidics: effects of flow geometry. Rheol Acta 50(3):277–290

    Article  Google Scholar 

  • McKinley GH, Rodd LE, Oliverira MS, Cooper-White J (2007) Extensional flows of polymer solutions in microfluidic converging/diverging geometries. J Cent South Univ Technol 14(1):6–9

    Article  Google Scholar 

  • Meinhart C, Wereley S, Gray M (2000) Volume illumination for two-dimensional particle image velocimetry. Meas Sci Technol 11(6):809

    Article  Google Scholar 

  • Meinhart CD, Wereley ST (2003) The theory of diffraction-limited resolution in microparticle image velocimetry. Meas Sci Technol 14(7):1047

    Article  Google Scholar 

  • Mulligan MK, Rothstein JP (2011) The effect of confinement-induced shear on drop deformation and breakup in microfluidic extensional flows. Phys Fluids (1994-present) 23(2):022004

    Article  Google Scholar 

  • Oliveira MSN, Alves MA, McKinley GH, Pinho FT (2006) Extensional flow of water and a semi-dilute aqueous solution of polyethylene oxide through microfabricated hyperbolic contractions. In: 13th Int symp on applications of laser techniques to fluid mechanics, Lisbon, Portugal, 26–29 June

  • Oliveira MSN, Alves MA, Pinho FT, McKinley GH (2007) Viscous flow through microfabricated hyperbolic contractions. Exp Fluids 43(2–3):437–451

    Article  Google Scholar 

  • Omowunmi SC, Yuan X-F (2013) Time-dependent non-linear dynamics of polymer solutions in microfluidic contraction flow: a numerical study on the role of elongational viscosity. Rheol Acta 52(4):337–354

    Article  Google Scholar 

  • Perkins TT, Smith DE, Chu S (1997) Single polymer dynamics in an elongational flow. Science 276(5321):2016–2021

    Article  Google Scholar 

  • Pipe CJ, Majmudar TS, McKinley GH (2008) High shear rate viscometry. Rheol Acta 47(5–6):621–642

    Article  Google Scholar 

  • Randall GC, Schultz KM, Doyle PS (2006) Methods to electrophoretically stretch DNA: microcontractions, gels, and hybrid gel-microcontraction devices. Lab Chip 6(4):516–525

    Article  Google Scholar 

  • Rodd LE, Scott TP, Boger DV, Cooper-White JJ, McKinley GH (2005) The inertio-elastic planar entry flow of low-viscosity elastic fluids in micro-fabricated geometries. J Nonnewton Fluid Mech 129(1):1–22

    Article  Google Scholar 

  • Rodd L, Cooper-White J, Boger D, McKinley G (2007) Role of the elasticity number in the entry flow of dilute polymer solutions in micro-fabricated contraction geometries. J Nonnewton Fluid Mech 143(2):170–191

    Article  Google Scholar 

  • Santiago JG, Wereley ST, Meinhart CD, Beebe D, Adrian RJ (1998) A particle image velocimetry system for microfluidics. Exp Fluids 25(4):316–319

    Article  Google Scholar 

  • Schroeder CM, Babcock HP, Shaqfeh ES, Chu S (2003) Observation of polymer conformation hysteresis in extensional flow. Science 301(5639):1515–1519

    Article  Google Scholar 

  • Schroeder CM, Teixeira RE, Shaqfeh ES, Chu S (2005) Dynamics of DNA in the flow-gradient plane of steady shear flow: observations and simulations. Macromolecules 38(5):1967–1978

    Article  Google Scholar 

  • Smith DE, Chu S (1998) Response of flexible polymers to a sudden elongational flow. Science 281(5381):1335–1340

    Article  Google Scholar 

  • Sousa P, Pinho F, Oliveira M, Alves M (2011) Extensional flow of blood analog solutions in microfluidic devices. Biomicrofluidics 5(1):014108

    Article  Google Scholar 

  • White F (1991) Viscous fluid flow. McGraw-Hill, New York

    Google Scholar 

Download references

Acknowledgments

The authors would like to acknowledge the financial support of the Engineering and Physical Sciences Research Council (EP/E032699) and Linkam Scientific Instruments Ltd., and to thank Malcolm Mackley, Jeff Odell, Simon Haward and Sunday Omowunmi for insightful discussions.

Author information

Authors and Affiliations

  1. Manchester Institute of Biotechnology, School of Chemical Engineering and Analytical Science, The University of Manchester, Manchester, M13 9PL, UK

    Alfredo Lanzaro

  2. State Key Laboratory of Pollution Control and Resource Reuse, Tongji University, Shanghai, 200092, People’s Republic of China

    Zhuo Li

  3. National Supercomputer Centre in Guangzhou, Research Institute on Application of High Performance Computing, Sun Yat-Sen University, Guangzhou, People’s Republic of China

    Xue-Feng Yuan

Authors
  1. Alfredo Lanzaro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhuo Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xue-Feng Yuan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xue-Feng Yuan.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lanzaro, A., Li, Z. & Yuan, XF. Quantitative characterization of high molecular weight polymer solutions in microfluidic hyperbolic contraction flow. Microfluid Nanofluid 18, 819–828 (2015). https://doi.org/10.1007/s10404-014-1474-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10404-014-1474-z

Keywords

Search

Navigation