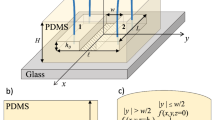
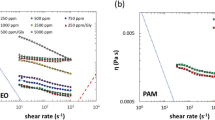
Abstract
Extensional flows and the underlying stability/instability mechanisms are of extreme relevance to the efficient operation of inkjet printing, coating processes and drug delivery systems, as well as for the generation of micro droplets. The development of an extensional rheometer to characterize the extensional properties of low viscosity fluids has therefore stimulated great interest of researchers, particularly in the last decade. Microfluidics has proven to be an extraordinary working platform and different configurations of potential extensional microrheometers have been proposed. In this review, we present an overview of several successful designs, together with a critical assessment of their capabilities and limitations.




















Similar content being viewed by others
References
Alves MA (2008) Design a cross-slot flow channel for extensional viscosity measurements. AIP Conf Proc 1027:240–242
Anna SL, McKinley GH (2008) Effect of a controlled pre-deformation history on extensional viscosity of dilute polymer solutions. Rheol Acta 47:841–859
Anna SL, Rogers C, McKinley GH (1999) On controlling the kinematics of a filament stretching rheometer using a real-time active control mechanism. J Non Newton Fluid Mech 87:307–335
Anna SL, McKinley GH, Nguyen DA, Sridhar T, Muller S, Huang J, James DF (2001) An interlaboratory comparison of measurements from filament-stretching rheometers using common test fluids. J Rheol 45(1):83–114
Ardekani AM, Sharma V, McKinley GH (2010) Dynamics of bead formation, filament thinning and breakup in weakly viscoelastic jets. J Fluid Mech 665:46–56
Arratia PE, Thomas CC, Diorio J, Gollub JP (2006) Elastic instabilities of polymer solutions in cross-channel flow. Phys Rev Lett 96:144502
Arratia PE, Gollub JP, Durian DJ (2008) Polymeric filament thinning and breakup in microchannels. Phys Rev E 77:036309
Babcock HP, Teixeira RE, Hur JS, Shaqfeh ESG (2003) Visualization of molecular fluctuations near the critical point of the coil-stretch transition in polymer elongation. Macromolecules 36:4544–4548
Balducci AG, Tang J, Doyle PS (2008) Electrophoretic stretching of dna molecules in cross-slot nanoslit channels. Macromolecules 41:9914–9918
Bandalusena HCH, Zimmerman WB, Rees JM (2009) Microfluidic rheometry of a polymer solution by micron resolution particle image velocimetry: a model validation study. Meas Sci Technol 20:115404
Banpurkar AG, Duits MHG, van den Ende D, Mugele F (2009) Electrowetting of complex fluids: perspectives for rheometry on chip. Langmuir 25:1245–1252
Bänsch E, Berg CP, Ohlhoff A (2004) Uniaxial extensional flows in liquid bridges. J Fluid Mech 521:353–379
Barnes HA, Hutton JF, Walters K (1993) An introduction to rheology. Rheology series, 3rd edn. Elsevier, The Netherlands
Bazilevsky A, Entov V, Rozhkov A (1990) Liquid filament microrheometer and some of its applications. In: Oliver DR (ed) Proceedings of the third European rheology conference. Elsevier, The Netherlands, pp 41–43
Becherer P, Morozov AN, van Saarloos W (2008) Scaling of singular structures in extensional flow of dilute polymer solutions. J Non Newton Fluid Mech 153:183–190
Beni G, Hackwood S (1981) Electro-wetting displays. Appl Phys Lett 38(4):207–209
Berge B (1993) Electrocapillarité et mouillage de films isolants par l’eau. Comptes rendus de l’ Academie des sciences Seŕie 2 317(2):157–163
Bhattacharjee PK, McDonnell AG, Prabhakar R, Yeo LY, Friend J (2011) Extensional flow of low-viscosity fluids in capillary bridges formed by pulsed surface acoustic wave jetting. New J Phys 13:023005
Bird RB, Armstrong RC, Hassager O (1987) Dynamics of polymer liquids. Fluid mechanics, vol 1, 2nd edn. Wiley, USA
Campo-Deaño L, Clasen C (2010) The slow retraction method (SRM) for the determination of ultra-short relaxation times in capillary breakup extensional rheometry experiments. J Non Newton Fluid Mech 165:1688–1699
Campo-Deaño L, Galindo-Rosales FJ, Pinho FT, Alves MA, Oliveira MSN (2011) Flow of low viscosity boger fluids through a microfluidic hyperbolic contraction. J Non Newton Fluid Mech 166:1286–1296
Cubaud T, Mason TG (2009) High-viscosity fluids threads in weakly diffusive microfluidic systems. New J Phys 11:075029
Dontula P, Pasquali M, Scriven LE, Macosko CW (1997) Can extensional viscosity be measured with opposed-nozzle devices. Rheol Acta 36:429–448
Dukhin A, Zelenev A (2010) Rheology: shear, extensional, longitudinal. Sci Topics. http://www.scitopics.com/Rheology_shear_extensional_longitudinal.html (accessed 20 June 2012)
Dylla-Spears R, Townsend JE, Jen-Jacobson L, Sohn LL, Muller SJ (2010) Single-molecule sequence detection via microfluidic planar extensional flow at a stagnation point. Lab Chip 10:1543–1549
Erni P, Varagnat M, Clasen C, Crest J, McKinley GH (2011) Microrheometry of sub-nanolitre biopolymer samples: non-newtonian flow phenomena of carnivorous plant mucilage. Soft Matter 7:10889
Franck A (2011) The ARES-EVF: option for measuring extensional viscosity of polymer melts. http://www.tainstruments.com/pdf/literature/APN002_V2_ARES_EVF_to_measure_elongation_viscosity.pdf (accessed 20 June 2012)
Fuller GG, Cathey CA, Hubbard B, Zebrowski BE (1987) Extensional viscosity measurements for low-viscosity fluids. J Rheol 31:235–249
Funami T (2011) Next target for food hydrocolloid studies: texture design of foods using hydrocolloid technology. Food Hydrocolloids 25:1904–1914
Gaudet S, McKinley GH (1998) Extensional deformation of non-Newtonian liquid bridges. Comput Mech 21:461–476
Göttfert (2011) Elongational testing—rheotens and haul-off. http://www.goettfert.com/images/stories/downloads/produkte/Rheotens_71-97_en.pdf (accessed 20 June 2012)
Guillot P, Panizza P, Salmon JP, Joanicot M, Colin A (2006) Viscosimeter on a microfluidic chip. Langmuir 22:6438–6445
Guillot P, Moulin T, Kotitz RMG, Dodge A, Joanicot M, Colin A, Bruneau CH, Colin T (2008) Towards a continuous microfluidic rheometer. Microfluid Nanofluid 5:619–630
Gupta RK, Sridhar T (1988) Rheological Measurements. In: Clegg D, Collyer AA (eds) Elsevier, The Netherlands
Haward SJ (2010) Buckling instabilities in dilute polymer solution elastic strands. Rheol Acta 49:1219–1225
Haward SJ, Odell JA, Berry M, Hall T (2011) Extensional rheology of human saliva. Rheol Acta 50:869–879
Haward SJ, Ober TJ, Oliveira MSN, Alves MA, McKinley GH (2012a) Extensional rheology and elastic instabilities of a wormlike micellar solution in a microfluidic cross-slot device. Soft Matter 8:536–555
Haward SJ, Oliveira MSN, Alves MA, McKinley GH (2012b) Optimized cross-slot flow geometry for microfluidic extensional rheometry. Phys Rev Lett (submitted)
Hermansky CG, Boger DV (1995) Opposing-jet viscometry of fluids with viscosity approaching that of water. J Non Newton Fluid Mech 56:1–14
Hsieh SS, Huang YC (2008) Passive mixing in micro-channels with geometric variations through μPIV and μLIF measurements. J Micromech Microeng 18:065017
Hudson SD, Phelan FR, Handler MD, Cabral JT, Migler KB, Amis EJ (2004) Microfluidic analog of the four-roll mill. Appl Phys Lett 85(2):335–337
Husny J, Cooper-White JJ (2006) The effect of elasticity on drop creation in T-shaped microchannels. J Non Newton Fluid Mech 137:121–136
Kojic N, Bico J, Clasen C, McKinley GH (2006) Ex vivo rheology of spider silk. J Exp Biol 209:4355–4362
Kumari N, Garimella SV (2011) Electrowetting-induced dewetting transitions on superhydrophobic surfaces. Langmuir 27:10342–10346
Lagnado RR, Leal LG (1990) Visualization of three-dimensional flow in a four-roll mill. Exp Fluids 9:25–32
Lee JS, Dylla-Spears R, Teclemariam NP, Muller SJ (2007) Microfluidic four-roll mill for all flow types. Appl Phys Lett 90:074103
Lee W, Walker LM, Anna SL (2011) Competition between viscoelasticity and surfactant dynamics in flow focusing microfluidics. Macromol Mater Eng 296:203–213
Lin YY, Lin CW, Yang LJ, Wang AB (2007) Micro-viscometer based on electrowetting on dielectric. Electrochim Acta 52:2876–2883
Link DR, Anna SL, Weitz DA, Stone HA (2004) Geometrically mediated breakup of drops in microfluidic devices. Phys Rev Lett 92(5):054503
Lippmann G (1875) Relations entre les phénomènes électriques et capillaires. Ann Chim Phys 5:494
Macosko CW (1994) Rheology: principles, measurements, and applications. Wiley, USA
Maia JM, Covas JA, Nóbrega JM, Dias TF, Alves FE (1999) Measuring uniaxial extensional viscosity using a modified rotational rheometer. J Non Newton Fluid Mech 80:183–197
Matta JE, Tytus RP (1990) Liquid stretching using a falling cylinder. J Non Newton Fluid Mech 35:215–229
McKinley GH (2005) Visco-elasto-capillary thinning and break-up of complex fluids. In: Binding DM, Walters K (eds) Rheology reviews. The British Society of Rheology
McKinley GH, Hall NR (2011a) Shear history extensional rheology experiment II (SHERE II). http://issresearchproject.grc.nasa.gov/MSG/SHERE_II/ (accessed 20 June 2012)
McKinley GH, Hall NR (2011b) Shear history extensional rheology experiment (SHERE). http://issresearchproject.grc.nasa.gov/MSG/SHERE/ (accessed 20 June 2012)
McKinley GH, Sridhar T (2002) Filament-stretching rheometry of complex fluids. Annu Rev Fluid Mech 34:375–415
McKinley GH, Tripathi A (2000) How to extract the Newtonian viscosity from capillary breakup measurements in a filament rheometer. J Rheol 44(3):653–671
McKinley GH, Brauner O, Yao M (2001) Kinematics of filament stretching in dilute and concentrated polymer solutions. Korea Austral J Rheol 13(1):29–35
Meissner J (1985a) Experimental aspects in polymer melt elongational rheometry. Chem Eng Commun 33:159–180
Meissner J (1985b) Rheometry of polymer melts. Annu Rev Fluid Mech 17:45–64
Mugele F, Baret JC (2005) Electrowetting: from basics to applications. J Phys Condens Matter 17:R705–R774
Münsted H (1979) New universal extensional rheometer for polymer melts. Measurements on a polystyrene sample. J Rheol 23(4):421–436
Nelson WC, Kavehpour HP, Kim CJ (2010) A micro extensional filament rheometer enabled by EWOD. In: IEEE 23rd international conference on micro electro mechanical systems (MEMS), pp 75–78
Nelson WC, Kavehpour HP, Kim CJ (2011) A miniature capillary breakup extensional rheometer by electrostatically assisted generation of liquid filaments. Lab Chip 11:2424–2431
Ng SL, Mun RP, Boger DV, James DF (1996) Extensional viscosity measurements of dilute polymer solutions of various polymers. J Non Newton Fluid Mech 65:291–298
Niedzwiedz K, Arnolds O, Willenbacher N, Brummer R (2009) How to characterize yield stress fluids with capillary breakup extensional rheometry (CaBER). Appl Rheol 19(4):41969
Niedzwiedz K, Buggisch H, Willenbacher N (2010) Extensional rheology of concentrated emulsions as probed by capillary breakup elongational rheometry (CaBER). Rheol Acta 49:1103–1116
Nijenhuis K, McKinley GH, Spiegelberg S, Barnes HA, Aksel N, Heymann L, Odell JA (2007) Springer handbook on experimental fluid mechanics, Chap 9. Non-Newtonian flows. Springer, Berlin
Odell JA, Carrington SA (2006) Extensional flow oscillatory rheometry. J Non Newton Fluid Mech 137:110–120
Oliveira MSN, McKinley GH (2005) Iterated stretching and multiple beads-on-a-string phenomena in dilute solutions of highly extensible flexible polymers. Phys Fluids 17:071704
Oliveira MSN, Yeh R, McKinley GH (2006) Iterated stretching, extensional rheology and formation of beads-on-a-string structures in polymer solutions. J Non Newton Fluid Mech 137:137–148
Oliveira MSN, Alves MA, Pinho FT, McKinley GH (2007a) Viscous flow through microfabricated hyperbolic contractions. Exp Fluids 43:437–451
Oliveira MSN, Oliveira PJ, Pinho FT, Alves MA (2007b) Effect of contraction ratio upon viscoelastic flow in contractions: the axisymmetric case. J Non Newton Fluid Mech 147:92–108
Oliveira MSN, Alves MA, Pinho FT (2008a) Extensional effects in viscoelastic fluid flow through a micro-scale double cross-slot. AIP Conf Proc 1027:982–984
Oliveira MSN, Rodd LE, McKinley GH, Alves MA (2008b) Simulations of extensional flow in microrheometric devices. Microfluid Nanofluid 5:809–826
Oliveira MSN, Pinho FT, Poole RJ, Oliveira PJ, Alves MA (2009) Purely-elastic flow asymmetries in flow focusing devices. J Non Newton Fluid Mech 160:31–39
Oliveira MSN, Alves MA, Pinho FT (2012) Transport and mixing in laminar flows: from microfluidics to oceanic currents, Chap 6. Microfluidic flows of viscoelastic fluids. Wiley, New York
Padmanabhan M (1995) Measurement of extensional viscosity of viscoelastic liquid foods. J Food Eng 25:311–327
Padmanabhan M, Bhattacharya M (1993) Planar extensional viscosity of corn meal dough. J Food Eng 18:389–411
Pathak JA, Hudson SD (2006) Rheo-optics of equilibrium polymer solutions: wormlike micelles in elongational flow in a microfluidic cross-slot. Macromolecules 39:8782–8792
Perkins TT, Smith DE, Chu S (1997) Single polymer dynamics in an elongation flow. Science 314:216–221
Petrie CJS (1997) Three-dimensional presentation of extensional flow data. J Non Newton Fluid Mech 70:205–218
Petrie CJS (2006) Extensional viscosity: a critical discussion. J Non Newton Fluid Mech 137:15–23
Phelan FR Jr, Hudson SD, Handler MD (2005) Fluid dynamics analysis of channel flow geometries for materials characterization in microfluidic devices. Rheol Acta 45:59–71
Pipe C, McKinley GH (2009) Microfluidic rheometry. Mech Res Commun 36:110–120
Pipe C, Majmudar TS, McKinley GH (2008) High shear rate viscometry. Rheol Acta 47:621–642
Pollack MG, Fair RB, Shenderov AD (2010) Electrowetting-based actuation of liquid droplets for microfluidic applications. Appl Phys Lett 77:1725–1727
Poole RJ, Alves MA, Oliveira PJ (2007) Purely elastic flow asymmetries. Phys Rev Lett 99:164503
Remmelgas J, Singh P, Leal LG (1999) Computational studies of nonlinear elastic dumbbell models of boger fluids in a cross-slot flow. J Non Newton Fluid Mech 88:31–61
Ríos S, Díaz J, Galindo A, Soto E, Calderas F, Mena B (2002) Instrumentation and start up of a new elongational rheometer with preshearing history. Rev Sci Instrum 73:3007–3011
Roche M, Kellay H, Stone HA (2011) Heterogeneity and the role of normal stresses during the extensional thinning of non-Brownian shear-thickening fluids. Phys Rev Lett 107:34503
Rodd LE, Scott TP, Boger DV, Cooper-White JJ, McKinley GH (2005a) The inertio-elastic planar entry flow of low-viscosity elastic fluids in micro-fabricated geometries. J Non Newtonian Fluid Mech 129:1–22
Rodd LE, Scott TP, Cooper-White JJ, McKinley GH (2005b) Capillary break-up rheometry of low-viscosity elastic fluids. Appl Rheol 15:12–27
Rodd LE, Cooper-White JJ, Boger DV, McKinley GH (2007) The role of elasticity number in the entry flow of dilute polymer solutions in micro-fabricated contraction geometries. J Non Newtonian Fluid Mech 143:170–191
Rothstein JP (2008) Strong flows of viscoelastic wormlike micelle solutions. In: Binding DM, Walters K (eds) Rheology reviews. The British Society of Rheology
Rothstein JP, McKinley GH (1999) Extensional flow of a polystyrene boger fluid through a 4:1:4 axisymmetric contraction/expansion. J Non Newton Fluid Mech 86:61–88
Rothstein JP, McKinley GH (2001) The axisymmetric contraction–expansion: the role of extensional rheology on vortex growth dynamics and the enhanced pressure drop. J Non Newton Fluid Mech 98:33–63
Sattler R, Wagner C, Eggers J (2008) Blistering pattern and formation of nanofibers in capillary thinning of polymer solutions. Phys Rev Lett 100:164502
Schultz WW, Davis SH (1982) One-dimensional liquid fibers. J Rheol 26(4):331–345
Schweizer T (2000) The uniaxial elongational rheometer rme—six years of experience. Rheol Acta 39:428–443
Sentmanat M, Wang BN, McKinley GH (2005) Measuring the transient extensional rheology of polyethylene melts using the ser universal testing platform. J Rheol 49(3):585–606
Shaqfeh ESG (2005) The dynamics of single-molecule dna in flow. J Non Newton Fluid Mech 130:1–28
Song JH, Evans R, Lin YY, Hsu BN, Fair RB (2009) A scaling model for electrowetting-on-dielectric microfluidic actuators. Microfluid Nanofluid 7:75–89
Soulages J, Le Goupil F, Hostettler J, McKinley GH (2009a) An opposed-nozzle fixture for measuring the extensional properties of low viscosity liquids using a conventional controlled strain rheometer. In: Co A (ed) The Society of Rheology. 81st annual meeting program and abstracts, p 115
Soulages J, Oliveira MSN, Sousa PC, Alves MA, McKinley GH (2009b) Investigating the stability of viscoelastic stagnation flows in t-shaped microchannels. J Non Newton Fluid Mech 163:9–24
Sousa PC, Coelho PM, Oliveira MSN, Alves MA (2011) Laminar flow in three-dimensional square–square expansion. J Non Newton Fluid Mech 166:1033–1048
Squires TM, Mason TG (2010) Fluid mechanics of microrheology. Annu Rev Fluid Mech 42:413–438
Squires TM, Quake SR (2005) Microfluidics: fluid physics at the nanoliter scale. Rev Mod Phys 77:977–1026
Sridhar T (2000) From rheometry to rheology. Korea Austral Rheol J 12(1):39–53
Tan MK, Friend JR, Yeo LY (2009) Interfacial jetting phenomena induced by focusing surface vibrations. Phys Rev Lett 103:024501
Tanner RI (1988) Pressure-hole errors—an alternative approach. J Non Newtonian Fluid Mech 28:309–318
Tanyeri M, Johnson-Chavarria EM, Schroeder CM (2010) Hydrodynamic trap for single particles and cells. Appl Phys Lett 96:224101
Tanyeri M, Ranka M, Sittipolkul N, Schroeder CM (2011) A microfluidic-based hydrodynamic trap: design and implementation. Lab Chip 11:1786–1794
Taylor GI (1934) The formation of emulsions in definable fields of flow. Proc Roy Soc Lond Ser A 146:501–523
Trouton FT (1906) On the coefficient of viscous traction and its relation to that of viscosity. Proc Roy Soc Lond 77:426–440
Wang J, James DF (2011) Lubricated extensional flow of viscoelastic fluid in a convergent microchannel. J Rheol 55:1103–1126
Whitesides GM (2006) The origins and future of microfluidics. Nature 42:368–370
Zheng R, Tanner RI, Fan XJ (2011) Injection molding: integration of theory and modeling methods. Springer, Berlin
Acknowledgments
The authors would like to acknowledge Fundação para a Ciência e a Tecnologia (FCT), COMPETE and FEDER for financial support through projects PTDC/EME-MFE/099109/2008, PTDC/EME-MFE/114322/2009, PTDC/EQU-FTT/118716/2010 and the scholarship SFRH/BPD /69663/2010. The authors also thank Dr. Rob Poole (University of Liverpool) for helpful comments.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Galindo-Rosales, F.J., Alves, M.A. & Oliveira, M.S.N. Microdevices for extensional rheometry of low viscosity elastic liquids: a review. Microfluid Nanofluid 14, 1–19 (2013). https://doi.org/10.1007/s10404-012-1028-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10404-012-1028-1