Vertical coupling and dynamical source for the intraseasonal variability in the deep Kuroshio Extension

Abstract

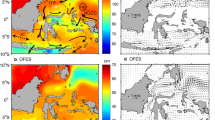
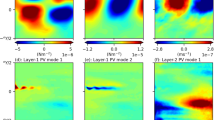
In the power spectrum, the upper and deep parts of the Kuroshio Extension have distinctly different peaks. The former peaks around 200 days, while the latter is mainly at the intraseasonal band. How the upper meandering jet links the deep intraseasonal eddy current then makes an issue. In this study, it is investigated using the outputs from a 1/10° ocean general circulation model. The theoretical framework is the theory of canonical transfer that gives a faithful representation of the energy transfers among distinct scales in the light of energy conservation, and a space-time-dependent energetics formalism with three-scale windows, namely, a slowly varying background flow window, an intraseasonal eddy window, and a high-frequency synoptic eddy window. The vertical pressure work is found to be the primary driver of the deep intraseasonal variability; it transports intraseasonal kinetic energy (IKE) to the deep layer (below 3000 m) from the interior layer (~ 200–3000 m) where the intraseasonal variability is generated through baroclinic instabilities. Besides the downward IKE fluxes, significant upward fluxes also exist in the surface mixed layer of the upstream Kuroshio Extension (above ~ 200 m, west of 146°E) as a comparable IKE source as baroclinic instability. The accumulated upstream IKE is advected eastward, forming the primary KE source of the intraseasonal variability in the surface layer of the downstream Kuroshio Extension (east of 146°E). Regarding the IKE sinks, the deep layer IKE is damped by bottom drag, while in the surface (interior) layer, IKE is damped by the wind stress and may also be given back to the background flow (the up/downward IKE fluxes via pressure work).

Appendix

1.1 Multiscale window transform (MWT)

The Lorenz (1955) energy cycle theory has now been a standard approach to investigate the eddy-mean flow interaction and hydro-dynamical stability in atmosphere and ocean sciences (e.g., Böning and Budich 1992; Ivchenko et al. 1997; von Storch et al. 2012). The theory is based on Reynolds decomposition, with an ensemble mean (usually time mean in oceanic studies for practical reason) and its associated “eddy” component (i.e., deviation from the mean). Such decomposition results in four mechanical energy reservoirs, i.e., the kinetic energy (KE) and available potential energy (APE) for the mean flow and those for the eddy flow, and hence, energy exchanges among the four reservoirs can be quantitatively evaluated. It should be noted that the classical Lorenz energy cycle is formulated in a global form, and therefore is not suitable for investigation of energy burst processes which are in nature highly localized (i.e., nonstationary/inhomogeneous). A remedy for this is to use filter to separate a field into several parts and then take the square of the filtered part as the energy for that part. This practice, which has appeared trivially in a lot of publications (e.g., Hsu et al. 2011; Chapman et al. 2015), is by no means trivial. To see why, suppose a time series u(t) consists of two sinusoidal components with frequencies ω₀ and ω₁ (suppose ω₀ < ω₁), i.e.:

(10)

The energies for the low- and high-frequency component are the square of their respective transform coefficients, i.e., ${a}_0^2+{b}_0^2$ and ${a}_1^2+{b}_1^2$, which are obviously not equal to the square of the respective reconstructed (or filtered) fields, i.e., ${\left[\overline{u}(t)\right]}^2$ and [u^′(t)]². From the above example, it is important to realize that transform coefficients, and hence multiscale energy, are concepts in phase space, while reconstructed fields are concepts in physical space. The two concepts are related through the Parseval equality. Particularly, when $\overline{u}$ is a constant (i.e., time mean), it is easy to obtain ${a}_1^2+{b}_1^2=\overline{{\left[{u}^{\prime }(t)\right]}^2}$. This explains why the time-averaging operator in the Reynolds-based energetics formalism cannot be simply removed.

So it is by no means a trivial problem to obtain a localized multiscale energy (here, localized means time-dependent since the decomposition is conducted in the time domain). General filters fail in the presentation of multiscale energy because they only yield reconstructions (filtered variables) but no transform coefficients. The multiscale window transform (MWT), developed by Liang and Anderson (2007), is used for this very purpose. Briefly speaking, MWT is a functional analysis tool that orthogonally decomposes a function space into a direct sum of subspaces, or scale windows as termed by Liang and Anderson (2007). Just like the Fourier transform and inverse transform pair, there exists a transform-reconstruction pair, which is the MWT and its peer, multiscale window reconstruction (MWR). For each MWR of a time series u(t), denoted as u^∼ϖ(t), where ϖ indicates a specific scale window, there is a corresponding transform coefficient, denoted as ${\hat{u}}_n^{\sim \varpi }$ with n the discrete time step. The time-dependent energy on window ϖ proves to be the square of the MWT coefficients, i.e., ${\left({\hat{u}}_n^{\sim \varpi}\right)}^2$(up to some constant; cf. Liang and Anderson 2007).

1.2 Canonical transfer

As we mentioned in Section 2.b, the MWT-based canonical transfer bears a conservation property which is not satisfied in classical Reynolds-based formalism. To see the difference between the canonical transfer and that appearing in the classical Reynolds-based formalism, consider a scalar field T in an incompressible flow v, with diffusion neglected for simplicity:

$$ \frac{\partial T}{\partial t}+\nabla \cdotp \left(\mathbf{v}T\right)=0. $$

(11)

By decomposing the original field into mean and eddy components, denoted by overbar and prime, respectively, one can obtain the energy equations for the mean and the eddy fields:

$$ \frac{\partial }{\partial t}\left(\frac{1}{2}{\overline{T}}^2\right)+\nabla \cdotp \left(\frac{1}{2}\overline{\mathbf{v}}{\overline{T}}^2\right)=-\overline{T}\nabla \cdotp \left(\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\right), $$

(12)

$$ \frac{\partial }{\partial t}\left(\frac{1}{2}\overline{{T^{\prime}}^2}\right)+\nabla \cdotp \left(\frac{1}{2}\overline{\mathbf{v}{T^{\prime}}^2}\right)=-\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\cdotp \nabla \overline{T}, $$

(13)

where the second terms on the left-hand side of Eqs. (12) and (13) are the nonlocal transport processes by advection, and the terms on the right-hand sides are considered as energy transfers associated with eddy-mean flow interactions. It is important to note that the two terms on right-hand sides generally do not cancel out, meaning that the so-obtained transfer does not conserve energy among scales. This problem is actually not new and has long been realized that the transfer might not have a unique expression (e.g., Holopainen 1978; Plumb 1983). Based on MWT, Liang (2016) derived the energy equations for the special case (11):

$$ \frac{\partial }{\partial t}\left(\frac{1}{2}\overline{{T^{\prime}}^2}\right)+\nabla \cdotp \left(\frac{1}{2}\overline{\mathbf{v}{T^{\prime}}^2}+\frac{1}{2}\overline{T}\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\right)=\Gamma . $$

(14)

$$ \frac{\partial }{\partial t}\left(\frac{1}{2}{\overline{T}}^2\right)+\nabla \cdotp \left(\frac{1}{2}\overline{\mathbf{v}}{\overline{T}}^2+\frac{1}{2}\overline{T}\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\right)=-\Gamma, $$

(15)

where the canonical transfer:

$$ \Gamma =\frac{1}{2}\left[\overline{T}\nabla \cdotp \left(\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\right)-\overline{{\mathbf{v}}^{\prime }{T}^{\prime }}\cdotp \nabla \overline{T}\right]. $$

(16)

Now the transfer terms on the right-hand sides of Eqs. (14) and (15) sum to zero, distinctly different from the classical ones. As a validation, Liang and Robinson (2007) showed that, for a benchmark barotropic model whose instability structure is analytically known, the traditional formalism fails to give the correct source of barotropic instability, while canonical transfer Γ does.