Abstract
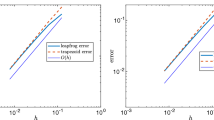
The purpose of this work is to study solution techniques for problems involving fractional powers of symmetric coercive elliptic operators in a bounded domain with Dirichlet boundary conditions. These operators can be realized as the Dirichlet-to-Neumann map for a degenerate/singular elliptic problem posed on a semi-infinite cylinder, which we analyze in the framework of weighted Sobolev spaces. Motivated by the rapid decay of the solution to this problem, we propose a truncation that is suitable for numerical approximation. We discretize this truncation using first degree tensor product finite elements. We derive a priori error estimates in weighted Sobolev spaces. The estimates exhibit optimal regularity but suboptimal order for quasi-uniform meshes. For anisotropic meshes instead, they are quasi-optimal in both order and regularity. We present numerical experiments to illustrate the method’s performance.






Similar content being viewed by others
References
M. Abramowitz and I.A. Stegun. Handbook of mathematical functions with formulas, graphs, and mathematical tables, volume 55 of National Bureau of Standards Applied Mathematics Series. For sale by the Superintendent of Documents, U.S. Government Printing Office, Washington, D.C., 1964.
G. Acosta. Lagrange and average interpolation over 3D anisotropic elements. J. Comput. Appl. Math., 135(1):91–109, 2001.
R.A. Adams. Sobolev spaces. Academic Press [A subsidiary of Harcourt Brace Jovanovich, Publishers], New York-London, 1975. Pure and Applied Mathematics, Vol. 65.
T. Apel. Interpolation of non-smooth functions on anisotropic finite element meshes. M2AN Math. Model. Numer. Anal., 33(6):1149–1185, 1999.
O.G. Bakunin. Turbulence and diffusion. Springer Series in Synergetics. Springer-Verlag, Berlin, 2008. Scaling versus equations.
W. Bangerth, R. Hartmann, and G. Kanschat. deal.II–differential equations analysis library. Technical Reference: http://dealii.org.
W. Bangerth, R. Hartmann, and G. Kanschat. deal.II–a general-purpose object-oriented finite element library. ACM Trans. Math. Software, 33(4):Art. 24, 27, 2007.
P.W. Bates. On some nonlocal evolution equations arising in materials science. In Nonlinear dynamics and evolution equations, volume 48 of Fields Inst. Commun., pages 13–52. Amer. Math. Soc., Providence, RI, 2006.
Z. Belhachmi, Ch. Bernardi, and S. Deparis. Weighted Clément operator and application to the finite element discretization of the axisymmetric Stokes problem. Numer. Math., 105(2):217–247, 2006.
D.A. Benson, S.W. Wheatcraft, and M.M. Meerschaert. Application of a fractional advection-dispersion equation. Water Resources Res., 36:91–109, 2000.
C. Bernardi. Optimal finite-element interpolation on curved domains. SIAM J. Numer. Anal., 26(5):1212–1240, 1989.
J. Bertoin. Lévy processes, volume 121 of Cambridge Tracts in Mathematics. Cambridge University Press, Cambridge, 1996.
M.Š. Birman and M.Z. Solomjak. Spektralnaya teoriya samosopryazhennykh operatorov v gilbertovom prostranstve. Leningrad. Univ., Leningrad, 1980.
K. Bogdan, K. Burdy, and Chen Z.Q. Censored stable processes. Probab. Theory Related Fields, 127(R-2):89–152, 2003.
A. Bonito and J.E. Pasciak. Numerical approximation of fractional powers of elliptic operators. Math. Comp. (to appear), 2014.
C. Brändle, E. Colorado, A. de Pablo, and U. Sánchez. A concave-convex elliptic problem involving the fractional Laplacian. Proc. Roy. Soc. Edinburgh Sect. A, 143(1):39–71, 2013.
S.C. Brenner and L.R. Scott. The mathematical theory of finite element methods, volume 15 of Texts in Applied Mathematics. Springer, New York, third edition, 2008.
Kevin Burrage, Nicholas Hale, and David Kay. An efficient implicit FEM scheme for fractional-in-space reaction-diffusion equations. SIAM J. Sci. Comput., 34(4):A2145–A2172, 2012.
X. Cabré and Y. Sire. Nonlinear equations for fractional Laplacians ii: Existence, uniqueness and qualitative properties of solutions. arXiv:1111.0796v1, 2011.
X. Cabré and J. Tan. Positive solutions of nonlinear problems involving the square root of the Laplacian. Adv. Math., 224(5):2052–2093, 2010.
L. Caffarelli and L. Silvestre. An extension problem related to the fractional Laplacian. Comm. Partial Differential Equations, 32(7–9):1245–1260, 2007.
A. Capella, J. Dávila, L. Dupaigne, and Y. Sire. Regularity of radial extremal solutions for some non-local semilinear equations. Comm. Partial Differential Equations, 36(8):1353–1384, 2011.
P. Carr, H. Geman, D.B. Madan, and M. Yor. The fine structure of asset returns: An empirical investigation. Journal of Business, 75:305–332, 2002.
P.G. Ciarlet. The finite element method for elliptic problems, volume 40 of Classics in Applied Mathematics. Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA, 2002. Reprint of the 1978 original [North-Holland, Amsterdam; MR0520174 (58 #25001)].
P.G. Ciarlet and P.-A. Raviart. Interpolation theory over curved elements, with applications to finite element methods. Comput. Methods Appl. Mech. Engrg., 1:217–249, 1972.
P. Clément. Approximation by finite element functions using local regularization. RAIRO Analyse Numérique, 9(2):77–84, 1975.
J. Cushman and T. Glinn. Nonlocal dispersion in media with continuously evolving scales of heterogeneity. Trans. Porous Media, 13:123–138, 1993.
E. Di Nezza, G. Palatucci, and E. Valdinoci. Hitchhiker’s guide to the fractional Sobolev spaces. Bull. Sci. Math., 136(5):521–573, 2012.
T. Dupont and R. Scott. Polynomial approximation of functions in sobolev spaces. Math. Comp., 34:441–463, 1980.
R.G. Durán and A.L. Lombardi. Error estimates on anisotropic \(Q_1\) elements for functions in weighted Sobolev spaces. Math. Comp., 74(252):1679–1706 (electronic), 2005.
R.G. Durán, A.L. Lombardi, and M.I. Prieto. Superconvergence for finite element approximation of a convection-diffusion equation using graded meshes. IMA Journal of Numerical Analysis, 32(2):511–533, 2012.
R.G. Durán and F. López García. Solutions of the divergence and Korn inequalities on domains with an external cusp. Ann. Acad. Sci. Fenn. Math., 35(2):421–438, 2010.
G. Duvaut and J.-L. Lions. Inequalities in mechanics and physics. Springer-Verlag, Berlin, 1976. Translated from the French by C. W. John, Grundlehren der Mathematischen Wissenschaften, 219.
A.C. Eringen. Nonlocal continuum field theories. Springer-Verlag, New York, 2002.
L.C. Evans. Partial differential equations, volume 19 of Graduate Studies in Mathematics. American Mathematical Society, Providence, RI, second edition, 2010.
E. B. Fabes, C.E. Kenig, and R.P. Serapioni. The local regularity of solutions of degenerate elliptic equations. Comm. Partial Differential Equations, 7(1):77–116, 1982.
D. Gilbarg and N.S. Trudinger. Elliptic partial differential equations of second order. Classics in Mathematics. Springer-Verlag, Berlin, 2001. Reprint of the 1998 edition.
G. Gilboa and S. Osher. Nonlocal operators with applications to image processing. Multiscale Model. Simul., 7(3):1005–1028, 2008.
V. Gol’dshtein and A. Ukhlov. Weighted Sobolev spaces and embedding theorems. Trans. Amer. Math. Soc., 361(7):3829–3850, 2009.
Q.Y. Guan and Z.M. Ma. Reflected symmetric \(\alpha \)-stable processes and regional fractional laplacian. Probab. Theory Related Fields, 134(2):649–694, 2006.
J. Heinonen, T. Kilpeläinen, and O. Martio. Nonlinear potential theory of degenerate elliptic equations. Oxford Mathematical Monographs. The Clarendon Press Oxford University Press, New York, 1993. Oxford Science Publications.
M. Ilic, F. Liu, I. Turner, and V. Anh. Numerical approximation of a fractional-in-space diffusion equation. I. Fract. Calc. Appl. Anal., 8(3):323–341, 2005.
M. Ilic, F. Liu, I. Turner, and V. Anh. Numerical approximation of a fractional-in-space diffusion equation. II. With nonhomogeneous boundary conditions. Fract. Calc. Appl. Anal., 9(4):333–349, 2006.
V.G. Korneev. The construction of variational difference schemes of a high order of accuracy. Vestnik Leningrad. Univ., 25(19):28–40, 1970. (In Russian).
V.G. Korneev and S.E. Ponomarev. Application of curvilinear finite elements in schemes for solution of \(2n\)-order linear elliptic equations. I. Čisl. Metody Meh. Splošn. Sredy, 5(5):78–97, 1974. (In Russian).
A. Kufner. Weighted Sobolev spaces. A Wiley-Interscience Publication. John Wiley & Sons Inc., New York, 1985. Translated from the Czech.
N.S. Landkof. Foundations of modern potential theory. Springer-Verlag, New York, 1972. Translated from the Russian by A. P. Doohovskoy, Die Grundlehren der mathematischen Wissenschaften, Band 180.
J.-L. Lions and E. Magenes. Non-homogeneous boundary value problems and applications. Vol. I. Springer-Verlag, New York, 1972. Translated from the French by P. Kenneth, Die Grundlehren der mathematischen Wissenschaften, Band 181.
B.M. McCay and M.N.L. Narasimhan. Theory of nonlocal electromagnetic fluids. Arch. Mech. (Arch. Mech. Stos.) 33(3):365–384, 1981.
W. McLean. Strongly elliptic systems and boundary integral equations. Cambridge University Press, Cambridge, 2000.
K.S. Miller and S.G. Samko. Completely monotonic functions. Integral Transform. Spec. Funct., 12(4):389–402, 2001.
B. Muckenhoupt. Weighted norm inequalities for the Hardy maximal function. Trans. Amer. Math. Soc., 165:207–226, 1972.
R.H. Nochetto, E. Otárola, and A.J Salgado. A pde approach to space-time fractional diffusion. arXiv:1404.0068, 2014.
R.H. Nochetto, E. Otárola, and A.J. Salgado. Piecewise polynomial interpolation in Muckenhoupt weighted Sobolev spaces and appications. arXiv:1402.1916, 2014.
G. Savaré. Regularity and perturbation results for mixed second order elliptic problems. Comm. Partial Differential Equations, 22(5–6):869–899, 1997.
L.R. Scott and S. Zhang. Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comp., 54(190):483–493, 1990.
R. Servadei and E. Valdinoci. On the spectrum of two different fractional operators. preprint, 2012.
S.A. Silling. Reformulation of elasticity theory for discontinuities and long-range forces. J. Mech. Phys. Solids, 48(1):175–209, 2000.
E.M. Stein. Singular integrals and differentiability properties of functions. Princeton Mathematical Series, No. 30. Princeton University Press, Princeton, N.J., 1970.
P.R. Stinga and J.L. Torrea. Extension problem and Harnack’s inequality for some fractional operators. Comm. Partial Differential Equations, 35(11):2092–2122, 2010.
L. Tartar. An introduction to Sobolev spaces and interpolation spaces, volume 3 of Lecture Notes of the Unione Matematica Italiana. Springer, Berlin, 2007.
Q. Yang, I. Turner, F. Liu, and M. Ilić. Novel numerical methods for solving the time-space fractional diffusion equation in two dimensions. SIAM J. Sci. Comput., 33(3):1159–1180, 2011.
Acknowledgments
This work is supported by NSF Grants DMS-1109325 and DMS-0807811. A.J.S. is also supported by NSF Grant DMS-1008058 and an AMS-Simons Grant. E.O. is supported by the Conicyt–Fulbright Fellowship Beca Igualdad de Oportunidades.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Albert Cohen.
Rights and permissions
About this article
Cite this article
Nochetto, R.H., Otárola, E. & Salgado, A.J. A PDE Approach to Fractional Diffusion in General Domains: A Priori Error Analysis. Found Comput Math 15, 733–791 (2015). https://doi.org/10.1007/s10208-014-9208-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10208-014-9208-x
Keywords
- Fractional diffusion
- Finite elements
- Nonlocal operators
- Degenerate and singular equations
- Second-order elliptic operators
- Anisotropic elements