Skip to main content
SpringerLink
Log in

Bifurcations of limit cycles from a quintic Hamiltonian system with a heteroclinic cycle

  • Published:
Acta Mathematica Sinica, English Series Aims and scope Submit manuscript

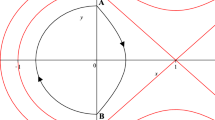
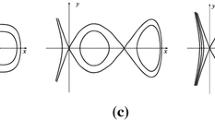
Abstract

In this paper, we consider Liénard systems of the form

$$\frac{{dx}} {{dt}} = y, \frac{{dy}} {{dt}} = - \left( {x + bx^3 - x^5 } \right) + \varepsilon \left( {\alpha + \beta x^2 + \gamma x^4 } \right)y,$$

where b ∈ ℝ, 0 < |∈| ≪ 1, (α, β, γ) ∈ D ∈ ℝ3 and D is bounded. We prove that for |b| ≫ 1 (b < 0) the least upper bound of the number of isolated zeros of the related Abelian integrals is 2 (counting the multiplicity) and this upper bound is a sharp one.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Asheghi, R., Zangeneh, H. R. Z.: Bifurcations of limit cycles from quintic Hamiltonian systems with an eye-figure loop. Nonlinear Anal., 68, 2957–2976 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  2. Asheghi, R., Zangeneh, H. R. Z.: Bifurcations of limit cycles from quintic Hamiltonian systems with an eye-figure loop (II). Nonlinear Anal., 69, 4143–4162 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  3. Asheghi, R., Zangeneh, H. R. Z.: Bifurcations of limit cycles from quintic Hamiltonian system with a double cuspidal loop. Comput. Math. Appl., 59, 1409–1418 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  4. Chicone, C.: The monotonicity of the period function for planar Hamiltonian vector fields. J. Differential Equations, 69, 310–321 (1987)

    Article  MATH  MathSciNet  Google Scholar 

  5. Dumortier, F., Li, C.: Perturbations from an elliptic Hamiltonian of degree four: (I) saddle loop and two saddle cycle. J. Differential Equations, 176, 114–157 (2001); (II) cuspidal loop. J. Differential Equations, 175, 209–243 (2001); (III) Global center. J. Differential Equations, 188, 473–511 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  6. Han, M., Yang, J., Tarta, A., et al.: Limit cycles near Homoclinic and heterclinic loops. J. Dynam. Differential Equations, 20, 923–944 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  7. Horozov, E., Iliev, I.: On the number of limit cycles in perturbations of quadratic Hamiltonian systems. Proc. London Math. Soc., 69, 198–224 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  8. Li, C., Zhang, Z.: A criterion for determinging the monotonicity of the ratio of two Abelian integrals. J. Differential Equations, 124, 407–424 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  9. Li, C., Zhang, Z.: Remarks on 16th weak Hilbert problem for n = 2. Nonlinearity, 15, 1975–1992 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  10. Petrov, G. S.: Number of zeros of complete elliptic integrals. Funct. Anal. Appl., 18, 73–74 (1984); English transl., Funct. Anal. Appl., 18, 148–149 (1984)

    Article  MATH  Google Scholar 

  11. Petrov, G. S.: Elliptic integrals and their nonoscillation. Funct. Anal. Appl., 20, 46–49 (1986); English transl., Funct. Anal. Appl., 20, 37–40 (1986)

    Article  Google Scholar 

  12. Petrov, G. S.: The Chebyschev property of elliptic integrals. Funct. Anal. Appl., 22, 83–84 (1986); English transl., Funct. Anal. Appl., 22, 72–73 (1988)

    Google Scholar 

  13. Pontryagin, D.: On dynamical systems close to hamiltonian ones. Zh. Exp. Theor. Phys., 4, 234–238 (1934)

    Google Scholar 

  14. Sabatini, M.: On the period function of x″ + f(x)x ′2 + g(x) = 0. J. Differential Equations, 196, 151–168 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  15. Wang, J., Xiao, D.: On the number of limit cycles in small perturbations of a class of hyper-elliptic Hamiltonian systems with one nilpotent saddle. J. Differential Equations, 250, 2227–2243 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  16. Zhang, T., Tadé, M. O., Tian, Y.: On the zeros of the Abelian integrals for a class of Liénard systems. Physics Letters A, 358, 262–274 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  17. Zhao, L., Qi, M., Liu, C.: The cyclicity of period annuli of a class of quintic Hamiltonian systems. J. Math. Anal. Appl., 403, 391–407 (2013)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Beijing Normal University, Laboratory of Mathematics and Complex Systems, Ministry of Education, Beijing, 100875, P. R. China

    Li Qin Zhao & De Ping Li

Authors
  1. Li Qin Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. De Ping Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Li Qin Zhao.

Additional information

Supported by National Natural Science Foundation of China (Grant No. 11271046)

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhao, L.Q., Li, D.P. Bifurcations of limit cycles from a quintic Hamiltonian system with a heteroclinic cycle. Acta. Math. Sin.-English Ser. 30, 411–422 (2014). https://doi.org/10.1007/s10114-014-2615-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10114-014-2615-8

Keywords

MR(2010) Subject Classification

Search

Navigation