Skip to main content
SpringerLink
Log in

Parametric dependencies of the post-ultimate strength behavior of a ship’s hull girder in waves

  • Original Article
  • Published:
Journal of Marine Science and Technology Aims and scope Submit manuscript

Abstract

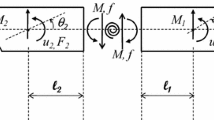
To rationally assess the consequence of a ship’s hull girder collapse, it is necessary to know the post-ultimate strength behavior of the hull girder including the global deformation and motions under extreme wave-induced loads. In the foregoing research, the authors proposed a numerical analysis system to predict the collapse behavior in waves including the post-ultimate strength behavior. The primary objective of the present paper is to clarify the parametric dependencies of the severity of the collapse in a rational manner. The parameters may include those related to load-carrying capacity and the extreme loads. To this end, an analytical solution to describe the post-ultimate strength behavior is derived. Assuming that a plastic hinge is formed at the midship during the collapse procedure, the whole ship is modeled as a two-rigid-bodies system connected to each other amidship via a nonlinear rotational spring, which represents the nonlinear relationship between the bending moment and the rotational angle. The relationship may be modeled as piece-wise linear curves. It is further assumed that large motions and elastic/plastic deformations of the hull girder may not affect the load evaluations, and that the hull girder is subjected to a large single wave. Some important parameters to predict the severity of the collapse are specified based on the analytical solution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. IACS (2006a) Common structural rules for double hull oil tankers, Sect 9

  2. IACS (2006b) Common structural rules for bulk carriers, Chap 5

  3. Yao T, Nikolov PI (1991) Progressive collapse analysis of a ship’s hull under longitudinal bending. J Soc Nav Arch Jpn 170:449–461

    Article  Google Scholar 

  4. Yao T, Nikolov PI (1992) Progressive collapse analysis of a ship’s hull under longitudinal bending (2nd report). J Soc Nav Arch Jpn 172:437–446

    Article  Google Scholar 

  5. Paik JK, Kim BJ (2008) Progressive collapse analysis of thin-walled box columns. Thin Walled Struct 46:541–550

    Article  Google Scholar 

  6. Gordo JM, Guedes Soares C (1996) Approximate method to evaluate the hull girder collapse strength. Mar Struct 9:449–470

    Article  Google Scholar 

  7. Lehmann E (2006) Discussion on “Report of committee III.1: ultimate strength”. In: Proceedings of the 16th ISSC (3), Southampton, UK, pp 121–131

  8. Yao T, Fujikubo M, Iijima K, Pei Z (2009) Total system including capacity calculation applying ISUM/FEM and loads calculation for progressive collapse analysis of ship’s hull girder in longitudinal bending. In: Proceedings of the 19th international offshore and polar engineering conference, ISOPE2009, Osaka, Japan, pp 706–713

  9. Masaoka K, Okada H (2003) A numerical approach for ship hull girder collapse behavior in waves. In: Proceedings of the thirteenth international offshore and polar engineering conference, Honolulu, Hawaii, USA, pp 369–375

  10. Xu W, Iijima K, Fujikubo M (2011) Hydro-elastoplasticity approach to ship’s hull girder collapse behavior in waves. In: Proceedings of MARSTRUCT 2011, Hamburg, Germany, pp 239–247

  11. Kimura K, Iijima K, Wada R, Xu W, Fujikubo M (2010) Dynamic collapse behavior of ship’s hull girder in waves. In: Advanced maritime engineering conference and 4th Pan Asian association of maritime engineering societies forum, Singapore, pp 261–266

  12. Iijima K, Kimura K, Xu W, Fujikubo M (2011) Hydroelasto-plasticity approach to predicting the post-ultimate strength behavior of ship’s hull girder in waves (submitted)

  13. Wada R, Iijima K, Kimura K, Xu W, Fujikubo M (2010) Development of a design methodology for a scaled model for hydro-elastoplasticity of a hull girder in waves. In: Advanced maritime engineering conference and 4th Pan Asian association of maritime engineering societies forum, Singapore, pp 248–253

  14. Xu W, Iijima K, Fujikubo M (2011) Investigation into post-ultimate strength behavior of ship’s hull girder in waves by analytical solution. In: Proceedings of the 30th international conference on ocean, offshore and arctic engineering, OMAE2011, Rotterdam, Netherlands, OMAE2011-49617

Download references

Acknowledgments

This research was partly supported by the Ministry of Education, Science, Sports and Culture, Grant-in-Aid for Scientific Research (A), (20246126), 2010.

Author information

Authors and Affiliations

  1. Department of Naval Architecture and Ocean Engineering, Osaka University, Suita, Japan

    Weijun Xu, Kazuhiro Iijima & Masahiko Fujikubo

  2. College of Shipbuilding Engineering, Harbin Engineering University, Harbin, China

    Weijun Xu

Authors
  1. Weijun Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kazuhiro Iijima
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Masahiko Fujikubo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Weijun Xu.

Appendix

Appendix

The parameters in the solution of each path

  1. 1.

    Path OA

$$ \theta_{\text{OA}} = e^{ - nt} (C_{1} \cos \omega_{{{\text{d}}1}} t + C_{2} \sin \omega_{{{\text{d}}1}} t) + R_{1} \sin \omega_{0} t + T_{1} \cos \omega_{0} t + \frac{{M_{\text{s}} }}{{\omega_{1}^{2} I}} $$
$$ R_{1} = \frac{{M_{0} }}{I} \cdot \frac{{\omega_{1}^{2} - \omega_{0}^{2} }}{{(\omega_{1}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} \, $$
$$ T_{1} = - \frac{{M_{0} }}{I} \cdot \frac{{2n\omega_{0} }}{{(\omega_{1}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} $$
$$ C_{1} = \frac{{M_{0} }}{I} \cdot \frac{{2n\omega_{0} }}{{(\omega_{1}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} - \frac{{M_{\text{s}} }}{{\omega_{1}^{2} I}} $$
$$ C_{2} = \frac{{M_{0} \omega_{0} }}{I} \cdot \frac{{2n^{2} - \omega_{1}^{2} + \omega_{0}^{2} }}{{\sqrt {\omega_{1}^{2} - n^{2} } \left[ {(\omega_{1}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} } \right]}} $$
$$ \omega_{1}^{2} = \frac{{(2k_{{{\text{s}}1}} + \rho gV \cdot GM)}}{I} $$
$$ \omega_{{{\text{d}}1}}^{2} = \omega_{1}^{2} - n^{2} ,\quad n = \frac{C}{2I},\quad k_{{{\text{s}}1}} = k_{1} $$
  1. 2.

    Path AB

$$ \theta_{\text{AB}} = D_{1} e^{{\omega_{21} t}} + D_{2} e^{{\omega_{22} t}} + R_{2} \sin \omega_{0} t + T_{2} \cos \omega_{0} t + \frac{{2k_{2} \theta_{\text{OA}} (t_{1} ) - 2M_{\text{u}} + M_{\text{s}} }}{{\omega_{2}^{2} I}} $$
$$ R_{2} = \frac{{M_{0} }}{I} \cdot \frac{{\omega_{2}^{2} - \omega_{0}^{2} }}{{(\omega_{2}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }},\quad T_{2} = - \frac{{M_{0} }}{I} \cdot \frac{{2n\omega_{0} }}{{(\omega_{2}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} \, $$
$$ D_{1} = \frac{{\omega_{22} R^{\prime }_{2} - T^{\prime }_{2} }}{{(\omega_{22} - \omega_{21} )e^{{\omega_{21} t_{1} }} }} $$
$$ D_{2} = \frac{{\omega_{21} R^{\prime }_{2} - T^{\prime }_{2} \, }}{{(\omega_{21} - \omega_{22} )e^{{\omega_{22} t_{1} }} }} $$
$$ \omega_{2}^{2} = \frac{{2k_{2} + \rho gV \cdot GM}}{I} $$
$$ \omega_{21} = - n + \sqrt {n^{2} - \omega_{2}^{2} } ,\quad \omega_{22} = - n - \sqrt {n^{2} - \omega_{2}^{2} } $$
$$ \begin{aligned} R^{\prime }_{2} & = e^{{ - nt_{1} }} (C_{1} \cos \omega_{d1} t_{1} + C_{2} \sin \omega_{{{\text{d}}1}} t_{1} ) + (R_{1} - R_{2} )\sin \omega_{0} t_{1} \\ & \quad + (T_{1} - T_{2} )\cos \omega_{0} t_{1} - \frac{{2k_{2} \theta_{\text{OA}} (t_{1} ) - 2M_{\text{u}} + M_{\text{s}} }}{{\omega_{2}^{2} I}} + \frac{{M_{\text{s}} }}{{\omega_{1}^{2} I}} \\ \end{aligned} $$
$$ \begin{aligned} T_{2}^{\prime } & = - ne^{{ - nt_{1} }} (C_{1} \cos \omega_{{{\text{d}}1}} t_{1} + C_{2} \sin \omega_{{{\text{d}}1}} t_{1} ) \\ & \quad + e^{{ - nt_{1} }} ( - C_{1} \omega_{{{\text{d}}1}} \sin \omega_{{{\text{d}}1}} t_{1} + C_{2} \omega_{{{\text{d}}1}} \cos \omega_{{{\text{d}}1}} t_{1} ) \\ & \quad + (R_{1} - R_{2} )\omega_{0} \cos \omega_{0} t_{1} - (T_{1} - T_{2} )\omega_{0} \sin \omega_{0} t_{1} \\ \end{aligned} $$
  1. 3.

    Path BC

$$ \theta_{\text{BC}} = e^{ - nt} (E_{1} \cos \omega_{{{\text{d}}3}} t + E_{2} \sin \omega_{{{\text{d}}3}} t) + R_{3} \sin \omega_{0} t + T_{3} \cos \omega_{0} t + \frac{{2k_{3} \theta_{\text{AB}} (t_{2} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{3}^{2} I}} $$
$$ R_{3} = \frac{{M_{0} }}{I} \cdot \frac{{\omega_{3}^{2} - \omega_{0}^{2} }}{{(\omega_{3}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }},\quad T_{3} = - \frac{{M_{0} }}{I} \cdot \frac{{2n\omega_{0} }}{{(\omega_{3}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} \, $$
$$ E_{1} = R^{\prime }_{3} \cos \omega_{{{\text{d}}3}} t_{2} - T^{\prime }_{3} \sin \omega_{{{\text{d}}3}} t_{2} $$
$$ E_{2} = R^{\prime }_{3} \sin \omega_{{{\text{d}}3}} t_{2} + T^{\prime }_{3} \cos \omega_{{{\text{d}}3}} t_{2} $$
$$ \omega_{{{\text{d}}3}}^{2} = \omega_{3}^{2} - n^{2} ,\quad \omega_{3}^{2} = \frac{\rho gV \cdot GM}{I} $$
$$ \begin{aligned} R^{\prime }_{3} & = D_{1} e^{{(\omega_{21} + n)t_{2} }} + D_{2} e^{{(\omega_{22} + n)t_{2} }} + e^{{nt_{2} }} (R_{2} - R_{3} )\sin \omega_{0} t_{2} \\ & \quad + e^{{nt_{2} }} (T_{2} - T_{3} )\cos \omega_{0} t_{2} + e^{{nt_{2} }} \left[ {\frac{{2k_{2} \theta_{\text{OA}} (t_{1} ) - 2M_{\text{u}} + M_{\text{s}} }}{{\omega_{2}^{2} I}} + \frac{{2M_{\text{BC}} - M_{\text{s}} }}{{\omega_{3}^{2} I}}} \right] \\ \end{aligned} $$
$$ \begin{aligned} T^{\prime }_{3} & = \frac{{\omega_{21} + n}}{{\omega_{{{\text{d}}3}} }}D_{1} e^{{(\omega_{21} + n)t_{2} }} + \frac{{\omega_{22} + n}}{{\omega_{{{\text{d}}3}} }}D_{2} e^{{(\omega_{22} + n)t_{2} }} \\ & \quad + e^{{nt_{2} }} (R_{2} - R_{3} )\left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}3}} }}\cos \omega_{0} t_{2} + \frac{n}{{\omega_{{{\text{d}}3}} }}\sin \omega_{0} t_{2} } \right) \\ & \quad - e^{{nt_{2} }} (T_{2} - T_{3} )\left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}3}} }}\sin \omega_{0} t_{2} - \frac{n}{{\omega_{{{\text{d}}3}} }}\cos \omega_{0} t_{2} } \right) \\ & \quad + \frac{{ne^{{nt_{2} }} }}{{\omega_{{{\text{d}}3}} }}\left( {\frac{{2k_{2} \theta_{\text{OA}} (t_{1} ) - 2M_{\text{u}} + M_{\text{s}} }}{{\omega_{2}^{2} I}} + \frac{{2M_{\text{BC}} - M_{\text{s}} }}{{\omega_{3}^{2} I}}} \right) \\ \end{aligned} $$
  1. 4.

    Path CD′

$$ \theta_{{{\text{CD}}^{\prime } }} = e^{ - nt} (F_{1} \cos \omega_{{{\text{d}}4}} t + F_{2} \sin \omega_{{{\text{d}}4}} t) + R_{4} \sin \omega_{0} t + T_{4} \cos \omega_{0} t + \frac{{2k_{4} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{4}^{2} I}} $$
$$ R_{4} = \frac{{M_{0} }}{I} \cdot \frac{{\omega_{4}^{2} - \omega_{0}^{2} }}{{(\omega_{4}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} $$
$$ T_{4} = - \frac{{M_{0} }}{I} \cdot \frac{{2n\omega_{0} }}{{(\omega_{4}^{2} - \omega_{0}^{2} )^{2} + 4n^{2} \omega_{0}^{2} }} \, $$
$$ F_{1} = R^{\prime }_{4} \cos \omega_{{{\text{d}}4}} t_{3} - T^{\prime }_{4} \sin \omega_{{{\text{d}}4}} t_{3} $$
$$ F_{2} = R^{\prime }_{4} \sin \omega_{{{\text{d}}4}} t_{3} + T^{\prime }_{4} \cos \omega_{{{\text{d}}4}} t_{3} $$
$$ \omega_{{{\text{d}}4}}^{2} = \omega_{4}^{2} - n^{2} ,\quad \omega_{4}^{2} = \frac{{2k_{4} + \rho gV \cdot GM}}{I},\quad k_{4} = k_{1} $$
$$ \begin{aligned} R^{\prime }_{4} & = (E_{1} \cos \omega_{{{\text{d}}3}} t_{3} + E_{2} \sin \omega_{{{\text{d}}3}} t_{3} ) + e^{{nt_{3} }} (R_{3} - R_{4} )\sin \omega_{0} t_{3} \\ & \quad + e^{{nt_{3} }} (T_{3} - T_{4} )\cos \omega_{0} t_{3} - e^{{nt_{3} }} \left[ {\frac{{2M_{\text{BC}} - M_{\text{s}} }}{{\omega_{3}^{2} I}} + \frac{{2k_{4} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{4}^{2} I}}} \right] \\ \end{aligned} $$
$$ \begin{aligned} T^{\prime }_{4} & = \left( { - E_{1} \frac{{\omega_{{{\text{d}}3}} }}{{\omega_{{{\text{d}}4}} }}\sin \omega_{{{\text{d}}3}} t_{3} + E_{2} \frac{{\omega_{{{\text{d}}3}} }}{{\omega_{{{\text{d}}4}} }}\cos \omega_{{{\text{d}}3}} t_{3} } \right) \\ & \quad + e^{{nt_{3} }} (R_{3} - R_{4} )\left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}4}} }}\cos \omega_{0} t_{3} + \frac{n}{{\omega_{{{\text{d}}4}} }}\sin \omega_{0} t_{3} } \right) \\ & \quad - e^{{nt_{3} }} (T_{3} - T_{4} )\left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}4}} }}\sin \omega_{0} t_{3} - \frac{n}{{\omega_{{{\text{d}}4}} }}\cos \omega_{0} t_{3} } \right) \\ & \quad - \frac{{ne^{{nt_{3} }} }}{{\omega_{{{\text{d}}4}} }}\left( {\frac{{2M_{\text{BC}} - M_{\text{s}} }}{{\omega_{3}^{2} I}} + \frac{{2k_{4} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{4}^{2} I}}} \right) \\ \end{aligned} $$
  1. 5.

    Path D′D

$$ \theta_{{{\text{D}}^{\prime } {\text{D}}}} = e^{ - nt} (G_{1} \cos \omega_{{{\text{d}}5}} t + G_{2} \sin \omega_{{{\text{d}}5}} t) + \frac{{2k_{5} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{5}^{2} I}} $$
$$ G_{1} = R^{\prime }_{5} \cos \omega_{{{\text{d}}5}} t_{4} - T^{\prime }_{5} \sin \omega_{{{\text{d}}5}} t_{4} \, $$
$$ G_{2} = R^{\prime }_{5} \sin \omega_{{{\text{d}}5}} t_{4} + T^{\prime }_{5} \cos \omega_{{{\text{d}}5}} t_{4} $$
$$ \omega_{{{\text{d}}5}}^{2} = \omega_{5}^{2} - n^{2} ,\quad \omega_{5}^{2} = \frac{{2k_{5} + \rho gV \cdot GM}}{I},\quad k_{5} = k_{4} = k_{1} $$
$$ \begin{aligned} R^{\prime }_{5} & = (F_{1} \cos \omega_{{{\text{d}}4}} t_{4} + F_{2} \sin \omega_{{{\text{d}}4}} t_{4} ) + e^{{nt_{4} }} R_{4} \sin \omega_{0} t_{4} \\ & \quad + e^{{nt_{4} }} T_{4} \cos \omega_{0} t_{4} + e^{{nt_{4} }} \left[ {\frac{{2k_{4} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{4}^{2} I}} - \frac{{2k_{5} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{5}^{2} I}}} \right] \\ \end{aligned} $$
$$ \begin{aligned} T_{5}^{\prime } & = \left( { - F_{1} \frac{{\omega_{{{\text{d}}4}} }}{{\omega_{{{\text{d}}5}} }}\sin \omega_{{{\text{d}}4}} t_{4} + F_{2} \frac{{\omega_{{{\text{d}}4}} }}{{\omega_{{{\text{d}}5}} }}\cos \omega_{{{\text{d}}4}} t_{4} } \right) \\ & \quad + e^{{nt_{4} }} R_{4} \left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}5}} }}\cos \omega_{0} t_{4} + \frac{n}{{\omega_{{{\text{d}}5}} }}\sin \omega_{0} t_{4} } \right) \\ & \quad - e^{{nt_{4} }} T_{4} \left( {\frac{{\omega_{0} }}{{\omega_{{{\text{d}}5}} }}\sin \omega_{0} t_{4} - \frac{n}{{\omega_{{{\text{d}}5}} }}\cos \omega_{0} t_{4} } \right) \\ & \quad + \frac{{ne^{{nt_{4} }} }}{{\omega_{{{\text{d}}5}} }}\left( {\frac{{2k_{4} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{4}^{2} I}} - \frac{{2k_{5} \theta_{\text{BC}} (t_{3} ) - 2M_{\text{BC}} + M_{\text{s}} }}{{\omega_{5}^{2} I}}} \right) \\ \end{aligned} $$

About this article

Cite this article

Xu, W., Iijima, K. & Fujikubo, M. Parametric dependencies of the post-ultimate strength behavior of a ship’s hull girder in waves. J Mar Sci Technol 17, 203–215 (2012). https://doi.org/10.1007/s00773-012-0158-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00773-012-0158-1

Keywords

Search

Navigation