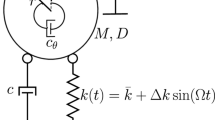Skip to main content
SpringerLink
Log in

Composite Poincaré mapping of double grazing in non-smooth dynamical systems: bifurcations and insights

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

This article investigates the phenomenon of bifurcation induced by double grazing in a piecewise-smooth oscillator with a play, which is a common occurrence in many non-smooth dynamical systems namely gear systems with approval, flange contact in wheel-rail systems, and spacecraft docking. The study is carried out using a composite Poincaré mapping with bilateral constraint, which extends the local normal form mapping of one discontinuity boundary to two discontinuity boundaries. The paper first derives the local discontinuous mapping at the unilateral grazing point using the normal form of discontinuity mapping and determines the type of 3/2 singularity of the zero-time discontinuous mapping with a continuous vector field at the discontinuity boundary. The composite Poincaré mapping is then used to obtain non-classical local bifurcations caused by double grazing, including bifurcation from period-1 motion to period-2 motion, bifurcation from period-1 motion to period-3 motion, and bifurcation from period-1 motion to chaos. The results of the study are consistent with those obtained via direct numerical simulations, demonstrating the efficacy of the composite Poincaré mapping of double grazing. The paper sheds light on the behavior of non-smooth dynamical systems and provides insights into the mechanisms underlying bifurcation induced by double grazing. The findings have potential applications in various fields including engineering, physics, and biology.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.

References

  1. Shaw, S.W., Holmes, P.: Periodically forced linear oscillator with impacts: chaos and long-period motions. Phys. Rev. Lett. 51(8), 623–626 (1983)

    Article  MathSciNet  Google Scholar 

  2. Shaw, S.W., Holmes, P.: A periodically forced piecewise linear oscillator. J. Sound Vib. 90(1), 129–155 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  3. Luo, A.C.J., Chen, L.: Periodic motions and grazing in a harmonically forced, piecewise linear oscillator with impacts. Chaos, Solitons Fractals 24(2), 567–578 (2005)

    Article  MATH  Google Scholar 

  4. di Bernardo, M., Feigin, M.I., Hogan, S.J., Homer, M.E.: Local analysis of C-bifurcations in n-dimensional piecewise smooth dynamical systems. Chaos Solitons Fractals 10(11), 1881–1908 (1999)

    MathSciNet  MATH  Google Scholar 

  5. Nordmark, A.B.: Non-periodic motion caused by grazing incidence in an impact oscillator. J. Sound Vib. 145(2), 279–297 (1991)

    Article  Google Scholar 

  6. di Bernardo, M., Budd, C.J., Champneys, A.R.: Normal form maps for grazing bifurcations in n-dimensional piecewise-smooth dynamical systems. Phys. D 160(3–4), 222–254 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  7. di Bernardo, M., Kowalczyk, P., Nordmark, A.B.: Bifurcations of dynamical systems with sliding: derivation of normal-form mappings. Phys. D 170(3–4), 175–205 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  8. Xu, H.D., Xie, J.H.: Bifurcation andchaos control of a single degree offreedom system with piecewise-linearity. J. Vib. Shock. 27(6), 20–24 (2008)

    Google Scholar 

  9. Xu, H.D., Xie, J.H.: Bifurcation and chaos of a two-degree-of-freedomnon-smooth system with piecewise-linearity. J. Vib. Eng. 21(3), 279–285 (2008)

    Google Scholar 

  10. Hu, H.Y.: Nonsmooth analysis of dynamics of a piecewise linear system. Acta Mech. 28(4), 483–488 (1996)

    MathSciNet  Google Scholar 

  11. Hu, H.Y.: Detection of grazing orbits and incident bifurcations of a forced continuous, piecewise-linear oscillator. J. Sound Vib. 187(3), 485–493 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  12. Granados, A., Granados, H.: Gluing and grazing bifurcations in periodically forced 2-dimensional integrate-and-fire models. Commun. Nonlinear Sci. Numer. Simul. 70, 48–73 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  13. Zhang, W., Li, Q., Meng, Z.: Complex bifurcation analysis of an impacting vibration system based on path-following method. Int. J. Non-Linear Mech. 133, 103715 (2021)

    Article  Google Scholar 

  14. James, I., Ekaterina, P., Marian, W.: Dynamics of a nearly symmetrical piecewise linear oscillator close to grazing incidence: Modelling and experimental verification. Nonlinear Dyn. 46(3), 225–238 (2006)

    Article  MATH  Google Scholar 

  15. Zhang, Z., Chávez, J.P., Sieber, J., Liu, Y.: Controlling grazing-induced multistability in a piecewise-smooth impacting system via the time-delayed feedback control. Nonlinear Dyn. 107, 1595–1610 (2021)

    Article  Google Scholar 

  16. Zhang, Z., Liu, Y., Sieber, J.: Calculating the Lyapunov exponents of a piecewise smooth soft impacting system with a time-delayed feedback controller. Commun. Nonlinear Sci. Numer. Simul. 91, 105451 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  17. Misra, S., Dankowicz, H.: Control of near-grazing dynamics and discontinuity-induced bifurcations in piecewise-smooth dynamical systems. Int. J. Robust Nonlinear Control 20(16), 1836–1851 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  18. Wang, Z.J., Pi, D.H.: Regularization of planar piecewise smooth systems with a heteroclinic loop. Int. J. Bifurc. Chaos 31(15), 2150228 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  19. Guo, B.Y., Chávez, J.P., Liu, Y., Liu, C.S.: Discontinuity-induced bifurcations in a piecewise-smooth capsule system with bidirectional drifts. Commun. Nonlinear Sci. Numer. Simul. 102, 105909 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  20. Zhang, H., Ding, W.C., Li, J.F.: Structure change mechanism of the attractor basin in a piecewise-smooth vibro-impact system. J. Vib. Shock 38(18), 141–147 (2019)

    Google Scholar 

  21. Chen, J.B., Han, M.A.: Bifurcation of limit cycles by perturbing a piecewise linear Hamiltonian system. Qual. Theory Dyn. Syst. 21(2), 34 (2022)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors gratefully acknowledge the founding form the National Natural Science Foundation of China (NNSFC) (Nos. 12072291, and 11732014).

Author information

Authors and Affiliations

  1. School of Mechanics and Aerospace Engineering, Southwest Jiaotong University, Chengdu, 610031, China

    Run Liu & Yuan Yue

Authors
  1. Run Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yuan Yue
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors agreed on the content of the study. RL and YY collected all the data for analysis. YY agreed on the methodology. RL and YY completed the analysis based on agreed steps. Results and conclusions are discussed and written together. All authors read and approved the final manuscript.

Corresponding author

Correspondence to Yuan Yue.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Consent to participate

Not applicable.

Consent for publication

Not applicable.

Human and animal rights

This article does not contain any studies with human or animal subjects performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix 1: Expressions for ZDM

Appendix 1: Expressions for ZDM

$$ \gamma_{1} = \sqrt { - \frac{{x_{0H} }}{{a_{1H} }}} = \sqrt { - 2\frac{{\langle \nabla H,x_{0} \rangle }}{{\langle \nabla H,\left( {\partial F_{1} /\partial x} \right)F_{1} \rangle }}} $$
$$ \gamma_{2} = \frac{{\left( {b_{1} \,x_{0} } \right)_{H} + c_{1H} \gamma_{1}^{2} }}{{2a_{1H} }} $$
$$ \gamma_{3} = - \frac{1}{2}\frac{{a_{1H} \gamma_{2}^{2} + \left( {e_{1} x_{0} } \right)_{H} \gamma_{1}^{2} - \left( {b_{1} x_{0} } \right)_{H} \gamma_{2} - 3c_{1H} \gamma_{1}^{2} \gamma_{2} + f_{1H} \gamma_{1}^{4} }}{{a_{1H} \gamma_{1} }} $$
$$ v_{1} = \frac{{\left( {b_{2} \,\chi_{1} } \right)_{H} }}{{a_{2H} }} = \frac{{\left\langle {\nabla H,b_{2} F_{1} } \right\rangle \gamma_{1} }}{{\left\langle {\nabla H,a_{2} } \right\rangle }} $$
$$ v_{2} = - \frac{{v_{1} \left[ {\,\left( {d_{2} \chi_{1}^{2} } \right)_{H} + c_{2H} \,v_{1}^{2} + \left( {b_{2} \,\chi_{2} } \right)_{H} + \left( {e_{2} \,\chi_{1} } \right)_{H} v_{1} \,} \right]}}{{2a_{2H} v_{1} + \left( {b_{2} \chi_{1} } \right)_{H} }} $$
$$ \begin{aligned} v_{3} & = - \frac{{\left( {j_{2} \chi_{1} } \right)_{H} v_{1}^{3} + \left( {b_{2} \chi_{3} } \right)_{H} v_{1} + a_{2H} v_{2}^{2} + \left( {2d_{2} \chi_{1} \chi_{2} } \right)_{H} v_{1} + \left( {b_{2} \chi_{2} } \right)_{H} v_{2} + f_{2H} v_{1}^{4} }}{{2a_{2H} v_{1} + \left( {b_{2} \chi_{1} } \right)_{H} }} \\ & \quad + \frac{{2\left( {e_{2} \chi_{1} } \right)_{H} v_{1} v_{2} + 3c_{2H} v_{1}^{2} v_{2} + \left( {e_{2} \chi_{2} } \right)_{H} v_{1}^{2} + \left( {h_{2} \chi_{1} } \right)_{H} v_{1}^{2} + \left( {d_{2} \chi_{1}^{2} } \right)_{H} v_{2} + \left( {g_{2} \chi_{1}^{3} } \right)_{H} v_{1} }}{{2a_{2H} v_{1} + \left( {b_{2} \chi_{1} } \right)_{H} }} \\ \end{aligned} $$
$$ \eta_{1} = 8c_{2} - c_{1} + \left( {e_{1} - 4e_{2} } \right)F + \left( {2d_{2} - d_{1} } \right)F^{2} $$
$$ \eta_{2} = \left( {2b_{2} - b_{1} } \right)\chi_{2} + 2\left( {a_{2} - a_{1} } \right)\,v_{2} $$
$$ \eta_{3} = \chi_{3} + F\gamma_{3} $$
$$ L_{1} = L_{2} = \left[ {\begin{array}{*{20}c} 1 &\enspace 0&\enspace 0 \\ {Z_{1} } &\enspace {Z_{2} }&\enspace {Z_{3} } \\ {Z_{4} } &\enspace {Z_{5} }&\enspace {Z_{6} } \\ \end{array} } \right] $$
$$ Z_{1} = z_{1} /2\sqrt {k_{1} - } \xi^{2} \left( {k_{1}^{2} - 2k_{1} \omega^{2} + 4\xi^{2} \omega^{2} + \omega^{4} } \right) $$
$$ \begin{aligned} {\rm Z}_{1} & = a\omega e^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} \left( { - i\xi \omega^{2} e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) - \omega^{2} \sqrt {k_{1} - \xi^{2} } e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right)} \right. \\ \,\,\,\,\,\,\, & + 2\omega^{2} \sqrt {k_{1} - \xi^{2} } e^{{\left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} {\text{sin}}\left( {\omega \left( {\left( {T/2} \right) + t_{0} } \right)} \right) + i\omega \,{\text{cos}}\left( {\omega \,{\text{t}}_{{0}} } \right) \left( \omega^{2} \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right) \right. \\ & \left. + 2\xi^{2} \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right) + 2i\xi \sqrt {k_{1} - \xi^{2} } \left( {1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right) - k_{1} e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} + k_{1} \right) \\ \,\,\,\,\,\,\, & - ik_{1} \xi e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + k_{1} \sqrt {k_{1} - \xi^{2} } e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) \\ \,\,\,\,\,\,\, & - 2k_{1} \sqrt {k_{1} - \xi^{2} } e^{{\left( {T/2\sqrt {k_{1} - \xi^{2} } } \right)}} {\text{sin}}\left( {\omega \left( {\left( {T/2} \right) + t_{0} } \right)} \right) \\ \,\,\,\,\,\,\,\, & 4e\omega \sqrt {k_{1} - \xi^{2} } e^{{\left( {T/2\sqrt {k_{1} - \xi^{2} } } \right)}} {\text{cos}}\left( {\omega \left( {\left( {T/2} \right) + t_{0} } \right)} \right) - \omega^{2} \sqrt {k_{1} - \xi^{2} } {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + k_{1} \sqrt {k_{1} - \xi^{2} } \,{\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) \\ \,\,\,\,\,\,\, & + ik_{1} \xi \,{\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + i\xi \omega^{{2}} \,{\text{sin}}\left. {\left( {\omega \,{\text{t}}_{{0}} } \right)} \right) \\ \end{aligned} $$
$$ Z_{2} = \frac{{e^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k - \xi^{2} } } \right)}} \left( {\sqrt {k_{1} - \xi^{2} } \left( {1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right) - i\xi \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right)} \right)}}{{2\sqrt {k_{1} - \xi^{2} } }} $$
$$ Z_{3} = - \frac{{ie^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right)}}{{2\sqrt {k_{1} - \xi^{2} } }} $$
$$ Z_{4} = z_{4} /2\sqrt {k_{1} - \xi^{2} } \left( {k_{1}^{2} - 2k_{1} \omega^{2} + 4\xi^{2} \omega^{2} + \omega^{4} } \right) $$
$$ \begin{aligned} {\rm Z}_{4} & = a\omega e^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} \\ & \left( { - ik_{1}^{2} \,\xi e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + ik_{1}^{2} {\text{ sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + ik_{1} \,\omega^{2} e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right.{\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) \\ \,\,\,\,\,\,\, &- 2i\xi^{2} \omega^{2} e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) - 2\xi \omega^{2} \sqrt {k_{1} - \xi^{2} } e^{{2i\,\left( {T/2} \right)}} \sqrt {k_{1} - \xi^{2} } {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) \\ \,\,\,\,\,\,\, &+ 4\xi \omega^{2} \sqrt {k_{1} - \xi^{2} } e^{{\left( {T/2} \right)}} \left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right){\text{sin}}\left( {\omega \left( {\left( {T/2} \right) + t_{0} } \right)} \right) + \omega \,{\text{cos}}\left( {\omega \,{\text{t}}_{{0}} } \right)\omega^{2} \left( {\sqrt {k_{1} - \xi^{2} } } \right.\left( { - 1 + e^{{2i\left( {T/2} \right)}} \sqrt {k_{1} - \xi^{2} } } \right) \\ \,\,\,\,\,\,\, & + i\xi \left( { - 1 + \left. {e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right)} \right) + ik_{1} \xi \left( { - 1 + e^{{^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} }} } \right) - k_{1} \sqrt {k_{1} - \xi^{2} } \left( {\left. { - 1 + e^{{^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} }} } \right)} \right) \\ \,\,\,\,\,\,\,\, & + 2\omega \sqrt {k_{1} - \xi^{2} } \left( {k_{1} - \omega^{2} } \right)e^{{\left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} {\text{cos}}\left( {\omega \left( {\delta \,{\text{t}} + t_{0} } \right)} \right) \\ \,\,\,\,\,\,\, & + 2\xi \omega^{2} \sqrt {k_{1} - \xi^{2} } {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) - ik_{1} \omega^{2} {\text{sin}}\left( {\omega \,{\text{t}}_{{0}} } \right) + 2i\xi^{2} \omega^{2} {\text{sin}}\left. {\left( {\omega \,{\text{t}}_{{0}} } \right)} \right) \\ \end{aligned} $$
$$ Z_{5} = - \frac{{ik_{1} e^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k_{1} - \xi^{2} } } \right)}} \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right)}}{{2\sqrt {k_{1} - \xi^{2} } }} $$
$$ Z_{6} = \frac{{e^{{ - \left( {T/2} \right)\left( {\xi + i\sqrt {k - \xi^{2} } } \right)}} \left( {\sqrt {k_{1} - \xi^{2} } \left( {1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right) - i\xi \left( { - 1 + e^{{2i\left( {T/2} \right)\sqrt {k_{1} - \xi^{2} } }} } \right)} \right)}}{{2\sqrt {k_{1} - \xi^{2} } }} $$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, R., Yue, Y. Composite Poincaré mapping of double grazing in non-smooth dynamical systems: bifurcations and insights. Acta Mech 234, 4573–4587 (2023). https://doi.org/10.1007/s00707-023-03602-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-023-03602-6

Search

Navigation