Skip to main content
SpringerLink
Log in

A multiresolution adaptive grid method for solving the generalized probability density equation in stochastic dynamic analysis

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

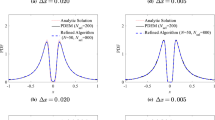
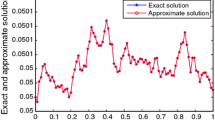
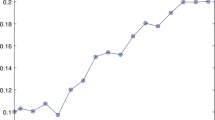
The development of the generalized probability density evolution equation (GDEE) provides a new direction for analyzing stochastic dynamical systems. In particular, it offers an effective solution for nonlinear problems. In this paper, a multiresolution adaptive grid method (MAGM) is proposed for the numerical solution of the GDEE. In this method, the size of multiresolution adaptive grids, which are constructed based on the second-generation wavelet transform, can be dynamically adjusted according to the change of the probability density function (PDF). Besides, the MAGM, which combines the spatial discretization scheme WENO-Z and the temporal discretization scheme third-order TVD Runge–Kutta, ensures the accuracy of the PDF. The method's advantages and accuracy are verified by analyzing a single-degree-of-freedom system, a two-dimensional frame structure, and a three-dimensional frame structure. The adaptive grids can capture the local singularity of PDF and provide more details for local mutation areas. Moreover, the algorithm configures fewer grid points in the region with high regularity to speed up the calculation efficiency.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26

Similar content being viewed by others

References

  1. Ju, P., Li, H.Y., Pan, X.P., Gan, C., Liu, Y., Liu, Y.L.: Stochastic dynamic analysis for power systems under uncertain variability. IEEE Trans. Power Syst. 33, 3789–3799 (2018)

    Article  Google Scholar 

  2. Chandra, S., Sepahvand, K., Matsagar, V.A., Marburg, S.: Stochastic dynamic analysis of composite plate with random temperature increment. Compos. Struct. 226, 111159 (2019)

    Article  Google Scholar 

  3. Ni, P.H., Xia, Y., Li, J., Hao, H., Bi, K.M., Zuo, H.R.: Multi-scale stochastic dynamic response analysis of offshore risers with lognormal uncertainties. Ocean Eng. 189, 14 (2019)

    Article  Google Scholar 

  4. Shinozuka, M.: Monte Carlo solution of structural dynamics. Comput. Struct. 2, 855–874 (1972)

    Article  Google Scholar 

  5. Wang, X.-Y., Cen, S., Li, C.F., Owen, D.R.J.: A priori error estimation for the stochastic perturbation method. Comput. Method. Appl. M 286, 1–21 (2015)

    Article  MathSciNet  Google Scholar 

  6. Lin, J.H., Zhang, W.S., Williams, F.W.: Pseudo-excitation algorithm for nonstationary random seismic responses. Eng. Struct. 16, 270–276 (1994)

    Article  Google Scholar 

  7. Vadlamani, S., Arun, C.O.: A stochastic B-spline wavelet on the interval finite element method for problems in elasto-statics. Probab. Eng. Mech. 58, 102996 (2019)

    Article  Google Scholar 

  8. Vadlamani, S., Arun, C.O.: A stochastic B-spline wavelet on the interval finite element method for beams. Comput. Struct. 233, 106246 (2020)

    Article  Google Scholar 

  9. Vadlamani, S., Arun, C.O.: A perturbation-based stochastic nonlinear beam element formulation using the B-spline wavelet on the interval finite element method. Acta Mech. 232, 4987–5001 (2021)

    Article  MathSciNet  Google Scholar 

  10. Falsone, G., Laudani, R.: A probability transformation method (PTM) for the dynamic stochastic response of structures with non-Gaussian excitations. Eng. Comput. Swansea Wales 35, 1978–1997 (2018)

    Article  Google Scholar 

  11. Falsone, G., Laudani, R.: Matching the principal deformation mode method with the probability transformation method for the analysis of uncertain systems. Int. J. Numer. Methods Eng. 118, 395–410 (2019)

    Article  MathSciNet  Google Scholar 

  12. Laudani R, Falsone, G.: Use of the probability transformation method in some random mechanic problems. ASCE-ASME J. Risk Uncertain. Eng. Syst. Part A Civ. Eng., 7, 04020054 (2021)

  13. Tootkaboni, M., Graham-Brady, L.: Stochastic direct integration schemes for dynamic systems subjected to random excitations. Probab. Eng. Mech. 25, 163–171 (2010)

    Article  Google Scholar 

  14. Li, J., Chen, J.B.: Probability density evolution method for dynamic response analysis of structures with uncertain parameters. Comput. Mech. 34, 400–409 (2004)

    Article  Google Scholar 

  15. Xiao, X., Yan, Y., Chen, B.: Stochastic dynamic analysis for vehicle-track-bridge system based on probability density evolution method. Eng. Struct. 188, 745–761 (2019)

    Article  Google Scholar 

  16. Zheng, Y.: Predicting stochastic characteristics of generalized eigenvalues via a novel sensitivity-based probability density evolution method. Appl. Math. Model. 88, 437–460 (2020)

    Article  MathSciNet  Google Scholar 

  17. Yu, Z.W., Mao, J.F., Guo, F.Q., Guo, W.: Non-stationary random vibration analysis of a 3D train-bridge system using the probability density evolution method. J. Sound Vib. 366, 173–189 (2016)

    Article  Google Scholar 

  18. Li, J., Chen, J.B.: The number theoretical method in response analysis of nonlinear stochastic structures. Comput. Mech. 39, 693–708 (2007)

    Article  MathSciNet  Google Scholar 

  19. Chen, J.B., Yang, J.Y., Li, J.: A GF-discrepancy for point selection in stochastic seismic response analysis of structures with uncertain parameters. Struct. Saf. 59, 20–31 (2016)

    Article  Google Scholar 

  20. Papadopoulos, V., Kalogeris, I.: A Galerkin-based formulation of the probability density evolution method for general stochastic finite element systems. Comput. Mech. 57, 701–716 (2016)

    Article  MathSciNet  Google Scholar 

  21. Tao, W.F., Li, J.: An ensemble evolution numerical method for solving generalized density evolution equation. Probab. Eng. Mech. 48, 1–11 (2017)

    Article  Google Scholar 

  22. To, C.W.S.: The stochastic central difference method in structural dynamics. Comput. Struct. 23, 813–818 (1986)

    Article  Google Scholar 

  23. Li, J., Chen, J.B.: Dynamic response and reliability analysis of structures with uncertain parameters. Int. J. Numer. Methods Eng. 62, 289–315 (2005)

    Article  Google Scholar 

  24. Lin, Y.M., Xu, C.J.: Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 225, 1533–1552 (2007)

    Article  MathSciNet  Google Scholar 

  25. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for two-sided space-fractional partial differential equations. Appl. Numer. Math. 56, 80–90 (2006)

    Article  MathSciNet  Google Scholar 

  26. Yuste, S.B., Acedo, L.: An explicit finite difference method and a new von Neumann-type stability analysis for fractional diffusion equations. SIAM J. Numer. Anal. 42, 1862–1874 (2005)

    Article  MathSciNet  Google Scholar 

  27. Castro, M., Gallardo, J.E.M., Pares, C.: High order finite volume schemes based on reconstruction of states for solving hyperbolic systems with nonconservative products. Applications to shallow-water systems. Math. Comput. 75, 1103–1134 (2006)

    Article  MathSciNet  Google Scholar 

  28. Dumbser, M., Enaux, C., Toro, E.F.: Finite volume schemes of very high order of accuracy for stiff hyperbolic balance laws. J. Comput. Phys. 227, 3971–4001 (2008)

    Article  MathSciNet  Google Scholar 

  29. Sweldens, W.: The lifting scheme: a construction of second generation wavelets. SIAM J. Math. Anal. 29, 511–546 (1998)

    Article  MathSciNet  Google Scholar 

  30. Song, X.D., Zhou, C.K., Hepburn, D.M., Zhang, G.B., Michel, M.: Second generation wavelet transform for data denoising in PD measurement. IEEE Trans. Dielectr. Electr. Insul. 14, 1531–1537 (2007)

    Article  Google Scholar 

  31. Vasilyev, O.V., Bowman, C.: Second-generation wavelet collocation method for the solution of partial differential equations. J. Comput. Phys. 165, 660–693 (2000)

    Article  MathSciNet  Google Scholar 

  32. Borges, R., Carmona, M., Costa, B., Don, W.S.: An improved weighted essentially non-oscillatory scheme for hyperbolic conservation laws. J. Comput. Phys. 227, 3191–3211 (2008)

    Article  MathSciNet  Google Scholar 

  33. Balsara, D.S., Shu, C.W.: Monotonicity preserving weighted essentially non-oscillatory schemes with increasingly high order of accuracy. J. Comput. Phys. 160, 405–452 (2000)

    Article  MathSciNet  Google Scholar 

  34. Shu, J.C.W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 26, 202–228 (1996)

    MathSciNet  MATH  Google Scholar 

  35. Gottlieb, S., Shu, C.W.: Total variation diminishing Runge–Kutta schemes. Math. Comput. 67, 73–85 (1998)

    Article  MathSciNet  Google Scholar 

  36. Takeda, T., Sozen Mete, A., Nielsen, N.N.: Reinforced concrete response to simulated earthquakes. J. Struct. Div. 96, 2557–2573 (1970)

    Article  Google Scholar 

  37. Kent, D.C., Park, R.: Flexural members with confined concrete. J. Struct. Div. 97, 1969–1990 (1971)

    Article  Google Scholar 

  38. GB50010-2010.: Code for design of concrete structures. Beijing: National Standards of the People’s Republic of China, China Architecture & Building Press (2010)

Download references

Acknowledgements

The research is financially supported by the National Natural Science Foundation of China (Grant No. 51878274).

Author information

Authors and Affiliations

  1. School of Mechanics, Civil Engineering and Architecture, Northwestern Polytechnical University, Xi’an, 710072, China

    Tianlong Wang & Xun’an Zhang

  2. College of Civil Engineering, Huaqiao University, Xiamen, 361021, China

    Shixuan Yang

Authors
  1. Tianlong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xun’an Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Shixuan Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xun’an Zhang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, T., Zhang, X. & Yang, S. A multiresolution adaptive grid method for solving the generalized probability density equation in stochastic dynamic analysis. Acta Mech 233, 1911–1940 (2022). https://doi.org/10.1007/s00707-022-03183-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03183-w

Search

Navigation