Abstract
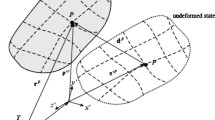
The reduction in the number of coordinates for flexible multibody systems is necessary in order to achieve acceptable simulation times of real-life structures and machines. The conventional model order reduction technique for flexible multibody systems is based on the floating frame of reference formulation (FFRF), using a rigid body frame and superimposed small flexible deformations. The FFRF leads to strongly coupled terms in rigid body and flexible coordinates as well as to a non-constant mass matrix. As an alternative to the FFRF, a formulation based on absolute coordinates has been proposed which uses a co-rotational strain. In this way, a constant mass matrix and a co-rotational stiffness matrix are obtained. In order to perform a reduction in the number of coordinates, by means of the component mode synthesis, e.g., the number of modes needs to be increased, such that all modes are represented in every possible rotated configuration. This approach leads to the method of generalized component mode synthesis (GCMS). The present paper shows in detail how the equations of motion of the FFRF evolve from the ones of the GCMS by considering rigid body constraint conditions and subsequently eliminating them via an appropriate null-space projection. This approach allows a straightforward, term-by-term interpretation of the FFRF mass matrix and of the generalized gyroscopic forces, which, to the same extent, cannot be deduced from former publications on the FFRF. From a practical point of view, the resulting expressions allow to calculate all inertia coefficients from the constant finite element mass matrix together with standard input data of the finite element model in the course of a preprocessing step. Then, the repeated updates of the FFRF mass matrix and of the gyroscopic forces in the course of time integration involve only simple vector matrix operations of low dimensions. In contrast to previous implementations of the FFRF, no evaluations of extra inertia integrals are required. Consequently, the present formulation can be implemented entirely independent of the related finite element code.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Belytschko, T., Hsieh, B.J.: Nonlinear transient finite element analysis with convected coordinates. Int. J. Numer. Methods Eng. 7, 255–271 (1973)
Betsch, P.: The discrete null space method for the energy consistent integration of constrained mechanical systems. Part I: holonomic constraints. Comput. Methods Appl. Mech. Eng. 194(50–52), 5159–5190 (2005)
Betsch, P., Leyendecker, S.: The discrete null space method for the energy consistent integration of constrained mechanical systems. Part II: multibody dynamics. Int. J. Numer. Methods Eng. 67(4), 499–552 (2006)
Brüls, O., Duysinx, P., Golinval, J.C.: The global modal parameterization for non-linear model-order reduction in flexible multibody dynamics. Int. J. Numer. Methods Eng. 69, 948–977 (2007)
César de Sá, J.M.A., Natal Jorge, R.M., Fontes Valente, R.A., Areias, P.M.A.: Development of shear locking-free shell elements using an enhanced assumed strain formulation. Int. J. Numer. Methods Eng. 53, 1721–1750 (2002)
Frischkorn, J., Reese, S.: A solid-beam finite element and non-linear constitutive modelling. Comput. Methods Appl. Mech. Eng. 265, 195–212 (2013)
García de Jalón, J., Bayo, E.: Kinematic and Dynamic Simulation of Multibody Systems: The Real-Time Challenge. Springer, New York (1994)
Gerstmayr, J.: Strain tensors in the absolute nodal coordinate and the floating frame of reference formulation. Nonlinear Dyn. 34, 133–145 (2003)
Gerstmayr, J., Ambrósio, J.A.C.: Component mode synthesis with constant mass and stiffness matrices applied to flexible multibody systems. Int. J. Numer. Methods Eng. 73(11), 1518–1546 (2008)
Gerstmayr, J., Schöberl, J.: A 3D finite element method for flexible multibody systems. Multibody Syst. Dyn. 15(4), 305–320 (2006)
Humer, A., Gerstmayr, J.: Energy-momentum conserving time integration of modally reduced flexible multibody systems. In: Proceedings of the ASME 2013 IDETC/CIE Conference. Portland, USA (2013)
Lehner, M.: Modellreduktion in elastischen Mehrkörpersystemen. Ph.D. thesis, Universität Stuttgart (2007)
Liang, C.G., Lance, G.M.: A differentiable null space method for constrained dynamic analysis. J. Mech. Transm. Autom. Des. 109, 405–411 (1987)
Naets, F., Tamarozzi, T., Heirman, G.H.K., Desmet, W.: Real-time flexible multibody simulation with global modal parameterization. Multibody Syst. Dyn. 27, 267–284 (2012)
Orzechowski, G., Matikainen, M.K., Mikkola, A.M.: Inertia forces and shape integrals in the floating frame of reference formulation. Nonlinear Dyn. 88, 19531968 (2017)
Pechstein, A., Reischl, D., Gerstmayr, J.: A generalized component mode synthesis approach for flexible multibody systems with a constant mass matrix. J. Comput. Nonlinear Dyn. 8(1), 11,019 (2013)
Rao, S.S.: The Finite Element Method in Engineering, 6th edn. Elsevier, Amsterdam (2018)
Schwertassek, R., Wallrapp, O.: Dynamik Flexibler Mehrkrpersysteme. Springer, Wiesbaden (1999)
Shabana, A.A.: Dynamics of Multibody Systems, 3rd edn. Cambridge University Press, New York (2013)
Shabana, A.A., Schwertassek, R.: Equivalance of the floating frame of reference approach and finite element formulations. Int. J. Non Linear Mech. 33, 417–432 (1998)
Sherif, K., Nachbagauer, K.: A detailed derivation of the velocity-dependent inertia forces in the floating frame of reference formulation. J. Comput. Nonlinear Dyn. 9(044), 501 (2014)
Simmonds, J.G.: A Brief on Tensor Analysis, 2nd edn. Springer, New York (1994)
Vetyukov, Y.: Finite element modeling of Kirchhoff–Love shells as smooth material surfaces. ZAMM Zeitschrift für angewandte Mathematik und Mechanik 94, 150–163 (2014)
Wempner, G.A.: Finite elements, finite rotations and small strains. Int. J. Solids Struct. 5, 117–153 (1969)
Yakoub, Y., Shabana, A.A.: Three dimensional absolute nodal coordinate formulation for beam elements. ASME J. Mech. Des. 123, 606–621 (2001)
Acknowledgements
Open access funding provided by University of Innsbruck and Medical University of Innsbruck.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Winkler, R., Gerstmayr, J. A projection-based approach for the derivation of the floating frame of reference formulation for multibody systems. Acta Mech 230, 1–29 (2019). https://doi.org/10.1007/s00707-018-2306-0
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00707-018-2306-0