Skip to main content
SpringerLink
Log in

Convective heat transfer and fluid flow of two counter-rotating cylinders in tandem arrangement

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

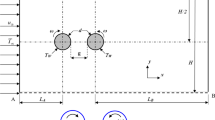
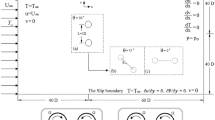
This paper discusses the forced convective heat transfer and fluid flow around two counter-rotating cylinders in tandem arrangement at a constant Reynolds number of 200. The upstream and downstream cylinders rotate in counterclockwise and clockwise directions, respectively, with an identical non-dimensional rotating speed (RS) in the range of \(0\le \hbox {RS}\le 4\). Computations are carried out at various non-dimensional gap spaces between the cylinders such as the \({G{/}D}=1.5\), 2.0, and 3.0. It is found that counter-rotating the tandem cylinders deforms the wake region downstream of both cylinders in which the vortex strength of the upstream cylinder is realized to be stronger at larger gap spaces. On the other hand, it is stated that the instabilities of the shear layer of both cylinders become maximum and minimum at \(\hbox {RS}=1\) and \(\hbox {RS}=2\), respectively. Examination of the Nusselt number distributions on the cylinders indicates that at the high RS values, more or less, all points on the each individual cylinder have identical roles in the heat dissipation rate. Finally, it is concluded that the maximum heat transfer occurs at \(\hbox {RS}=1\) for both cylinders.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

A :

Projected area

\(C_\mathrm{D} \) :

Drag coefficient (\({=}\,\frac{F_\mathrm{D}}{0.5\rho U^{2}A})\)

\(\bar{{C}}_\mathrm{D} \) :

Mean drag coefficient

\(C_\mathrm{L}\) :

Lift coefficient (\({=}\,\frac{F_\mathrm{L}}{0.5\rho U^{2}A})\)

\(\bar{{C}}_\mathrm{L}\) :

Mean lift coefficient

\(C_\mathrm{p} \) :

Pressure coefficient (\({=}\,\frac{p-p_\infty }{0.5\rho U^{2}})\)

\(c_\mathrm{p} \) :

Specific pressure

D :

Cylinder diameter

\(F_\mathrm{D}\) :

Drag force

\(F_\mathrm{L}\) :

Lift force

G :

Gap space between the cylinders

k :

Conductivity

n :

Surface vertical vector

Nu :

Nusselt number

\(\overline{Nu} \) :

Mean Nusselt number

\(p_\infty \) :

Free-stream pressure

p :

Pressure

Pr :

Prandtl number (\({=}\,\frac{\mu c_\mathrm{p}}{k})\)

r :

Radial coordinate

R :

Cylinder radius

Re :

Reynolds number (\({=}\,\frac{\rho UD}{\mu })\)

RS:

Non-dimensional rotational speed \(({=}\,\frac{\omega D}{2U})\)

t :

Time

T :

Temperature

\(T_\infty \) :

Free-stream temperature

u :

Streamwise velocity

\(\bar{{u}}\) :

Time-averaged streamwise velocity

U :

Free-stream velocity

\(u_\mathrm{{rms}} \) :

Root-mean-square of the streamwise velocity

v :

Vertical velocity

\(\bar{{v}}\) :

Time-averaged vertical velocity

\(v_\mathrm{{rms}} \) :

Root-mean-square of the vertical velocity

x :

Streamwise dimension of coordinates

y :

Vertical dimension of coordinates

\(\mu \) :

Dynamic viscosity of the fluid

\(\upsilon \) :

Kinematic viscosity of the fluid

\(\rho \) :

Density of the fluid

\(\alpha \) :

Angular location

\(\omega \) :

Rotating speed

\(\xi \) :

Element size

1:

Upstream cylinder

2:

Downstream cylinder

\(\max \) :

Maximum

\(\min \) :

Minimum

s:

Surface of the cylinder

\(\infty \) :

Free-stream

References

  1. Rajani, B.N., Kandasamy, A., Majumdar, S.: Numerical simulation of laminar flow past a circular cylinder. Appl. Math. Model. 33, 1228–1247 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  2. Ramos-García, N., Sarlak, H., Andersen, S.J., Sørensen, J.N.: Simulation of the flow past a circular cylinder using an unsteady panel method. Appl. Math. Model. 44, 206–222 (2017)

    Article  MathSciNet  Google Scholar 

  3. Abrahamsen Prsic, M., Ong, M.C., Pettersen, B., Myrhaug, D.: Large Eddy Simulations of flow around a smooth circular cylinder in a uniform current in the subcritical flow regime. Ocean Eng. 77, 61–73 (2014)

    Article  Google Scholar 

  4. Durante, D., Rossi, E., Colagrossi, A., Graziani, G.: Numerical simulations of the transition from laminar to chaotic behaviour of the planar vortex flow past a circular cylinder. Commun. Nonlinear Sci. Numer. Simul. 48, 18–38 (2017)

    Article  MathSciNet  Google Scholar 

  5. Stringer, R.M., Zang, J., Hillis, A.J.: Unsteady RANS computations of flow around a circular cylinder for a wide range of Reynolds numbers. Ocean Eng. 87, 1–9 (2014)

    Article  Google Scholar 

  6. Rajagopalan, S., Antonia, R.A.: Flow around a circular cylinder–structure of the near wake shear layer. Exp. Fluids 38, 393–402 (2005)

    Article  Google Scholar 

  7. Yeon, S.M., Yang, J., Stern, F.: Large-eddy simulation of the flow past a circular cylinder at sub- to super-critical Reynolds numbers. Appl. Ocean Res. 59, 663–675 (2016)

    Article  Google Scholar 

  8. Aljure, D.E., Lehmkhul, O., Rodríguez, I., Oliva, A.: Three dimensionality in the wake of the flow around a circular cylinder at Reynolds number 5000. Comput. Fluids. 147, 102–118 (2017)

    Article  MathSciNet  Google Scholar 

  9. Perret, L.: PIV investigation of the shear layer vortices in the near wake of a circular cylinder. Exp. Fluids 47, 789–800 (2009)

    Article  Google Scholar 

  10. Kang, S.: Uniform-shear flow over a circular cylinder at low Reynolds numbers. J. Fluids Struct. 22, 541–555 (2006)

    Article  Google Scholar 

  11. Alam, MdM, Zheng, Q., Hourigan, K.: The wake and thrust by four side-by-side cylinders at a low Re. J. Fluids Struct. 70, 131–144 (2017)

    Article  Google Scholar 

  12. Chen, W., Ji, C., Wang, R., Xu, D., Campbell, J.: Flow-induced vibrations of two side-by-side circular cylinders: asymmetric vibration, symmetry hysteresis and near-wake patterns. Ocean Eng. 110, 244–257 (2015)

    Article  Google Scholar 

  13. Elhimer, M., Harran, G., Hoarau, Y., Cazin, S., Marchal, M., Braza, M.: Coherent and turbulent processes in the bistable regime around a tandem of cylinders including reattached flow dynamics by means of high-speed PIV. J. Fluids Struct. 60, 62–79 (2016)

    Article  Google Scholar 

  14. Gopalan, H., Jaiman, R.: Numerical study of the flow interference between tandem cylinders employing non-linear hybrid URANS-LES methods. J. Wind Eng. Ind. Aerodyn. 142, 111–129 (2015)

    Article  Google Scholar 

  15. Kim, S., Alam, MdM: Characteristics and suppression of flow-induced vibrations of two side-by-side circular cylinders. J. Fluids Struct. 54, 629–642 (2015)

    Article  Google Scholar 

  16. Liu, J., Xie, G., Sundén, B.: Flow pattern and heat transfer past two tandem arranged cylinders with oscillating inlet velocity. Appl. Therm. Eng. 120, 614–625 (2017)

    Article  Google Scholar 

  17. Pang, J.H., Zong, Z., Zou, L., Wang, Z.: Numerical simulation of the flow around two side-by-side circular cylinders by IVCBC vortex method. Ocean Eng. 119, 86–100 (2016)

    Article  Google Scholar 

  18. Thapa, J., Zhao, M., Cheng, L., Zhou, T.: Three-dimensional simulations of flow past two circular cylinders in side-by-side arrangements at right and oblique attacks. J. Fluids Struct. 55, 64–83 (2015)

    Article  Google Scholar 

  19. Tu, J., Zhou, D., Bao, Y., Ma, J., Lu, J., Han, Z.: Flow-induced vibrations of two circular cylinders in tandem with shear flow at low Reynolds number. J. Fluids Struct. 59, 224–251 (2015)

    Article  Google Scholar 

  20. Vakil, A., Green, S.I.: Two-dimensional side-by-side circular cylinders at moderate Reynolds numbers. Comput. Fluids. 51, 136–144 (2011)

    Article  MATH  Google Scholar 

  21. Karabelas, S.J.: Large Eddy simulation of high-Reynolds number flow past a rotating cylinder. Int. J. Heat Fluid Flow 31, 518–527 (2010)

    Article  Google Scholar 

  22. Lam, K.M.: Vortex shedding flow behind a slowly rotating circular cylinder. J. Fluids Struct. 25, 245–262 (2009)

    Article  Google Scholar 

  23. Thakur, P., Mittal, S., Tiwari, N., Chhabra, R.P.: The motion of a rotating circular cylinder in a stream of Bingham plastic fluid. J. Nonnewton. Fluid Mech. 235, 29–46 (2016)

    Article  MathSciNet  Google Scholar 

  24. Ghazanfarian, J., Nobari, M.R.H.: A numerical study of convective heat transfer from a rotating cylinder with cross-flow oscillation. Int. J. Heat Mass Transf. 52, 5402–5411 (2009)

    Article  MATH  Google Scholar 

  25. Ikhtiar, U., Manzoor, S., Sheikh, N.A., Ali, M.: Free stream flow and forced convection heat transfer around a rotating circular cylinder subjected to a single gust impulse. Int. J. Heat Mass Transf. 99, 851–861 (2016)

    Article  Google Scholar 

  26. Mittal, S., Kumar, B.: Flow past a rotating cylinder. J. Fluid Mech. 476, 303–334 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  27. Stojković, D., Breuer, M., Durst, F.: Effect of high rotation rates on the laminar flow around a circular cylinder. Phys. Fluids 14(9), 3160–3178 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  28. Patankar, S.V.: Numerical Heat Transfer and Fluid Flow. Taylor & Francis, New York (1980)

    MATH  Google Scholar 

  29. Meneghini, J.R., Siqueira, Cd.L.R., Saltara, F., Ferrari, Jr. J.A.: An investigation of the flow around two circular cylinders in tandem arrangements, \(15{{\rm th}}\) Brazilian congress of mechanical engineering, Sao Paulo, November 22–26

  30. Slaoutian, A., Stansby, P.K.: Flow around two circular cylinders by the random-vortex method. J. Fluids Struct. 6, 641–670 (1992)

    Article  Google Scholar 

  31. Mahir, N., Altaç, Z.: Numerical investigation of convective heat transfer in unsteady flow past two cylinders in tandem arrangements. Int. J. Heat Fluid Flow 29, 1309–1318 (2008)

    Article  Google Scholar 

  32. R. Hilpert, Forsch. Geb. Ingenieurwes 4, 215 (1933)

  33. Churchill, S.W., Bernstein, M.: A correlation equation for forced convection from gases and liquids to a circular cylinder in cross Flow. ASME J. Heat Transf. Trans. 94, 300–306 (1977)

    Article  Google Scholar 

  34. Harimi, I., Saghafian, M.: Numerical simulation of fluid flow and forced convection heat transfer from tandem circular cylinders using overset grid method. J. Fluids Struct. 28, 309–327 (2012)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Urmia University of Technology, Urmia, Iran

    Mohsen Darvishyadegari & Rahim Hassanzadeh

Authors
  1. Mohsen Darvishyadegari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Rahim Hassanzadeh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rahim Hassanzadeh.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Darvishyadegari, M., Hassanzadeh, R. Convective heat transfer and fluid flow of two counter-rotating cylinders in tandem arrangement. Acta Mech 229, 1783–1802 (2018). https://doi.org/10.1007/s00707-017-2070-6

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-017-2070-6

Search

Navigation