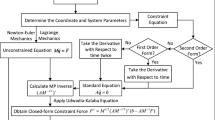
Abstract
This paper proposes that Kane’s equations for a simple nonholonomic system are the first-order form of generalized speeds. When the first-order form of Kane’s equations is put in matrix form, the element of the mass matrix is identical to the inertia coefficient. Because the orthogonal set of partial velocities will decouple the first-order equations, one can use the orthogonal criterion to generate efficient equations of motion. With the presented first-order form, Kane’s equations are different from Maggi’s or Gibbs–Appell’s equations. Moreover, in order to clarify the relationship between Kane’s approach and classical approaches, we start from Kane’s equations and introduce kinetic energy or acceleration energy functions to derive Lagrange’s or Gibbs–Appell’s forms of Kane’s equations for the system. We found that the Lagrange’s forms of Kane’s equations can be used to solve nonholonomic systems without introducing Lagrangian multipliers. At last, the first-order form, Lagrange’s forms, and Gibbs–Appell’s forms of Kane’s equations are, respectively, used to depict the derivation of first-order equations of motion for a rolling coin.
Similar content being viewed by others
Abbreviations
- \(a_i (i=1, 2, 3)\) :
-
Components of acceleration
- A :
-
Function used in Lagrange’s and Gibbs–Appell’s forms of Kane’s equations
- \(A_{sr},\;B_s \) :
-
Functions of \(q_s (s=1,\ldots ,n)\) and t
- \(c_i \) :
-
Abbreviation of \(\cos q_i \)
- \(C, C^{*}\) :
-
A rigid body and its mass center
- \(F_i \) :
-
Components of force \(\mathbf{F}^{C}\)
- g :
-
Local gravitational acceleration
- G :
-
Gibbs function or acceleration energy function
- \(\tilde{G}\) :
-
Equal to G minus A
- \(I_i \) :
-
Components of principal moment of inertia
- \(K_r, K_r^*, \hat{{K}}_r^*\) :
-
rth generalized active force, rth generalized inertia force, and part of the rth generalized inertia forces for particles system
- \({ }^CK_r, { }^CK_r^*, { }^C\hat{{K}}_r^*\) :
-
rth generalized active force, rth generalized inertia force, and part of the rth generalized inertia forces for rigid body C
- \(m_i \) :
-
Mass of a particle
- \(m_{rs}, { }^C(m_{rs} )\) :
-
Inertia coefficients for a particles system and for rigid body C
- M :
-
Mass of a rigid body
- n :
-
Number of generalized coordinates
- N :
-
Newtonian reference frame
- p :
-
Degree of freedom
- \(P_i \) :
-
A typical particle
- \(P_r \) :
-
Functions used in Lagrange’s form of Kane’s equations
- \(q_i (i=1,\ldots ,n)\) :
-
Generalized coordinates
- R :
-
Radius of a rolling coin
- \(s_i \) :
-
Abbreviation of \(\sin q_i \)
- S :
-
A nonholonomic system
- t :
-
Time
- T :
-
Kinetic energy function
- \(T_i \) :
-
Components of force \(\mathbf{T}^{C}\)
- \(u_r \) :
-
rth generalized speed
- \(v_i (i=1, 2, 3)\) :
-
Velocity components of a rolling coin
- \(\bar{{v}}_i (i=1, 2, 3)\) :
-
Velocity components of mass center of a rolling coin
- \(W_{sr},\;X_s,\;Y_{rs},\;Z_r \) :
-
Functions of \(q_s (s=1,\ldots ,n)\) and t
- \(\mathbf{a}_i \) :
-
Acceleration of \(P_i \)
- \(\mathbf{a}^{C^{*}}\) :
-
Acceleration of mass center of rigid body \(C^{*}\)
- \(\mathbf{b}_i \) :
-
Constitute the base vectors of a reference frame
- \(\mathbf{c}_\mathbf{i} \) :
-
Constitute the base vectors of a rigid body
- \(\mathbf{F}^{C}, \mathbf{F}^{C^{*}}\) :
-
Resultant active force and inertia force in rigid body C
- \(\mathbf{F}_i \) :
-
Resultant of all contact forces and/or distance forces
- \(\mathbf{I}\) :
-
Central inertia dyadic of a rigid body
- \(\mathbf{n}_i \;(i=x,y,z)\) :
-
The Newtonian unit vector used in the rolling coin
- \(\mathbf{r}_i \) :
-
Position vector
- \(\mathbf{T}^{C}, \mathbf{T}^{C^{*}}\) :
-
Resultant active torque and inertia torque whose line of action passes through \(C^{*}\)
- \(\mathbf{v}^{C^{*}}\) :
-
Velocity of mass center of rigid body C
- \(\mathbf{v}_i \) :
-
Velocity of particle \(P_i \)
- \(\tilde{\mathbf{v}}_r^{C^{*}}, \tilde{\mathbf{v}}_t^{C^{*}} \) :
-
Vector functions of \(q_i (i=1,\ldots ,n)\) and t for a rigid body C
- \(\tilde{\mathbf{v}}_r^{P_i }, \tilde{\mathbf{v}}_t^{P_i } \) :
-
Vector functions of \(q_i (i=1,\ldots ,n)\) and t for a particle \(P_i \)
- \(\varvec{\upalpha }^{C}\) :
-
Angular acceleration of a rigid body C
- \(\alpha _i (i=1, 2, 3)\) :
-
Components of angular acceleration
- \(\nu \) :
-
number of particles
- \(\varvec{\upomega }^{C}\) :
-
Angular velocity of rigid body C
- \(\omega _i (i=1, 2, 3)\) :
-
Components of angular velocity
- \(\tilde{\varvec{\upomega }}_r^C, \tilde{\varvec{\upomega }}_t^C \) :
-
Angular velocities of \(q_i (i=1,\ldots ,n)\) and t for rigid body C
- \(i, r,\;s,\;t\) :
-
Indices
References
Kane, T.R.: Analytical Elements of Mechanics, vol. 2, 2nd edn. Academic Press, New York, London (1959)
Kane, T.R.: Dynamics of nonholonomic systems. ASME J. Appl. Mech. 28, 574–578 (1961)
Kane, T.R., Wang, C.F.: On the derivation of equations of motion. J. Soc. Ind. Appl. Math. 13, 487–492 (1965)
Kane, T.R.: Dynamics. Holt, Rinehart, and Winston, New York (1968)
Kane, T.R., Levinson, D.A.: Formulation of equations of motion for complex spacecraft. J. Guid. Control 3, 99–112 (1980)
Kane, T.R.: Formulation of dynamical equations of motion. Am. J. Phys. 51, 974–977 (1983)
Kane, T.R., Levinson, D.A.: The multibody dynamics. ASME J. Appl. Mech. 50, 1071–1078 (1983)
Kane, T.R., Levinson, D.A.: The use of Kane’s dynamical equations in robotics. Int. J. Robot. Res. 2, 3–21 (1983)
Kane, T.R., Likins, P.W., Levinson, D.A.: Spacecraft Dynamics. McGraw-Hill Book Co., New York (1983)
Kane, T.R., Levinson, D.A.: Dynamics: Theory and Applications. McGraw-Hill Book Co., New York (1985)
Radetsky, P.: The man who mastered motion. Science 86, 52–60 (1985)
Huston, R.L.: Multibody Dynamics. Butterworth-Heinemann, Inc., Oxford (1990)
Loduha, T.A., Ravani, B.: On first-order decoupling of equations of motion for constrained dynamical systems. ASME J. Appl. Mech. 62, 216–222 (1995)
Chen, B.: Analytical Dynamics. Taiwan Advanced Education Inc., Taipei (1988). (in Chinese)
Desloge, E.A.: A comparison of Kane’s equation of motion and the Gibbs–Appell equations of motion. Am. J. Phys. 54, 470–472 (1986)
Desloge, E.A.: Relationship between Kane’s equations and the Gibbs–Appell equations. J. Guid. Control Dyn. 10, 120–122 (1987)
Borri, M., Bottasso, C., Mantegazza, P.: Equivalence of Kane’s and Maggi’s equations. Meccanica 25, 272–274 (1990)
Kane, T.R.: Rebuttal to ‘A comparison of Kane’s equation of motion and the Gibbs–Appell equations of motion’. Am. J. Phys. 54, 472 (1986)
Levinson, D.A.: Rebuttal to ‘Equivalence of Kane’s and Maggi’s equations’. Meccanica 27, 63–64 (1992)
Wang, L.S., Pao, Y.H.: Jourdain’s variational equation and Appell’s equations of motion for nonholonomic dynamical systems. Am. J. Phys. 71, 72–82 (2003)
Ginsberg, J.H.: Advanced Engineering Dynamics. Harper and Row Publishers Inc., New York (1988)
Chen, Y.H.: Equations of motion of mechanical systems under servo constraints: the Maggi approach. Mechatronics 18, 208–217 (2008)
Passerello, C.E., Huston, R.L.: Another look at nonholonomic systems. ASME J. Appl. Mech. 40, 101–104 (1973)
Desloge, E.A.: Efficacy of the Gibbs–Appell method for generating equations of motion for complex systems. J. Guid. 12, 114–116 (1989)
Mitiguy, P.C., Kane, T.R.: Motion variables leading to efficient equations of motion. Int. J. Robot. Res. 15, 522–532 (1996)
Acknowledgments
This research was supported by the National Science Council of Taiwan, the Republic of China, under Grant NSC 87-2212-E-344-001 and is gratefully acknowledged.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Tu, T.W. First-order form, Lagrange’s form, and Gibbs–Appell’s form of Kane’s equations. Acta Mech 227, 1885–1901 (2016). https://doi.org/10.1007/s00707-016-1611-8
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00707-016-1611-8