Skip to main content
SpringerLink
Log in

Eshelby’s tensors for plane strain and cylindrical inclusions based on a simplified strain gradient elasticity theory

  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

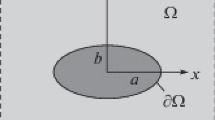
The Eshelby tensor for a plane strain inclusion of arbitrary cross-sectional shape is first presented in a general form, which has 15 independent non-zero components (as opposed to 36 such components for a three-dimensional inclusion of arbitrary shape). It is based on a simplified strain gradient elasticity theory that involves one material length scale parameter. The Eshelby tensor for an infinitely long cylindrical inclusion is then derived using the general form, with its components obtained in explicit (closed-form) expressions for the two regions inside and outside the inclusion for the first time based on a higher-order elasticity theory. This Eshelby tensor is separated into a classical part and a gradient part. The latter depends on the position, the inclusion size, the length scale parameter, and Poisson’s ratio. As a result, the new Eshelby tensor is non-uniform even inside the cylindrical inclusion and captures the size effect. When the strain gradient effect is not considered, the gradient part vanishes and the newly obtained Eshelby tensor reduces to its counterpart based on classical elasticity. The numerical results quantitatively show that the components of the new Eshelby tensor vary with the position, the inclusion size, and the material length scale parameter, unlike their classical elasticity-based counterparts. When the inclusion radius is comparable to the material length scale parameter, it is found that the gradient part is too large to be ignored. In view of the need for homogenization analyses of fiber-reinforced composites, the volume average of the newly derived Eshelby tensor over the cylindrical inclusion is obtained in a closed form. The components of the average Eshelby tensor are observed to depend on the inclusion size: the smaller the inclusion radius, the smaller the components. However, as the inclusion size becomes sufficiently large, these components are seen to approach from below the values of their classical elasticity-based counterparts.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Eshelby J.D.: The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. Lond. A241, 376–396 (1957)

    MathSciNet  Google Scholar 

  2. Eshelby J.D.: The elastic field outside an ellipsoidal inclusion. Proc. R. Soc. Lond. A252, 561–569 (1959)

    MathSciNet  Google Scholar 

  3. Mura T.: Micromechanics of Defects in Solids, 2nd edn. Martinus Nijhoff, Dordrecht (1987)

    Google Scholar 

  4. Li S., Wang G.: Introduction to Micromechanics and Nanomechanics. World Scientific, Singapore (2008)

    MATH  Google Scholar 

  5. Mori T., Tanaka K.: Average stress in matrix and average elastic energy of materials with misfitting inclusions. Acta Metall. 21, 571–574 (1973)

    Article  Google Scholar 

  6. Weng G.J.: The theoretical connection between Mori-Tanaka’s theory and the Hashin-Shtrikman-Walpole bounds. Int. J. Eng. Sci. 28, 1111–1120 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  7. Li S., Wang G., Sauer R.A.: The Eshelby tensors in a finite spherical domain—Part II: applications to homogenization. ASME J. Appl. Mech. 74, 784–797 (2007)

    Article  MathSciNet  Google Scholar 

  8. Hill R.: A self-consistent mechanics of composites materials. J. Mech. Phys. Solids 13, 213–222 (1965)

    Article  Google Scholar 

  9. Budiansky B.: On the elastic moduli of some heterogeneous materials. J. Mech. Phys. Solids 13, 223–227 (1965)

    Article  Google Scholar 

  10. Huang Y., Hu K.X., Wei X., Chandra A.: A generalized self-consistent mechanics method for composite materials with multiphase inclusions. J. Mech. Phys. Solids 42, 491–504 (1994)

    Article  MATH  Google Scholar 

  11. Le Quang H., He Q.-C.: A one-parameter generalized self-consistent model for isotropic multiphase composites. Int. J. Solids Struct. 44, 6805–6825 (2007)

    Article  MATH  Google Scholar 

  12. Marcadon V., Herve E., Zaoui A.: Micromechanical modeling of packing and size effects in particulate composites. Int. J. Solids Struct. 44, 8213–8228 (2007)

    Article  MATH  Google Scholar 

  13. Vollenberg P.H.T., Heikens D.: Particle size dependence of the Young’s modulus of filled polymers: 1. Preliminary experiments. Polymer 30, 1656–1662 (1989)

    Article  Google Scholar 

  14. Vollenberg P.H.T., de Haan J.W., van de Ven L.J.M., Heikens D.: Particle size dependence of the Young’s modulus of filled polymers: 2. Annealing and solid-state nuclear magnetic resonance experiments. Polymer 30, 1663–1668 (1989)

    Article  Google Scholar 

  15. Reynaud E., Jouen T., Gauthier C., Vigier G., Varlet J.: Nanofillers in polymeric matrix: a study on silica reinforced PA6. Polymer 42, 8759–8768 (2001)

    Article  Google Scholar 

  16. Cho J., Joshi M.S., Sun C.T.: Effect of inclusion size on mechanical properties of polymeric composites with micro and nano particles. Compos. Sci. Tech. 66, 1941–1952 (2006)

    Article  Google Scholar 

  17. Nikolov S., Han C.-S., Raabe D.: On the origin of size effects in small-strain elasticity of solid polymers. Int. J. Solids Struct. 44, 1582–1592 (2007)

    Article  MATH  Google Scholar 

  18. Cheng Z.-Q., He L.-H.: Micropolar elastic fields due to a spherical inclusion. Int. J. Eng. Sci. 33, 389–397 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  19. Cheng Z.-Q., He L.-H.: Micropolar elastic fields due to a circular cylindrical inclusion. Int. J. Eng. Sci. 35, 659–668 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  20. Ma H.S., Hu G.K.: Eshelby tensors for an ellipsoidal inclusion in a micropolar material. Int. J. Eng. Sci. 44, 595–605 (2006)

    Article  Google Scholar 

  21. Liu X.N., Hu G.K.: Inclusion problem of microstretch continuum. Int. J. Eng. Sci. 42, 849–860 (2004)

    Article  Google Scholar 

  22. Kiris A., Inan E.: Eshelby tensors for a spherical inclusion in microstretch elastic fields. Int. J. Solids Struct. 43, 4720–4738 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  23. Ma H.S., Hu G.K.: Eshelby tensors for an ellipsoidal inclusion in a microstretch material. Int. J. Solids Struct. 44, 3049–3061 (2007)

    Article  MATH  Google Scholar 

  24. Zheng Q.-S., Zhao Z.-H.: Green’s function and Eshelby’s fields in couple-stress elasticity. Int. J. Multiscale Comput. Eng. 2, 15–27 (2004)

    Article  Google Scholar 

  25. Gao, X.-L., Ma, H.M.: Green’s function and Eshelby’s tensor based on a simplified strain gradient elasticity theory. Acta Mech. (in press) (2008). doi:10.1007/s00707-008-0109-4

  26. Lakes R.: Experimental methods for study of Cosserat elastic solids and other generalized elastic continua. In: Muhlhaus, H. (eds) Continuum Models for Materials with Micro-Structure, pp. 1–22. Wiley, New York (1995)

    Google Scholar 

  27. Lam D.C.C., Yang F., Chong A.C.M., Wang J., Tong P.: Experiments and theory in strain gradient elasticity. J. Mech. Phys. Solids 51, 1477–1508 (2003)

    Article  MATH  Google Scholar 

  28. Luo H.A., Weng G.J.: On Eshelby’s S-tensor in a three-phase cylindrically concentric solid, and the elastic moduli of fiber-reinforced composites. Mech. Mater. 8, 77–88 (1989)

    Article  Google Scholar 

  29. Gao X.-L., Park S.K.: Variational formulation of a simplified strain gradient elasticity theory and its application to a pressurized thick-walled cylinder problem. Int. J. Solids Struct. 44, 7486–7499 (2007)

    Article  MATH  Google Scholar 

  30. Ashby M.F.: The deformation of plastically non-homogeneous materials. Phil. Mag. 21, 399–424 (1970)

    Article  Google Scholar 

  31. Fleck N.A., Muller G.M., Ashby M.F., Hutchinson J.W.: Strain gradient plasticity: theory and experiment. Acta Metall. Mater. 42, 475–487 (1994)

    Article  Google Scholar 

  32. Arfken G.B., Weber H.-J.: Mathematical Methods for Physicists, 6th edn. Elsevier, San Diego (2005)

    MATH  Google Scholar 

  33. Xun F., Hu G.K., Huang Z.P.: Effective in plane moduli of composites with a micropolar matrix and coated fibers. Int. J. Solids Struct. 41, 247–265 (2004)

    Article  MATH  Google Scholar 

  34. Gradshteyn I.S., Ryzhik I.M.: In: Jeffrey, A., Zwillinger, D. (eds.) Table of Integrals, Series, and Products, 7th edn. Academic Press, Boston (2007)

  35. Magnus W., Oberhettinger F., Soni R.P.: Formulas and Theorems for the Special Functions of Mathematical Physics. Springer-Verlag, New York (1966)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Texas A&M University, 3123 TAMU, College Station, TX, 77843-3123, USA

    H. M. Ma & X. -L. Gao

Authors
  1. H. M. Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. X. -L. Gao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to X. -L. Gao.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ma, H.M., Gao, X.L. Eshelby’s tensors for plane strain and cylindrical inclusions based on a simplified strain gradient elasticity theory. Acta Mech 211, 115–129 (2010). https://doi.org/10.1007/s00707-009-0221-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-009-0221-0

Keywords

Search

Navigation