Skip to main content
SpringerLink
Log in

Contribution to the brachistochrone problem with Coulomb friction

  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

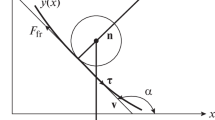
This paper formulates and solves in closed form (expressed by elementary functions) the brachistochrone problem with Coulomb friction of a particle which moves down a rough curve in a uniform gravitational field assuming that the initial velocity of the particle is different from zero. The problem is solved by the application of variational calculus. Two variants are considered: first, the initial position and the final position of the particle are given; second, the initial position is given, and the final position lies on a given vertical straight line. The new approach in treating this problem by variational calculus lies in the fact that the projection sign of the normal reaction force of the rough curve onto the normal to the curve is introduced as the additional constraint in the form of an inequality. This inequality is transformed into an equality by introducing a new state variable. Although this is fundamentally a constrained variational problem, by further introducing a new functional with an expanded set of unknown functions, it is transformed into an unconstrained problem where broken extremals appear. Brachistochrone equations in parametric form are obtained for both variants which are examined, with the slope angle of the tangent to the brachistochrone being taken as the parameter. These equations contain a certain number of unknown constants which are determined from the corresponding systems of nonlinear algebraic equations. They are solved by an alternative approach which is based on the application of differential evolution. The obtained brachistochrones are generally two-segment curves with the initial line segment representing a free-fall parabola in nonresistant medium. It is shown that regarding the special values of the parameters the results of the paper coincide with the known results from literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ashby N., Brittin W.E., Love W.F., Wyss W.: Brachistochrone with Coulomb friction. Am. J. Phys. 43(10), 902–906 (1975)

    Article  MathSciNet  Google Scholar 

  2. Chapra S.C., Canale R.P.: Numerical Methods for Engineers: with Software and Programming Applications, 4th edn. McGraw Hill, New York (2002)

    Google Scholar 

  3. Charlton W., Chiarella C., Roberts A.W.: Gravity flow of granular materials in chutes: optimizing flow properties. J. Agr. Eng. Res. 20, 39–45 (1975)

    Article  Google Scholar 

  4. Čović V., Vesković M.: Extension of the Bernoulli’s case of brachistochronic motion to the multibody system having the form of a kinematic chain with external constraints. Eur. J. Mech. A/Solids 21, 347–354 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  5. Čović V., Lukačević M.: Extension of the Bernoulli’s case of a brachistochronic motion to the multibody system in the form of a closed kinematic chain. Facta Univ. Ser. Mech. Autom. Control Robot. 2(9), 973–982 (1999)

    MATH  Google Scholar 

  6. Čović V., Vesković M.: Brachistochrone on a surface with Coulomb friction. Int. J. Nonlinear Mech. 43(5), 437–450 (2008)

    Article  Google Scholar 

  7. Djukić Dj.: On the brachistochronic motion of a dynamic system. Acta Mech. 32, 181–186 (1979)

    Article  MATH  MathSciNet  Google Scholar 

  8. Elsgolc L.E.: Calculus of Variations. Pergamon Press, Oxford (1963)

    Google Scholar 

  9. Gelfand I.M., Fomin S.V.: Calculus of Variations. Prentice Hall, Englewood Cliffs (1964)

    Google Scholar 

  10. Gregory J., Lin C.: An unconstrained calculus of variations formulation for generalized optimal control problems and for the constrained problem of Bolza. J. Math. Anal. Appl. 187, 826–841 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  11. Hayen J.C.: Brachistochrone with Coulomb friction. Int. J. Nonlinear Mech. 40, 1057–1075 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  12. Hirsch M.J., Meneses C.N., Pardalos P.M., Resende M.G.C.: Global optimization by continuous grasp. Opt. Lett. 1(2), 201–212 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  13. Karr C.L., Weck B., Freeman l.M.: Solutions to systems of nonlinear equations via a genetic algorithm. Eng. Appl. Artif. Intell. 11, 369–375 (1998)

    Article  Google Scholar 

  14. Lipp S.C.: Brachistochrone with Coulomb friction. SIAM J. Control Optim. 35(2), 562–584 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  15. Maisser P.: Brachystochronen als zeitkürzeste Fahrspuren von Bobschlitten. ZAMM-Z. Angew. Math. Mech. 78(5), 311–319 (1998) (in German)

    Article  MATH  MathSciNet  Google Scholar 

  16. Martinez J.M.: Algorithms for solving nonlinear systems of equations. In: Spedicato, E. (eds) Continuous Optimization: The State of the Art, pp. 81–108. Kluwer, Dordrecht (1994)

    Google Scholar 

  17. Papastavridis J.G.: On a Lagrangean action based kinetic instability theorem of Kelvin and Tait. Int. J. Eng. Sci. 24(1), 1–17 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  18. Price K.V., Storn R.M., Lampinen J.A.: Differential Evolution: a Practical Approach to Global Optimization, 2nd edn. Springer, Berlin (2005)

    MATH  Google Scholar 

  19. Stork D.G., Yang J.: The general unrestrained brachistochrone. Am. J. Phys. 56(1), 22–26 (1988)

    Article  MathSciNet  Google Scholar 

  20. Zeković D.: The brachistochrone motion of mechanical system with nonholonomic, nonlinear and nonstationary constraints. PMM-J. Appl. Math. Mech. 54(6), 765–768 (1990)

    Article  Google Scholar 

  21. Zeković D., Čović V.: On the brachistochronic motion of mechanical systems with linear nonholonomic nonhomogeneous constraints. Mech. Res. Commun. 20(1), 25–35 (1993)

    Article  MATH  Google Scholar 

  22. Valentine, F.A.: The problem of Lagrange with differential inequalities as added side conditions. Contributions to the calculus of variations, 1933–1937, pp. 407–448. University of Chicago Press, Chicago (1937)

  23. Vander Heijden A.M.A., Diepstraten J.D.: On the brachystochrone with dry friction. Int. J. Nonlinear Mech. 10, 97–112 (1975)

    Article  Google Scholar 

  24. Vratanar B., Saje M.: On the analytical solution of the brachistochrone problem in a nonconservative field. Int. J. Nonlinear Mech. 33(3), 489–505 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  25. Wensrich C.M.: Evolutionary solutions to the brachistochrone problem with Coulomb friction. Mech. Res. Commun. 31, 151–159 (2004)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Mechanical Engineering, University of Kragujevac, Dositejeva 19, 36000, Kraljevo, Serbia

    Slaviša Šalinić

Authors
  1. Slaviša Šalinić
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Slaviša Šalinić.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Šalinić, S. Contribution to the brachistochrone problem with Coulomb friction. Acta Mech 208, 97–115 (2009). https://doi.org/10.1007/s00707-008-0134-3

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-008-0134-3

Keywords

Search

Navigation