Skip to main content
SpringerLink
Log in

On a class of sofic affine invariant subsets of the 2-torus related to an Erdős problem

  • Published:
Monatshefte für Mathematik Aims and scope Submit manuscript

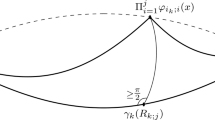
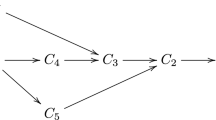
Abstract

Let 1 < β < 2 be a real number and G be the closed projection on the 2-torus of the (modified) Rademacher graph in base β. The smallest compact containing G and left invariant by the diagonal endomorphism \({(x,y)\mapsto(2x,\beta y)}\) (mod 1) is denoted by K. For β a simple Parry number of PV-type, K is proved to be a sofic affine invariant set with a fractal geometry closed to the one of G. When β is the golden number, we prove the uniqueness of the measure with full Hausdorff dimension on K.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alexander J.C., Zagier D.: The entropy of a certain infinitely convolved Bernoulli measure. J. Lond. Math. Soc. 44, 121–134 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  2. Bertrand A.: Développement en base de Pisot et répartition modulo 1. C. R. Acad. Sc. Paris 285, 419–421 (1977)

    MathSciNet  MATH  Google Scholar 

  3. Bertrand Mathis A.: Développement en base θ; répartition modulo 1 de la suite \({(x\theta^n)_{n\ge0}}\) ; langage codé et θ-shift. Bull. Soc. Math. France 114(3), 271–323 (1986)

    MathSciNet  MATH  Google Scholar 

  4. Blanchard F.: β-expansions and symbolic dynamics. Theoret. Comput. Sci. 65(2), 131–141 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bowen R.: Some systems with unique equilibrium states. Math. Syst. Theory 8, 193–202 (1974)

    Article  MathSciNet  Google Scholar 

  6. Bowen, R.: Equilibrium states and the ergodic theory of Anosov diffeomorphisms. In: Lecture Notes in Mathematics, vol. 470. Springer, Berlin (1975)

  7. Boyle M., Kitchens C., Marcus B.: A note on minimal covers for sofic systems. Proc. Am. Math. Soc. 95, 403–411 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  8. Boyle M., Tuncel S.: Infinite-to-one code and Markov measures. Trans. Am. Math. Soc. 285, 657–683 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  9. Buzzi J., Sarig O.: Uniqueness of equilibrium measures for countable Markov shifts and multi-dimensional piecewise expanding maps. Ergod. Theory Dyn. Syst. 23, 1383–1400 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  10. Cigler, J.: Ziffernverteilung in ϑ-adischen Brűchen. Math. Z. 75, 8–13 (1960/1961)

    Google Scholar 

  11. Denker, M., Grillenberger, C., Sigmund, K.: Ergodic theory on compact spaces. In: Lecture Notes in Mathematics, vol. 527. Springer, Berlin (1976)

  12. Dumont J.M., Sidorov N., Thomas A.: Number of representations related to a linear recurrent basis. Acta Arith. 88, 371–394 (1999)

    MathSciNet  MATH  Google Scholar 

  13. Erdős P.: On the smoothness properties of a family of symmetric Bernoulli convolutions. Am. J. Math. 62, 180–186 (1940)

    Article  Google Scholar 

  14. Erdős P.: On a family of symmetric Bernoulli convolutions. Am. J. Math. 61, 974–976 (1939)

    Article  Google Scholar 

  15. Feng D.-J.: The limited Rademacher functions and Bernoulli convolutions associated with Pisot numbers. Adv. Math. 195, 24–101 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  16. Feng D.-J.: Equilibrium states for factor maps between subshifts. Adv. Math. 226, 2470–2502 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  17. Feng D.-J., Olivier E.: Multifractal analysis of weak Gibbs measures and phase transition—application to some Bernoulli convolutions. Ergod. Theory Dyn. Syst. 23, 1751–1784 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  18. Garsia A.M.: Entropy and singularity of infinite convolutions. Pac. J. Math. 13, 1159–1169 (1963)

    MathSciNet  MATH  Google Scholar 

  19. Gatzouras, D., Peres, Y.: The variational principle for Hausdorff dimension: a survey. Erg. Th. of Z d actions (Warwick, 1993–1994). London Math. Soc. Lecture Note Ser., vol. 228, pp. 113–125. Cambridge University Press, Cambridge (1996)

  20. Gatzouras D., Peres Y.: Invariant measures of full dimension for some expanding maps. Ergod. Theory Dyn. Syst. 17, 147–167 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  21. Gurevic B.-M.: Topological entropy of a countable Markov chain. Dokl. Akad. Nauk. SSSR 187, 715–718 (1969)

    MathSciNet  Google Scholar 

  22. Gurevic B.-M.: Shift entropy and Markov measures in the space of paths on a countable graph. Dokl. Akad. Nauk. SSSR 192, 963–965 (1970)

    MathSciNet  Google Scholar 

  23. Haydn N.T.A., Ruelle D.: Equivalence of Gibbs and equilibrium states for homeomorphisms satisfying expansiveness and specification. Commun. Math. Phys. 148(1), 155–167 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  24. Ito S., Takahashi Y.: Markov subshifts and realization of β-expansions. J. Math. Soc. Jpn. 26, 33–55 (1974)

    Article  MathSciNet  MATH  Google Scholar 

  25. Jessen B., Wintner A.: Distribution functions and the Riemann zeta function. Trans. AMS 38, 48–88 (1935)

    Article  MathSciNet  Google Scholar 

  26. Kenyon R., Peres Y.: Measures of full dimension on affine-invariant sets. Ergod. Theory Dyn. Syst. 16, 307–323 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  27. Kenyon R., Peres Y.: Hausdorff dimensions of sofic affine-invariant sets. Isr. J. Math. 94, 157–178 (1996)

    Article  MathSciNet  Google Scholar 

  28. Ledrappier F.: Principe variationnel et systèmes dynamiques symboliques. Z. Wahr. Verw. Geb. 30, 185–202 (1974)

    Article  MathSciNet  MATH  Google Scholar 

  29. Ledrappier F., Walters P.: A relativised variational principle for continuous transformations. J. Lond. Math. Soc. 16, 568–576 (1976)

    Article  MathSciNet  Google Scholar 

  30. Ledrappier F., Young L.S.: The metric entropy of diffeomorphisms. Part 1: characterisation of measures satisfying Pesin’s entropy formula; Part 2: relation between entropy, exponents and dimension. Ann. Math. 122, 540–574 (1985)

    Article  MathSciNet  Google Scholar 

  31. Mauldin R.D., Urbański M.: Gibbs states on the symbolic space over an infinite alphabet. Isr. J. Math 125, 93–130 (2001)

    Article  MATH  Google Scholar 

  32. Olivier E., Sidorov N., Thomas A.: On the Gibbs properties of Bernoulli convolutions related to β-numeration in multinacci bases. Monatsh. Math. 145, 145–174 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  33. Olivier, E., Thomas, A.: Infinite convolution of Bernoulli measures. PV numbers and related problems in the dynamics of fractal geometry. Compte Rendus de la SMF (2008, to appear)

  34. Olivier E.: Uniqueness of the measure with full dimension on sofic affine invariant subsets of the 2-torus. Ergod. Theory Dyn. Syst. 30, 1503–1528 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  35. Olivier, E.: Measures with full dimension on self-affine graphs. In: Barral, J., Seuret, S. (eds.) Recent Developments in Fractals and Related Fields, pp. 295–308. Birkhäuser, Boston (2010)

  36. Parry W., Pollicott M.: Zeta functions and the periodic structure of hyperbolic dynamics. Astérisque 187–188, 1–268 (1990)

    Google Scholar 

  37. Parry W.: On the β-expansions of real numbers. Acta Math. Acad. Sci. Hung. 11, 401–416 (1960)

    Article  MathSciNet  MATH  Google Scholar 

  38. Parry W.: Intrinsic Markov chains. Trans. Am. Math. Soc. 112, 55–66 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  39. Peres, Y., Schlag, W., Solomyak, B.: Sixty years of Bernoulli convolutions, Fractal Geometry and Stochastics II. Progress in Probability, vol. 46, pp. 95–106. Birkhäuser (2000)

  40. Petersen, K.: Information compression and retention in dynamical processes. In: Maass, A., Martí nez, S., San Martí n, J. (eds.) Dynamics and Randomness, pp. 147–218 (2002)

  41. Peyrière, J.: An introduction to fractal measures and dimensions. Lectures at Xiangfan (1995)

  42. Przytycki, F., Urbański, M.: On the Hausdorff dimension of some fractal sets. Studio Math. XCIII, 155–186 (1989)

  43. Rényi A.: Representation of real numbers and their ergodic properties. Acta Math. Acad. Sci. Hungar. 8, 477–493 (1957)

    Article  MathSciNet  MATH  Google Scholar 

  44. Rauzy G.: Nombres algŽbriques et substitutions. Bull. Soc. Math. France 110(2), 147–178 (1982)

    MathSciNet  MATH  Google Scholar 

  45. Ruelle D.: Thermodynamic Formalism. Addison Wesley, Reading (1978)

    MATH  Google Scholar 

  46. Ruelle D.: Thermodynamic formalism of maps satisfying positive expansiveness and specification. Nonlinearity 5, 1223–1236 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  47. Sarig O.: Thermodynamic formalism for countable Markov shifts. Ergod. Theory Dyn. Syst. 19, 1565–1593 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  48. Sarig O.: Thermodynamic formalism for null recurrent potentials. Isr. J. Math. 121, 285–311 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  49. Sarig O.: Existence of Gibbs measures for countable Markov shifts. Proc. AMS 131(6), 1751–1758 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  50. Schmidt K.: On periodic expansions of Pisot numbers and Salem numbers. Bull. Lond. Math. Soc. 12, 269–278 (1980)

    Article  MATH  Google Scholar 

  51. Seneta, E.: Non-negative matrices and Markov chains. In: Springer Series in Statistics, vol. XV. Springer, New York-Heidelberg-Berlin (1981)

  52. Shelton, K.: Ergodic properties of a Class of Erdős measures. Ph.D. Thesis, University of North Carolina (1998)

  53. Sidorov N., Vershik A.: Ergodic properties of the Erdős measure, the entropy of the Goldenshift and related problems. Monatsh. Math. 126, 215–261 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  54. Shin S.: Measures that maximize weighted entropy for factor maps between subshifts of finite type. Ergod. Theory Dyn. Syst. 21, 1249–1272 (2001)

    MATH  Google Scholar 

  55. Solomyak B.: On the random series \({\sum\pm\lambda^n}\) (an Erdős problem). Ann. Math. 142, 611–625 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  56. Thurston, W.P.: Groups, tiling and finite automata. In: Summer 1989 AMS Colloquium lecures (1989)

  57. Walters P.: Invariant measures and equilibrium states for some mappings which expands distances. Trans. Am. Math. Soc. 236, 127–153 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  58. Walters P.: An Introduction to Ergodic Theory. Springer, Berlin-Heidelberg-New York (1982)

    Book  MATH  Google Scholar 

  59. Walters P.: Relative pressure, relative equilibrium states, compensation functions and many-to-one codes between subshifts. Trans. Am. Math. Soc. 296, 1–31 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  60. Weiss B.: Subshift of finite type and sofic systems. Monatsh. Math. 77, 462–474 (1973)

    Article  MathSciNet  MATH  Google Scholar 

  61. Yayama Y.: Dimension of compact invariant sets of some expanding maps. Ergod. Theory Dyn. Syst. 29, 281–315 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  62. Young L.-S.: Dimension, entropy and Lyapunov exponents. Ergod. Theory Dyn. Syst. 2, 109–124 (1982)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Université de Provence, 3 Pl. V. Hugo, 13331, Marseille Cedex 03, France

    Eric Olivier

Authors
  1. Eric Olivier
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Eric Olivier.

Additional information

Communicated by Klaus Schmidt.

To the memory of Gérard Rauzy.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Olivier, E. On a class of sofic affine invariant subsets of the 2-torus related to an Erdős problem. Monatsh Math 165, 447–497 (2012). https://doi.org/10.1007/s00605-011-0296-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00605-011-0296-2

Keywords

Mathematics Subject Classification (2000)

Search

Navigation