Abstract
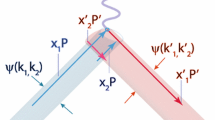
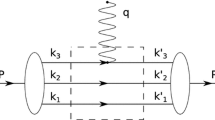
The endpoint model explains the scaling laws observed in exclusive hadronic reactions at large momentum transfer in all experimentally important regimes. The model, originally conceived by Feynman and others, assumes a single valence quark carries most of the hadron momentum. The quark wave function is directly related to the momentum transfer dependence of the reaction. After extracting the momentum dependence of the quark wave function from one process, it explains all the others. Endpoint quark-counting rules relate the number of quarks in a hadron to the power-law. A universal linear endpoint behavior explains the proton electromagnetic form factors \(F_{1}\) and \(F_{2}\), proton–proton scattering at fixed-angle, the t-dependence of proton–proton scattering at large \(s>> t\), and Compton scattering at fixed t. The model appears to be the only comprehensive mechanism consistent with all experimental information.
Similar content being viewed by others
References
S.J. Brodsky, G.R. Farrar, Phys. Rev. D 11, 1309 (1975)
G.R. Farrar, D.R. Jackson, Phys. Rev. Lett. 43, 246 (1979)
G.P. Lepage, S.J. Brodsky, Phys. Rev. D 22, 2157 (1980)
A.V. Efremov, A.V. Radyushkin, Theor. Math. Phys. 42, 97 (1980)
A.V. Efremov, A.V. Radyushkin, Phys. Lett. B94, 245 (1980)
S.J. Brodsky, G.P. Lepage, Phys. Rev. D 24, 2848 (1981)
P.V. Landshoff, Phys. Rev. D 10, 1024 (1974)
P.D.B. Collins, F.D. Gault, A. Martin, Nucl. Phys. B 85, 141 (1975)
A. Donnachie, P.V. Landshoff, Z. Phys. C 2, 55 (1979)
P.D.B. Collins, F.D. Gault, Phys. Lett. B 112, 255 (1982)
A. Danagoulian et al., [Hall A Collaboration], Phys. Rev. Lett. 98, 152001 (2007)
N. Isgur, C. Llewelyn-Smith, Phys. Rev. Lett. 52, 1080 (1984)
P. Jain, B. Pire, J.P. Ralston, Phys. Rep. 271, 67 (1996)
S. Dagaonkar, P. Jain, J.P. Ralston, EPJC 74, 3000 (2014)
S. Dagaonkar, P. Jain, J.P. Ralston, EPJC 76, 368 (2016)
S. Dagaonkar, arXiv:1611.00147
R.P. Feynman, Phys. Rev. Lett. 23, 1415 (1969)
S.D. Drell, T.-M. Yan, Phys. Rev. Lett. 24, 181 (1970)
G.B. West, Phys. Rev. Lett. 24, 1206 (1970)
S.J. Brodsky, C.-R. Ji, M. Sawicki, Phys. Rev. D 32, 1530 (1985)
M. Sawicki, Phys. Rev. D 32, 2666 (1985)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Dagaonkar, S., Jain, P. & Ralston, J.P. Endpoint Model of Exclusive Processes. Few-Body Syst 59, 71 (2018). https://doi.org/10.1007/s00601-018-1395-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00601-018-1395-6