The structure of branched transportation networks

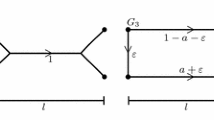
Abstract

The transportation problem can be formalized as the problem of finding the optimal paths to transport a measure μ ⁺ onto a measure μ ⁻ with the same mass. In contrast with the Monge–Kantorovich formalization, recent approaches model the branched structure of such supply networks by an energy functional whose essential feature is to favor wide roads. Given a flow s in a road or a tube or a wire, the transportation cost per unit length is supposed to be proportional to s ^α with 0 < α < 1. For the Monge–Kantorovich energy α = 1 so that it is equivalent to have two roads with flow 1/2 or a larger one with flow 1. If instead 0 < α < 1, a road with flow $s_1+s_2$ is preferable to two individual roads s ₁ and s ₂ because $(s_1+s_2)^\alpha < s_1^\alpha+s_2^\alpha$ . Thus, this very simple model intuitively leads to branched transportation structures. Such a branched structure is observable in ground transportation networks, in draining and irrigation systems, in electric power supply systems and in natural objects like the blood vessels or the trees. When $\alpha > 1-\frac 1N$ such structures can irrigate a whole bounded open set of ${\mathbb{R}}^N$ . The aim of this paper is to give a mathematical proof of several structure and regularity properties empirically observed in transportation networks. It is first proven that optimal transportation networks have a tree structure and can be monotonically approximated by finite graphs. An interior regularity result is then proven according to which an optimal network is a finite graph away from the irrigated measure. It is also proven that the branching number of optimal networks has everywhere a universal explicit bound. These results answer questions raised in two recent papers by Xia.