Skip to main content
SpringerLink
Log in

Realizing an m-Uniform Four-Chromatic Hypergraph with Disks

  • Original paper
  • Published:
Combinatorica Aims and scope Submit manuscript

Abstract

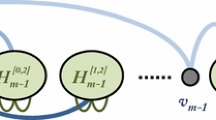
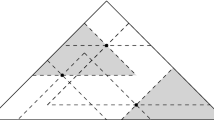
We prove that for every positive integer m there is a finite point set \(\cal{P}\) in the plane such that no matter how \(\cal{P}\) is three-colored, there is always a disk containing exactly m points, all of the same color. This improves a result of Pach, Tardos and Tóth who proved the same for two colors. The main ingredient of the construction is a subconstruction whose points are in convex position. Namely, we show that for every positive integer m there is a finite point set \(\cal{P}\) in the plane in convex position such that no matter how \(\cal{P}\) is two-colored, there is always a disk containing exactly m points, all of the same color. We also prove that for unit disks no similar construction can work, and several other results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. E. Ackerman, B. Keszegh and D. Pálvölgyi: Coloring hypergraphs defined by stabbed pseudo-disks and ABAB-free hypergraphs, SIAM Journal on Discrete Mathematics 34 (2020), 2250–2269.

    Article  MathSciNet  MATH  Google Scholar 

  2. E. Ackerman, B. Keszegh and M. Vizer: Coloring points with respect to squares, Discrete & Computational Geometry 58 (2017), 757–784.

    Article  MathSciNet  MATH  Google Scholar 

  3. N. Alon, G. Ding, B. Oporowski and D. Vertigan: Partitioning into graphs with only small components, J. Combin. Theory Ser. B 87 (2003), 231–243.

    Article  MathSciNet  MATH  Google Scholar 

  4. A. Asinowski, J. Cardinal, N. Cohen, S. Collette, T. Hackl, M. Hoffmann, K. Knauer, S. Langerman, M. Lasoń, P. Micek, G. Rote and T. Ueckerdt: Coloring hypergraphs induced by dynamic point sets and bottomless rectangles, in: Algorithms and data structures, volume 8037 of Lecture Notes in Comput. Sci., pages 73–84. Springer, Heidelberg, 2013.

    Chapter  MATH  Google Scholar 

  5. J. Cardinal, K. Knauer, P. Micek and T. Ueckerdt: Making triangles colorful, J. Comput. Geom. 4 (2013), 240–246.

    MathSciNet  MATH  Google Scholar 

  6. L. Esperet and G. Joret: Colouring planar graphs with three colours and no large monochromatic components, Combin. Probab. Comput. 23 (2014), 551–570.

    Article  MathSciNet  MATH  Google Scholar 

  7. M. Gibson and K. Varadarajan: Optimally decomposing coverings with translates of a convex polygon, Discrete Comput. Geom. 46 (2011), 313–333.

    Article  MathSciNet  MATH  Google Scholar 

  8. F. John: Extremum problems with inequalities as subsidiary conditions, Studies and Essays Presented to R. Courant on his 60th Birthday, January 8, 1948, Interscience Publishers, Inc., New York, N. Y., 1948, 187–204.

    Google Scholar 

  9. B. Keszegh: Coloring half-planes and bottomless rectangles, Computational geometry 45 (2012), 495–507.

    Article  MathSciNet  MATH  Google Scholar 

  10. B. Keszegh and D. Pálvölgyi: Octants are cover decomposable, Discrete Comput. Geom. 47 (2012), 598–609.

    Article  MathSciNet  MATH  Google Scholar 

  11. B. Keszegh and D. Pálvölgyi: Convex polygons are self-coverable, Discrete Comput. Geom. 51 (2014), 885–895.

    Article  MathSciNet  MATH  Google Scholar 

  12. B. Keszegh and D. Pálvölgyi: More on decomposing coverings by octants, J. Comput. Geom. 6 (2019), 300–315.

    MathSciNet  MATH  Google Scholar 

  13. B. Keszegh and D. Pálvölgyi: Proper coloring of geometric hypergraphs, Discrete Comput. Geom. 62 (2019), 674–689.

    Article  MathSciNet  MATH  Google Scholar 

  14. B. Keszegh and D. Pálvölgyi: An abstract approach to polychromatic coloring: shallow hitting sets in ABA-free hypergraphs and pseudohalfplanes, J. Comput. Geom. 10 (2019), 1–26.

    MathSciNet  MATH  Google Scholar 

  15. J. M. Kleinberg, R. Motwani, P. Raghavan and S. Venkatasubramanian: Storage management for evolving databases, in: Proceedings 38th Annual Symposium on Foundations of Computer Science, pages 353–362, 1997.

  16. I. Kovács: Indecomposable coverings with homothetic polygons, Discrete & Computational Geometry 53 (2015), 817–824.

    Article  MathSciNet  MATH  Google Scholar 

  17. N. Linial, J. Matoušek, O. Sheffet and G. Tardos: Graph coloring with no large monochromatic components, Electronic Notes in Discrete Mathematics 29 115–122, 2007. European Conference on Combinatorics, Graph Theory and Applications.

    Article  MATH  Google Scholar 

  18. S. Norin, A. Scott, P. D. Seymour and D. R. Wood: Clustered colouring in minor-closed classes, Combinatorica 39 (2019), 1387–1412.

    Article  MathSciNet  MATH  Google Scholar 

  19. J. Pach: Covering the plane with convex polygons, Discrete Comput. Geom. 1 (1986), 73–81.

    Article  MathSciNet  MATH  Google Scholar 

  20. J. Pach and D. Pálvölgyi: Unsplittable coverings in the plane, Advances in Mathematics 302 (2016), 433–457.

    Article  MathSciNet  MATH  Google Scholar 

  21. J. Pach, D. Pálvölgyi and G. Tóth: Survey on decomposition of multiple coverings, in: Geometry-Intuitive, Discrete, and Convex, 219–257. Springer, 2013.

  22. J. Pach, G. Tardos and G. Tóth: Indecomposable coverings, In Discrete geometry, combinatorics and graph theory, volume 4381 of Lecture Notes in Comput. Sci., pages 135–148. Springer, Berlin, 2007.

  23. D. Pálvölgyi: Indecomposable coverings with concave polygons, Discrete Comput. Geom. 44 (2010), 577–588.

    Article  MathSciNet  MATH  Google Scholar 

  24. D. Pálvölgyi and G. Tóth: Convex polygons are cover-decomposable, Discrete & Computational Geometry 43 (2010), 483–496.

    Article  MathSciNet  MATH  Google Scholar 

  25. S. Smorodinsky and Y. Yuditsky: Polychromatic coloring for half-planes, J. Combin. Theory Ser. A 119 (2012), 146–154.

    Article  MathSciNet  MATH  Google Scholar 

  26. G. Tardos and G. Tóth: Multiple coverings of the plane with triangles, Discrete & Computational Geometry 38 (2007), 443–450.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgment

We would like to thank Balázs Keszegh for useful discussions and for reading a draft of this manuscript, and the anonymous reviewers of the conference version for their suggestions.

Author information

Authors and Affiliations

  1. MTA-ELTE Lendület Combinatorial, Geometry Research Group, ELTE Eötvös Loránd University, Budapest, Hungary

    Gábor Damásdi & Dömötör Pálvölgyi

Authors
  1. Gábor Damásdi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dömötör Pálvölgyi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dömötör Pálvölgyi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Damásdi, G., Pálvölgyi, D. Realizing an m-Uniform Four-Chromatic Hypergraph with Disks. Combinatorica 42 (Suppl 1), 1027–1048 (2022). https://doi.org/10.1007/s00493-021-4846-5

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00493-021-4846-5

Mathematics Subject Classification (2010)

Search

Navigation