Abstract
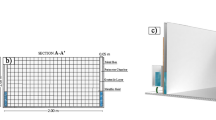
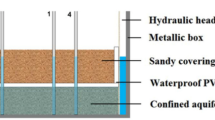
We deal with the spatial distribution of the hydraulic conductivity K within heterogeneous porous formations where a radial flow (typical of pumping and slug tests) is taking place. In particular, the study provides a wide data-set which is instrumental to corroborate theoretical findings about the stochastic behavior of K in the above flow configuration. Here, K-data pertain to a series of slug tests conducted within a large caisson which was densely instrumented in order to capture the transitional behavior of the conductivity from the near field (close to the pumping well) to the far field (away from the pumping well). For the experiments at stake, it is shown that the apparent conductivity \(K_{\mathrm{app}}\) is a very robust property. In fact, with the exception of a very tiny annulus surrounding the pumping well, \(K_{\mathrm{app}}\) can be used to solve flow (and transport) problems in close analogy to the effective theory approach adopted for a groundwater-type flow. It is hoped that the data-set exploited in the present study will be useful for other researchers who are engaged with similar studies.



Similar content being viewed by others
References
Aristodemo F, Ianchello M, Fallico C (2018) Smoothing analysis of slug tests data for aquifer characterization at laboratory scale. J Hydrol 562:125–139. https://doi.org/10.1016/j.jhydrol.2018.04.056
Bird N, Perrier E (2010) Multiscale percolation properties of a fractal pore network. Geoderma 160:105–110. https://doi.org/10.1016/j.geoderma.2009.10.009
Broyda S, Dentz M, Tartakovsky DM (2010) Probability density functions for advective-reactive transport in radial flow. Stoch Environ Res Risk Assess 24:985–992. https://doi.org/10.1007/s00477-010-0401-4
Caia A, Di Lullo AG, De Ghetto G, Guadagnini A (2018) Probabilistic analysis of risk and mitigation of deepwater well blowouts and oil spills. Stoch Environ Res Risk Assess 32:2647–2666. https://doi.org/10.1007/s00477-017-1509-6
Chevalier S, Bués MA, Tournebize J, Banton O (2001) Stochastic delineation of wellhead protection area in fractured aquifers and parametric sensitivity study. Stoch Environ Res Risk Assess 15:205–227. https://doi.org/10.1007/PL00009790
Cooper HH, Bredehoeft JD, Papadopulos IS (1967) Response of a finite-diameter well to an instantaneous charge of water. Water Resour Res 3:263–269. https://doi.org/10.1029/WR003i001p00263
Dagan G (1982) Stochastic modeling of groundwater flow by unconditional and conditional probabilities: 1. conditional simulation and the direct problem. Water Resour Res 18:813–833. https://doi.org/10.1029/WR018i004p00813
Dagan G, Lessoff SC (2007) Transmissivity upscaling in numerical aquifer models of steady well flow: unconditional statistics. Water Resour Res 43:W05431. https://doi.org/10.1029/2006WR005235
Dagan G, Lessoff SC, Fiori A (2009) Is transmissivity a meaningful property of natural formations? Conceptual issues and model development. Water Resour Res 45:W03425. https://doi.org/10.1029/2008WR007410
Darvini G (2014) An example of solute spreading in nonstationary, bounded geological formations. Stoch Environ Res Risk Assess 28:297–306. https://doi.org/10.1007/s00477-013-0749-3
De Bartolo S, Fallico C, Severino G (2018) A fractal analysis of the water retention curve. Hydrol Process 32:1401–1405. https://doi.org/10.1002/hyp.11498
Fallico C, De Bartolo S, Troisi S, Veltri M (2010) Scaling analysis of hydraulic conductivity and porosity on a sandy medium of an unconfined aquifer reproduced in the laboratory. Geoderma 160:3–12. https://doi.org/10.1016/j.geoderma.2010.09.014
Fallico C, Ianchello M, De Bartolo S, Severino G (2018) Spatial dependence of the hydraulic conductivity in a well-type configuration at the mesoscale. Hydrol Process. https://doi.org/10.1002/hyp.11422
Fallico C, Vita MC, De Bartolo S, Straface S (2012) Scaling effect of the hydraulic conductivity in a confined aquifer. Soil Sci 177:385–391. https://doi.org/10.1097/SS.0b013e31824f179c
Giménez D, Rawls W, Lauren J (1999) Scaling properties of saturated hydraulic conductivity in soil. Geoderma 88:205–220. https://doi.org/10.1016/S0016-7061(98)00105-0
Gómez S, Severino G, Randazzo L, Toraldo G, Otero J (2009) Identification of the hydraulic conductivity using a global optimization method. Agric Water Manag 96:504–510. https://doi.org/10.1016/j.agwat.2008.09.025
Harp DR, Vesselinov VV (2010) Stochastic inverse method for estimation of geostatistical representation of hydrogeologic stratigraphy using borehole logs and pressure observations. Stoch Environ Res Risk Assess 24:1023–1042. https://doi.org/10.1007/s00477-010-0403-2
Hvorslev MJ (1951) Time lag and soil permeability in ground-water observations. Waterways experiment station corps of engineers. US Army Bull 36:1–50
Indelman P (1996) Averaging of unsteady flows in heterogeneous media of stationary conductivity. J Fluid Mech 310:39–60. https://doi.org/10.1017/S0022112096001723
Indelman P (2000) Unsteady source flow in weakly heterogeneous porous media. Comput Geosci 4:351–381. https://doi.org/10.1023/A:101153141
Indelman P (2001) Steady-state source flow in heterogeneous porous media. Transp Porous Media 45:105–127. https://doi.org/10.1023/A:101181441
Indelman P (2004) On macrodispersion in uniform—radial divergent flow through weakly heterogeneous aquifers. Stoch Environ Res Risk Assess 18:16–21. https://doi.org/10.1007/s00477-003-0165-1
Indelman P, Abramovich B (1994) Nonlocal properties of nonuniform averaged flows in heterogeneous media. Water Resour Res 30:3385–3393. https://doi.org/10.1029/94WR01782
Indelman P, Fiori A, Dagan G (1996) Steady flow toward wells in heterogeneous formations: mean head and equivalent conductivity. Water Resour Res 32:1975–1983. https://doi.org/10.1029/96WR00990
Indelman P, Lessoff S, Dagan G (2006) Analytical solution to transport in three-dimensional heterogeneous well capture zones. J Contam Hydrol 87:1–21. https://doi.org/10.1016/j.jconhyd.2006.04.005
Knudby C, Carrera J (2006) On the use of apparent hydraulic diffusivity as an indicator of connectivity. J Hydrol 329:377–389. https://doi.org/10.1016/j.jhydrol.2006.02.026
Lichtner PC, Tartakovsky DM (2003) Stochastic analysis of effective rate constant for heterogeneous reactions. Stoch Environ Res Risk Assess 17:419–429. https://doi.org/10.1007/s00477-003-0163-3
Neuman SP (1994) Generalized scaling of permeabilities: validation and effect of support scale. Geophys Res Lett 21:349–352. https://doi.org/10.1029/94GL00308
Neuman SP, Tartakovsky D, Wallstrom TC, Winter CL (1996) Correction to “prediction of steady state flow in nonuniform geologic media by conditional moments: exact nonlocal formalism, effective conductivities, and weak approximation” by Shlomo P. Neuman and Shlomo Orr. Water Resour Res 32:1479–1480. https://doi.org/10.1029/96WR00489
Ritzi RW, Dominic DF, Slesers AJ, Greer CB, Reboulet EC, Telford JA, Masters RW, Klohe CA, Bogle JL, Means BP (2000) Comparing statistical models of physical heterogeneity in buried-valley aquifers. Water Resour Res 36:3179–3192. https://doi.org/10.1029/2000WR900143
Riva M, Guadagnini A, Neuman SP, Franzetti S (2001) Radial flow in a bounded randomly heterogeneous aquifer. Transp Porous Media 45:139–193. https://doi.org/10.1023/A:1011880602668
Riva M, Guadagnini L, Guadagnini A (2010) Effects of uncertainty of lithofacies, conductivity and porosity distributions on stochastic interpretations of a field scale tracer test. Stoch Environ Res Risk Assess 24:955–970. https://doi.org/10.1007/s00477-010-0399-7
Rubin Y (2003) Applied sthocastic hydrogeology. Oxford University Press, Oxford
Schulze-Makuch D, Cherkauer DS (1997) Method developed for extrapolating scale behavior. Eos Trans Am Geophys Union 78:3–3. https://doi.org/10.1029/97EO00005
Schulze-Makuch D, Cherkauer DS (1998) Variations in hydraulic conductivity with scale of measurement during aquifer tests in heterogeneous, porous carbonate rocks. Hydrogeol J 6:204–215. https://doi.org/10.1007/s100400050145
Severino G (2011a) Macrodispersion by point-like source flows in randomly heterogeneous porous media. Transp Porous Media 89:121–134. https://doi.org/10.1007/s11242-011-9758-1
Severino G (2011b) Stochastic analysis of well-type flows in randomly heterogeneous porous formations. Water Resour Res 47:W03520. https://doi.org/10.1029/2010WR009840
Severino G (2019) Effective conductivity in steady well-type flows through porous formations. Stoch Environ Res Risk Assess 33(3):827–835. https://doi.org/10.1007/s00477-018-1639-5
Severino G, Comegna A, Coppola A, Sommella A, Santini A (2010) Stochastic analysis of a field-scale unsaturated transport experiment. Adv Water Resour 33:1188–1198. https://doi.org/10.1016/j.advwatres.2010.09.004
Severino G, Coppola A (2012) A note on the apparent conductivity of stratified porous media in unsaturated steady flow above a water table. Transp Porous Media 91:733–740. https://doi.org/10.1007/s11242-011-9870-2
Severino G, Cuomo S (2019) On a class of integrals useful to solve well-type flows in heterogeneous porous formations. Water Resour Res. https://doi.org/10.1029/2019WR025062
Severino G, De Bartolo S, Toraldo G, Srinivasan G, Viswanathan H (2012a) Travel time approach to kinetically sorbing solute by diverging radial flows through heterogeneous porous formations. Water Resour Res 48:W12527. https://doi.org/10.1029/2012WR012608
Severino G, Leveque S, Toraldo G (2019) Uncertainty quantification of unsteady source flows in heterogeneous porous media. J Fluid Mech. https://doi.org/10.1017/jfm.2019.203
Severino G, Monetti VM, Santini A, Toraldo G (2006) Unsaturated transport with linear kinetic sorption under unsteady vertical flow. Transp Porous Media 63:147–174. https://doi.org/10.1007/s11242-005-4424-0
Severino G, Santini A (2005) On the effective hydraulic conductivity in mean vertical unsaturated steady flows. Adv Water Resour 28:964–974. https://doi.org/10.1016/j.advwatres.2005.03.003
Severino G, Santini A, Sommella A (2003) Determining the soil hydraulic conductivity by means of a field scale internal drainage. J Hydrol 273:234–248. https://doi.org/10.1016/S0022-1694(02)00390-6
Severino G, Santini A, Sommella A (2008) Steady flows driven by sources of random strength in heterogeneous aquifers with application to partially penetrating wells. Stoch Environ Res Risk Assess 22:567–582. https://doi.org/10.1007/s00477-007-0175-5
Severino G, Santini A, Sommella A (2011) Macrodispersion by diverging radial flows in randomly heterogeneous porous media. J Contam Hydrol 123:40–49. https://doi.org/10.1016/j.jconhyd.2010.12.005
Severino G, Scarfato M, Comegna A (2017) Stochastic analysis of unsaturated steady flows above the water table. Water Resour Res 53:6687–6708. https://doi.org/10.1002/2017WR020554
Severino G, Tartakovsky D, Srinivasan G, Viswanathan H (2012b) Lagrangian models of reactive transport in heterogeneous porous media with uncertain properties. Proc R Soc A 468:1154–1174. https://doi.org/10.1098/rspa.2011.0375
Ye M, Neuman SP, Guadagnini A, Tartakovsky DM (2004) Nonlocal and localized analyses of conditional mean transient flow in bounded, randomly heterogeneous porous media. Water Resour Res. https://doi.org/10.1029/2003WR002099
Acknowledgements
The constructive comments from two anonymous Referees have been deeply appreciated, and they have significantly improved the early version of the manuscript. This study was developed within the GNCS (Gruppo Nazionale Calcolo Scientifico - INdAM) framework.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Severino, G., De Bartolo, S., Brunetti, G. et al. Experimental evidence of the stochastic behavior of the conductivity in radial flow configurations. Stoch Environ Res Risk Assess 33, 1651–1657 (2019). https://doi.org/10.1007/s00477-019-01704-z
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00477-019-01704-z