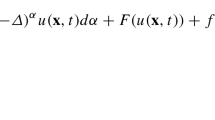Skip to main content
SpringerLink
Log in

A Petrov–Galerkin finite element-meshfree formulation for multi-dimensional fractional diffusion equations

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

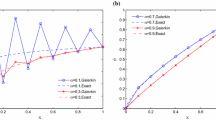
Meshfree methods with arbitrary order smooth approximation are very attractive for accurate numerical modeling of fractional differential equations, especially for multi-dimensional problems. However, the non-local property of fractional derivatives poses considerable difficulty and complexity for the numerical simulations of fractional differential equations and this issue becomes much more severe for meshfree methods due to the rational nature of their shape functions. In order to resolve this issue, a new weak formulation regarding multi-dimensional Riemann–Liouville fractional diffusion equations is introduced through unequally splitting the original fractional derivative of the governing equation into a fractional derivative for the weight function and an integer derivative for the trial function. Accordingly, a Petrov–Galerkin finite element-meshfree method is developed, where smooth reproducing kernel meshfree shape functions are adopted for the trial function approximation to enhance the solution accuracy, and the discretization of weight function is realized by the explicit finite element shape functions with an analytical fractional derivative evaluation to further reduce the computational complexity and improve efficiency. The proposed method enables a direct and efficient employment of meshfree approximation, and also eliminates the undesirable singular integration problem arising in the fractional derivative computation of meshfree shape functions. A nonlinear extension of the proposed method to the fractional Allen–Cahn equation is presented as well. The effectiveness of the proposed methodology is consistently demonstrated by numerical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30
Fig. 31
Fig. 32
Fig. 33
Fig. 34
Fig. 35

Similar content being viewed by others

References

  1. Miller KS, Ross B (1993) An introduction to the fractional calculus and differential equations. Wiley, Hoboken

    MATH  Google Scholar 

  2. Podlubny I (1999) Fractional differential equations. Academic Press, Cambridge

    MATH  Google Scholar 

  3. Kilbas AA, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations. Elsevier, Amsterdam

    MATH  Google Scholar 

  4. Yang X, Machado JT (2017) A new fractional operator of variable order: application in the description of anomalous diffusion. Physica A 481:276–283

    MathSciNet  Google Scholar 

  5. Zhang X, Liu L, Wu Y, Wiwatanapataphee B (2017) Nontrivial solutions for a fractional advection dispersion equation in anomalous diffusion. Appl Math Lett 66:1–8

    MathSciNet  MATH  Google Scholar 

  6. Weron A, Janczura J, Boryczka E, Sungkaworn T, Calebiro D (2019) Statistical testing approach for fractional anomalous diffusion classification. Phys Rev E 99:042149

    Google Scholar 

  7. Chang A, Sun H (2018) Time-space fractional derivative models for CO2 transport in heterogeneous media. Fract Calc Appl Anal 21:151–173

    MathSciNet  MATH  Google Scholar 

  8. Obembe AD, Hossain ME, Abu-Khamsin SA (2017) Variable-order derivative time fractional diffusion model for heterogeneous porous media. J Petrol Sci Eng 152:391–405

    Google Scholar 

  9. Ezzat MA (2010) Thermoelectric MHD non-Newtonian fluid with fractional derivative heat transfer. Physica B 405:4188–4194

    Google Scholar 

  10. Feng L, Liu F, Turner I, Zheng L (2018) Novel numerical analysis of multi-term time fractional viscoelastic non-Newtonian fluid models for simulating unsteady MHD Couette flow of a generalized Oldroyd-B fluid. Fract Calc Appl Anal 21:1073–1103

    MathSciNet  MATH  Google Scholar 

  11. Meerschaert MM, Tadjeran C (2004) Finite difference approximations for fractional advection–dispersion flow equations. J Comput Appl Math 172:65–77

    MathSciNet  MATH  Google Scholar 

  12. Lin Y, Xu CJ (2007) Finite difference/spectral approximations for the time-fractional diffusion equation. J Comput Phys 225:1533–1552

    MathSciNet  MATH  Google Scholar 

  13. Alikhanov AA (2015) A new difference scheme for the time fractional diffusion equation. J Comput Phys 280:424–438

    MathSciNet  MATH  Google Scholar 

  14. Guo X, Li Y, Wang H (2018) A fourth-order scheme for space fractional diffusion equations. J Comput Phys 373:410–424

    MathSciNet  MATH  Google Scholar 

  15. Duo S, Wyk HW, Zhang Y (2018) A novel and accurate finite difference method for the fractional Laplacian and the fractional Poisson problem. J Comput Phys 355:233–252

    MathSciNet  MATH  Google Scholar 

  16. Tang S, Ying Y, Lian Y, Lin S, Yang Y, Wagner GJ, Liu WK (2016) Differential operator multiplication method for fractional differential equations. Comput Mech 58:879–888

    MathSciNet  MATH  Google Scholar 

  17. Jia J, Wang H (2016) A fast finite volume method for conservative space-fractional diffusion equations in convex domains. J Comput Phys 310:63–84

    MathSciNet  MATH  Google Scholar 

  18. Simmons A, Yang Q, Moroney T (2017) A finite volume method for two-sided fractional diffusion equations on non-uniform meshes. J Comput Phys 335:747–759

    MathSciNet  MATH  Google Scholar 

  19. Li J, Liu F, Feng L, Turner I (2017) A novel finite volume method for the Riesz space distributed-order advection–diffusion equation. Appl Math Model 46:536–553

    MathSciNet  MATH  Google Scholar 

  20. Deng W (2008) Finite element method for the space and time fractional Fokker–Planck equation. SIAM J Numer Anal 47:204–226

    MathSciNet  MATH  Google Scholar 

  21. Zhao Y, Zhang Y, Shi D, Liu F, Turner I (2016) Superconvergence analysis of nonconforming finite element method for two-dimensional time fractional diffusion equations. Appl Math Lett 59:38–47

    MathSciNet  MATH  Google Scholar 

  22. Lian Y, Ying Y, Tang S, Lin S, Wagner GJ, Liu WK (2016) A Petrov–Galerkin finite element method for the fractional advection–diffusion equation. Comput Methods Appl Mech Eng 309:388–410

    MathSciNet  MATH  Google Scholar 

  23. Luan S, Lian Y, Ying Y, Tang S, Wagner GJ, Liu WK (2017) An enriched finite element method to fractional advection–diffusion equation. Comput Mech 60:181–201

    MathSciNet  MATH  Google Scholar 

  24. Lin Z, Wang D (2018) A finite element formulation preserving symmetric and banded diffusion stiffness matrix characteristics for fractional differential equations. Comput Mech 62:185–211

    MathSciNet  MATH  Google Scholar 

  25. Xu Q, Hesthaven JS (2014) Stable multi-domain spectral penalty methods for fractional partial differential equations. J Comput Phys 257:241–258

    MathSciNet  MATH  Google Scholar 

  26. Zayernouri M, Ainsworth M, Karniadakis GE (2015) A unified Petrov–Galerkin spectral method for fractional PDEs. Comput Methods Appl Mech Eng 283:1545–1569

    MathSciNet  MATH  Google Scholar 

  27. Song F, Xu CJ, Karniadakis GE (2016) A fractional phase-field model for two-phase flows with tunable sharpness: algorithms and simulations. Comput Methods Appl Mech Eng 305:376–404

    MathSciNet  MATH  Google Scholar 

  28. Mao Z, Shen J (2017) Hermite spectral methods for fractional PDEs in unbounded domains. SIAM J Sci Comput 39:A1928–A1950

    MathSciNet  MATH  Google Scholar 

  29. Wang J, Xiao A (2019) Conservative Fourier spectral method and numerical investigation of space fractional Klein–Gordon–Schrödinger equations. Appl Math Comput 350:348–365

    MathSciNet  MATH  Google Scholar 

  30. Belytschko T, Krongauz Y, Organ D, Fleming M, Krysl P (1996) Meshless methods: an overview and recent developments. Comput Methods Appl Mech Eng 139:3–47

    MATH  Google Scholar 

  31. Liu WK, Chen Y, Jun S, Chen JS, Belytschko T, Pan C, Uras RA, Chang CT (1996) Overview and applications of the reproducing kernel particle methods. Arch Comput Methods Eng 3:3–80

    MathSciNet  Google Scholar 

  32. Atluri SN, Shen S (2002) The meshless local Petrov–Galerkin (MLPG) method. Tech Science, Henderson

    MATH  Google Scholar 

  33. Babuška I, Banerjee U, Osborn JE (2003) Survey of meshless and generalized finite element methods: a unified approach. Acta Numer 12:1–125

    MathSciNet  MATH  Google Scholar 

  34. Li S, Liu WK (2004) Meshfree particle methods. Springer, Berlin

    MATH  Google Scholar 

  35. Zhang X, Liu Y (2004) Meshless methods. Tsinghua University Press & Springer, Beijing

    Google Scholar 

  36. Nguyen VP, Rabczuk T, Bordas S, Duflot M (2008) Meshless methods: a review and computer implementation aspects. Math Comput Simul 79:763–813

    MathSciNet  MATH  Google Scholar 

  37. Liu GR (2009) Meshfree methods: moving beyond the finite element method, 2nd edn. CRC Press, Boca Raton

    Google Scholar 

  38. Chen JS, Hillman M, Chi SW (2017) Meshfree methods: progress made after 20 years. J Eng Mech ASCE 143:04017001

    Google Scholar 

  39. Wang D, Wu J (2019) An inherently consistent reproducing kernel gradient smoothing framework toward efficient Galerkin meshfree formulation with explicit quadrature. Comput Methods Appl Mech Eng 349:628–672

    MathSciNet  MATH  Google Scholar 

  40. Zhuang P, Gu YT, Liu F, Turner I, Yarlagadda PK (2011) Time-dependent fractional advection–diffusion equations by an implicit MLS meshless method. Int J Numer Methods Eng 88:1346–1362

    MathSciNet  MATH  Google Scholar 

  41. Liu Q, Gu YT, Zhuang P, Liu F, Nie Y (2011) An implicit RBF meshless approach for time fractional diffusion equations. Comput Mech 48:1–12

    MathSciNet  MATH  Google Scholar 

  42. Mohebbi A, Abbaszadeh M, Dehghan M (2013) The use of a meshless technique based on collocation and radial basis functions for solving the time fractional nonlinear Schrödinger equation arising in quantum mechanics. Eng Anal Bound Elem 37:475–485

    MathSciNet  MATH  Google Scholar 

  43. Lian Y, Gregory JW, Liu WK (2017) A meshfree method for the fractional advection–diffusion equation. In: Griebel M, Schweitzer M (eds) Meshfree methods for partial differential equations VIII. Springer, Cham, pp 53–66

    Google Scholar 

  44. Ying Y, Lian Y, Tang S, Liu WK (2018) Enriched reproducing kernel particle method for fractional advection–diffusion equation. Acta Mech Sin 34:515–527

    MathSciNet  MATH  Google Scholar 

  45. Lin Z, Liu F, Wang D, Gu YT (2018) Reproducing kernel particle method for two-dimensional time-space fractional diffusion equations in irregular domains. Eng Anal Bound Elem 97:131–143

    MathSciNet  MATH  Google Scholar 

  46. Tayebi A, Shekari Y, Heydari MH (2017) A meshless method for solving two-dimensional variable-order time fractional advection–diffusion equation. J Comput Phys 340:655–669

    MathSciNet  MATH  Google Scholar 

  47. Shekari Y, Tayebi A, Heydari MH (2019) A meshfree approach for solving 2D variable-order fractional nonlinear diffusion-wave equation. Comput Methods Appl Mech Eng 350:154–168

    MathSciNet  MATH  Google Scholar 

  48. Hussain M, Haq S, Ghafoor A (2020) Meshless RBFs method for numerical solutions of two-dimensional high order fractional Sobolev equations. Comput Math Appl 79:802–816

    MathSciNet  Google Scholar 

  49. Nayroles B, Touzot G, Villon P (1992) Generalizing the finite element method: diffuse approximation and diffuse elements. Comput Mech 10:307–318

    MathSciNet  MATH  Google Scholar 

  50. Belytschko T, Lu YY, Gu L (1994) Element-free Galerkin methods. Int J Numer Methods Eng 37:229–256

    MathSciNet  MATH  Google Scholar 

  51. Liu WK, Jun S, Zhang YF (1995) Reproducing kernel particle methods. Int J Numer Methods Fluids 20:1081–1106

    MathSciNet  MATH  Google Scholar 

  52. Chen JS, Pan C, Wu CT, Liu WK (1996) Reproducing kernel particle methods for large deformation analysis of non-linear structures. Comput Methods Appl Mech Eng 139:195–227

    MathSciNet  MATH  Google Scholar 

  53. Wu CT, Park CK, Chen JS (2011) A generalized approximation for the meshfree analysis of solids. Int J Numer Methods Eng 85:693–722

    MATH  Google Scholar 

  54. Wang D, Chen P (2014) Quasi-convex reproducing kernel meshfree method. Comput Mech 54:689–709

    MathSciNet  MATH  Google Scholar 

  55. Yin B, Liu Y, Li H, He S (2019) Fast algorithm based on TT-M FE system for space fractional Allen–Cahn equations with smooth and non-smooth solutions. J Comput Phys 379:351–372

    MathSciNet  Google Scholar 

  56. Zheng L, Zhang X (2017) Modeling and analysis of modern fluid problems. Academic Press, Cambridge

    MATH  Google Scholar 

  57. Roop JP (2006) Computational aspects of FEM approximation of fractional advection dispersion equations on bounded domains in R2. J Comput Appl Math 193:243–268

    MathSciNet  MATH  Google Scholar 

  58. Teodoro GS, Machado JT, Oliveira EC (2019) A review of definitions of fractional derivatives and other operators. J Comput Phys 388:195–208

    MathSciNet  Google Scholar 

  59. Samko SG, Kilbas AA, Marichev OI (1993) Fractional integrals and derivatives: theory and applications. Gordon and Breach Science Publishers, London

    MATH  Google Scholar 

  60. Lions JL, Magenes E (1972) Non-homogeneous boundary value problems and applications. Springer, Berlin

    MATH  Google Scholar 

  61. Li X, Xu CJ (2009) A space-time spectral method for the time fractional diffusion equation. SIAM J Numer Anal 47:2108–2131

    MathSciNet  MATH  Google Scholar 

  62. Zhao Y, Bu WP, Zhao X, Tang YF (2017) Galerkin finite element method for two-dimensional space and time fractional Bloch–Torrey equation. J Comput Phys 350:117–135

    MathSciNet  MATH  Google Scholar 

  63. Feng L, Liu F, Turner I (2019) Finite difference/finite element method for a novel 2D multi-term time-fractional mixed sub-diffusion and diffusion-wave equation on convex domains. Commun Nonlinear Sci Numer Simul 70:354–371

    MathSciNet  Google Scholar 

  64. Shen J, Tang T, Wang L (2011) Spectral methods: algorithms, analysis and applications. Springer, Berlin

    MATH  Google Scholar 

  65. Li X, Xu CJ (2010) Existence and uniqueness of the weak solution of the space-time fractional diffusion equation and a spectral method approximation. Commun Comput Phys 8:1016–1051

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The support of this work by the National Natural Science Foundation of China (11772280, 11472233) and the SIAT Innovation Program for Excellent Young Researchers is gratefully acknowledged.

Author information

Authors and Affiliations

  1. Shenzhen Institutes of Advanced Technology, Chinese Academy of Sciences, Shenzhen, 518055, Guangdong, China

    Zeng Lin

  2. Department of Civil Engineering, Xiamen University, Xiamen, 361005, Fujian, China

    Zeng Lin, Dongdong Wang, Dongliang Qi & Like Deng

Authors
  1. Zeng Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dongdong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dongliang Qi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Like Deng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dongdong Wang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lin, Z., Wang, D., Qi, D. et al. A Petrov–Galerkin finite element-meshfree formulation for multi-dimensional fractional diffusion equations. Comput Mech 66, 323–350 (2020). https://doi.org/10.1007/s00466-020-01853-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-020-01853-x

Keywords

Search

Navigation