Skip to main content
SpringerLink
Log in

Simulating the pervasive fracture of materials and structures using randomly close packed Voronoi tessellations

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

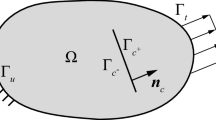
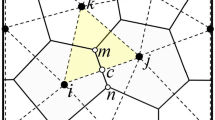
Under extreme loading conditions most often the extent of material and structural fracture is pervasive in the sense that a multitude of cracks are nucleating, propagating in arbitrary directions, coalescing, and branching. Pervasive fracture is a highly nonlinear process involving complex material constitutive behavior, material softening, localization, surface generation, and ubiquitous contact. A pure Lagrangian computational method based on randomly close packed Voronoi tessellations is proposed as a rational and robust approach for simulating the pervasive fracture of materials and structures. Each Voronoi cell is formulated as a finite element using the Reproducing Kernel Method. Fracture surfaces are allowed to nucleate only at the intercell faces, and cohesive tractions are dynamically inserted. The randomly seeded Voronoi cells provide a regularized random network for representing fracture surfaces. Example problems are used to demonstrate the proposed numerical method. The primary numerical challenge for this class of problems is the demonstration of model objectivity and, in particular, the identification and demonstration of a measure of convergence for engineering quantities of interest.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Aste R, Weaire D (2000) The pursuit of perfect packing. Institute of Physics Publishing, Bristol

    Book  MATH  Google Scholar 

  2. Attaway S, Heinstein M, Swegle J (1994) Coupling of smooth particle hydrodynamics with the finite element method. Nuclear Eng Design 150: 199–205

    Article  Google Scholar 

  3. Bazant Z (2005) Scaling of structural strength. Elsevier, Amsterdam

    MATH  Google Scholar 

  4. Bazant Z, Planas J (1997) Fracture and size effect in concrete and other quasibrittle materials. CRC Press, Boca Raton

    Google Scholar 

  5. Belytschko T, Black T (1999) Elastic crack growth in finite elements with minimal remeshing. Int J Numer Methods Eng 45: 601–620

    Article  MATH  MathSciNet  Google Scholar 

  6. Belytschko T, Krongauz Y, Organ D, Flemin M, Krysl P (1996) Meshless melthods: an overview and recent developments. Comput Methods Appl Mech Eng 139: 3–47

    Article  MATH  Google Scholar 

  7. Belytschko T, Krysl P, Krongauz Y (1997) A three-dimensional explicit element-free Galerkin method. Int J Numer Methods Fluids 24: 1253–1270

    Article  MATH  Google Scholar 

  8. Belytschko T, Liu WK, Moran B (2000) Nonlinear finite elements for continua and structures, Ch. 4.7. Wiley, London

    Google Scholar 

  9. Belytschko T, Liu WK, Moran B (2000) Nonlinear finite elements for continua and structures, Ch. 6.2. Wiley, London

    Google Scholar 

  10. Bolander J, Saito S (1998) Fracture analyses using spring networks with random geometry. Eng Fract Mech 61: 569–591

    Article  Google Scholar 

  11. Bolander J, Sukumar N (2005) Irregular lattice model for quasistatic crack propagation. Phys Rev B 71, 094106-1:12

    Google Scholar 

  12. Bowyer A (1981) Computing dirichlet tessellations. Comput J 2: 162–166

    Article  MathSciNet  Google Scholar 

  13. Camacho G, Ortiz M (1996) Computational modeling of impact damage in brittle materials. Int J Solids Struct 33: 2899–2938

    Article  MATH  Google Scholar 

  14. Carpinteri A, Chiaia B, Invernizzi S (1999) Three-Dimensional fractal analysis of concrete fracture at the meso-level. Theor Appl Fract Mech 31: 163–172

    Article  Google Scholar 

  15. Carpinteri A, Pugno N (2005) Fractal fragmentation theory for size effects of Quasi-Brittle materials in compression. Mag Concr Res 57: 309–313

    Article  Google Scholar 

  16. Chen J, Wu C, Yoon S, You Y (2001) A stabalized conforming nodal integration for Galerkin mesh-free methods. Int J Numer Methods Eng 50: 435–466

    Article  MATH  Google Scholar 

  17. Cusatis G, Cedolin L (2007) Two-scale study of concrete fracturing behavior. Eng Fract Mech 74: 3–17

    Article  Google Scholar 

  18. Dai K, Liu G, Nguyen T (2007) An n-sided polygonal smoothed finite element method (nSFEM) for solid mechanics. Finite Elem Anal Des 43: 847–860

    Article  MathSciNet  Google Scholar 

  19. di Bernardo M, Budd C, Champneys A, Kowalczyk P (2008) Piecewise-smooth dynamical systems: theory and applications. Springer, Berlin

    MATH  Google Scholar 

  20. Finney J (1970) Random packings and the structure of simple liquids. I. The geometry of random close packing. In: Proceedings of the royal society of London. Series A, Mathematical and Physical Sciences, vol 319, pp 479–493

  21. Gallian J (1994) Contemporary abstract algebra, 3rd edn. D.C Heath and Company

  22. Ghosh S, Moorthy S (2004) Three dimensional Voronoi cell finite element model for microstructures with ellipsoidal heterogeneities. Comput Mech 34: 510–531

    Article  MATH  Google Scholar 

  23. Hamming R. (1950) Error detecting and error correcting codes. Bell Syst Tech J 26: 147–160

    MathSciNet  Google Scholar 

  24. Heinstein M, Mello F, Attaway S, Laurson T (2000) Contact-impact modeling in explicit transient dynamics. Comput Methods Appl Mech Eng 187: 621–640

    Article  MATH  Google Scholar 

  25. Hinton E, Rock T, Zienkiewicz O (1976) A note on mass lumping and related processes in the finite element method. Earthq Eng Struct Dyn 4: 245–249

    Article  Google Scholar 

  26. Hornbogen E (1989) Fractals in microstructure of metals. Int Mater Rev 34: 277–296

    Google Scholar 

  27. Hu C, Ghosh S (2008) Locally enhanced Voronoi cell finite element model (LE-VCFEM) for simulating evolving fracture in ductile microstructures containing inclusions. Int J Numer Methods Eng 76: 1955–1992

    Article  MathSciNet  Google Scholar 

  28. Hughes T (1987) The finite element method: linear static and dynamic finite element analysis, Ch. 4.4. Prentice-Hall, Englewood Cliffs

    Google Scholar 

  29. Hughes T (1987) The finite element method: linear static and dynamic finite element analysis, Ch. 4.5. Prentice-Hall, Englewood Cliffs

    Google Scholar 

  30. Hughes T (1987) The finite element method: linear static and dynamic finite element analysis, Ch. 7.3.2. Prentice-Hall, Englewood Cliffs

    Google Scholar 

  31. Idelsohn S, Onate E, Calvo N, Pin F (2003) The meshless finite element method. Int J Numer Methods Eng 58: 893–912

    Article  MATH  Google Scholar 

  32. Johnson G, Beissel S, Stryk R (2002) An improved generalized particle algorithm that includes boundaries and interfaces. Int J Numer Methods Eng 53: 875–904

    Article  Google Scholar 

  33. Josuttis N (1999) The C++ standard library: a tutorial and reference. Addison-Wesley, Reading

    Google Scholar 

  34. Kreyszig E (1989) Introductory functional analysis with applications, Ch. 1. Wiley, London

    Google Scholar 

  35. Kythe P (1995) An introduction to boundary element methods. CRC Press, Boca Raton

    MATH  Google Scholar 

  36. Li S, Ghosh S (2006) Multiple cohesive crack growth in brittle materials by the extended Voronoi cell finite element model. Int J Fract 141: 373–393

    Article  Google Scholar 

  37. Li S, Ghosh S (2006) Extended Voronoi cell finite element model for multiple cohesive crack propagation in brittle materials. Int J Numer Methods Eng 65: 1028–1067

    Article  MATH  Google Scholar 

  38. Lindgren B (1976) Statistical theory, 3rd edn., Ch. 2.5. Macmillan Publishing Co., New York

    Google Scholar 

  39. Liu W, Jun S, Zhang Y (1995) Reproducing kernel particle methods. Int J Numer Methods Eng 20: 1081–1106

    Article  MATH  MathSciNet  Google Scholar 

  40. Lowen H (2000) Fun with hard spheres. In: Mecke K, Stoyan D (eds) Statistical physics and spatial statistics. The art of analyzing spatial structures and pattern formation. Springer lecture notes in physics, vol 554, pp 295–331

  41. Mohammadi S (2003) Discontinuum mechanics: using discrete and finite elements. WIT Press, Billerica

    MATH  Google Scholar 

  42. Molinari J, Gazonas G, Raghupathy R, Rusinek A, Zhou F (2007) The cohesive element approach to dynamic fragmentation: the question of energy convergence. Int J Numer Methods Eng 69: 484–503

    Article  Google Scholar 

  43. Okabe A, Boots B, Sugihara K, Chiu S (2000) Spatial tessellations: concepts and applications of Voronoi diagrams. Wiley, London

    MATH  Google Scholar 

  44. Ortiz M, Pandolfi A (1999) Finite-Deformation irreversible cohesive elements for three-dimensional crack-propagation analysis. Int J Numer Methods Eng 44: 1267–1282

    Article  MATH  Google Scholar 

  45. Pandolfi A, Krysl P, Ortiz M (1999) Finite element simulation of ring expansion and fragmentation: the capturing of length and time scales through cohesive models of fracture. Int J Fract 95: 279–297

    Article  Google Scholar 

  46. Pandolfi A, Ortiz M (2002) An efficient adaptive procedure for three-dimensional fragmentation simulations. Eng Comput 18: 148–159

    Article  Google Scholar 

  47. Park Y, Fahrenthold E (2005) A Kernel free Particle-Finite element method for hypervelocity impact simulation. Int J Numer Methods Eng 63: 737–759

    Article  MATH  Google Scholar 

  48. Perfect E (1997) Fractal models for the fragmentation of rocks and soils: a review. Eng Geol 48: 185–198

    Article  Google Scholar 

  49. Planas J, Elices M, Guinea G, Gomez F, Cendon D, Arbilla I (2003) Generalizations and specializations of cohesive crack models. Eng Fract Mech 70: 1759–1776

    Article  Google Scholar 

  50. Rabczuk T, Belytschko T (2004) Cracking particles: a simplified meshfree method for arbitrary evolving cracks. Int J Numer Methods Eng 61: 2316–2343

    Article  MATH  Google Scholar 

  51. Rashid M, Selimotic M (2006) A three-dimensional finite element method with arbitrary polyhedral elements. Int J Numer Methods Eng 67: 226–252

    Article  MATH  MathSciNet  Google Scholar 

  52. Ruiz G, Ortiz M, Pandolfi A (2000) Three-Dimensional finite-element simulation of the dynamic brazilian test on concrete cylinders. Int J Numer Methods Eng 48: 963–994

    Article  MATH  Google Scholar 

  53. Ruiz G, Pandolfi A, Ortiz M (2001) Three-Dimensional cohesive modeling of dynamic mixed-mode fracture. Int J Numer Methods Eng 52: 97–120

    Article  Google Scholar 

  54. Schuster H, Just W (2005) Deterministic chaos, an introduction, 4th edn. Wiley-VCH, New York

    MATH  Google Scholar 

  55. Silling S, Askari E (2005) A meshfree method based on the peridynamic model of solid mechanics. Comput Struct 83: 1526–1535

    Article  Google Scholar 

  56. Strogatz S (1994) Nonlinear dynamics and chaos with applications to physics, biology, chemistry, and engineering, Ch. 9. Westview Press, Boulder

    Google Scholar 

  57. Strouboulis T, Copps K, Babuska I (2000) The generalized finite element method: an example of its implementation and illustration of its performance. Int J Numer Methods Eng 47: 1401–1417

    Article  MATH  MathSciNet  Google Scholar 

  58. Sukumar N, Tabarraei A (2004) Conforming polygonal finite elements. Int J Numer Methods Eng 61: 2045–2066

    Article  MATH  MathSciNet  Google Scholar 

  59. Sulsky D, Schreyer L (2004) MPM simulation of dynamic material failure with a decohesion constitutive model. Eur J Mech A/Solids 23: 423–445

    Article  MATH  Google Scholar 

  60. Wachspress E (1975) A rational finite element basis. Academic Press, London

    MATH  Google Scholar 

  61. Watson D (1981) Computing the n-Dimensional tessellation with application to Voronoi polytopes. Comput J 2: 167–172

    Article  Google Scholar 

  62. Yip M, Li Z, Liao B, Bolander J (2006) Irregular lattice models of fracture of multiphase particulate materials. Int J Fract 140: 113–124

    Article  MATH  Google Scholar 

  63. Yip M, Mohle J, Bolander J (2005) Automated modeling of three-dimensional structural components using irregular lattices. Comput Aided Civil Infrastructure Eng 20: 393–407

    Article  Google Scholar 

  64. Zallen R (2004) The physics of amorphous solids, Ch. 2. Wiley-VCH, New York

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Engineering Sciences Center, Sandia National Laboratories, Albuquerque, NM, 87185, USA

    Joseph E. Bishop

Authors
  1. Joseph E. Bishop
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Joseph E. Bishop.

Additional information

Sandia is a multi-program laboratory operated by Sandia Corporation, a Lockheed Martin Company, for the United States Department of Energy’s National Nuclear Security Administration under Contract DE-AC04-94AL85000.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bishop, J.E. Simulating the pervasive fracture of materials and structures using randomly close packed Voronoi tessellations. Comput Mech 44, 455–471 (2009). https://doi.org/10.1007/s00466-009-0383-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-009-0383-6

Keywords

Search

Navigation