Skip to main content
SpringerLink
Log in

Corona Limits of Tilings: Periodic Case

  • Published:
Discrete & Computational Geometry Aims and scope Submit manuscript

Abstract

We study the limit shape of successive coronas of a tiling, which models the growth of crystals. We define basic terminologies and discuss the existence and uniqueness of corona limits, and then prove that corona limits are completely characterized by directional speeds. As an application, we give another proof that the corona limit of a periodic tiling is a centrally symmetric convex polyhedron [see Zhuravlev (St Petersbg Math J 13(2):201–220, 2002) and Maleev and Shutov (Layer-by-layer growth model for partitions, packings, and graphs, Tranzit-X, Vladimir, 2011)].

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Notes

  1. Uniformity is necessary for the existence of corona limit. In fact, we give an example of a repetitive tiling without a corona limit in Sect. 7.

  2. Nakano suggested us an easier proof of Lemma 3.3, which works for any real valued function g(x, t) in two variables.

  3. An infinite dimensional version is due to Krein–Milman (cf. [9, 21]).

References

  1. Akiyama, S., Brunotte, H., Pethő, A., Thuswaldner, J.M.: Generalized radix representations and dynamical systems III. Osaka J. Math. 45(2), 347–374 (2008)

    MathSciNet  MATH  Google Scholar 

  2. Akiyama, S., Imai, K.: The corona limit of Penrose tilings is a regular decagon. In: Proceedings of the 22nd International Workshop on Cellular Automata and Discrete Complex Systems (AUTOMATA’16). Lecture Notes in Computer Science, vol. 9664, pp. 35–48. Springer, Cham (2016)

  3. Baake, M., Grimm, U.: Coordination sequences for root lattices and related graphs. Z. Kristallogr. 212(4), 253–256 (1997)

    MathSciNet  MATH  Google Scholar 

  4. Baake, M., Grimm, U.: Averaged coordination numbers of planar aperiodic tilings. Philos. Mag. 86(3–5), 567–572 (2006)

    Article  Google Scholar 

  5. Bennema, P., van der Eerden, J.P.: Crystal graphs, connected nets, roughening transition and the morphology of crystals. In: Sunagawa, I. (ed.) Morphology of Crystals, Part A, pp. 1–75. Terra, Tokyo (1987)

    Google Scholar 

  6. Böröczky Jr., K., Schnell, U.: Wulff shape for nonperiodic arrangements. Lett. Math. Phys. 45(1), 81–94 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bravais, A.: Les systemes formes par des pointes distributes regulierement sur un plan ou dans l’espace. J. Ecole Polytech. XIX, 1–128 (1850)

  8. Chernov, A.A.: The kinetics of the growth forms of crystals. Sov. Phys. Crystallogr. 7, 728–730 (1963)

    Google Scholar 

  9. Conway, J.B.: A Course in Functional Analysis. Graduate Texts in Mathematics, vol. 96. Springer, New York (1985)

    MATH  Google Scholar 

  10. Donnay, J.D.H., Harker, D.: A new law of crystal morphology extending the law of Bravais. Amer. Miner. 22, 446–467 (1937)

    Google Scholar 

  11. Grünbaum, B., Shephard, G.C.: Tilings and Patterns. W.H. Freeman and Company, New York (1987)

    MATH  Google Scholar 

  12. Hartman, P., Perdok, W.G.: On the relations between structure and morphology of crystals I. Acta Crystallogr. 8, 49–52 (1955)

    Article  Google Scholar 

  13. Kechris, A.S.: Classical Descriptive Set Theory. Graduate Texts in Mathematics, vol. 156. Springer, New York (1995)

    MATH  Google Scholar 

  14. Kolmogorov, A.N.: On the “geometrical selection” of crystals. Dokl. Akad. Nauk SSSR 65, 681–684 (1949)

    Google Scholar 

  15. Maleev, A.V., Shutov, A.V.: Layer-by-Layer Growth Model for Partitions, Packings, and Graphs. Tranzit-X, Vladimir (2011) (in Russian)

  16. Maleev, A.V., Shutov, A.V., Zhuravlev, V.G.: 2D quasi-periodic Rauzy tiling as a section of 3D periodic tiling. Crystallogr. Rep. 55(5), 723–733 (2010)

    Article  Google Scholar 

  17. Schnell, U.: Wulff-shape and density deviation. Geom. Dedicata 79(1), 51–63 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  18. Shutov, A.V., Maleev, A.V.: Inverse problem in the layer-by-layer growth model. Crystallogr. Rep. 59(6), 855–861 (2014)

    Article  Google Scholar 

  19. Shutov, A.V., Maleev, A.V.: Layer-by-layer growth of vertex graph of Penrose tiling. Crystallogr. Rep. 62(5), 683–691 (2017)

    Article  Google Scholar 

  20. Shutov, A.V., Maleev, A.V., Zhuravlev, V.G.: On layer-by-layer growth model for tilings and graphs. In: Proceedings of the 5th All-Russia Scientific School Mathematical Research in Natural Sciences, pp. 126–130. Apatity, GI RAN (2009)

  21. Simon, B.: Convexity: An Analytic Viewpoint. Cambridge Tracts in Mathematics, vol. 187. Cambridge University Press, Cambridge (2011)

    Book  MATH  Google Scholar 

  22. Sunagawa, I.: Growth and morphology of crystals. Forma 14, 147–166 (1999)

    Google Scholar 

  23. Walters, P.: An Introduction to Ergodic Theory. Graduate Texts in Mathematics, vol. 79. Springer, New York (1982)

    MATH  Google Scholar 

  24. Wulff, G.: Zur Frage der Geschwindigkeit des Wachsturms und der Auflösung der Kristallflächen. Z. Kristallogr. 34, 449–530 (1901)

    Google Scholar 

  25. Zhuravlev, V.G.: Self-similar growth of periodic partitions and graphs. St. Petersbg. Math. J. 13(2), 201–220 (2002)

    MATH  Google Scholar 

  26. Zhuravlev, V.G., Maleev, A.V.: Layer-by-layer growth of quasi-periodic Rauzy tiling. Crystallogr. Rep. 52(2), 180–186 (2007)

    Article  Google Scholar 

Download references

Acknowledgements

We express our cordial gratitude to A.V. Shutov for informing us of the current status of the research on corona limits and for providing us some of the references, which are not easily accessible. We are also largely indebted to the anonymous referee and Fumihiko Nakano who gave us invaluable suggestions and related references. The first author is partially supported by JSPS Grants (17K05159, 17H02849, BBD30028). The third author is partially supported by JSPS Grants (26330016, 17K00015). The fourth author is supported by JSPS Grant (15K17505).

Author information

Authors and Affiliations

  1. Institute of Mathematics, University of Tsukuba, 1-1-1 Tennodai, Tsukuba, Ibaraki, Japan

    Shigeki Akiyama & Hajime Kaneko

  2. Institute of Mathematics, College of Science, University of the Philippines Diliman, Quezon City 1101, Philippines

    Jonathan Caalim

  3. Graduate School of Engineering, Hiroshima University, Higashi-Hiroshima, 739-8527, Japan

    Katsunobu Imai

Authors
  1. Shigeki Akiyama
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jonathan Caalim
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Katsunobu Imai
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Hajime Kaneko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shigeki Akiyama.

Additional information

Editor in Charge: Kenneth Clarkson

Appendices

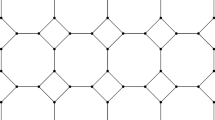
Appendix A: 1-Uniform Tilings and Velocities

Computation of corona limits of 1-uniform tilings using point adjacency and edge adjacency, see Remark 2.1 and Sect. 6. Each row consists of five figures: tiling, (finite) coronas, their velocities, (finite) edge-coronas and their velocities. The convex hull of velocities is the corona limit, see Theorem 5.1 and the description after it.

figure a
figure b

Appendix B: 2-Uniform Tilings and Velocities

The same computation of corona limits of 2-uniform tilings. Each tiling is designated by two vertex configurations joined by semi-colon.

figure c
figure d
figure e
figure f

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Akiyama, S., Caalim, J., Imai, K. et al. Corona Limits of Tilings: Periodic Case. Discrete Comput Geom 61, 626–652 (2019). https://doi.org/10.1007/s00454-018-0033-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00454-018-0033-x

Keywords

Mathematics Subject Classification

Search

Navigation