Optimal Packings of Congruent Circles on a Square Flat Torus

Abstract

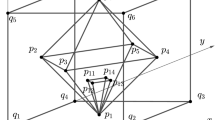
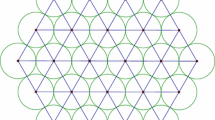
We consider packings of congruent circles on a square flat torus, i.e., periodic (w.r.t. a square lattice) planar circle packings, with the maximal circle radius. This problem is interesting due to a practical reason—the problem of “super resolution of images.” We have found optimal arrangements for $N=6$, 7 and 8 circles. Surprisingly, for the case $N=7$ there are three different optimal arrangements. Our proof is based on a computer enumeration of toroidal irreducible contact graphs.

Appendices

Appendix 1: Numerical Bounds for the Graphs

Here we present the numerical bounds for the edges of $G_1$ and $G_2$ produced by the code

For $G_1$ we have $\delta <0.002$ and $\varepsilon <0.002$. After calculating the inverse of the differential we get $h\ge \frac{1}{16}$, and so the sufficient inequality $\varepsilon <\frac{h}{4}$ holds.

Similarly, for $G_2$ we get $h\ge \frac{1}{35}$ and still $\varepsilon < \frac{h}{4}$.

Appendix 2: Coordinates of the Graphs

Here are some exact values for the coordinates of the vertices of the optimal graphs:

$$\begin{aligned} N= & {} 2: \big (0, 0\big ), \big (\tfrac{1}{2}, \tfrac{1}{2}\big ). \\ N= & {} 3: \big (0, 0\big ), \big (\tfrac{1}{2}, \tfrac{\sqrt{3}}{2}\big ), \big (\tfrac{\sqrt{3}-1}{2}, \tfrac{\sqrt{3}-1}{2}\big ). \\ N= & {} 4: \big (0, 0\big ), \big (\tfrac{1}{2}, \tfrac{\sqrt{3}}{2}\big ), \big (\tfrac{\sqrt{3}-1}{2}, \tfrac{\sqrt{3}-1}{2}\big ), \big (\tfrac{\sqrt{3}}{2}, \tfrac{1}{2}\big ). \\ N= & {} 5: \big (0, 0\big ), \big (\tfrac{2}{5}, \tfrac{1}{5}\big ), \big (\tfrac{4}{5}, \tfrac{2}{5}\big ), \big (\tfrac{1}{5}, \tfrac{3}{5}\big ), \big (\tfrac{3}{5}, \tfrac{4}{5}\big ). \end{aligned}$$

The following was achieved by writing down the system of equations for the graph as it appeared to be (i.e., the edges that seem to be horizontal were assumed horizontal, etc) and solving it in MATLAB.

$$\begin{aligned} N= & {} 6: (0, 0),\\&\Big (\tfrac{1}{4}\,\sqrt{3}-\tfrac{1}{12}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}+\tfrac{1}{12}, -\tfrac{1}{12}\, \Big ( -3\,\sqrt{3}+\sqrt{2}\sqrt{3\,\sqrt{3}+2}-1 \Big ) \sqrt{3}\Big ),\\&\Big ({\tfrac{11}{12}}-\tfrac{1}{4}\,\sqrt{3}+\tfrac{1}{12}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}, -\tfrac{1}{12}\, \Big ( -3\,\sqrt{3}+\sqrt{2}\sqrt{3\,\sqrt{3}+2}-1 \Big ) \sqrt{3}\Big ),\\&\Big (\tfrac{1}{2}, 1-\tfrac{1}{12}\,\sqrt{3}\sqrt{2}\sqrt{3\,\sqrt{3}+2}+\tfrac{1}{3}\,\sqrt{3}-\tfrac{1}{4}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}\Big ),\\&\Big ({\tfrac{5}{12}}-\tfrac{1}{4}\,\sqrt{3}+\tfrac{1}{12}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}, \tfrac{5}{4}+\tfrac{1}{4}\,\sqrt{3}-\tfrac{1}{4}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}\Big ),\\&\Big ({\tfrac{7}{12}}+\tfrac{1}{4}\,\sqrt{3}-\tfrac{1}{12}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}, \tfrac{5}{4}+\tfrac{1}{4}\,\sqrt{3}-\tfrac{1}{4}\,\sqrt{2}\sqrt{3\,\sqrt{3}+2}\Big ). \end{aligned}$$

For $N=7$ and 8 for all the optimal graphs we just write down the edge coordinates, as there are only 6 types of them and they look simpler than the point coordinates: $\Big (\tfrac{1}{2} \frac{1}{1+\sqrt{3}}, \pm \tfrac{\sqrt{3}}{2}\frac{1}{1+\sqrt{3}}\Big ), \Big (\tfrac{\sqrt{3}}{2}\frac{1}{1+\sqrt{3}}, \pm \tfrac{1}{2} \frac{1}{1+\sqrt{3}}\Big ), \Big (0, \frac{1}{1+\sqrt{3}}\Big )$, $\Big (\frac{1}{1+\sqrt{3}}, 0\Big )$.