Skip to main content
SpringerLink
Log in

Galerkin’s method to solve a fractional time-delayed jerk oscillator

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

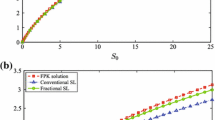
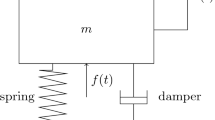
Abstract

An immediate analytic solution for the time-delayed fractional linear jerk oscillator is derived in the present study. The currently used method adheres to basic, easily implementable criteria. This proposed approach is based on transforming the time-delayed fractional oscillator to an equivalent oscillator with ordinary derivatives, which makes the solution procedure simple. The conversion process steps to an equivalent ordinary differential oscillator are displayed. Galerkin’s method is used to derive the jerk frequency. The good match between the analytic and numerical outcomes shows that the equivalent linearized mechanism leads to finding a satisfying and accurate solution. Stability discussion is conducted analytically and numerically.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data availability

In this present manuscript, no data are used.

References

  1. Schot, S.H.: Jerk: the time rate of change of acceleration. Am. J. Phys. 46(11), 1090–1094 (1978). https://doi.org/10.1119/1.11504

    Article  Google Scholar 

  2. El-Nabulsi, R.A.: Free variable mass nonlocal systems, jerks, and snaps, and their implications in rotating fluids in rockets. Acta Mech. 232(1), 89–109 (2021). https://doi.org/10.1007/s00707-020-02843-z

    Article  MathSciNet  MATH  Google Scholar 

  3. Duffy, B.R., Wilson, S.K.: A third-order differential equation arising in thin-film flows and relevant to Tanner’s law. Appl. Math. Lett. 10(3), 63–68 (1997). https://doi.org/10.1016/S0893-9659(97)00036-0

    Article  MathSciNet  MATH  Google Scholar 

  4. Tiryaki, A., Aktaş, M.F.: Oscillation criteria of a certain class of third order nonlinear delay differential equations with damping. J. Math. Anal. Appl. 325(1), 54–68 (2007). https://doi.org/10.1016/j.jmaa.2006.01.001

    Article  MathSciNet  MATH  Google Scholar 

  5. Kenmogne, F., Noubissie, S., Ndombou, G.B., Tebue, E.T., Sonna, A.V., Yemélé, D.: Dynamics of two models of driven extended jerk oscillators: Chaotic pulse generations and application in engineering. Chaos Solitons Fractals 152, 111291 (2021). https://doi.org/10.1016/j.chaos.2021.111291

    Article  MathSciNet  MATH  Google Scholar 

  6. Ainamon, C., Kingni, S.T., Tamba, V.K., Orou, J.B.C., Woafo, P.: Dynamics, circuitry implementation and control of an autonomous Helmholtz Jerk oscillator. J. Control Autom. Electr. Syst. 30(4), 501–511 (2019). https://doi.org/10.1007/s40313-019-00463-0

    Article  Google Scholar 

  7. Srisuchinwong, B., Nopchinda, D.: Current-tunable chaotic jerk oscillator. Electron. Lett. 49(9), 587–589 (2013). https://doi.org/10.1049/el.2013.0029

    Article  Google Scholar 

  8. Mboupda Pone, J.R., Kingni, S.T., Kol, G.R., Pham, V.-T.: Hopf bifurcation, antimonotonicity and amplitude controls in the chaotic Toda jerk oscillator: analysis, circuit realization and combination synchronization in its fractional-order form. Automatika. 60(2), 149–161 (2019). https://doi.org/10.1080/00051144.2019.1600109

    Article  Google Scholar 

  9. Tamba, V.K., Kingni, S.T., Kuiate, G.F., Fotsin, H.B., Talla, P.K.: Coexistence of attractors in autonomous Van der Pol-Duffing jerk oscillator: analysis, chaos control and synchronisation in its fractional-order form. Pramana 91, 12 (2018). https://doi.org/10.1007/s12043-018-1586-1

    Article  Google Scholar 

  10. Mekak-Egong, H.-D., Ramakrishnan, B., Telem, A.N.K., Rajagopal, K., Kengne, J.: Multiscroll in bidirectionally coupled jerk oscillators: theoretical analysis and pspice verification. Int. J. Bifurc. Chaos. 32(14), 2250211 (2022). https://doi.org/10.1142/S021812742250211X

    Article  MathSciNet  Google Scholar 

  11. Ainamon, C., Tamba, V.K., Pone, J.R.M., Kingni, S.T., Malwe, H.B., Orou, J.B.C.: Analysis, circuit realization and controls of an autonomous Morse jerk oscillator. SeMA J. 78, 415–433 (2021). https://doi.org/10.1007/s40324-021-00241-6

    Article  MathSciNet  MATH  Google Scholar 

  12. He, J., El-Dib, Y.O.: The reducing rank method to solve third-order Duffing equation with the homotopy perturbation. Numer. Methods Partial Differ. Equ. 37(2), 1800–1808 (2021). https://doi.org/10.1002/num.22609

    Article  MathSciNet  Google Scholar 

  13. El-Dib, Y.O.: Suppressing the vibration of the third-order critically damped Duffing equation. Int. J. Dyn. Control. 10(4), 1148–1155 (2022). https://doi.org/10.1007/s40435-021-00879-0

    Article  MathSciNet  Google Scholar 

  14. El-Dib, Y.O.: An efficient approach to solving fractional Van der Pol-Duffing jerk oscillator. Commun. Theor. Phys. 74, 105006 (2022). https://doi.org/10.1088/1572-9494/ac80b6

    Article  MathSciNet  MATH  Google Scholar 

  15. El-Dib, Y.O.: The simplest approach to solving the cubic nonlinear jerk oscillator with the non-perturbative method. Math. Methods Appl. Sci. 45(9), 5165–5183 (2022). https://doi.org/10.1002/mma.8099

    Article  MathSciNet  Google Scholar 

  16. El-Dib, Y.O.: Criteria of vibration control in delayed third-order critically damped Duffing oscillation. Arch. Appl. Mech. 92(1), 1–19 (2022). https://doi.org/10.1007/s00419-021-02039-4

    Article  Google Scholar 

  17. El-Dib, Y.O., Elgazery, N.S., Youmna, M.K., Haifa, A.A.: An innovative technique to solve a fractal damping Duffing-jerk oscillator. Commun. Theor. Phys. 75, 9 (2023). https://doi.org/10.1088/1572-9494/acc646

    Article  MathSciNet  MATH  Google Scholar 

  18. van den Berg, J.B., Groothedde, C., Lessard, J.-P.: A general method for computer-assisted proofs of periodic solutions in delay differential problems. J. Dyn. Differ. Equ. 5, 1–44 (2020). https://doi.org/10.1007/s10884-020-09908-6

    Article  MATH  Google Scholar 

  19. Yu, P., Yuan, Y., Xu, J.: Study of double Hopf bifurcation and chaos for an oscillator with time delayed feedback. Commun. Nonlinear Sci. Numer. Simul. 7(1–2), 69–91 (2002). https://doi.org/10.1016/S1007-5704(02)00007-2

    Article  MathSciNet  MATH  Google Scholar 

  20. Rusinek, R., Weremczuk, A., Kecik, K., Warminski, J.: Dynamics of a time delayed Duffing oscillator. Int. J. Non Linear Mech. 65, 98–106 (2014). https://doi.org/10.1016/j.ijnonlinmec.2014.04.012

    Article  Google Scholar 

  21. Cantisán, J., Coccolo, M., Seoane, J.M., Sanjuán, M.A.F.: Delay-induced resonance in the time-delayed duffing oscillator. Int. J. Bifurc. Chaos. 30(3), 2030007 (2020). https://doi.org/10.1142/S0218127420300074

    Article  MathSciNet  MATH  Google Scholar 

  22. Chembo, Y.K., Brunner, D., Jacquot, M., Larger, L.: Optoelectronic oscillators with time-delayed feedback. Rev. Mod. Phys. 91(3), 35006 (2019). https://doi.org/10.1103/RevModPhys.91.035006

    Article  MathSciNet  Google Scholar 

  23. El-Dib, Y.O., Elgazery, N.S., Gad, N.S.: A novel technique to obtain a time-delayed vibration control analytical solution with simulation of He’s formula. J. Low Freq. Noise Vib. Act Control (2023). https://doi.org/10.1177/14613484221149518

    Article  Google Scholar 

  24. Anjum, N., Ain, Q.T., Li, X.X.: Two-scale mathematical model for tsunami wave. GEM Int. J. Geomath. 12(1), 1–12 (2021). https://doi.org/10.1007/s13137-021-00177-z

    Article  MathSciNet  MATH  Google Scholar 

  25. Milici, C., Drăgănescu, G., Machado, J.T.: Introduction to Fractional Differential Equations. Springer, Berlin (2018). https://doi.org/10.1007/978-3-030-00895-6

    Book  MATH  Google Scholar 

  26. Mohammed, P.O., Abdeljawad, T., Hamasalh, F.K.: On Riemann—Liouville and Caputo fractional forward difference monotonicity analysis. Mathematics. 9(11), 1303 (2021). https://doi.org/10.3390/math9111303

    Article  Google Scholar 

  27. Nonnenmacher, T.F., Metzler, R.: On the Riemann-Liouville fractional calculus and some recent applications. Fractals 3(3), 557–566 (1995). https://doi.org/10.1142/S0218348X95000497

    Article  MathSciNet  MATH  Google Scholar 

  28. Owolabi, K.M.: Riemann-Liouville fractional derivative and application to model chaotic differential equations. Prog. Fract. Differ. Appl. 4, 99–110 (2018). https://doi.org/10.18576/pfda/040204

    Article  Google Scholar 

  29. Agarwal, P., Choi, J., Paris, R.B.: Extended Riemann-Liouville fractional derivative operator and its applications. J. Nonlinear Sci. Appl. 8(5), 451–466 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  30. Babakhani, A., Daftardar-Gejji, V.: On calculus of local fractional derivatives. J. Math. Anal. Appl. 270(1), 66–79 (2002). https://doi.org/10.1016/S0022-247X(02)00048-3

    Article  MathSciNet  MATH  Google Scholar 

  31. El-Dib, Y.O.: Immediate solution for fractional nonlinear oscillators using the equivalent linearized method. J. Low Freq. Noise Vib. Act Control. 41(4), 1411–1425 (2022). https://doi.org/10.1177/14613484221098788

    Article  Google Scholar 

  32. Liang, Y.-H., Wang, K.-J.: A new fractal viscoelastic element: promise and applications to Maxwell-Rheological model. Therm. Sci. 25(2), 1221–1227 (2021). https://doi.org/10.2298/TSCI200301015L

    Article  Google Scholar 

  33. El-Dib, Y.O., Elgazery, N.S.: Effect of fractional derivative properties on the periodic solution of the nonlinear oscillations. Fractals 28(7), 1–12 (2020). https://doi.org/10.1142/S0218348X20500954

    Article  MATH  Google Scholar 

  34. Elgazery, N.S.: A periodic solution of the Newell-Whitehead-Segel (NWS) wave equation via fractional calculus. J. Appl. Comput. Mech. 6, 1293–1300 (2020). https://doi.org/10.22055/JACM.2020.33778.2285

    Article  Google Scholar 

  35. He, J.-H., Yang, Q., He, C.-H., Alsolami, A.A.: Pull-down instability of the quadratic nonlinear oscillators. Facta Univ. Ser. Mech. Eng. (2023). https://doi.org/10.22190/FUME230114007H

    Article  Google Scholar 

  36. Noack BR, Schlegel M, Morzynski M, Tadmor G. Galerkin method for nonlinear dynamics. In: Reduced-Order Modelling for Flow Control. Springer, Vienna; 2011:111–149. doi:https://doi.org/10.1007/978-3-7091-0758-4_3

Download references

Acknowledgements

The authors express their gratitude to Princess Nourah bint Abdulrahman University Researchers Supporting Project Number (PNURSP2023R17), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Education, Ain Shams University, Roxy, Cairo, Egypt

    Yusry O. El-Dib & Nasser S. Elgazery

  2. Department of Physics, College of Science, Princess Nourah bint Abdulrahman University, P.O. Box 84428, Riyadh, 11681, Saudi Arabia

    Haifa A. Alyousef

Authors
  1. Yusry O. El-Dib
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nasser S. Elgazery
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Haifa A. Alyousef
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yusry O. El-Dib.

Ethics declarations

Conflict of interest

The authors declare that there are no competing interests related to the publishing of this search.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

El-Dib, Y.O., Elgazery, N.S. & Alyousef, H.A. Galerkin’s method to solve a fractional time-delayed jerk oscillator. Arch Appl Mech 93, 3597–3607 (2023). https://doi.org/10.1007/s00419-023-02455-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-023-02455-8

Keywords

Search

Navigation