Vibration characteristics of rotating orthotropic cantilever plates using analytical approaches: a comprehensive parametric study

Abstract

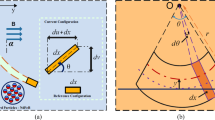
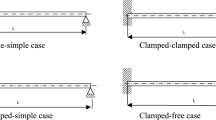
This manuscript is concerned with the free vibration analysis of rotating orthotropic cantilever plates attached with an arbitrary stagger angle to a hub. The general governing equations which include both the centrifugal inertia forces and Coriolis effects are derived using Hamilton’s principle. The results are obtained using extended Kantorovich method and extended Galerkin method which are compared with each other, and available data in the literature and in good agreements are observed. A very detailed study of the influence of varying stiffness ratio, rotation speed, stagger angle, hub radius ratio and aspect ratio on the dynamic characteristics is conducted. These investigations provide complementary results, which leads to improvement in design and appropriate optimization of the material and geometry in this class of problems. The observation of the results shows that the crossing/veering phenomenon is influenced by the stiffness ratio, stagger angle and hub radius ratio. It is found that the centrifugal stiffening rate in the spanwise bending modes is constant, while in the torsion mode is changeable. The plate with the lower stiffness ratio has the higher centrifugal stiffening rate.

Appendices

Appendix A

$$\begin{aligned} G_{i_{Ip} }= & {} \int {\xi _i ^{2}\hbox {d}x} , \quad \qquad \qquad \quad \qquad G_{(i+2)_{Ip} } =-\int {\left( {\frac{\hbox {d}\xi _i }{\hbox {d}x}} \right) ^{2}\hbox {d}x} , \quad \qquad \qquad (i=1,2),\nonumber \\ G_{5_{Ip} }= & {} C\left( {a_{12} +a_{66} } \right) \int \xi _1 \frac{\hbox {d}\xi _2 }{\hbox {d}x}\hbox {d}x -\left. {Ca_{12} \xi _1 \xi _2 } \right| _0^1 , \quad \qquad G_{6_{Ip} } =C\left( {a_{12} +a_{66} } \right) \int {\xi _2 \frac{\hbox {d}\xi _1 }{\hbox {d}x}\hbox {d}x} -\left. {Ca_{66} \xi _1 \xi _2 } \right| _0^1\nonumber \\ G_{7_{Ip} }= & {} \int \xi _{1} \xi _{2} \hbox {d}x , \quad \qquad \qquad \qquad \,\, G_{8_{Ip} } =\int {\xi _1 \xi _3 \hbox {d}x} , \quad \qquad \qquad \qquad G_{9_{Ip} } =\int {\xi _2 \xi _3 \hbox {d}x} ,\nonumber \\ G_{1_{Op} }= & {} \int {\xi _3 ^{2}\hbox {d}x} , \quad \qquad \qquad \qquad \quad G_{2_{Op} } =\int {\xi _3 \frac{\hbox {d}^{2}\xi _3 }{\hbox {d}x^{2}}\hbox {d}x} , \quad \qquad \qquad \,\, G_{3_{Op} } =\int {\xi _3 \frac{\hbox {d}^{4}\xi _3 }{\hbox {d}x^{4}}\hbox {d}x}\nonumber \\ G_{4_{Op} }= & {} \int {\left( {r+x} \right) \xi _3 \frac{\hbox {d}\xi _3 }{\hbox {d}x}\hbox {d}x} , \quad \quad G_{5_{Op} } =\int {\left( \int \left( {r+x} \right) \hbox {d}x\right) \xi _3 \frac{\hbox {d}^{2}\xi _3 }{\hbox {d}x^{2}}\hbox {d}x} \end{aligned}$$

(A.1)

Appendix B

$$\begin{aligned} F_{i_{Ip} }= & {} \int {\psi _i ^{2}\hbox {d}y} , \quad \qquad \qquad \qquad F_{(i+2)_{Ip} } =-\int {\left( {\frac{\hbox {d}\psi _i }{\hbox {d}y}} \right) ^{2}\hbox {d}y} , \quad \qquad \quad (i=1,2),\nonumber \\ F_{5_{Ip} }= & {} C\left( {a_{12} +a_{66} } \right) \int {\psi _1 \frac{\hbox {d}\psi _2 }{\hbox {d}y}\hbox {d}y-} \left. {Ca_{66} \psi _1 \psi _2 } \right| _{-1/2}^{1/2} , \quad \quad \quad F_{6_{Ip} } \nonumber \\= & {} C\left( {a_{12} +a_{66} } \right) \int {\psi _2 \frac{\hbox {d}\psi _1 }{\hbox {d}y}\hbox {d}y-} \left. {Ca_{12} \psi _1 \psi _2 } \right| _{-1/2}^{1/2}\nonumber \\ F_{7_{Ip} }= & {} \int {\psi _1 \psi _2 \hbox {d}y} , \quad \qquad \qquad F_{8_{Ip} } =\int {\psi _1 \psi _3 \hbox {d}y} , \quad \qquad \qquad F_{9_{Ip} } =\int {\psi _2 \psi _3 \hbox {d}y} ,\nonumber \\ F_{1_{Op} }= & {} \int {\psi _3 ^{2}\hbox {d}y} , \quad \qquad \qquad \quad F_{2_{Op} } =\int {\psi _3 \frac{\hbox {d}^{2}\psi _3 }{\hbox {d}y^{2}}\hbox {d}y} , \quad \qquad \,\, F_{3_{Op} } =\int {\psi _3 \frac{\hbox {d}^{4}\psi _3 }{\hbox {d}y^{4}}\hbox {d}y}\nonumber \\ F_{4_{Op} }= & {} \int {y\psi _3 \frac{\hbox {d}\psi _3 }{\hbox {d}y}\hbox {d}y} , \quad \qquad F_{5_{Op} } =\int {\left( \int y\hbox {d}y\right) \psi _3 \frac{\hbox {d}^{2}\psi _3 }{\hbox {d}y^{2}}\hbox {d}y} \end{aligned}$$

(B.1)