Skip to main content
SpringerLink
Log in

General finite element description for non-uniform and discontinuous beam elements

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

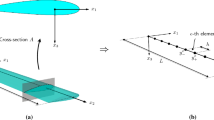
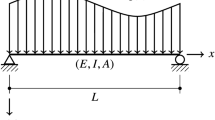
The theory of generalized functions is used to address the static equilibrium problem of Euler–Bernoulli non-uniform and discontinuous 2-D beams. It is shown that if simple integration rules are applied, the full set of response variables due to end nodal displacements and to in-span loads can be derived, in a closed form, for most common beam profiles and arbitrary discontinuity parameters. On this basis, for finite element analysis purposes, a non-uniform and discontinuous beam element is implemented, for which the exact stiffness matrix and the fixed-end load vector are derived. Upon computing the nodal response, no numerical integration is required to build the response variables along the beam element.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Vestroni F., Capecchi D.: Damage detection in beam structures based on frequency measurements. J. Eng. Mech. 126(7), 761–768 (2000)

    Article  Google Scholar 

  2. Kanwal R.P.: Generalized Functions Theory and Applications. Academic Press, New York (1983)

    Google Scholar 

  3. Yavari A., Sarkani S., Moyer E.T.: On applications of generalized functions to beam bending problems. Int. J. Solids Struct. 37, 5675–5705 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  4. Liang R.Y., Choy F., Hu J.: Identification of cracks in beam structure using measurements of natural frequencies. J. Franklin Inst. 324(4), 505–518 (1990)

    Google Scholar 

  5. Narkis Y.: Identification of crack location in vibrating simply supported beams. J. Sound Vib. 172(4), 549–558 (1994)

    Article  MATH  Google Scholar 

  6. Banerjee J.R., Williams F.W.: Exact Bernoulli-Euler static stiffness matrix for a range of tapered beam-columns. Int. J. Numer. Methods Eng. 23, 1615–1628 (1986)

    Article  MATH  Google Scholar 

  7. Arbabi F., Li F.: Macroelements for variable-section beams. Comput. Struct. 37(4), 553–559 (1990)

    Article  Google Scholar 

  8. Eisenberger M.: Exact solution for general variable cross-section members. Comput. Struct. 41(4), 765–772 (1991)

    Article  MATH  Google Scholar 

  9. Al-Gahtani H.J.: Exact stiffnesses for tapered members. J. Struct. Eng. 122(10), 1234–1239 (1996)

    Article  Google Scholar 

  10. Krenk S.: A general format for curved and non-homogeneous beam elements. Comput. Struct. 50(4), 449–454 (1994)

    Article  MATH  Google Scholar 

  11. Tena-Colunga A.: Stiffness formulation for nonprismatic beam elements. J. Struct. Eng. 122(12), 1484–1489 (1996)

    Article  Google Scholar 

  12. Friedman Z., Kosmatka J.B.: Exact stiffness matrix of a nonuniform beam—I. Extension, torsion, and bending of a Bernoulli-Euler beam. Comput. Struct. 42(5), 671–682 (1992)

    Article  MATH  Google Scholar 

  13. Ozay G., Topcu A.: Analysis of frames with non-prismatic members. Can. J. Civil Eng. 27, 17–25 (2000)

    Article  Google Scholar 

  14. Luo Y., Wu F., Xu X.: Element stiffness matrix and modified coefficients for circular tube with tapered ends. J. Construct. Steel Res. 62, 856–862 (2006)

    Article  Google Scholar 

  15. Luo Y., Xu X., Wu F.: Accurate stiffness matrix for nonprismatic members. J. Struct. Eng. 133(8), 1168–1175 (2007)

    Article  Google Scholar 

  16. Gimena F.N., Gonzaga P., Gimena L.: Stiffness and transfer matrices of a non-naturally curved 3D-beam element. Eng. Struct. 30, 1770–1781 (2008)

    Article  Google Scholar 

  17. Macaulay W.H.: Note on the deflection of beams. Messenger Math. 48, 129–130 (1919)

    Google Scholar 

  18. Brungraber R.J.: Singularity functions in the solution of beam-deflection problems. J. Eng. Educ. (Mech. Div. Bull.) 1(55(9), 278–280 (1965)

    Google Scholar 

  19. Falsone G.: The use of generalized functions in the discontinuous beam bending differential equation. Int. J. Eng. Educ. 18(3), 337–343 (2002)

    Google Scholar 

  20. Failla G., Santini A.: On Euler-Bernoulli discontinuous beam solutions via uniform-beam Green’s functions. Int. J. Solids Struct. 44(22-23), 7666–7687 (2007)

    Article  MATH  Google Scholar 

  21. Failla, G.: Closed-form solutions for Euler–Bernoulli arbitrary discontinuous beams. Arch. Appl. Mech., doi:10.1007/s00419-010-0434-7, (2010)

  22. Biondi B., Caddemi S.: Euler-Bernoulli beams with multiple singularities in the flexural stiffness. Eur. J. Mech. A/Solids 26(5), 789–809 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  23. Buda G., Caddemi S.: Identification of concentrated damages in Euler-Bernoulli beams under static loads. J. Eng. Mech. 133(8), 942–956 (2007)

    Article  Google Scholar 

  24. Di Paola M., Bilello C.: An integral equation for damage identification of Euler-Bernoulli beams under static loads. J. Eng. Mech. 130(2), 225–234 (2004)

    Article  Google Scholar 

  25. Eisenberger M.: Stiffness matrices for non-prismatic members including transverse shear. Comput. Struct. 40(4), 831–835 (1991)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Meccanica e Materiali (MECMAT), Università “Mediterranea” di Reggio Calabria, Via Graziella, Località Feo di Vito, 89122, Reggio Calabria, Italy

    Giuseppe Failla

  2. Dipartimento di Architettura (DARC), Università di Catania, P.zza Federico di Svevia, 96100, Siracusa, Italy

    Nicola Impollonia

Authors
  1. Giuseppe Failla
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nicola Impollonia
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Giuseppe Failla.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Failla, G., Impollonia, N. General finite element description for non-uniform and discontinuous beam elements. Arch Appl Mech 82, 43–67 (2012). https://doi.org/10.1007/s00419-011-0538-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-011-0538-8

Keywords

Search

Navigation