Skip to main content
SpringerLink
Log in

The transient response to an equatorial heat source and its convergence to steady state: implications for MJO theory

  • Published:
Climate Dynamics Aims and scope Submit manuscript

Abstract

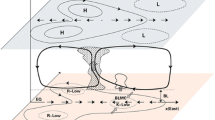
The Matsuno–Gill model for the atmospheric steady-state response to an imposed equatorial heat source is perhaps the most successful set of reduced equations in the theory of tropical dynamics. While it was originally designed to explain some key features of the tropical climatology such as the Walker and Hadley circulations, it is increasingly used for other transient tropical disturbances such as the Madden–Julian oscillation (MJO). Here, we provide a semi-analytic solution to the time dependent Matsuno–Gill equations, with periodic boundary conditions and without the long-wave approximation, based on a meridional expansion using the parabolic cylinder functions combined with the method of characteristics and Fourier series in the zonal direction. Our method of solution leads to an ODE system which is solved numerically. We use this transient solution to investigate the conditions under which the transient response convergences to the steady state and study the effect of a moving heat source on the structure of the response and speculate about its resemblance or not to the MJO structure. In particular, we look at the sensitivity of the long time behavior of the solution with respect to the model parameters, namely, the imposed dissipation rate and the speed of the moving heat source as well as the form of the heating itself.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  • Adames Ángel F, Kim Daehyun (2016) The MJO as a dispersive, convectively coupled moisture wave: theory and observations. J Atmos Sci 73(3):913–941

    Article  Google Scholar 

  • Bao M, Hartmann DL (2014) The response to mjo-like forcing in a nonlinear shallow water model. Geophys Res Lett 41:1322–1328

    Article  Google Scholar 

  • Biello J, Majda A (2004) Boundary layer dissipation and the nonlinear interaction of equatorial baroclinic barotropic rossby waves. Geophys Astrophys Fluid Dyn 98:85–127

    Article  Google Scholar 

  • Biello JA, Majda AJ (2010) Intraseasonal multi-scale moist dynamics of the tropical troposphere. Commun Math Sci 8:519–540

    Article  Google Scholar 

  • Chao WC (1987) On the origin of the tropical intraseasonal oscillation. J Atmos Sci 44:1940–1949

    Article  Google Scholar 

  • Dai A (2001) Global precipitation and thunderstorm frequencies. part ii: Diurnal variations. J Clim 14:1112–1128

    Article  Google Scholar 

  • Gill AE, Phlips PJ (1986) Nonlinear effects on heat-induced circulation of the tropical atmosphere. Q J R Meteorol Soc 110:69–91

    Article  Google Scholar 

  • Gill AE (1982) Atmosphere–Ocean dynamics. volume 30 of International Geophysics Series. Academic Press

  • Gill AE (1980) Some simple solutions for heat-induced tropical circulation. Q J R Meteorol Soc 106(449):447–462

    Article  Google Scholar 

  • Heckley WA, Gill AE (1984) Some simple analytical solutions to the problem of forced equatorial long waves. Q J R Meteorol Soc 110:203–2017

    Article  Google Scholar 

  • Hendon HH, Liebmann B (1994) Organization of convection within the Madden–Julian oscillation. J Geophys Res 99:8073–8084

    Article  Google Scholar 

  • Hendon HH, Salby ML (1999) The life cycle of the Madden–Julian oscillation. J Atmos Sci 51:2225–2237

    Article  Google Scholar 

  • Hung MP, Lin JL, Wang W, Kim D, Shinoda T, Weaver SJ (2013) MJO and convectively coupled equatorial waves simulated by CMIP5 climate models. J Clim 26:6185–6214

    Article  Google Scholar 

  • Jiang X, Waliser DE, Xavier PK, Petch J, Klingaman NP, Woolnough SJ, Guan B, Bellon G, Crueger T, DeMott C, Hannay C, Lin H, Wenting H, Kim D, Lappen CL, Mong-Ming L, Ma HY, Miyakawa T, Ridout JA, Schubert SD, Scinocca J, Seo KH, Shindo E, Song X, Stan C, Tseng WL, Wang W, Tongwen W, Xiaoqing W, Wyser K, Zhang GJ, Zhu H (2015) Vertical structure and physical processes of the Madden–Julian oscillation: exploring key model physics in climate simulations. J Geophys Res Atmos 120(10):4718–4748

    Article  Google Scholar 

  • Johnson RH, Rickenbach TM, Rutledge SA, Ciesielski PE, Schubert WH (1999) Trimodal characteristics of tropical convection. J Clim 12(8):2397–2418

    Article  Google Scholar 

  • Khouider B, Majda AJ (2006) A simple multicloud parameterization for convectively coupled tropical waves. part i: Linear analysis. J Atmos Sci 63:1308–1323

    Article  Google Scholar 

  • Khouider B, Majda AJ (2007) A simple multicloud parameterization for convectively coupled tropical waves. part ii: Nonlinear simulations. J Atmos Sci 64:381–400

    Article  Google Scholar 

  • Khouider B, Majda AJ, Stechmann SN (2013) Climate science in the tropics: waves, vortices and PDEs. Nonlinearity 26(1):R1

    Article  Google Scholar 

  • Kikuchi K, Wang B (2008) The diurnal cycle in the tropics. J Clim 21:26802696

    Article  Google Scholar 

  • Kiladis GN, Straub K, Haertel P (2005) Zonal and vertical structure of the Madden–Julian oscillation. J Atmos Sci 62:2790–2809

    Article  Google Scholar 

  • Lee SK, Wang C, Mapes BE (2009) A simple atmospheric model of the local and teleconnection responses to tropical heating anomalies. J Clim 22(2):272–284

    Article  Google Scholar 

  • Lin JL, Kiladis GN, Mapes BE, Weickmann KM, Sperber KR, Lin W, Wheeler MC, Schubert SD, Del Genio A, Donner LJ, Emori S, Gueremy JF, Hourdin F, Rasch PJ, Roeckner E, Scinocca JF (2006) Tropical intraseasonal variability in 14 IPCC AR4 climate models. Part I: Convective signals. J Clim 19(12):2665–2690

    Article  Google Scholar 

  • Lin JL, Mapes BE, Han W (2008) What are the sources of mechanical damping in Matsuno–Gill-type models? J Clim 21(2):165–179

    Article  Google Scholar 

  • Lin JL, Zhang M, Mapes B (2005) Zonal momentum budget of the Madden–Julian oscillation: the source and strength of equivalent linear damping. J Atmos Sci 62(7 I):2172–2188, 7

  • Madden R, Julian PR (1972) Description of global-scale circulation cells in the tropics with a \(40-50\)-day period. J Atmos Sci 29:1109–1123

    Article  Google Scholar 

  • Majda AJ (2003) Introduction to PDEs and Waves for the Atmosphere and Ocean, vol 9. Courant Lecture Notes in Mathematics. American Mathematical Society, Providence

  • Majda AJ, Biello JA (2004) A multiscale model for the intraseasonal oscillation. Proc Natl Acad Sci USA 101(14):4736–4741

    Article  Google Scholar 

  • Majda AJ, Stechmann SN (2009) The skeleton of tropical intraseasonal oscillations. Proc Natl Acad Sci USA 106:8417–8422

    Article  Google Scholar 

  • Majda AJ, Khouider B (2001) A numerical strategy for efficient modeling of the equatorial wave guide. Proc Natl Acad Sci USA 98(4):1341–1346

    Article  Google Scholar 

  • Mapes B, Tulich S, Lin J, Zuidema P (2006) The mesoscale convection life cycle: building block or prototype for large-scale tropical waves? Dyn Atmos Oceans 42(1–4):3–29

    Article  Google Scholar 

  • Matsuno T (1966) Quasi-geostrophic motions in the equatorial area. J Met Jap 44:25–41

    Google Scholar 

  • Monteiro JM, Adames ÁF, Wallace JM, Sukhatme JS (2014) Interpreting the upper level structure of the Madden–Julian oscillation. Geophys Res Lett 41:9158–9165

    Article  Google Scholar 

  • Pedlosky J (1987) Geophysical fluid dynamics, 2nd edn. Springer, Berlin

  • Phlips PJ, Gill AE (1987) An analytic model of the heat-induced tropical circulation in the presence of a mean wind. Q J R Meteorol Soc 113:213–236

    Article  Google Scholar 

  • Raupp Carlos FM, Pedro L (2009) Silva Dias. Resonant wave interactions in the presence of a diurnally varying heat source. J Atmos Sci 66:3165–3183

    Article  Google Scholar 

  • Raymond DJ, Zeng X (2000) Instability and large-scale circulations in a two-column model of the tropical troposphere. Q J R Meteorol Soc 126(570):3117–3135

    Article  Google Scholar 

  • Raymond DJ (2001) A new model of the Madden–Julian oscillation. J Atmos Sci 58(18):2807–2819

    Article  Google Scholar 

  • Romps DM (2012) On the equivalence of two schemes for convective momentum transport. J Atmos Sci 69(12):3491–3500

    Article  Google Scholar 

  • Romps DM (2014) Rayleigh damping in the free troposphere. J Atmos Sci 71(2):553–565

    Article  Google Scholar 

  • Sobel A, Maloney E (2012) An idealized semi-empirical framework for modeling the Madden–Julian oscillation. J Atmos Sci 69:1691–1705

    Article  Google Scholar 

  • Thual S, Majda AJ (2015) A suite of skeleton models for the mjo with refined vertical structure. Math Clim Weather Forecast 1(1)

  • Thual S, Majda AJ (2016) A skeleton model for the mjo with refined vertical structure. Clim Dyn 46(9):2773–2786

    Article  Google Scholar 

  • Tulich SN, Kiladis GN (2012) Squall lines and convectively coupled gravity waves in the tropics: why do most cloud systems propagate westward? J Atmos Sci 69:29953012

    Article  Google Scholar 

  • Wang B (1988) Dynamics of tropical low-frequency waves: an analysis of the moist kelvin wave. J Atmos Sci 45(14):2051–2065

    Article  Google Scholar 

  • Wang B (2005) Theory. In: Lau WKM, Waliser DE (eds) Intraseasonal variability in the atmosphere-ocean climate system, chapter 10. Praxis Springer, Berlin, pp 307–360

  • Wu Z, Sarachik E, Battisti D (2001) Thermally driven tropical circulations under rayleigh friction and newtonian cooling: Analytic solutions. J Atmos Sci 58:724–741

  • Zhaohua W, Battisti DS, Sarachik ES (2000) Rayleigh friction, newtonian cooling, and the linear response to steady tropical heating. J Atmos Sci 57(12):1937–1957

    Article  Google Scholar 

  • Yang G-Y, Slingo J (2001) The diurnal cycle in the tropics. Month Weather Rev 129:784–801

    Article  Google Scholar 

  • Zhang C (2005) Madden–Julian oscillation. Rev Geophys 43:RG2003

Download references

Acknowledgements

The research of B.K is supported in part by a Natural Sciences and Engineering Research Council of Canada Discovery grant. Parts of this work was realized when A.K. visited the university of Victoria on a government of Algeria research travel grant.

Author information

Authors and Affiliations

  1. Department of Mathematics, Laboratory AMNEDP University of Sciences and Technology Houari Boumedienne, Po Box 32, El-Alia, Bab Ezzouar, 16079, Algiers, Algeria

    Abderrahim Kacimi

  2. Department of Mathematics and Statistics, University of Victoria, Po Box 3060, STN CSC, Victoria, BC, Canada

    Boualem Khouider

Authors
  1. Abderrahim Kacimi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Boualem Khouider
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Boualem Khouider.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kacimi, A., Khouider, B. The transient response to an equatorial heat source and its convergence to steady state: implications for MJO theory. Clim Dyn 50, 3315–3330 (2018). https://doi.org/10.1007/s00382-017-3807-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00382-017-3807-6

Keywords

Search

Navigation