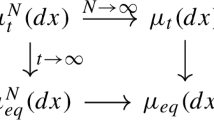Abstract
We study traveling wave solutions for a reaction–diffusion model, introduced in the article Calvez et al. (Regime switching on the propagation speed of travelling waves of some size-structured myxobacteriapopulation models, 2023), describing the spread of the social bacterium Myxococcus xanthus. This model describes the spatial dynamics of two different cluster sizes: isolated bacteria and paired bacteria. Two isolated bacteria can coagulate to form a cluster of two bacteria and conversely, a pair of bacteria can fragment into two isolated bacteria. Coagulation and fragmentation are assumed to occur at a certain rate denoted by k. In this article we study theoretically the limit of fast coagulation fragmentation corresponding mathematically to the limit when the value of the parameter k tends to \(+ \infty \). For this regime, we demonstrate the existence and uniqueness of a transition between pulled and pushed fronts for a certain critical ratio \(\theta ^\star \) between the diffusion coefficient of isolated bacteria and the diffusion coefficient of paired bacteria. When the ratio is below \(\theta ^\star \), the critical front speed is constant and corresponds to the linear speed. Conversely, when the ratio is above the critical threshold, the critical spreading speed becomes strictly greater than the linear speed.




Similar content being viewed by others
References
Adimy M, Chekroun A, Kazmierczak B (2017) Traveling waves in a coupled reaction–diffusion and difference model of hematopoiesis. J Differ Equ 262(7):4085–4128
Adimy M, Chekroun A, Kazmierczak B (2022) Traveling waves for reaction–diffusion pde coupled to difference equation with nonlocal dispersal term and time delay. Math Model Nat Phenom 17:17
Aizenman M, Bak TA (1979) Convergence to equilibrium in a system of reacting polymers. Commun Math Phys 65(3):203–230
Alfaro M, Coville J, Raoul G (2013) Travelling waves in a nonlocal reaction–diffusion equation as a model for a population structured by a space variable and a phenotypic trait. Commun Partial Differ Equ 38(12):2126–2154
An J, Henderson C, Ryzhik L (2021) Pushed, pulled and Pushmi-Pullyu fronts of the Burgers–FKPP equation. arXiv preprint arXiv:2108.07861
Arias M, Campos J, Robles-Pérez A et al (2004) Fast and heteroclinic solutions for a second order ode related to Fisher–Kolmogorov’s equation. Calc Var Partial Differ Equ 21(3):319–334
Aronson DG, Weinberger HF (1978) Multidimensional nonlinear diffusion arising in population genetics. Adv Math 30(1):33–76
Avery M, Garénaux L (2023) Spectral stability of the critical front in the extended Fisher–KPP equation. Z Angew Math Phys 74(2):71
Avery M, Scheel A (2022) Universal selection of pulled fronts. Commun Am Math Soc 2(05):172–231
Avery M, Holzer M, Scheel A (2023a) Pushed and pulled fronts in a logistic Keller–Segel model with chemorepulsion. arXiv preprint arXiv:2308.01754
Avery M, Holzer M, Scheel A (2023) Pushed-to-pulled front transitions: continuation, speed scalings, and hidden monotonicty. J Nonlinear Sci 33(6):102
Avery SV (2006) Microbial cell individuality and the underlying sources of heterogeneity. Nat Rev Microbiol 4(8):577–587
Banerjee M, Vougalter V, Volpert V (2017) Doubly nonlocal reaction–diffusion equations and the emergence of species. Appl Math Model 42:591–599
Benguria R, Depassier M (1996) Speed of fronts of the reaction–diffusion equation. Phys Rev Lett 77(6):1171
Benguria R, Depassier M (1996) Variational characterization of the speed of propagation of fronts for the nonlinear diffusion equation. Commun Math Phys 175:221–227
Billingham J, Needham D (1991) The development of travelling waves in quadratic and cubic autocatalysis with unequal diffusion rates. i. Permanent form travelling waves. Philos Trans R Soc Lond Ser A: Phys Eng Sci 334(1633):1–24
Bloch H, Calvez V, Gaudeul B, Gouarin L, Lefebvre-Lepot A, Mignot T, Romanos M, Saulnier J-B (2023) A new modeling approach of myxococcus xanthus bacteria using polarity-based reversals. https://hal.science/hal-04102694 (Preprint)
Bouin E, Calvez V (2014) Travelling waves for the cane toads equation with bounded traits. Nonlinearity 27(9):2233
Calvez V, El Abdouni A, Estavoyer M, Madrid I, Olivier J, Tournus M (2024) Regime switching on the propagation speed of travelling waves of some size-structured Myxobacteria population models. https://hal.science/hal-04532644 (Preprint)
Carrillo JA, Desvillettes L, Fellner K (2009) Rigorous derivation of a nonlinear diffusion equation as fast-reaction limit of a continuous coagulation–fragmentation model with diffusion. Commun Partial Differ Equ 34(11):1338–1351
Desvillettes L, Fellner K (2010) Large time asymptotics for a continuous coagulation-fragmentation model with degenerate size-dependent diffusion. SIAM J Math Anal 41(6):2315–2334
Ducrot A, Magal P (2009) Travelling wave solutions for an infection-age structured model with diffusion. Proc R Soc Edinb Sect A Math 139(3):459–482
Elliott EC, Cornell SJ (2012) Dispersal polymorphism and the speed of biological invasions. PLoS ONE 7(7):e40496
Engler H (1985) Relations between travelling wave solutions of quasilinear parabolic equations. Proc Am Math Soc 93(2):297–302
Faye G, Peltier G (2018) Anomalous invasion speed in a system of coupled reaction–diffusion equations. Commun Math Sci 16(2):441–461
Fisher RA (1937) The wave of advance of advantageous genes. Ann Eugen 7(4):355–369
Focant S, Gallay T (1998) Existence and stability of propagating fronts for an autocatalytic reaction–diffusion system. Physica D 120(3–4):346–368
Griette Q (2019) Singular measure traveling waves in an epidemiological model with continuous phenotypes. Trans Am Math Soc 371(6):4411–4458
Hadeler K (1983) Free boundary problems in biological models. Free Bound Probl Theory Appl 2:664–671
Hadeler KP, Rothe F (1975) Travelling fronts in nonlinear diffusion equations. J Math Biol 2(3):251–263
Hodgkin J, Kaiser D (1979) Genetics of gliding motility in Myxococcus xanthus (myxobacterales): two gene systems control movement. Mol Gen Genet MGG 171(2):177–191
Holzer M (2014) Anomalous spreading in a system of coupled Fisher–KPP equations. Physica D 270:1–10
Holzer M (2014b) A proof of anomalous invasion speeds in a system of coupled Fisher–KPP equations. arXiv preprint arXiv:1409.8641
Holzer M, Scheel A (2012) A slow pushed front in a Lotka–Volterra competition model. Nonlinearity 25(7):2151
Holzer M, Scheel A (2014) Criteria for pointwise growth and their role in invasion processes. J Nonlinear Sci 24:661–709
Kolmogorov A (1937) Étude de l’équation de la diffusion avec croissance de la quantité de matière et son application à un problème biologique. Moscow Univ Bull Math 1:1–25
Lewis MA, Li B, Weinberger HF (2002) Spreading speed and linear determinacy for two-species competition models. J Math Biol 45:219–233
Marcelli C, Papalini F (2018) A new estimate on the minimal wave speed for travelling fronts in reaction–diffusion–convection equations. Electron J Qual Theory Differ Equ 10:1–13
Panigrahi S, Murat D, Le Gall A et al (2021) Misic, a general deep learning-based method for the high-throughput cell segmentation of complex bacterial communities. Elife 10:e65151
Rombouts S (2021) Advanced microscopies for the study of motility behavior in predating Myxococcus xanthus. PhD thesis, Université Montpellier
Rombouts S, Mas A, Le Gall A et al (2022) Multi-scale dynamic imaging reveals that cooperative motility behaviors promote efficient predation in bacteria. bioRxiv pp 2022–12
Shapiro JA (1998) Thinking about bacterial populations as multicellular organisms. Annu Rev Microbiol 52(1):81–104
Van Saarloos W (2003) Front propagation into unstable states. Phys Rep 386(2–6):29–222
Volpert AI, Volpert VA, Volpert VA (1994) Traveling wave solutions of parabolic systems, vol 140. American Mathematical Society
Volpert V (2014) Elliptic partial differential equations, vol 2. Springer, Berlin
Weinberger HF, Lewis MA, Li B (2007) Anomalous spreading speeds of cooperative recursion systems. J Math Biol 55:207–222
Acknowledgements
The authors were funded by the ANR via the project PLUME under Grant Agreement ANR-21-CE13-0040. The authors would like to thank the supervisors and students of the previous CEMRACS project: Vincent Calvez, Adil El Abdouni, Florence Hubert, Ignacio Madrid, Julien Olivier, Magali Tournus. This CEMRACS project has received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (Grant Agreement No. 865711).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Estavoyer, M., Lepoutre, T. Travelling waves for a fast reaction limit of a discrete coagulation–fragmentation model with diffusion and proliferation. J. Math. Biol. 89, 2 (2024). https://doi.org/10.1007/s00285-024-02099-4
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00285-024-02099-4