Multiple invasion speeds in a two-species integro-difference competition model

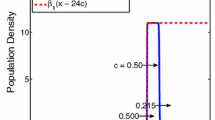
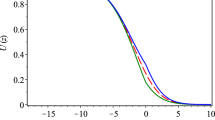
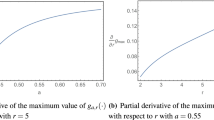
Abstract

We study an integro-difference competition model for the case that two species consecutively invade a habitat. We show that if a species spreads into a traveling wave of its rival, or if two species expand their spatial ranges in both directions, in a direction where open space is available, the species with larger invasion speed can always establish a wave moving into open space with its own speed. We demonstrate that when one species is stronger in competition, under appropriate conditions, the speeds at which the boundaries between two species move can be analytically determined. We find that in general there are multiple invasion speeds in the model. It is possible for a species to develop two separate waves propagating with different invasion speeds. It is also possible for each species to establish a single wave spreading with distinct speeds in both directions. The mathematical analysis relies on linear determinacy and new techniques developed.

Appendix

In this section we provide the proofs for the theorems given in Sects. 3 and 4. We need some lemmas regarding the bounds of invasion speeds in both directions.

Throughout this section, we define $(u_1(x), u_2(x))\ge (v_1(x), v_2(x))$ to mean that $u_i(x) \ge v_i(x)$ for $i=1,2$ and x, and $(u_1(x), u_2(x))> (v_1(x), v_2(x))$ to mean that $u_i(x) \ge v_i(x)$ for $i=1,2$ and x where one inequality is strict.

1.1 Bounds of invasion speeds

For the sake of simplicity, in general we use $u^0(x)$ (or $v^0(x)$) to denote the actual initial value of species u (or v). For example, in ($\text{ IV }_2$), if $u_{-\ell }(x)$ is given, we say $u^0(x)=u_{-\ell }(x)$, and if $\ell =0$ we say $(u^0(x), v^0(x))=(u_0(x), v_0(x))$.

We note from the first equation of (2.1) that

$$\begin{aligned} {\displaystyle u_{n+1}(x) \le \int _{-\infty }^{\infty }k_1(x-y) \frac{(1 + \rho _1)u_n(y)}{ 1 + \rho _1u_n( y)}dy}. \end{aligned}$$

This shows that $c^*_1$ and $c^*_{1-}$ are upper bounds of the rightward and leftward spreading speeds of species u, respectively. That is, if $u^0(x)$ is zero for sufficiently large x, then for any positive ${\varepsilon }$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\ge n (c^*_1+\varepsilon )} u_n(x) \right] =0}, \end{aligned}$$

(6.1)

and if $u^0(x)$ is zero for sufficiently negative x, then for any positive ${\varepsilon }$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\le -n (c^*_{1-}+\varepsilon )} u_n(x) \right] =0.} \end{aligned}$$

(6.2)

Similarly, if $v^0(x)$ is zero for sufficiently large x, then for any positive ${\varepsilon }$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\ge n (c^*_2+\varepsilon )} v_n(x) \right] =0}, \end{aligned}$$

(6.3)

and if $v^0(x)$ is zero for sufficiently negative x, then for any positive ${\varepsilon }$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\le -n (c^*_{2-}+\varepsilon )} v_n(x) \right] =0.} \end{aligned}$$

(6.4)

Consider the smooth nonincreasing function $\zeta$ with the property

$$\begin{aligned} \zeta (s)= \left\{ \begin{array}{ll} 1, &{} ~ \mathrm{if} ~ s\le 1/2,\\ 0, &{} ~ \mathrm{if} ~ s \ge 1. \end{array} \right. \end{aligned}$$

For $i=1,2$ and $b>0$, let

$$\begin{aligned} k_{ib}(x)=\zeta \left( \frac{|x|}{b}\right) k_i(x) \end{aligned}$$

which has bounded support. Let

$$\begin{aligned} {\bar{k}}_{ib}(\mu )= \int _{-\infty }^{\infty }k_{ib}(x)e^{\mu x}dx. \end{aligned}$$

${k}_{ib}$ and ${\bar{k}}_{ib}$ are needed in the proofs of the lemmas in this section.

Lemma 6.1

The follow statements hold.

• i. Let $c^*_1> c_0$. Assume that $0\le u^0(x)\le 1$ and $u^0(x)\not \equiv 0$, $0\le v^0(x)\le 1$, $v^0(\infty )= 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\ge nc_0}v_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c_0, c^*_{1-}\}-\varepsilon )\le x\le n(c^*_1-\varepsilon )} |1-u_n (x)|\right] =0.} \end{aligned}$$

• ii. Let $c^*_{1-}> c_0$. Assume that $0\le u^0(x)\le 1$ and $u^0(x)\not \equiv 0$, $0\le v^0(x)\le 1$, $v^0(-\infty )= 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\le -nc_0}v_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(c^*_{1-}-{\varepsilon })\le x\le n(\min \{-c_0, c^*_1\}-{\varepsilon })} |1-u_n (x)|\right] =0.} \end{aligned}$$

• iii. Let $c^*_2> c_0$. Assume that $0\le u^0(x)\le 1$ and $u^0(\infty )= 0$, $0\le v^0(x)\le 1$, $v^0(x)\not \equiv 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\ge nc_0}u_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c_0, c^*_{2-}\}-\varepsilon )\le x\le n(c^*_2-\varepsilon )} |1-v_n (x)|\right] =0.} \end{aligned}$$

• iv. Let $c^*_{2-}> c_0$. Assume that $0\le u^0(x)\le 1$ and $u^0(-\infty ) = 0$, $0\le v^0(x)\le 1$, $v^0(x) \not \equiv 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\le -nc_0}u_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(c^*_{2-}-{\varepsilon })\le x\le n(\min \{-c_0, c^*_2\}-{\varepsilon })} |1-v_n (x)|\right] =0.} \end{aligned}$$

• v. Let $c\ge c^*_1>c_0$. Assume that $u_n(x)=w(x-nc)$ is a nonincreasing traveling wave solution of the u equation in (2.1) in the absence of v with $w(-\infty )=1$ and $w(\infty )=0$. Assume also that $u_0(x)=w(x)$, $0\le v_0(x)\le 1$ and $v_0(\infty )= 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\ge nc_0}v_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c_0, c^*_{1-}\}-\varepsilon )\le x\le n(c-\varepsilon )} |1-u_n (x)|\right] =0.} \end{aligned}$$

• vi. Let $c^*_{1-}>c_0$. Assume that $u_n(x)=w(x-nc)$ is a nonincreasing traveling wave solution of the u equation in (2.1) in the absence of v with $c\ge c^*_1$, $w(-\infty )=1$ and $w(\infty )=0$. Assume also that $u_0(x)=w(x)$, $0\le v_0(x)\le 1$, $v_0(-\infty )= 0$. If $\lim _{n{\rightarrow }\infty }\sup _{x\le -nc_0}v_n(x)=0$, then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\le n(\min \{ -c_0, c^*_{1} \}-{\varepsilon })} |1-u_n (x)|\right] =0.} \end{aligned}$$

Proof

We first prove the statement (i). Since $\lim _{n{\rightarrow }\infty }\sup _{x\ge nc_0}v_n(x)=0$, for any small positive $\eta$, there exists a positive $n_0$ such that for $n> n_0$ and $x\ge nc_0$, $v_n(x)\le \eta .$ On the other hand, since $v^0(\infty )= 0$, there exists $x_0>0$ such that for $n\le n_0$ and $x\ge x_0$, $v_n(x)\le \eta .$ Define

$$\begin{aligned} \delta (x)= \left\{ \begin{array}{ll} \rho _1\alpha _1\eta , &{} ~ \mathrm{if} ~ x\ge 0,\\ \rho _1\alpha _1, &{} ~ \mathrm{if} ~ x< 0. \end{array} \right. \end{aligned}$$

$\delta (x)$ is a positive and nonincreasing function with $\delta (+\infty )=\rho _1\alpha _1\eta < \rho _1\alpha _1=\delta (-\infty )$. Let $x_1=\max \{0, x_0-ic_0, i\le n_0 \}$. It is easily seen $\rho _1\alpha _1v_n(x)\le \delta (x-x_1-nc)$ for all n and $x\in {{\mathbb {R}}}$. We therefore have that for any $b>0$, all n, and $x\in {{\mathbb {R}}}$,

$$\begin{aligned} {\displaystyle u_{n+1}(x) \ge \int _{-\infty }^{\infty }k_{1b}(x-y) \frac{(1+\rho _1)u_n(y)}{1+\rho _1 u_n(y)+\delta (y-x_1-nc_0)}dy}. \end{aligned}$$

(6.5)

The corresponding integro-difference equation is

$$\begin{aligned} {\displaystyle N_{n+1}(x) = \int _{-\infty }^{\infty }k_{1b}(x-y) \frac{(1+\rho _1)N_n(y)}{1+\rho _1 N_n(y)+\delta (y-x_1-nc_0)}dy}. \end{aligned}$$

(6.6)

For any small ${\varepsilon }>0$, we can choose b sufficiently large and $\eta$ sufficiently small such that

$$\begin{aligned} \inf _{\mu>0}\frac{\ln [(1+\rho _1){\bar{k}}_{1b}(\mu )/(1+\rho _1\alpha _1\eta )]}{\mu }> & {} c^*_1-\frac{{\varepsilon }}{2},\\ \inf _{\mu>0}\frac{\ln [(1+\rho _1){\bar{k}}_{1b}(-\mu )/(1+\rho _1\alpha _1\eta )]}{\mu }> & {} c^*_{1-}-\frac{{\varepsilon }}{2}, \end{aligned}$$

and

$$\begin{aligned} N^*:=\frac{(1+\rho _1)\int _{-\infty }^{\infty }k_{1b}(x)dx -1-\rho _1\alpha _1\eta }{\rho _1}>1-2\alpha _1 \eta . \end{aligned}$$

Note that $N^*$ is the positive equilibrium of (6.6) if $\delta (x-x_1-nc_0)$ is replaced by $\rho _1\alpha _1\eta$.

The Eq. (6.6) is a special case of the model (1) studied in Li et al. (2016). Choose the initial value for (6.6) to be $u^0(x)$. A direct application of Theorem 2 (i) (b) in Li et al. (2016) show that for any small positive ${\varepsilon }$ independent on $\eta$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c_0, c^*_{1-}\}-\varepsilon )\le x\le n(c^*_1-\varepsilon )} |N^*-N_n (x)|\right] =0.} \end{aligned}$$

Since $u_n(x)\ge N_n(x)$ for all n and $x\in {{\mathbb {R}}}$ and since $N^*>1-2\alpha _1\eta$, $\eta$ is arbitrary and $u_n(x)\le 1$ for all n and $x\in {{\mathbb {R}}}$, the statement (i) of the lemma is true.

The proofs of statements (ii)–(iv) are similar to that of statement (i), and are omitted.

The statement (v) follows from the statement (i) if $c=c^*_1$. To prove this statement for the case of $c>c^*_1$, we need some useful lower solutions. For any small positive ${\varepsilon }$, we can choose $\eta$ sufficiently small and b suffidently large such that

$$\begin{aligned} {\tilde{c}}:=\frac{1}{\mu _c}\ln \left[ \frac{1+\rho _1}{1+\rho _1\alpha _1 \eta }{\bar{k}}_{1b}(\mu _c) \right] \ge c-{\varepsilon }/2>c_0. \end{aligned}$$

Note $c>{\tilde{c}}$. Let

$$\begin{aligned} v_c (x)=\alpha _c \max \{0, e^{-\mu _c x}-e^{-\lambda x}\}, \end{aligned}$$

where $\mu _c<\lambda <\min \{\mu ^*_1, 2\mu _c\}$. This function is zero for $x\le 0$, and positive for $x>0$, and it has a maximum less than $\alpha _c$ at

$$\begin{aligned} \sigma _c=\frac{\ln \lambda -\ln \mu _c}{\lambda -\mu _c}. \end{aligned}$$

The first part of the proof of statement (i) still works to show that for any small positive $\eta$ there is a real number $x_1$ such that (6.5) holds. Let ${\tilde{Q}}^1_{n}$ denote the operator determined by the right-hand sides of (6.5). The work in Weinberger (1978) shows that for sufficiently small $\alpha _c$, and for $n\ge 1$ and $x\in {{\mathbb {R}}}$,

$$\begin{aligned} {\tilde{Q}}^1_{1}[v_c(\cdot -x_1- b )](x)\ge v_c(x-x_1- b-{\tilde{c}}). \end{aligned}$$

(6.7)

Since $u_0(x)=w(x)$ where w(x) is nondecreasing with $w(-\infty )=1$, $w(\infty )=0$, and (2.3) holds, for sufficiently small $\alpha _c$, we have $u_0(x)>v_c(x-x_1-b)$ for all x. (6.5) and (6.7) shows that for $x\in {{\mathbb {R}}}$, $u_1(x)\ge v_c (x-x_1-b-{\tilde{c}})$. (6.5), (6.7), and induction show that for all n and $x\in {{\mathbb {R}}}$,

$$\begin{aligned} u_n(x)\ge v_{c}(x- x_1-b-n{\tilde{c}}). \end{aligned}$$

Define

$$\begin{aligned} v ({\mu };x)= \left\{ \begin{array}{ll} \alpha e^{-\mu x}\sin \gamma x, &{} ~ \mathrm{if} ~ 0\le x\le \pi /\gamma ,\\ 0, &{} ~ \mathrm{elsewhere}, \end{array} \right. \end{aligned}$$

where $\alpha$, $\mu$ and $\gamma$ are positive numbers. (This function is called v(s) in Weinberger (1982)). $v ({\mu };x)$ has a maximum value less than $\alpha$ at

$$\begin{aligned} \sigma (\mu )=(1/\gamma )\tan ^{-1}(\gamma /\mu ). \end{aligned}$$

Let

$$\begin{aligned} v_-(\mu ; x)=v(\mu ; -x). \end{aligned}$$

Define

$$\begin{aligned} z({\mu }; \gamma )=\frac{1}{\gamma }\tan ^{-1}\frac{ \int _{-\infty }^{\infty }k_1(y)e^{\mu y}\sin \gamma y dy}{ \int _{-\infty }^{\infty }k_1(y)e^{\mu y}\cos \gamma ydy}, \end{aligned}$$

and

$$\begin{aligned} z_-({\mu }; \gamma )=-\frac{1}{\gamma }\tan ^{-1}\frac{ \int _{-\infty }^{\infty }k_1(y)e^{-\mu y}\sin \gamma y dy}{ \int _{-\infty }^{\infty }k_1(y)e^{-\mu y}\cos \gamma ydy}. \end{aligned}$$

Let

$$\begin{aligned} \psi (\mu )=\frac{\int _{-\infty }^{\infty }y k_1(y)e^{\mu y} dy}{\int _{-\infty }^{\infty }k_1(y)e^{\mu y} dy}, \ \ \psi _-(\mu )=-\frac{\int _{-\infty }^{\infty }y k_1(y)e^{-\mu y} dy}{\int _{-\infty }^{\infty }k_1(y)e^{-\mu y} dy}. \end{aligned}$$

Obviously $\psi _-(0)=-\psi (0)$. It is known $c^*_1>\psi (0)$ and $c^*_{1-}(0)>\psi _-(0)$ (Weinberger 1982).

Let $\epsilon$ and $\gamma$ be small positive numbers and L be a number with $L > 4\pi /\gamma$. Let $z(\mu _1; \gamma )=c_0+{\varepsilon }/2$ if $c_0\ge \psi (0)$ and $z_-(\mu _1; \gamma )=\min \{-c_0, c^*_{1-}\}- {\varepsilon }/2$ if $c_0< \psi (0)$. Define

$$\begin{aligned} u^{(n)}_{c; r}(\epsilon , \mu _1; x){=} \left\{ \begin{array}{ll} v({\mu _1}; x-nz(\mu _1; \gamma )), &{} \mathrm{if}~ nz(\mu _1; \gamma )\le x\le \sigma (\mu _1) {+}nz(\mu _1; \gamma ), \\ \\ \epsilon , &{} \mathrm{if}~ \sigma (\mu _1)+nz (\mu _1; \gamma ) \le x \le \sigma _c+L \\ &{} ~ ~ ~ -\frac{\pi }{\gamma } +nc, \\ dv_{c}\left( x-L+ \frac{\pi }{\gamma }-nc\right) , &{} \mathrm{if}~ x\ge \sigma _c+L-\frac{\pi }{\gamma }+nc \\ 0, &{} ~\mathrm{elsewhere,} \end{array} \right. \end{aligned}$$

(6.8)

and

$$\begin{aligned} u^{(n)}_{c}(\epsilon , \mu _1; x)= \left\{ \begin{array}{ll} v_-({\mu _1}; x+nz_-(\mu _1; \gamma )), &{} \mathrm{if}~ -nz_-(\mu _1; \gamma )-\frac{\pi }{\gamma }\le x\le -nz_-(\mu _1; \gamma ) \\ &{} -\sigma (\mu _1),\\ \\ \epsilon , &{} \mathrm{if}~ -nz_- (\mu _1; \gamma ) - \sigma (\mu _1) \le x \le \sigma _c+L \\ &{} ~~~ -\frac{\pi }{\gamma } +nc, \\ dv_{c}\left( x-L+ \frac{\pi }{\gamma }-nc\right) , &{} \mathrm{if}~ x\ge \sigma _c+L-\frac{\pi }{\gamma }+nc\\ 0, &{} ~\mathrm{elsewhere.} \end{array} \right. \end{aligned}$$

(6.9)

In (6.8), $\alpha$, $\alpha _c$ and d satisfy $v({\mu _1};\sigma (\mu _1))=dv_{c}(\sigma _c)=\epsilon$. In (6.9), $\alpha$, $\alpha _c$ and d satisfy $v_-({\mu _1};-\sigma (\mu _1))=dv_c(\sigma _c)=\epsilon$.

$u^{(n)}_{c,r}(\epsilon , \mu _1; x)$ and $u^{(n)}_{c}(\epsilon , \mu _1; x)$ are similar to $u^{(n)}_{r}(\epsilon , \mu _1, \mu _2; x)$ and $u^{(n)}(\epsilon , \mu _1, \mu _2; x)$ given in Li et al. (2016), respectively. Note $\lim _{x\rightarrow \infty }u_0(x) >0$. (6.5) and (6.6), and the proofs of (ii) and (iii) of Lemma 4 in Li et al. (2016) with $\sigma (\mu _2)$ replaced by $\sigma _c$ show that for sufficiently small $\eta$, $\gamma$, $\alpha$, $\alpha _c$, and $L>4\pi /\gamma$ there exists $x_2$ such that if $c_0\ge \psi (0)$,

$$\begin{aligned} u_n(x)\ge u^{(n)}_{c,r}(\mu _1; x-nz(\mu _1; \gamma )-(x_2+b)) \end{aligned}$$

for all $n\ge 1$ and $x\in {{\mathbb {R}}}$, and if $c_0< \psi (0)$,

$$\begin{aligned} u_n(x)\ge u^{(n)}_{c}(\mu _1; x+nz_-(\mu _1; \gamma )-(x_2+b)) \end{aligned}$$

for all $n\ge 1$ and $x\in {{\mathbb {R}}}$. The proof of the statement (ii) (b) of Theorem 2 in Li et al. (2016) with $\sigma (\mu _2)$ replaced by $\sigma _c$ shows that

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c_0, c^*_{1-}\}+\varepsilon )\le x\le n(c-\varepsilon )} u_n(x)\right] \ge 1-4\alpha _1\eta ,} \end{aligned}$$

which leads to the conclusion of the statement (v), since $\eta$ is arbitrary and $u_n(x)\le 1$ for all n and $x\in {{\mathbb {R}}}$.

We finally prove the statement (vi). Using an argument similar to that for showing (6.5), we obtain that for any small $\eta >0$ there exits a real number $x_1$ such that for $n\ge 1$ and $x\in {{\mathbb {R}}}$,

$$\begin{aligned} {\displaystyle u_{n+1}(x) \ge \int _{-\infty }^{\infty }k_{1b}(x-y) \frac{(1+\rho _1)u_n(y)}{1+\rho _1 u_n(y)+\delta _- (y-x_1+nc_0)}dy}, \end{aligned}$$

(6.10)

where

$$\begin{aligned} \delta _- (x)= \left\{ \begin{array}{ll} \rho _1\alpha _1, &{} ~ \mathrm{if}~ x\ge 0, \\ \rho _1\alpha _1\eta , &{} ~ \mathrm{if} ~ x< 0. \end{array}\right. \end{aligned}$$

The integro-difference equation corresponding to (6.10) may be written as

$$\begin{aligned} {\displaystyle N_{n+1}(x) = \int _{-\infty }^{\infty }k_{1b}(x-y) \frac{(1+\rho _1)N_n(y)}{1+\rho _1 N_n(y)+\delta _- (y-x_1+nc_0)}dy}. \end{aligned}$$

(6.11)

This takes the form of model (6) studied in Li et al. (2016) with the growth function $g(y, N)= \frac{(1+\rho _1)N}{1+\rho _1 N+\delta _- (y-x_1+nc_0)}$. This is increasing in N and nonincreasing in y. In Li et al. (2016), g(y, N) is assumed to be nondecreasing in both N and y. The results about leftward spreading speeds given in Li et al. (2016) work to determine the rightward spreading speed for (6.11). Choose b sufficiently large and $\eta$ sufficiently small so that $N^*=\frac{(1+\rho _1)\int _{-\infty }^{\infty }k_{1b}(x)dx -1-\rho _1\alpha _1\eta }{\rho _1} >1-2\alpha _1\eta$. Note that $N^*$ is the positive equilibrium of (6.11) with $\delta _- (y-x_1+nc_0)$ replaced by $\rho _1\alpha _1\eta$.

For $-c_0>\psi (0)$, choose $z(\mu _1; \gamma )=\min \{c^*_1, -c_0 \}-{\varepsilon }/2$ and define

$$\begin{aligned} {\hat{u}}^{(n)}(\epsilon , \mu _1; x)= \left\{ \begin{array}{ll} \epsilon , &{} \mathrm{if}~ x \le \sigma (\mu _1)+nz(\mu _1; \gamma ),\\ dv({\mu _1}; x -nz(\mu _1; \gamma )), &{} \mathrm{if}~ x>\sigma (\mu _1)+nz(\mu _1; \gamma ), \\ 0, &{} ~\mathrm{elsewhere,} \end{array} \right. \end{aligned}$$

where ${\epsilon }$ is a small positive number, ${\epsilon }=dv(\mu _1; \sigma (\mu _1))$.

For $-c_0\le \psi (0)$, since $c_0<c^*_{1-}$, we may choose $z_-(\mu _1; \gamma )=c_0+{\varepsilon }/2$ and define

$$\begin{aligned} {\hat{u}}^{(n)}(\epsilon , \mu _1; x)= \left\{ \begin{array}{ll} \epsilon , &{} \mathrm{if}~ x \le -\sigma (\mu _1)-nz_-(\mu _1; \gamma ),\\ dv_-({\mu _1}; x +nz_-(\mu _1; \gamma )), &{} \mathrm{if}~ x>-\sigma (\mu _1)-nz_-(\mu _1; \gamma ), \\ 0, &{} ~\mathrm{elsewhere,} \end{array} \right. \end{aligned}$$

where ${\epsilon }$ is a small positive number, ${\epsilon }=dv_-(\mu _1; -\sigma (\mu _1))$.

${\hat{u}}^{(n)}(\epsilon , \mu _1; x)$ is a one-sided function. Lemma 4 in Li et al. (2016) provides several two-sided lower solutions for (6.11). Since $\lim _{x{\rightarrow }-\infty }u_0(x)>0$, there exists a small positive number ${\epsilon }$ and a real number $x_2$ such that $u_0(x)\ge {\hat{u}}^{(0)}(\epsilon , \mu _1; x-x_2)$ for all x. Choose $N_0(x)=u_0(x)$ in (6.11). A simplified version of the proof of Lemma 4 (i) shows that for any small positive number ${\varepsilon }$, there exist small positive numbers $\alpha$, ${\epsilon }$, and $\gamma$, and a real number $x_2$ such that

$$\begin{aligned} N_n(x)\ge {\hat{u}}^{(n)}(\epsilon , \mu _1; x-x_2) \end{aligned}$$

for all n and $x\in {{\mathbb {R}}}$.

Since $u_n(x)\ge N_n(x)$ for all n and $x\in {{\mathbb {R}}}$, $u_n(x)\ge {\hat{u}}^{(n)}(\epsilon , \mu _1; x-x_2)$ for all n and $x\in {{\mathbb {R}}}$. Thus

$$\begin{aligned} u_n(x)\ge {\epsilon }, \ \ \ \text{ for } x\le \sigma (\mu _1)+n(\min \{c^*_1, -c_0 \}-{\varepsilon }/2)+x_2 \end{aligned}$$

(6.12)

if $-c_0>\psi (0)$, or

$$\begin{aligned} u_n(x)\ge {\epsilon }, \ \ \ \text{ for } x\le -\sigma (\mu _1)-n(c_0 +{\varepsilon }/2)+x_2 \end{aligned}$$

(6.13)

if $-c_0\le \psi (0)$. Note that under this condition, $c^*_1>-c_0$, and $\min \{c^*_1, -c_0 \}=-c_0$.

We use ${\hat{Q}}^1_{\eta }$ to denote the operator determined by the left-hand side of (6.11) with $\delta (x-x_1+nc_0)$ replaced by $\rho _1\eta$. Let $({\hat{Q}}^1_{\eta })^n$ be the nth iteration of ${\hat{Q}}^1_{\eta }$. Since $\lim _{n{\rightarrow }\infty }({\hat{Q}}^1_{\eta })^n [{\epsilon }]=N^*$, there is an integer N such that $({\hat{Q}}^1_{\eta })^N [{\epsilon }]\ge N^*-\alpha _1\eta >1-3\alpha _1\eta$. It follows from this, (6.12), and (6.13) that for $n\ge N$,

$$\begin{aligned} u_n(x)\ge 1-3\alpha _1\eta , \ \ \ \text{ for } x\le -\sigma (\mu _1)+n(\min \{c^*_1, -c_0 \}-{\varepsilon }/2)+x_2-Nb. \end{aligned}$$

On the other hand, there exists $n_1$ such that for $n\ge n_1$, $n(\min \{c^*_1, -c_0 \}-{\varepsilon }\le -\sigma (\mu _1)+n(\min \{c^*_1, -c_0 \}-{\varepsilon }/2)+x_2-Nb$. We therefore have that $\liminf _{x\le n(\min \{c^*_1, -c_0 \}-{\varepsilon })}u_n(x) \ge 1-3\alpha _1\eta$. Since $\eta$ is arbitrarily small and $u_n(x)\le 1$, the statement (vi) follows.

The proof is complete. $\square$

When the equilibrium (0, 1) is unstable and the equilibrium (1, 0) is asymptotically stable, i.e., $\alpha _2>1>\alpha _1$, using the change of variables $p_n(x)=u_n(x)$, $q_n(x)=1-v_n(x)$, we convert (2.1) into the cooperative system

$$\begin{aligned} \begin{aligned} \displaystyle {p}_{n+1}(x)&=\int _{-\infty }^{\infty }k_1(x-y) \frac{(1+\rho _1)p_n(y)}{1+\rho _1 (\alpha _1+p_n(y)-\alpha _1 q_n(y)))}dy, \\ \displaystyle {q}_{n+1}(x)&= \int _{-\infty }^{\infty }k_2(x-y) \frac{\alpha _2\rho _2p_n(y)+q_n(y)}{1+\rho _2(1-q_n(y)+\alpha _2p_n(y))}dy. \end{aligned} \end{aligned}$$

(6.14)

Similarly when the equilibrium (1, 0) is unstable and the equilibrium (0, 1) is asymptotically stable, i.e., if $\alpha _1>1>\alpha _2$, using the change of variables ${p}_n=v_n$ and ${q}_n(x)=1-u_n(x)$ we convert (2.1) into the cooperative system

$$\begin{aligned} \begin{aligned} \displaystyle {p}_{n+1}(x)&=\int _{-\infty }^{\infty }k_2(x-y) \frac{(1+\rho _2){p}_n(y)}{1+\rho _2 (\alpha _2+{p}_n(y)-\alpha _2 {q}_n(y)))}dy,\\ \displaystyle {{q}}_{n+1}(x)&= \int _{-\infty }^{\infty }k_1(x-y) \frac{\alpha _1\rho _1{p}_n(y)+{q}_n(y)}{1+\rho _1(1-{q}_n(y)+\alpha _1{p}_n(y))}dy. \end{aligned} \end{aligned}$$

(6.15)

If subscripts 1 and 2 are interchanged, system (6.15) becomes (6.14). For both systems (0, 0) is unstable, and (1, 1) is asymptotically stable. These two systems will be used in the proofs of the next two lemmas.

Lemma 6.2

Assume that (H) holds.

• i. Let $\alpha _2>1>\alpha _1$ and (${LD}_1$) hold. Then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\ge n({\bar{c}}_1+\varepsilon )} u_n (x)\right] =0}, \end{aligned}$$

  and

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{n({\bar{c}}_1 +\varepsilon )\le x\le n (c^*_2-\varepsilon )} |1- v_n (x)|\right] =0}, \end{aligned}$$

  provided that one of the following conditions holds

  • a. (${IV}_1$) is satisfied, ${\bar{\mu }}_1<\mu _{c}$, and ${\displaystyle c^*_2> \frac{\mu _{c}c}{\mu _{c}-{\bar{\mu }}_1} }.$

  • b. (${IV}_2$) is satisfied, and ${\displaystyle c^*_2> \frac{\mu ^*_1c^*_1}{\mu ^*_1-{\bar{\mu }}_1} }$.

• ii. Let $\alpha _2>1>\alpha _1$ and (${LD}_{1-}$) hold. Then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\le - n({\bar{c}}_{1-}+\varepsilon )} u_n (x)\right] =0}, \end{aligned}$$

  and

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n (c^*_{2-} -\varepsilon )\le x\le -n ({\bar{c}}_{1-}+\varepsilon )} |1- v_n (x)|\right] =0}, \end{aligned}$$

  provided that (${IV}_2$) is satisfied and ${\displaystyle c^*_{2-}> \frac{\mu ^*_{1-}c^*_{1-}}{\mu ^*_{1-}-{\bar{\mu }}_{1-} }}$.

• iii. Let $\alpha _1>1>\alpha _2$ and (${LD}_{2}$) hold. Then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{n({\bar{c}}_{2}+\varepsilon )\le x\le n(c-\varepsilon )} |1-u_n (x)|\right] =0}, \end{aligned}$$

  and

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\ge n ({\bar{c}}_{2}+\varepsilon )} v_n (x)\right] =0}, \end{aligned}$$

  provided that one of the following conditions holds:

  • a. (${IV}_1$) is satisfied, and ${\displaystyle c > \frac{\mu ^*_{2}c^*_2}{\mu ^*_{2}-{\bar{\mu }}_2}}$.

  • b. (${IV}_2$) is satisfied, and ${c=\displaystyle c^*_1 > \frac{\mu ^*_{2}c^*_2}{\mu ^*_{2}-{\bar{\mu }}_2} }.$

• iv. Let $\alpha _1>1>\alpha _2$ and (${LD}_{2-}$) hold. Then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{-n(c^*_{1-}-\varepsilon )\le x\le -n({\bar{c}}_{2-}+\varepsilon )} |1-u_n (x)|\right] =0}, \end{aligned}$$

  and

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\le -n({\bar{c}}_{2-}+\varepsilon ) } v_n (x)\right] =0}, \end{aligned}$$

  provided that (${IV}_2$) is satisfied and ${\displaystyle c^*_{1-}> \frac{\mu ^*_{2-}c^*_{2-}}{\mu ^*_{2-}-{\bar{\mu }}_{2-} }}$.

• v. Let $\alpha _1>1>\alpha _2$ and (${LD}_{2-}$) hold. Then for any small positive $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\le -n({\bar{c}}_{2-}+\varepsilon )} |1-u_n (x)|\right] =0}, \end{aligned}$$

  and

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{x\le -n({\bar{c}}_{2-}+\varepsilon ) } v_n (x)\right] =0}, \end{aligned}$$

  provided that (${IV}_1$) is satisfied.

Proof

We first prove the statement (i) under condition (a). Note that $u_n(x)\le w(x-nc)$ for all n and $x\in {{\mathbb {R}}}$, and thus $\lim _{x\ge nc_0} u_n(x)=0$ for any $c_0>c$. Since $c^*_2>c$, Lemma 6.1 (iii) implies that

$$\begin{aligned} \lim _{n\rightarrow \infty }\left[ \sup _{-n(\min \{-c, \ c^*_{2-}\}-\varepsilon )\le x\le n(c^*_2-\varepsilon )} |1-v_n(t, x)| \right] =0. \end{aligned}$$

It follows that for any small positive $\eta$, there is $N_1$ such that

$$\begin{aligned} 1-\eta \le v_n(x) \le 1, \ \ \text{ for } n\ge N_1 \text{ and } n(\max \{c, \ -c^*_{2-}\}+\varepsilon )\le x\le n(c^*_2-\varepsilon ). \end{aligned}$$

(6.16)

We use Q to denote the operator determined by the right-hand sides of the two equations in (6.14) and $\mathbf{w}$ to denote a vector with two components. We rewrite (6.14) in the form

$$\begin{aligned} \mathbf{w}_{n+1}(x)=Q[\mathbf{w}_n](x) \end{aligned}$$

where $\mathbf{w}_n(x)=(p_n(x), q_n(x))$.

Let ${\varvec{\eta }}=(0, \eta )$. Then

$$\begin{aligned} Q[{\varvec{\eta }}]=\left( 0, \frac{\eta }{1+\rho _2(1-\eta )} \right) . \end{aligned}$$

Since $\eta$ is small, $\frac{\eta }{1+\rho _2(1-\eta )}<\eta$, and thus

$$\begin{aligned} Q[{\varvec{\eta }}]<{\varvec{\eta }}. \end{aligned}$$

Consider the operator ${Q}_{\eta }$ defined by

$$\begin{aligned} {Q}_{\eta }[\mathbf{w}](x):=Q[\mathbf{w}+{\varvec{\eta }}]-Q[{\varvec{\eta }}]. \end{aligned}$$

Clearly ${Q}_{\eta }[\mathbf{0 }]=\mathbf{0 }=(0,0).$ We introduce the notation

$$\begin{aligned} {Q}_{\eta }[( p (\cdot ), q (\cdot ))](x)=({Q}_{\eta 1}[( p (\cdot ), q (\cdot ))](x), {Q}_{\eta 2}[(p (\cdot ), q (\cdot ))](x)) \end{aligned}$$

where

$$\begin{aligned} {\displaystyle {Q}_{\eta 1}[( p (\cdot ), q (\cdot ))](x) =\int _{-\infty }^{\infty }k_1(x-y) \frac{(1+\rho _1) {p}(y)}{1+\rho _1 ({p}(y)+\alpha _1(1-\eta -{q}(y)))}dy,} \end{aligned}$$

and

$$\begin{aligned}&\displaystyle {Q}_{\eta 2}[( p (\cdot ), q (\cdot ))](x)\\&\quad = \int _{-\infty }^{\infty }k_2(x-y)\frac{\alpha _2\rho _2(1+\rho _2(1-\eta )-\eta ) {p}(y)+(1+\rho _2){q}(y)}{(1+\rho _2(1-\eta ))(1+\rho _2(1-\eta -{q}(y) +\alpha _2{p}(y))}dy. \end{aligned}$$

We use $L_{\eta }$ to denote the linerization of $Q_{\eta }$ at ${ 0}$, and introduce the notation

$$\begin{aligned} {L}_{\eta }[(p (\cdot ), q (\cdot ))](x)=({L}_{\eta 1}[(p (\cdot ), q (\cdot ))](x), {L}_{\eta 2}[( p (\cdot ), q (\cdot ))](x)) \end{aligned}$$

where

$$\begin{aligned} {\displaystyle {L}_{\eta 1}[( p (\cdot ), q (\cdot ))](x) =\int _{-\infty }^{\infty }k_1(x-y) \frac{1+\rho _1}{1+\rho _1\alpha _1(1-\eta )}{p}(y)dy,} \end{aligned}$$

and

$$\begin{aligned}&\displaystyle {L}_{\eta 2}[( p (\cdot ), q (\cdot ))](x)\\&\quad =\int _{-\infty }^{\infty }k_2(x-y) \frac{\alpha _2\rho _2(1+\rho _2(1-\eta )-\eta ){p}(y)+(1+\rho _2){q}(y)}{(1+\rho _2(1-\eta ))^2}dy. \end{aligned}$$

Direct calculations show that ${Q}_{\eta }[( p, q )] \le {L}_{\eta }[(p, q)]$, i.e., ${Q}_{\eta 1}[( p, q)]\le {L}_{\eta 1}[( p, q)]$ and ${Q}_{\eta 1}[( p, q)]\le {L}_{\eta 2}[( p, q)]$, if

$$\begin{aligned} p\ge \alpha _1 q, \ \ \alpha _2 p\ge q, \end{aligned}$$

or equivalently

$$\begin{aligned} p\ge \max \{\alpha _1, 1/\alpha _2 \} q. \end{aligned}$$

(6.17)

In order to calculate the spreading speed for the linear operator $L_{\eta }$, we need the moment generating matrix ${ B}_\eta (\mu )$, which is defined by setting ${p} = a_1 e^{-\mu x}$ and ${q} = a_2e^{-\mu x}$ in $L_{\eta }[ p (\cdot ), q (\cdot )](x)$, multiplying the result by $e^{\mu x}$, and writing the vector so obtained as a matrix product ${ B}_{\eta }(\mu )\left( \begin{array}{c} a_1 \\ a_2 \end{array} \right) .$ We find

$$\begin{aligned} { B}_{\eta }(\mu )=\left( \begin{array}{cc} \frac{1+\rho _1}{1+\rho _1\alpha _1(1-\eta )}{\bar{k}}_1(\mu ) &{} 0 \\ \\ \frac{\alpha _2\rho _2(1+\rho _2(1-\eta )-\eta )}{(1+\rho _2(1-\eta ))^2}{\bar{k}}_2(\mu ) &{} \frac{1+\rho _2}{(1+\rho _2(1-\eta ))^2}{\bar{k}}_2(\mu ) \end{array} \right) . \end{aligned}$$

(6.18)

The eigenvalues of this matrix are the diagonal entries

$$\begin{aligned} {\lambda }_{\eta 1} (\mu ) = \frac{1+\rho _1}{1+\rho _1\alpha _1(1-\eta )}{\bar{k}}_1(\mu ), \end{aligned}$$

and

$$\begin{aligned} {\lambda }_{\eta 2}( \mu )=\frac{1+\rho _2}{(1+\rho _2(1-\eta ))^2}{\bar{k}}_2(\mu ). \end{aligned}$$

For sufficiently small positive $\epsilon$ and $\eta$,

$$\begin{aligned} \frac{\ln {\lambda }_{\eta 1} ({\bar{\mu }}_1)}{{\bar{\mu }}_1} \le {\bar{c}}_1+\epsilon , \ \ {\lambda }_{\eta 1} ({\bar{\mu }}_1)>{\lambda }_{\eta 2} ({\bar{\mu }}_1). \end{aligned}$$

An eigenvector of ${B}_{{\eta }}({\bar{\mu }}_1)$ corresponding to ${\lambda }_{\eta 1} ({\bar{\mu }}_1)$ is ${\varvec{\xi }}_{\eta }({\bar{\mu }}_1)=(\xi _{\eta 1}({\bar{\mu }}_1), \ \ \xi _{\eta 2}({\bar{\mu }}_1))$

where

$$\begin{aligned} \xi _{\eta 1}({\mu })= & {} \frac{1+\rho _1}{1+\rho _1\alpha _1(1-\eta )} {\bar{k}}_1({\mu })-\frac{1+\rho _2}{(1+\rho _2(1-\eta ))^2}{\bar{k}}_2(\mu ),\nonumber \\ \xi _{\eta 2}({\mu })= & {} \frac{\alpha _2\rho _2(1+\rho _2(1-\eta )-\eta )}{(1+\rho _2(1-\eta ))^2}{\bar{k}}_2({\mu }). \end{aligned}$$

(6.19)

Since $\eta$ is sufficiently small, $\xi _{\eta 2}({\bar{\mu }}_1)>0$, and $\xi _{\eta 1}({\bar{\mu }}_1)>0$ due to (${\text{ LD }}_1$). This linear determinacy condition implies that $p=\xi _{\eta 1}({\bar{\mu }}_1), q=\xi _{\eta 2}({\bar{\mu }}_1)$ satisfy (6.17), so that $Q_\eta$ is dominated by $L_{\eta }$ in the direction of ${\varvec{\xi }}_{\eta }({\bar{\mu }}_1)$.

For $\epsilon$ and ${\bar{\mu }}_1$, there is $b>0$ such that $b>\max \{|c^*_2|, |c^*_{2-}| \}$ and for $i=1, 2$,

$$\begin{aligned} {\bar{k}}_i({\bar{\mu }}_1)\le \left( 1+\frac{{\bar{\mu }}_1\epsilon }{2}\right) \int _{-\infty }^{\infty } k_{ib}(x)e^{{\bar{\mu }}_1 x} dx. \end{aligned}$$

(6.20)

We use ${L}_{\eta b}$ to denote the linear operator determined by $L_{\eta }$ where $k_i(x)$ are replaced by $k_{ib}(x)$.

We use ${\tilde{\mu }}_c$ to denote the smaller positive solution of

$$\begin{aligned} \frac{\ln ((1+\rho _1){\bar{k}}_{1b}(\mu ))}{\mu }=c+\epsilon . \end{aligned}$$

$\mu _{c}>{\tilde{\mu }}_c> {\bar{\mu }}_{1}$. Since $\epsilon$ is small, $\mu _{c}\approx {\tilde{\mu }}_c$. We choose a large integer $N_2$ such that

$$\begin{aligned} N_2{>}\max \left\{ N_1,\ \ \frac{{\tilde{\mu }}_c (c{+}{\epsilon })-{\bar{\mu }}_1({\bar{c}}_1{+}{\epsilon }){+}b({\tilde{\mu }}_c {-}{\bar{\mu }}_1)}{({\tilde{\mu }}_c{-}{\bar{\mu }}_1)(c^*_2-{\varepsilon })- {\tilde{\mu }}_c (c+{\epsilon })}, \ \ \frac{b{+}\max \{c, -c^*_{2-}\}+\varepsilon }{c^*_2-\max \{c, -c^*_{2-}\}-2\varepsilon }\right\} . \end{aligned}$$

(6.21)

The first equation of (6.14) and $0\le q_n(x) \le 1$ show that

$$\begin{aligned} {\displaystyle {p}_{n+1}(x) =\int _{-\infty }^{\infty }k_1(x-y) \frac{(1+\rho _1)p_n(y)}{1+\rho _1 p_n(y)} dy \le \int _{-\infty }^{\infty }k_1(x-y) (1+\rho _1)p_n(y) dy. } \end{aligned}$$

(6.22)

Because of (2.3) and (2.4), one can choose $A_1$ sufficiently large such that $p_0(x)=w(x)\le A_1\xi _{\eta 1} ({\bar{\mu }}_1) e^{-{\tilde{\mu }}_c x}$. Then (6.22) and induction show that

$$\begin{aligned} p_n(x)\le A_1\xi _{\eta 1} ({\bar{\mu }}_1)e^{-{\tilde{\mu }}_c(x-n(c+\epsilon ))}, \text{ for } \text{ all } \text{ n } \text{ and } \text{ x. } \end{aligned}$$

(6.23)

Particularly we have that

$$\begin{aligned} p_{N_2}(x)\le A_1\xi _{\eta 1}( {\bar{\mu }}_1) e^{{\tilde{\mu }}_c (c+\epsilon ) N_2} e^{-{\tilde{\mu }}_c x}, \text{ for } \text{ all } x. \end{aligned}$$

Since $q_n(x)\le 1$ for all n and $x\in {{\mathbb {R}}}$, in view of (6.16), we can choose $A_2$ sufficiently large such that for $x\le N_2(c^*_2-\varepsilon )$,

$$\begin{aligned} q_{N_2}(x)\le A_2 \xi _{\eta 2} ({\bar{\mu }}_1) e^{- {\bar{\mu }}_1 x } +\eta . \end{aligned}$$

One can further choose large $A_1$ and $A_2$ in such a way that

$$\begin{aligned} A_2 \xi _{\eta 1}({\bar{\mu }}_1)> A_1 \xi _{\eta 1} ({\bar{\mu }}_1), \ \ A_1 \xi _{\eta 1} ({\bar{\mu }}_1) e^{{\tilde{\mu }}_c (c+\epsilon ) N_2}>1. \end{aligned}$$

(6.24)

This and the condition ${\bar{\mu }}_1<{\tilde{\mu }}_c$ imply that $A_2 \xi _{\eta 2} ({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 x }$ and $A_1 \xi _{\eta 1} ({\bar{\mu }}_1) e^{{\tilde{\mu }}_c (c+\epsilon ) N_2} e^{-{\tilde{\mu }}_c x}$ intersect at a number where the value of the two functions is greater than 1, and $A_2 \xi _{\eta 2} ({\bar{\mu }}_1)e^{-{\bar{\mu }}_1 x }$is above $A_1 \xi _{\eta 1} ({\bar{\mu }}_1) e^{-{\tilde{\mu }}_c (c+\epsilon ) N_2} e^{-{\tilde{\mu }}_c x}$ after the intersection point. Since $p_{N_2}(x)\le 1$, in view of (6.23),

$$\begin{aligned} p_{N_2}(x) \le A_2\xi _{\eta 1} ({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 x } +\eta \end{aligned}$$

for all x.

Assume that for some nonnegative integer m

$$\begin{aligned} p_{N_2+m}(x)\le A_2\xi _{\eta 1} ({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 (x-m({\bar{c}}_1 +\epsilon ))}, \ \ \text{ for } \text{ all } x, \end{aligned}$$

(6.25)

and

$$\begin{aligned} q_{N_2+m}(x)\le A_2 \xi _{\eta 2} ({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 (x-m({\bar{c}}_1 +\epsilon )} +\eta , \ \ \text{ for } x\le (N_2+m)(c^*_2-\varepsilon ). \end{aligned}$$

(6.26)

Then for $x\le (N_2+m) (c^*_2-\varepsilon )-b$,

$$\begin{aligned} \begin{aligned} (p_{N_2+m+1}(x), p_{N_2+m+1}(x))&=Q[(p_{N_2+m}(\cdot ), p_{N_2+m}(\cdot ))](x) \\&\le Q[ A_2 e^{-{\bar{\mu }}_1 (\cdot -m ({\bar{c}}_1+\varepsilon ))} {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1) +{\varvec{\eta }}](x)\\&={Q}_{\eta }[ A_2 e^{-{\bar{\mu }}_1 (\cdot -m({\bar{c}}_1+\varepsilon )} {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)](x)+Q[{\varvec{\eta }}]. \end{aligned} \end{aligned}$$

(6.27)

Since $( p, q)=A_2 e^{-{\bar{\mu }}_1 x } {\varvec{\xi }}_\eta ({\bar{\mu }}_1)$ satisfies (6.17), the inequalities $Q[{\varvec{\eta }}]\le {\varvec{\eta }}$, (6.20), and (6.27) show

$$\begin{aligned} \begin{aligned} (p_{N_2+m+1}(x), q_{N_2+m+1}(x))&\le L_{\eta } [ A_2 e^{-{\bar{\mu }}_1 (\cdot -m({\bar{c}}_1+\varepsilon )) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)](x)+{\varvec{\eta }}\\&\le \left( 1+\frac{{\bar{\mu }}_1\epsilon }{2}\right) L_{\eta b}[ A_2 e^{-{\bar{\mu }}_1 (\cdot -m({{\bar{c}}}+\varepsilon )) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1) ](x)+{\varvec{\eta }}. \end{aligned} \end{aligned}$$

This shows that for $x\le (N_2+m) (c^*_2-\varepsilon )-b$,

$$\begin{aligned} \begin{aligned} (p_{N_2+m+1}(x), q_{N_2+m+1}(x))&\le \left( 1+\frac{{\bar{\mu }}_1\epsilon }{2}\right) L_{\eta b} [ A_2 e^{-{\bar{\mu }}_1 (\cdot -m({\bar{c}}_1+\epsilon ) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)](x)+{\varvec{\eta }}\\&\le \left( 1+\frac{{\bar{\mu }}_1 \epsilon }{2}\right) L_{\eta } [ A_2 e^{-{\bar{\mu }}_1 (\cdot -m({\bar{c}}_1+\epsilon ) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)](x)+{\varvec{\eta }}\\&=\left( 1+\frac{{\bar{\mu }}_1 \epsilon }{2}\right) \lambda _{\eta 1}({\bar{\mu }}_1)A_2 e^{-{\bar{\mu }}_1 (x -m({\bar{c}}_1+\epsilon ) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)+{\varvec{\eta }}\\&\le A_2 \left( 1+\frac{{\bar{\mu }}_1\epsilon }{2}\right) e^{-{\bar{\mu }}_1 (x-m({\bar{c}}_1+\epsilon )- ({\bar{c}}_1+\epsilon /2)) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_1)+{\varvec{\eta }}\\&\le A_2 e^{-{\bar{\mu }}_1 (x-(m+1)({\bar{c}}_1+\epsilon )) } {\varvec{\xi }}_{\eta }( {\bar{\mu }}_1)+{\varvec{\eta }}. \end{aligned} \end{aligned}$$

(6.28)

On the other hand, since $A_2>A_1$ according to (6.24), the condition $N_2> \frac{{\tilde{\mu }}_c (c+{\epsilon })-{\bar{\mu }}_1({\bar{c}}_1+{\epsilon })+b({\tilde{\mu }}_c-{\bar{\mu }}_1)}{({\tilde{\mu }}_c-{\bar{\mu }}_1)(c^*_2-{\varepsilon })- {\tilde{\mu }}_c (c+{\epsilon })}$ from (6.21) implies that for $x\ge (N_2+m) (c^*_2-\varepsilon )-b$,

$$\begin{aligned} A_1\xi _{\eta 1}({\bar{\mu }}_1)e^{-{{\tilde{\mu }}_c }(x-(N_2+m+1)c^*_1 )}\le A_2\xi _{\eta 1}({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 (x-(m+1)({{\bar{c}}}+\epsilon )) }. \end{aligned}$$

This, (6.23), and (6.28) show that

$$\begin{aligned} p_{N_2+m+1}(x)\le A_2\xi _{\eta 1}({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 (x-(m+1)({\bar{c}}_1+\epsilon )) }, \text{ for } \text{ all } x. \end{aligned}$$

The inequality $c^*_2-2{\varepsilon }>\max \{c, -c^*_{2-}\}$ and the condition $N_2> \frac{b+\max \{c, -c^*_{2-}\}+\varepsilon }{c^*_2-\max \{c, -c^*_{2-}\}-2\varepsilon }$ from (6.21) show that for any nonegative integer m,

$$\begin{aligned} (N_2+m)(c^*_2-\varepsilon )-b>(N_2+m+1)(\max \{c, -c^*_{2-}\}+\varepsilon ). \end{aligned}$$

It follows from this and (6.16) that for $(N_2+m)(c^*_2-\varepsilon )-b \le x\le (N_2+m+1)(c^*_2-\varepsilon )$,

$$\begin{aligned} q_{N_2+m+1}(x)<\eta . \end{aligned}$$

This and (6.28) show that

$$\begin{aligned} q_{N_2+m+1}(x)\le A_2 \xi _{\eta 2}({\bar{\mu }}_1) e^{-{\bar{\mu }}_1 (x-(m+1)({{\bar{c}}}+\epsilon )) } +\eta , \ \text{ for } x\le (N_2+m+1) (c^*_2-\varepsilon ). \end{aligned}$$

We conclude that by induction, (6.25) and (6.26) are valid for all nonnegative integer m. It follows that for $x\ge m({\bar{c}}_1+3{\epsilon }/2)$

$$\begin{aligned} p_{N_2+m}(x)\le A_2\xi _{\eta 1}({\bar{\mu }}_1)e^{-{\bar{\mu }}_1 {\epsilon }m/2}, \end{aligned}$$

(6.29)

and for $m({\bar{c}}_1+3{\epsilon }/2)\le x\le m(c^*_2-\varepsilon )$,

$$\begin{aligned} q_{N_2+m}(x)\le A_2 \xi _{\eta 2}({\bar{\mu }}_1)e^{-{\bar{\mu }}_1 {\epsilon }m/2}+\eta . \end{aligned}$$

(6.30)

Choose ${\epsilon }=2{\varepsilon }/3$. Since $\eta$ is arbitrary and since $v_n(x)=1-p_n(x)$, (6.29) and (6.30) lead to statement (i) when condition (a) holds.

The above proof with c replaced by $c^*_1$ and $\mu _c$ replaced by $\mu ^*_1$ also works to prove statement (i) under condition (b).

The proofs for statements (ii)–(iv) are similar to that of statement (i), and are omitted.

The proof of statement (v) is a simplified version of that of statement (i). For the sake of completeness, we provide the details here.

We use ${\tilde{Q}}$ to denote the operator determined by (6.15). We rewrite (6.15) in the form

$$\begin{aligned} \mathbf{w}_{n+1}(x)={\tilde{Q}}[\mathbf{w}_n](x) \end{aligned}$$

where $\mathbf{w}_n(x)=(p_n(x), q_n(x))$. Let ${\varvec{\eta }}=(0, \eta )$ where $\eta$ is a small positive number. Define

$$\begin{aligned} {\tilde{Q}}_{\eta }[\mathbf{w}](x):={\tilde{Q}}[\mathbf{w}+{\varvec{\eta }}]-{\tilde{Q}}[{\varvec{\eta }}]. \end{aligned}$$

${\tilde{Q}}[{\varvec{\eta }}]<{\varvec{\eta }}$. We use ${\tilde{L}}_{\eta }$ to denote the linerization of ${\tilde{Q}}_{\eta }$ at ${ 0}$. ${Q}_{\eta }[( p, q )] \le {L}_{\eta }[(p, q)]$ if

$$\begin{aligned} p\ge \max \{\alpha _2, 1/\alpha _1 \} q. \end{aligned}$$

(6.31)

The moment generating matrix ${\tilde{B}}_\eta (\mu )$ for ${\tilde{L}}_{\eta }$ is given by ${ B}_{\eta }(\mu )$ in (6.18) with subscripts 1 and 2 interchanged. The eigenvalues of ${\tilde{B}}_\eta (\mu )$ are the diagonal entries

$$\begin{aligned} {\lambda }_{\eta 1} (\mu ) = \frac{1+\rho _2}{1+\rho _2\alpha _2(1-\eta )}{\bar{k}}_2(\mu ), \ \ {\lambda }_{\eta 2}( \mu )=\frac{1+\rho _1}{(1+\rho _1(1-\eta ))^2}{\bar{k}}_1(\mu ). \end{aligned}$$

The hypothesis (${\text{ LD }}_{2-}$) implies that when $\eta =0$, ${\lambda }_{\eta 1}( {\bar{\mu }}_{2-})>{\lambda }_{\eta 2}({\bar{\mu }}_{2-})$.

For sufficiently small positive $\epsilon$ and $\eta$,

$$\begin{aligned} \frac{\ln {\lambda }_{\eta 1} ({\bar{\mu }}_{2-})}{{\bar{\mu }}_{2-}} \le {\bar{c}}_{2-}+\epsilon , \ \ \ {\lambda }_{\eta 1} ({\bar{\mu }}_{2-})>{\lambda }_{\eta 2} ({\bar{\mu }}_{2-}). \end{aligned}$$

An eigenvector of ${\tilde{B}}_{{\eta }}({\bar{\mu }}_{2-})$ corresponding to ${\lambda }_{\eta 1} ({\bar{\mu }}_{2-})$ is ${\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})$ with ${\varvec{\xi }}_{\eta }({\mu })$ given by (6.19) and subscripts 1 and 2 interchanged. $\xi _{\eta 2}({\bar{\mu }}_{2-})>0$, and $\xi _{\eta 1}({\bar{\mu }}_{2-})>0$.

Since $u_0(x)=w(x)$ and $\lim _{x\rightarrow -\infty }w(x)=1$, and since $v_0(x)=0$ for sufficiently negative x, there exists $x_0$ such that for $x\le x_0$, $(p_0(x), q_0(x))\le {\varvec{\eta }}$. This implies that there exists a number A such that

$$\begin{aligned} (p_0(x), q_0(x))\le Ae^{{\bar{\mu }}_{2-} x}{\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})+{\varvec{\eta }}. \end{aligned}$$

The hypothesis (${\text{ LD }}_{2-}$) implies that the two components in the vector $( p, q)=A_2 e^{-{\bar{\mu }}_{2-} x } {\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})$ satisfies (6.31). Assume that for some nonnegative integer n and $x\in {{\mathbb {R}}}$,

$$\begin{aligned} (p_n(x), q_n(x))\le Ae^{{\bar{\mu }}_{2-} (x+n{\bar{c}}_{2-})}{\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})+{\varvec{\eta }}. \end{aligned}$$

(6.32)

Then for all $x\in {{\mathbb {R}}}$,

$$\begin{aligned} \begin{aligned} (p_{n+1}(x), p_{n+1}(x))&={\tilde{Q}}[(p_{n}(\cdot ), p_{n}(\cdot ))](x) \\&\le {\tilde{Q}}[ Ae^{{\bar{\mu }}_{2-} (\cdot +n{\bar{c}}_{2-})}{\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-}) +{\varvec{\eta }}](x)\\&\le {\tilde{Q}}_{\eta }[Ae^{{\bar{\mu }}_{2-} (\cdot +n{\bar{c}}_{2-})}{\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})](x)+Q[{\varvec{\eta }}].\\&\le {\tilde{L}}_{\eta } [Ae^{{\bar{\mu }}_{2-} (\cdot +n{\bar{c}}_{2-})}{\varvec{\xi }}_{\eta }({\bar{\mu }}_{2-})](x)+{\varvec{\eta }}\\&\le A e^{{\bar{\mu }}_{2-} (x+(n+1){\bar{c}}_{2-}) } {\varvec{\xi }}_{\eta } ({\bar{\mu }}_{2-})+{\varvec{\eta }}. \end{aligned} \end{aligned}$$

Induction shows that (6.32) is true for all n and $x\in {{\mathbb {R}}}$. If follows that for any ${\varepsilon }>0$, if $x\le -n({\bar{c}}_{2-}+{\varepsilon })$, $(p_n(x), q_n(x))\le A e^{-{\bar{\mu }}_{2-}n{\varepsilon }} {\varvec{\xi }}_{\eta } ({\bar{\mu }}_{2-})+{\varvec{\eta }}.$ Since $\eta$ are arbitrary and since $v_n(x)=1-p_n(x)$, it follows statement (v).

The proof is complete. $\square$

Lemma 6.3

• i. Let $\alpha _2>1>\alpha _1$. Consider (6.14). Suppose that the continuous initial functions $p_0(x)>0$ at some number and $q_0(x)\ge 0$ for $x\in {{\mathbb {R}}}$, and $(p_0(x), q_0(x))\le (1, 1)$. Then for any small positive number $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ -n({\bar{c}}_{1-}-\varepsilon ) \le x\le n({\bar{c}}_{1}+\varepsilon )} (|1-p_n(x)|+|1-q_n(x)|) \right] =0.} \end{aligned}$$

• ii. Let $\alpha _1>1>\alpha _2$. Consider (6.15). Suppose that the continuous initial functions $p_0(x)>0$ at some number and $q_0(x)\ge 0$ for $x\in {{\mathbb {R}}}$, and $(p_0(x), q_0(x))\le (1, 1)$. Then for any small positive number $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ -n({\bar{c}}_{2-}-\varepsilon ) \le x\le n({\bar{c}}_{2}+\varepsilon )} (|1-{p}_n(x)|+|1-{q}_n(x)|) \right] =0.} \end{aligned}$$

• iii. Let $\alpha _2>1>\alpha _1$. Consider (6.14). Suppose that the continuous initial functions $p_0(x)$ and $q_0(x)$ satisfy $(0,0)\le (p_0(x), q_0(x))\le (1, 1)$ and $\liminf _{x\rightarrow -\infty }p_0(x)>0$. Then for any small positive number $\varepsilon$,

  $$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ x\le n({\bar{c}}_{1}+\varepsilon )} (|1-p_n(x)|+|1-q_n(x)|) \right] =0.} \end{aligned}$$

Proof

We use ${\bar{c}}_{b1}$ to denote ${\bar{c}}_1$ with ${\bar{k}}_1(\mu )$ replaced by ${\bar{k}}_{b1}(\mu )$ and use ${\bar{c}}_{b1-}$ to denote ${\bar{c}}_{1-}$ with ${\bar{k}}_{1-}(\mu )$ replaced by ${\bar{k}}_{b1}(-\mu )$ in (2.5). It follows that for any small positive $\varepsilon$, there exists $b_0>0$ such that for $b>b_0$

$$\begin{aligned} {\bar{c}}_{1}>{\bar{c}}_{b1}\ge {\bar{c}}_{1}-\varepsilon /4, \ \ {\bar{c}}_{1-}>{\bar{c}}_{b1-}\ge {\bar{c}}_{1-}-\varepsilon /4. \end{aligned}$$

(6.33)

Since $0\le q_n(x)\le 1$ and $k_1(x)\ge k_{b1}(x)$, the first equation of (6.14) shows that

$$\begin{aligned} {\displaystyle {p}_{n+1}(x) \ge \int _{-\infty }^{\infty }k_{b1}(x-y) \frac{(1+\rho _1)p_n(y)}{1+\rho _1 (\alpha _1+p_n(y))}dy. } \end{aligned}$$

The corresponding equation is

$$\begin{aligned} {\displaystyle {\tilde{p}}_{n+1}(x) = \int _{-\infty }^{\infty }k_{b1}(x-y) \frac{(1+\rho _1){\tilde{p}}_n(y)}{1+\rho _1 (\alpha _1+{\tilde{p}}_n(y))}dy. } \end{aligned}$$

(6.34)

We assume that $b_0$ is chosen to be so large that fro $b\ge b_0$

$$\begin{aligned} \frac{1+\rho _1}{1+\rho _1 \alpha _1} \int _{-\infty }^{\infty }k_{b_01}(x)dx>1. \end{aligned}$$

(6.35)

Under this condition, (6.34) has zero as an unstable equilibrium and a positive equilibrium. Clearly with $p_0(x)= {\tilde{p}}_0(x)$, $p_n(x)\ge {\tilde{p}}_n(x)$ for all n and $x\in {{\mathbb {R}}}$. The rightward and leftward spreading speeds for this equation is ${\bar{c}}_{b1}$ and ${\bar{c}}_{b1-}$, respectively. Let $D>4\pi /\gamma +2b$. Define

$$\begin{aligned} u^{(n)}(\epsilon , \mu _1, \mu _2; x)= \left\{ \begin{array}{ll} v_-({\mu _1}; x+nz_-(\mu _1; \gamma )), &{} \mathrm{if}~ -nz_-(\mu _1; \gamma )-\frac{\pi }{\gamma }\le x\le -nz_-(\mu _1; \gamma ) \\ &{} -\sigma (\mu _1),\\ \\ \epsilon , &{} \mathrm{if}~ -nz_- (\mu _1; \gamma ) - \sigma (\mu _1) \le x \le \sigma (\mu _2)+D \\ &{} ~~~ -\frac{\pi }{\gamma } +nz (\mu _2; \gamma ), \\ dv\left( {\mu _2}; x-D+ \frac{\pi }{\gamma }-nz(\mu _2; \gamma )\right) , &{} \mathrm{if}~ \sigma (\mu _2)+D-\frac{\pi }{\gamma }+nz(\mu _2; \gamma ) \le x \le D \\ &{} ~~~ +nz(\mu _2; \gamma ), \\ 0, &{} ~\mathrm{elsewhere,} \end{array} \right. \end{aligned}$$

(This is the function given in (10) in Li et al. 2016). An argument same as the proof of Lemma 4 (iv) in Li et al. (2016) (with $c=0$) shows that for ${\tilde{p}}_0(x)=p_0(x)$ and any small positive number $\varepsilon$, there exist sufficiently small positive numbers $\alpha$ and $\alpha ^-$, $\epsilon$ and $\gamma$, a large number $D>4\pi /\gamma +2b$, positive numbers $\mu _1$ and $\mu _2$ with $z_-(\mu _1, \gamma )={\bar{c}}_{-1}-\varepsilon /2$ and $z(\mu _2, \gamma )={\bar{c}}_1-\varepsilon /2$, a real number ${\tilde{x}}$, and a positive integer $n_0$, such that for $n\ge n_0$,

$$\begin{aligned} {\tilde{p}}_n(x)\ge u^{(n-n_0)}(\epsilon , \mu _1, \mu _2; x-{\tilde{x}}), \end{aligned}$$

and thus

$$\begin{aligned} p_n(x) \ge u^{(n-n_0)}(\mu _1, \mu _2; x-{\tilde{x}}). \end{aligned}$$

(6.36)

Since ${\bar{c}}_{-1}+{\bar{c}}_1>0$ and ${\varepsilon }$ is small, $z (\mu _2; \gamma )+z_- (\mu _1; \gamma )>0$. (6.36) and the definition of $u^{(n)}(\mu _1, \mu _2; x)$ show that for $n\ge n_0$,

$$\begin{aligned} p_n(x){\ge }\epsilon , \ \text{ for } x\in \left[ {\tilde{x}} {-}nz_{-} (\mu _1; \gamma ) {-} \sigma (\mu _1), \ {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } +nz (\mu _2; \gamma )\right] . \end{aligned}$$

(6.37)

On the other hand, since $z (\mu _2; \gamma )+z_- (\mu _1; \gamma )>0$, there exists $n_1>n_0$ such that for $n\ge n_1$,

$$\begin{aligned} {\tilde{x}} -nz_- (\mu _1; \gamma ) - \sigma (\mu _1)+b < {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } +nz (\mu _2; \gamma )-b. \end{aligned}$$

We may assume that b is so large that

$$\begin{aligned} \int _{-\infty }^{\infty }k_{2b}(x)dx\ge 1-\epsilon . \end{aligned}$$

(6.38)

Since $0\le p_n(x), q_n(x)\le 1$, and $k_2(x)\ge k_{b2}(x)$, the second equation of (6.14) shows that

$$\begin{aligned} {\displaystyle {q}_{n+1}(x) \ge \int _{-\infty }^{\infty }k_{2b}(x-y) \frac{\alpha _2\rho _2p_n(y)}{1+\rho _2\alpha _2}dy}. \end{aligned}$$

(6.39)

This, the assumption $k_{2b}(x)=0$ for $|x|>b$, (6.37), and (6.38) lead to

$$\begin{aligned}&q_n(x){\ge }\frac{\alpha _2\rho _2(1{-}\epsilon )}{1{+}\rho _2\alpha _2}\epsilon >0,\\&\quad \text{ for } x{\in } \left[ {\tilde{x}} {-}nz_- (\mu _1; \gamma ) - \sigma (\mu _1)+b, \ \ {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } +nz (\mu _2; \gamma )-b\right] . \end{aligned}$$

This and (6.37) show that for $n\ge n_1$ and $x\in [{\tilde{x}} -nz_- (\mu _1; \gamma ) - \sigma (\mu _1)+b, \ \ {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } +nz (\mu _2; \gamma )-b]$,

$$\begin{aligned} (p_n(x), q_n(x))\ge \left( {\epsilon }, \frac{\alpha _2\rho _2(1-{\epsilon }){\epsilon }}{1+\rho _2\alpha _2}\right) . \end{aligned}$$

We use $Q^n$ to represent the n-th iteration of Q. Since $\lim _{n\rightarrow \infty }Q^{n}[({\epsilon }, \frac{\alpha _2\rho _2(1-{\epsilon }){\epsilon }}{1+\rho _2\alpha _2})] =(1,1)$, for any small positive $\eta$, there exists $N_1$ such that

$$\begin{aligned} Q^{N_1}\left[ \left( {\epsilon }, \frac{\alpha _2\rho _2(1-{\epsilon }){\epsilon }}{1+\rho _2\alpha _2}\right) \right] > (1-\eta ,1-\eta ). \end{aligned}$$

On the other hand, since $z (\mu _2; \gamma )+z_- (\mu _1; \gamma )>0$, there exists $N_2\ge n_1$ such that for $n\ge N_2$,

$$\begin{aligned} {\tilde{x}} -(n-n_0)z_- (\mu _1; \gamma ) - \sigma (\mu _1)+N_1b< & {} {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma }\\&+(n-n_0)z (\mu _2;\gamma )-N_1b. \end{aligned}$$

We therefore have that for $n\ge n_0+N_1+N_2$ and $x\in [{\tilde{x}} -(n-n_0)z_- (\mu _1; \gamma ) - \sigma (\mu _1)+N_1b < {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } +(n-n_0)z (\mu _2; \gamma )-N_1]$,

$$\begin{aligned} (p_n(x), q_n(x))\ge (1-\eta , 1-\eta ). \end{aligned}$$

Since $-z_- (\mu _1; \gamma ) =-{\bar{c}}_{1-}+{\varepsilon }/2< -{\bar{c}}_{1-}+{\varepsilon }< {\bar{c}}_{1}-{\varepsilon }<z (\mu _2; \gamma )={\bar{c}}_{1}-{\varepsilon }/2$, it follows that there exists an integer $N_3>n_0+N_1+N_2$ such that for $n\ge N_3$,

$$\begin{aligned} {\tilde{x}} -(n-n_0)z_- (\mu _1; \gamma ) - \sigma (\mu _1)+N_1b< & {} -n({\bar{c}}_{1-}-\varepsilon )< n({\bar{c}}_{1}-\varepsilon )\\< & {} {\tilde{x}}+\sigma (\mu _2)+D -\frac{\pi }{\gamma } \\&+(n-n_0)z (\mu _2; \gamma )-N_1b. \end{aligned}$$

We therefore have that for $n\ge N_3$,

$$\begin{aligned} \inf _{ -n({\bar{c}}_{1-}-\varepsilon ) \le x \le n({\bar{c}}_{1}-\varepsilon )} (p_n(x), q_n(x))\ge (1-\eta , 1-\eta ). \end{aligned}$$

Since $\eta$ is arbitrary and $(p_n(x), q_n(x))\le (1, 1)$ for all n and x, the statement (i) holds.

The proof of statement (ii) is similar and omitted.

We finally prove statement (iii). Again for any small positive ${\epsilon }$, we choose b so large that (6.33), (6.35) and (6.38) hold. Define

$$\begin{aligned} {\bar{u}}^{(n)}(\epsilon , \mu _1; x)= \left\{ \begin{array}{ll} \epsilon , &{} \mathrm{if}~ x \le \sigma (\mu _1)\\ &{} ~~~ -\frac{\pi }{\gamma } +nz (\mu _1; \gamma ), \\ dv\left( {\mu _2}; x+ \frac{\pi }{\gamma }-nz(\mu _1; \gamma )\right) , &{} \mathrm{if}~ \sigma (\mu _1)-\frac{\pi }{\gamma }+nz(\mu _1; \gamma ) \le x \le \\ &{} ~~~ +nz(\mu _1; \gamma ), \\ 0, &{} ~\mathrm{elsewhere,} \end{array} \right. \end{aligned}$$

We use $Q^1$ to denote the operator determined by (6.34). The results from Weinberger (1982) shows that for any small positive number $\varepsilon$, there exist sufficiently small positive numbers $\alpha$, $\epsilon$ and $\gamma$, a positive numbers $\mu _1$ and $z(\mu _1, \gamma )={\bar{c}}_1-\varepsilon /2$ such that

$$\begin{aligned} Q^1[{\bar{u}}^{(n)}](x)\ge {\bar{u}}^{(n+1)}(\epsilon , \mu _1; x) \end{aligned}$$

(6.40)

for $n\ge 0$ and $x\in {{\mathbb {R}}}$. Since $\liminf _{x\rightarrow -\infty }p_0(x)>0$, there exists a sufficiently small ${\epsilon }$ and a real number $x_1$ such that $p_0(x)\ge {\epsilon }$ for $x\in (-\infty , x_1]$. This, (6.40), the fact that $p_n(x)\ge {\tilde{p}}_n(x)$, and induction show that for ${\tilde{x}}=x_1-\pi /\gamma$,

$$\begin{aligned} p_n(x)\ge {\bar{u}}^{(n)}(\epsilon , \mu _1; x- {\tilde{x}}). \end{aligned}$$

This yields

$$\begin{aligned} p_n(x)\ge \epsilon , \ \ \text{ for } x\in (-\infty , {\tilde{x}}+\sigma (\mu _1)+nz(\mu _1,\gamma )]. \end{aligned}$$

(6.41)

This, the assumption $k_{2b}(x)=0$ for $|x|>b$ and (6.39) lead to

$$\begin{aligned} q_n(x)\ge \frac{\alpha _2\rho _2(1-\epsilon )}{1+\rho _2\alpha _2}\epsilon , \ \text{ for } x\in (-\infty , {\tilde{x}}+\sigma (\mu _1)+nz(\mu _1,\gamma )-b]. \end{aligned}$$

We therefore have that for any small $\eta >0$ there exists $N_1$ such that for $n\ge N_1$ and $x\in (-\infty , {\tilde{x}}+\sigma (\mu _1)+nz(\mu _1,\gamma )-N_1b]$

$$\begin{aligned} (p_n(x), q_n(x))\ge (1-\eta , 1-\eta ). \end{aligned}$$

Since $z (\mu _1; \gamma )={\bar{c}}_{1}-{\varepsilon }/2$, it follows that there exists an integer $N_2>N_1$ such that for $n\ge N_2$,

$$\begin{aligned} n({\bar{c}}_{1}-\varepsilon ) < {\tilde{x}}+\sigma (\mu _1)+nz(\mu _1,\gamma )-N_1b. \end{aligned}$$

We therefore have that for $n\ge N_2$,

$$\begin{aligned} \inf _{ x \le n({\bar{c}}_{1}-\varepsilon )} (p_n(x), q_n(x))\ge (1-\eta , 1-\eta ). \end{aligned}$$

Since $\eta$ is arbitrary and $(p_n(x), q_n(x))\le (1, 1)$ for all n and x, the statement (iii) holds.

The proof is complete. $\square$

1.2 Proof of Theorem 3.1

The statement (i) (a) follows from (6.3). Since $u_n(x)\le w(x-cn)$ for all n and $x\in {{\mathbb {R}}}$ and since $\lim _{x{\rightarrow }\infty }\sup _{x\ge n(c+{\varepsilon })} w(x)=0$ for any ${\varepsilon }>0$, Lemma 6.1 (iii) leads to the statement (i)(b).

The statement (ii) (a) follows from that $u_n(x)\le w(x-cn)$ and $\lim _{x{\rightarrow }\infty }\sup _{x\ge n(c+{\varepsilon })} w(x)=0$ for any ${\varepsilon }>0$. The statement (ii) (b) directly follows from Lemma 6.1 (v).

1.3 Proof of Theorem 3.2

The statement (i) (a)–(b) of the theorem follows from Lemma 6.2 (i). The statement (i) (c) of the theorem follows from Lemma 6.3 (iii).

It follows from Lemma 6.3 (ii) that for any small positive number $\varepsilon$,

$$\begin{aligned} {\displaystyle \lim _{n\rightarrow \infty }\left[ \sup _{ -n({\bar{c}}_{2-}-\varepsilon ) \le x\le n({\bar{c}}_{2}-\varepsilon )} (u_n(x)+|1-v_n(x)|) \right] =0.} \end{aligned}$$

(6.42)

On the other hand,

$$\begin{aligned} \lim _{n{\rightarrow }\infty }\sup _{x\ge n(c+{\varepsilon })} u_n(x)=0. \end{aligned}$$

(6.43)

for and positive ${\varepsilon }$. Since ${\bar{c}}_2>c$, (6.42) and (6.43) yield the statement (ii)(a). This statement and Lemma 6.1 (iii) lead to the statement (ii) (b). The statement (ii) (c) follows from Lemma 6.2 (v).

1.4 Proof of Theorem 3.3

The statement (a) follows from Lemma 6.3 (ii). The statements (b)(c) follow from Lemma 6.2 (iii). The statement (d) follows from Lemma 6.2 (v).

1.5 Proof of Theorem 4.1

The statement (i) follows from (6.3) and (6.4). The statement (ii) follows from from (6.1) and Lemma 6.1 (iii). The statement (iii) follows from from (6.2) and Lemma 6.1 (iv). The statement (iv) (a) follows from (6.1) and (6.2), and the statement (iv) (b) follows from (6.4) and and Lemma 6.1 (ii).

1.6 Proof of Theorem 4.2

The statement (i)(a) follows from Lemma 6.2 (i) and the Lemma 6.2 (ii). Due to (4.1), $c^*_2>c^*_1$ and $c^*_{2-}>c^*_{1-}$, which implies ${\bar{c}}_1>-c^*_{2-}$ and ${\bar{c}}_{1-}>-c^*_{2}$. The statement (i)(b) then follows from the statement (i)(a) and Lemma 6.1 (iii)–(iv). The statement (i)(c) follows from Lemma 6.3 (i).

The statement (ii)(a) follows from (6.1), and (6.2), the assumption ${\bar{c}}_2>c^*_1$ and ${\bar{c}}_{2-}>c^*_{1-}$, and Lemma 6.3 (ii). The statement (ii)(b) follows from (6.3) and (6.4). Since ${\bar{c}}_2>c^*_1$, ${\bar{c}}_{2-}>c^*_{1-}$, the statement (ii)(c) follows from (6.1), (6.2), and Lemma 6.1 (iii)–(iv), and Lemma 6.3 (ii).

1.7 Proof of Theorem 4.3

The statement (i) (a) follows from (6.2) and Lemma 6.2 (i). The assumption $\bar{c}_{1-}>c^*_{2-}$ and the fact $c^*_1>\bar{c}_1>-\bar{c}_{1-}$ show $\min \{-c^*_{2-},\ c^*_1 \}> -{\bar{c}}_{1-}.$ The statement (i) (b) then follows from Lemma 6.3 (i), (6.4), and Lemma 6.1 (ii). Since ${\bar{c}}_{1-}>c^*_{2-}$, (6.3) and Lemma 6.3 (i) yield the statement (i) (c). The statement (i) (d) follows from Lemma 6.2 (i).

The statement (ii) (a) follows from (6.3) and Lemma 6.2 (iv). The assumption ${\bar{c}}_{2}>c^*_1$ and the fact ${{c}}^*_{2-}>\bar{c}_{2-}>-\bar{c}_2$ show $\min \{-c^*_1, c^*_{2-}\}>-{\bar{c}}_2$. The statement (ii) (b) then follows from Lemma 6.3 (ii), (6.1), and Lemma 6.1 (iii). Since ${\bar{c}}_2>c^*_1$, the statement (ii) (c) follows from (6.1) and Lemma 6.3 (ii). The statement (ii) (d) follows from Lemma 6.2 (iv).