Skip to main content

Advertisement

SpringerLink
Log in

Abrupt transitions to tumor extinction: a phenotypic quasispecies model

  • Published:
Journal of Mathematical Biology Aims and scope Submit manuscript

Abstract

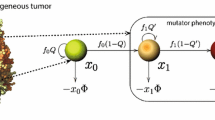
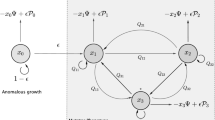
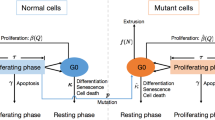
The dynamics of heterogeneous tumor cell populations competing with healthy cells is an important topic in cancer research with deep implications in biomedicine. Multitude of theoretical and computational models have addressed this issue, especially focusing on the nature of the transitions governing tumor clearance as some relevant model parameters are tuned. In this contribution, we analyze a mathematical model of unstable tumor progression using the quasispecies framework. Our aim is to define a minimal model incorporating the dynamics of competition between healthy cells and a heterogeneous population of cancer cell phenotypes involving changes in replication-related genes (i.e., proto-oncogenes and tumor suppressor genes), in genes responsible for genomic stability, and in house-keeping genes. Such mutations or loss of genes result into different phenotypes with increased proliferation rates and/or increased genomic instabilities. Despite bifurcations in the classical deterministic quasispecies model are typically given by smooth, continuous shifts (i.e., transcritical bifurcations), we here identify a novel type of bifurcation causing an abrupt transition to tumor extinction. Such a bifurcation, named as trans-heteroclinic, is characterized by the exchange of stability between two distant fixed points (that do not collide) involving tumor persistence and tumor clearance. The increase of mutation and/or the decrease of the replication rate of tumor cells involves this catastrophic shift of tumor cell populations. The transient times near bifurcation thresholds are also characterized, showing a power law dependence of exponent \(-1\) of the transients as mutation is changed near the bifurcation value. These results are discussed in the context of targeted cancer therapy as a possible therapeutic strategy to force a catastrophic shift by simultaneously delivering mutagenic and cytotoxic drugs inside tumor cells.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  • Adams J, Bellomo N (1997) A survey of models for tumor-immune system dynamics. Birkhäuser

  • Anderson GR, Stoler DL, Brenner BM (2001) Cancer as an evolutionary consequence of a destabilized genome. Bioessays 23:103746

    Article  Google Scholar 

  • Bi P, Ruan S, Zhang X (2014) Periodic and chaotic oscillations in a tumor and immune system interaction model with three delays. Chaos 24:023101

    Article  MathSciNet  MATH  Google Scholar 

  • Bielas JH, Loeb KR, Rubin BP, True LD et al (2006) Human cancers express a mutator phenotype. Proc Natl Acad Sci USA 103:18238–18242

    Article  Google Scholar 

  • Bjedov I, Tenaillon O, Gérard B, Souza V et al (2003) Stress-induced mutagenesis in bacteria. Science 300:1404–1409

    Article  Google Scholar 

  • Bowman P, Jonkers J (2012) The effects of deregulated DNA damage signaling on cancer chemotherapy response and resistance. Nat Rev Cancer 12:587–598

    Article  Google Scholar 

  • Bull JJ, Meyers LA, Lachmann M (2005) Quasispecies made simple. PLOS Comput Biol 1(6):e61

    Article  Google Scholar 

  • Bull JJ, Sanjuán R, Wilke CO (2007) Theory of lethal mutagenesis for viruses. J Virol 81(6):2930–2939

    Article  Google Scholar 

  • Cahill DP, Kinzler KW, Vogelstein B, Lengauer C (1999) Genetic instabilities and Darwinian selection in tumors. Trends Genet 15:M57–61

    Article  Google Scholar 

  • Castillo V, Lázaro JT, Sardanyés J (2016) Dynamics and bifurcations in a simple quasispecies model of tumorigenesis. Comp Appl Math (in press)

  • Chari RV (2008) Targeted cancer therapy: conferring specificity to cytotoxic drugs. Acc Chem Res 41(1):98–107

    Article  Google Scholar 

  • Domingo E, Sheldon J, Perales C (2012) Viral quasispecies evolution. Microbiol Mol Biol 7:159–216

    Article  Google Scholar 

  • Eigen M (1971) Self-organization of matter and the evolution of biological macromolecules. Naturwiss 58:465–523

    Article  Google Scholar 

  • Eigen M, McCaskill J, Schuster P (1989) The molecular quasi-species. Adv Chem Phys 75:149–263

    Google Scholar 

  • Eisenberg E, Levanon EY (2003) Housekeeping genes are compact. Trends Genet 19:362–366

    Article  Google Scholar 

  • Feig DI, Loeb LA (1992) Mechanisms of mutation by oxidative DNA damage: reduced fidelity of mammalian DNA polymerase \(\beta \). Biochemistry 32:4466–4473

    Article  Google Scholar 

  • Fornari C, Balbo G, Halawani SM, Ba-Rukab O, Ahmad AR, Calogero RA et al (2015) A versatile mathematical work-flow to explore how cancer stem cell fate influences tumor progression. BMC Syst Biol 9:1–20

    Article  Google Scholar 

  • Fox EJ, Loeb LA (2010) Lethal mutagenesis: targeting the mutator phenotype in cancer. Sem Cancer Biol 20:353–359

    Article  Google Scholar 

  • Franz S, Peliti L (1997) Error threshold in simple landscapes. J Phys A Math Gen 30:4481–4487

    Article  MathSciNet  MATH  Google Scholar 

  • Garay RP, Lefever R (1978) A kinetic approach of the immunology of cancer: stationary states properties of effector-target cell reactions. J Theor Biol 73:417–438

    Article  MathSciNet  Google Scholar 

  • Gatenby RA, Frieden BR (2002) Application of information theory and extreme physical information to carcinogenesis. Cancer Res 62:3675–3684

    Google Scholar 

  • Hanahan D, Weinberg RA (2000) The hallmarks of cancer. Cell 100:57–70

    Article  Google Scholar 

  • Hanahan D, Weinberg RA (2011) Hallmarks of cancer: the next generation. Cell 144:646–674

  • Helleday H, Petermann E, Lundin C, Hodgson B et al (2008) DNA repair pathways as targets for cancer therapy. Nat Rev Cancer 8:193–200

    Article  Google Scholar 

  • Hoeijmakers JH (2001) Genome maintenance mechanisms for preventing cancer. Nature 411:366–374

    Article  Google Scholar 

  • Itik M, Banks SP (2010) Chaos in a three-dimensional cancer model. Int J Bifurc Chaos 20:71–79

    Article  MathSciNet  MATH  Google Scholar 

  • Kirschner D, Oanetta JC (1998) Modeling immunotherapy of the tumor-immune interaction. J Math Biol 37:235–252

    Article  MATH  Google Scholar 

  • Komarova NL, Wodarz D (2003) Evolutionary dynamics of mutator phenotypes in cancer: implications and chemotherapy. Cancer Res 63:6635–6642

    Google Scholar 

  • Kuznetsov VA, Makalkin IA, Taylor MA, Perelson AS (1994) Nonlinear dynamics of immunogenic tumors: parameter estimation and global bifurcation analysis. Bull Math Biol 56:295–321

    Article  MATH  Google Scholar 

  • Lengauer C, Kinzler KW, Vogelstein B (1998) Genetic instabilities in human cancers. Nature 396:643–649

    Article  Google Scholar 

  • Loeb LA, Essigman JM, Kazazi F, Zhang J, Rose KD, Mullins JI (1999) Lethal mutagenesis of HIV with mutagenic nucleoside analogs. Proc Natl Acad Sci USA 96:1492–1497

    Article  Google Scholar 

  • Loeb LA (2001) A mutator phenotype in cancer. Cancer Res 61:3230–3239

  • Loeb LA (2011) Human cancers express the mutator phenotypes: origin, consequences and targeting. Nature 11:450–457

    Google Scholar 

  • Malgorzata E, Jonkers J (2011) Studying therapy response and resistance in mouse models for BRCA-1deficient breast cancer. J Mammary Gland Biol Neoplasia 16:41–50

    Article  Google Scholar 

  • Merlo LMF, Pepper JW, Reid BJ, Maley CC (2006) Cancer as an evolutionary and ecological process. Nat Rev Cancer 6:924–935

    Article  Google Scholar 

  • Oliver A, Canton R, Campo P, Baquero F et al (2000) High frequency of hypermutable Pseudomonas aeruginosa in cystic fibrosis lung infection. Science 288:1251–1254

    Article  Google Scholar 

  • O’Neil NJ, van Pel DM, Hieter P (2013) Synthetic lethality and cancer: cohesin and PARP at the replication fork. Trends Genet 8:e1002574

  • Rayner E, van Gool IC, Palles C, Kearsey SE et al (2016) A panoply of errors: polymerase proofreading domain mutations in cancer. Nat Rev 16:71–81

    Article  Google Scholar 

  • Sardanyés J, Simó C, Martínez R, Solé RV, Elena SF (2014) Variability in mutational fitness effects prevents full lethal transitions in large quasispecies populations. Sci Rep 4:4625

    Article  Google Scholar 

  • Sardanyés J, Elena SF (2010) Error threshold in RNA quasispecies models with complementation. J Theor Biol 265(3):278-86

  • Sniegowski PD, Gerrish PJ, Lenski RE (1997) Evolution of high mutation rates in experimental populations of E. coli. Nature 387:703–705

    Article  Google Scholar 

  • Solé RV (2003) Phase transitions in unstable cancer cell populations. Eur J Phys B 35:117–124

    Article  Google Scholar 

  • Solé RV (2012) Phase transitions in cancer. In: d’Onofrio A et al. (eds.) New challenges for cancer systems biomedicine. Springer, pp 35–51

  • Solé RV, Sardanyés J, Díez J, Mas A (2006) Information catastrophe in RNA viruses through replication thresholds. J Theor Biol 240(3):353–359

    Article  MathSciNet  Google Scholar 

  • Solé RV, Rodríguez-Caso C, Deisboeck TS, Saldaña J (2008) Cancer stem cells as the engine of unstable tumor progression. J Theor Biol 253:629–637

    Article  MathSciNet  Google Scholar 

  • Solé RV, Valverde S, Rodriguez-Caso C, Sardanyés J (2014) Can a minimal replicating construct be identified as the embodiment of cancer? Bioessays 36:503–512

    Article  Google Scholar 

  • Solé RV, Deisboeck TS (2004) An error catastrophe in cancer? J Theor Biol 228:47–54

  • Sun W, Jiang T, Lu Y, Reiff M, Mo R, Gu Z (2014) Cocoon-like self-degradable DNA nano clew for anticancer drug delivery. J Am Chem Soc 136:14722–14725

    Article  Google Scholar 

  • Vogelstein B, Kinzler KW (2004) Cancer genes and the pathways they control. Nat Med 10:789–799

    Article  Google Scholar 

  • Wilke CO, Ronnewinkel C, Martinetz T (2001) Dynamic fitness landscapes in molecular evolution. Phys Rep 349:395–446

    Article  MathSciNet  MATH  Google Scholar 

  • Wylie CS, Shakhnovic EL (2012) Mutation induced extinction in finite populations: lethal mutagenesis and lethal isolation. PLOS Comput Biol 8:e1002609

    Article  MathSciNet  Google Scholar 

  • Yue QX, Liu X, Guo DA (2010) Microtubule-binding natural products for cancer therapy. Planta Medica 76(11):1037–1043

    Article  Google Scholar 

Download references

Acknowledgments

We thank the members of the Complex Systems Lab for their helpful comments, as well as the members of the Institute of Evolutionary Biology (IBE) for their critics and comments. The authors acknowledge the computing facilities of the Dynamical Systems Group (Universitat de Barcelona). This article was partially funded by the Botín Foundation (JS, RVS), by the Spanish grant FIS2012-39288 (RVS), by the Santa Fe Institute (RVS), by the Spanish Secretaria de Estado de Investigación, Desarrollo e Innovación grants MTM2013-41168-P (CS, RM), and by grant 2014-SGR-1145 from the Catalan government (CS, RM). This work has also counted with the support of Secretaria d’Universitats i Recerca del Departament d’Economia i Coneixement de la Generalitat de Catalunya.

Author information

Authors and Affiliations

  1. ICREA-Complex Systems Lab, Department of Experimental and Health Sciences, Universitat Pompeu Fabra, Barcelona, Spain

    Josep Sardanyés & Ricard Solé

  2. Institut de Biologia Evolutiva, CSIC-Universitat Pompeu Fabra, Barcelona, Spain

    Josep Sardanyés & Ricard Solé

  3. Departament de Matemàtiques, Edifici C. Facultat de Ciències, Universitat Autònoma de Barcelona, Bellaterra, Barcelona, Spain

    Regina Martínez

  4. Departament de Matemàtiques i Informàtica, Universitat de Barcelona, Barcelona, Spain

    Carles Simó

  5. The Santa Fe Institute, Santa Fe, New Mexico, USA

    Ricard Solé

Authors
  1. Josep Sardanyés
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Regina Martínez
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Carles Simó
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ricard Solé
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Josep Sardanyés.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sardanyés, J., Martínez, R., Simó, C. et al. Abrupt transitions to tumor extinction: a phenotypic quasispecies model. J. Math. Biol. 74, 1589–1609 (2017). https://doi.org/10.1007/s00285-016-1062-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00285-016-1062-9

Keywords

Mathematics Subject Classification

Search

Navigation