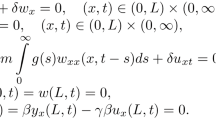Skip to main content
SpringerLink
Log in

Stabilization Results of a Piezoelectric Beams with Partial Viscous Dampings and Under Lorenz Gauge Condition

  • Published:
Applied Mathematics & Optimization Aims and scope Submit manuscript

Abstract

In this paper, we investigate the stabilization of a one-dimensional piezoelectric (Stretching system) with partial viscous dampings. First, by using Lorenz gauge conditions, we reformulate our system to achieve the existence and uniqueness of the solution. Next, by using General criteria of Arendt–Batty, we prove the strong stability in different cases. Finally, we prove that it is sufficient to control the stretching of the center-line of the beam in x-direction to achieve the exponential stability. Numerical results are also presented to validate our theoretical result.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Afilal, M., Soufyane, A., de Lima Santos, M.: Piezoelectric beams with magnetic effect and localized damping. Math. Control Relat. Fields (2021)

  2. Akil, M.: Stability of piezoelectric beam with magnetic effect under (coleman or pipkin)-gurtin thermal law. Z. Angew. Math. Phys. 73(6), 236 (2022)

  3. An, Y., Liu, W., Kong, A.: Stability of piezoelectric beams with magnetic effects of fractional derivative type and with/without thermal effects (2021)

  4. Arendt, W., Batty, C.J.K.: Tauberian theorems and stability of one-parameter semigroups. Trans. Am. Math. Soc. 306(2), 837–852 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  5. Banks, H.T., Smith, R.C., Wang, Y.: Smart material structures: modeling, estimation, and control (1996)

  6. Destuynder, P., Legrain, I., Castel, L., Richard, N.: Theoretical, numerical and experimental discussion on the use of piezoelectric devices for control-structure interaction. Eur. J. Mech. A Solids 11, 181–213 (1992)

    MathSciNet  Google Scholar 

  7. Hansen, S.: Analysis of a plate with a localized piezoelectric patch. In: Proceedings of the 37th IEEE Conference on Decision and Control (Cat. No.98CH36171), vol. 3, pp. 2952–2957 (1998)

  8. Huang, F.L.: Characteristic conditions for exponential stability of linear dynamical systems in Hilbert spaces. Ann. Differ. Equ. 1(1), 43–56 (1985)

    MathSciNet  MATH  Google Scholar 

  9. Kapitonov, B., Miara, B., Menzala, G.P.: Boundary observation and exact control of a quasi-electrostatic piezoelectric system in multilayered media. SIAM J. Control Optim. 46(3), 1080–1097 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  10. Lasiecka, I., Miara, B.: Exact controllability of a 3d piezoelectric body. Comptes Rendus Mathematique 347(3), 167–172 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  11. Lions, J.L.: Exact controllability, stabilization and perturbations for distributed systems. SIAM Rev. 30(1), 1–68 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  12. Morris, K., Özer, A.: Strong stabilization of piezoelectric beams with magnetic effects, pp 3014–3019 (2013)

  13. Morris, K.A., Özer, A.Ö.: Modeling and stabilizability of voltage-actuated piezoelectric beams with magnetic effects. SIAM J. Control. Optim. 52, 2371–2398 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  14. Oh, S.J., Tataru, D.: Local well-posedness of the (4 + 1)-dimensional maxwell-klein-gordon equation at energy regularity. Ann. PDE 2, 2 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  15. Özer, A.Ö.: Potential formulation for charge or current-controlled piezoelectric smart composites and stabilization results: electrostatic versus quasi-static versus fully-dynamic approaches. IEEE Trans. Autom. Control 64, 989–1002 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  16. Özer, Ahmet Özkan: Stabilization results for well-posed potential formulations of a current-controlled piezoelectric beam and their approximations. Appl. Math. Optim. 84, 877–914 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  17. Özer, A.Ö., Morris, K.A.: Modeling and stabilization of current-controlled piezo-electric beams with dynamic electromagnetic field. ESAIM: COCV 26, 8 (2020)

    MathSciNet  MATH  Google Scholar 

  18. Prüss, J.: On the spectrum of \(C_{0}\) -semigroups. Trans. Am. Math. Soc. 284(2), 847–857 (1984)

    MATH  Google Scholar 

  19. Ramos, A.J.A., Freitas, M.M., Almeida, D.S., Jesus, S.S., Moura, T.R.S.: Equivalence between exponential stabilization and boundary observability for piezoelectric beams with magnetic effect. Zeitschrift far angewandte Mathematik und Physik 70(2), 60 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  20. Ramos, A.J., Gonçalves, C.S., Neto, S.S.: Exponential stability and numerical treatment for piezoelectric beams with magnetic effect. ESAIM: M2AN 52(1), 255–274 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  21. Rogacheva, N.: The Theory of Piezoelectric Shells and Plates, 1st edn. CRC Press, Cambridge University Press, Cambridge (1994)

    MATH  Google Scholar 

  22. Selberg, S., Tesfahun, A.: Finite-energy global well-posedness of the Maxwell–Klein–Gordon system in Lorenz gauge. Commun. Partial Differ. Equ. 35(6), 1029–1057 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  23. Smith, R.: Smart Material Systems. Frontiers in Applied Mathematics. Society for Industrial and Applied Mathematics, 1 edn (2005)

  24. Soufyane, A., Afilal, M., Santos, M.L.: Energy decay for a weakly nonlinear damped piezoelectric beams with magnetic effects and a nonlinear delay term. Zeitschrift für angewandte Mathematik und Physik, 72(4) (2021)

  25. Tebou, L.T., Zuazua, E.: Uniform boundary stabilization of the finite difference space discretization of the 1-d wave equation. Adv. Comput. Math. 26(1), 337 (2006)

    MathSciNet  MATH  Google Scholar 

  26. Tiersten, H.: Linear Piezoelectric Plate Vibrations, 1st edn. CRC Press, Springer, New York, NY (1969)

    Book  Google Scholar 

  27. Tzou, H.: Piezoelectric Shells: Sensing, Energy Harvesting, and Distributed Control. Solid Mechanics and Its Applications, 2nd edition. Springer, Dordrecht (2019)

  28. Yang, J.: An Introduction to the Theory of Piezoelectricity, 1st edn. Advances in Mechanics and Mathematics. Springer, New York (2005)

Download references

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. Univ. Polytechnique Hauts-de-France, INSA Hauts-de-France, CERAMATHS-Laboratoire de Matériaux Céramiques et de Mathématiques, 59313, Valenciennes, France

    Mohammad Akil

  2. Department of Mathematics, College of Science, University of Sharjah, P.O.Box 27272, Sharjah, UAE

    Abdelaziz Soufyane

  3. Department of Mathematics and Statistics, American University of Sharjah, Sharjah, UAE

    Youssef Belhamadia

Authors
  1. Mohammad Akil
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abdelaziz Soufyane
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Youssef Belhamadia
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mohammad Akil.

Ethics declarations

Competing interest

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Akil, M., Soufyane, A. & Belhamadia, Y. Stabilization Results of a Piezoelectric Beams with Partial Viscous Dampings and Under Lorenz Gauge Condition. Appl Math Optim 87, 26 (2023). https://doi.org/10.1007/s00245-022-09935-3

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00245-022-09935-3

Keywords

Search

Navigation