Skip to main content
SpringerLink
Log in

On Numerical Approaches for Solving an Inverse Cauchy Stokes Problem

  • Published:
Applied Mathematics & Optimization Aims and scope Submit manuscript

Abstract

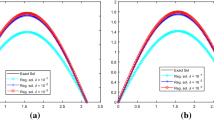
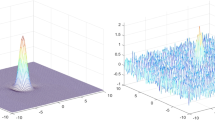
In this paper, we are interested in the study of an inverse Cauchy problem governed by Stokes equation. It consists in determining the fluid velocity and the flux over a part of the boundary, by introducing given measurements on the remaining part. As it’s known, it is one of highly ill-posed problems in the Hadamard’s sense (Phys Today 6:18, 1953), it is then an interesting challenge to carry out a numerical procedure for approximating their solutions, in particular, in the presence of noisy data. To solve this problem, we propose here a regularizing approach based on a Tikhonov regularization method. We show the existence of the regularization optimization problem and prove the convergence of subsequence of optimal solutions of Tikhonov regularization formulations to the solution of the Cauchy problem, when the noise level goes to zero. Then, we suggest the numerical approximation of this problem using the finite elements method of \(P_{1Bubble}/P_1\) type’s, we show the existence of the discrete optimal regularized solution without noise and prove the convergence of subsequence of discrete optimal solutions to the solution of the continuous optimization problem. Finally, we provide some numerical results showing the accuracy and the efficiency of the proposed approach.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Alessandrini, G., Rondi, L., Rosset, E., Vessella, S.: The stability for the Cauchy problem for elliptic equations. Inverse Prob. 25(12), 123004 (2009)

    Article  MathSciNet  Google Scholar 

  2. Andrieux, S., Baranger, T.N.: An energy error-based method for the resolution of the Cauchy problem in 3d linear elasticity. Comput. Methods Appl. Mech. Eng. 197(9–12), 902–920 (2008)

    Article  MathSciNet  Google Scholar 

  3. Andrieux, S., Abda, A.B., Baranger, T.N.: Data completion via an energy error functional. Comptes Rendus Mécanique 333(2), 171–177 (2005)

    Article  Google Scholar 

  4. Bauer, F., Kindermann, S.: The quasi-optimality criterion for classical inverse problems. Inverse Prob. 24(3), 035002 (2008)

    Article  MathSciNet  Google Scholar 

  5. Belgacem, F.B., El Fekih, H.: On Cauchy’s problem: I. A variational Steklov-Poincaré theory. Inverse Prob. 21(6), 1915 (2005)

    Article  Google Scholar 

  6. Berntsson, F., Kozlov, V.A., Mpinganzima, L., Turesson, B.O.: An accelerated alternating procedure for the Cauchy problem for the Helmholtz equation. Comput. Math. Appl. 68(1), 44–60 (2014)

    Article  MathSciNet  Google Scholar 

  7. Bourgeois, L.: Contrôle optimal et problemes inverses en plasticité. PhD thesis, Palaiseau, Ecole polytechnique (1998)

  8. Boussetila, N., Hamida, S., Rebbani, F.: Spectral regularization methods for an abstract ill-posed elliptic problem. In: Abstract and Applied Analysis, vol. 2013. Hindawi Publishing Corporation (2013)

  9. Boyer, F., Fabrie, P.: Mathematical Tools for the Study of the Incompressible Navier–Stokes Equations and Related Models, vol. 183. Springer, New York (2012)

    MATH  Google Scholar 

  10. Brezis, H.: Functional Analysis, Sobolev Spaces and Partial Differential Equations. Springer, New York (2010)

    Google Scholar 

  11. Brezzi, F., Fortin, M.: Mixed and Hybrid Finite Element Methods, No. 15 in Springer Series in Computational Mathematics. Springer, New York (1991)

    Book  Google Scholar 

  12. Chakib, A., Nachaoui, A.: Convergence analysis for finite element approximation to an inverse Cauchy problem. Inverse Prob. 22(4), 1191 (2006)

    Article  MathSciNet  Google Scholar 

  13. Chakib, A., Ouaissa, H.: On numerical resolution of an inverse Cauchy problem modeling the airflow in the bronchial tree. Comput. Appl. Math. 40(1), 1–31 (2021)

    Article  MathSciNet  Google Scholar 

  14. Chakib, A., Nachaoui, A., Nachaoui, M., Ouaissa, H.: On a fixed point study of an inverse problem governed by Stokes equation. Inverse Prob. 35(1), 015008 (2018)

    Article  MathSciNet  Google Scholar 

  15. Chen, J.-T., Chen, K.: Analytical study and numerical experiments for laplace equation with overspecified boundary conditions. Appl. Math. Model. 22(9), 703–725 (1998)

    Article  Google Scholar 

  16. Chen, L., Chen, J.-T., Hong, H.-K., Chen, C.: Application of cesaro mean and the l-curve for the deconvolution problem. Soil Dyn. Earthq. Eng. 14(5), 361–373 (1995)

    Article  Google Scholar 

  17. Chen, J.-T., Hsiao, C.-C., Leu, S.-Y.: A new method for Stokes problems with circular boundaries using degenerate kernel and Fourier series. Int. J. Numer. Method Eng. 74(13), 1955–1987 (2008)

    Article  MathSciNet  Google Scholar 

  18. Cheng, X., Gong, R., Han, W., Zheng, X.: A novel coupled complex boundary method for solving inverse source problems. Inverse Prob. 30(5), 055002 (2014)

    Article  MathSciNet  Google Scholar 

  19. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. SIAM, Philadelphia (2002)

    Book  Google Scholar 

  20. Clément, P.: Approximation by finite element functions using local regularization. Revue française d’automatique, informatique, recherche opérationnelle. Anal. Numér. 9(R2), 77–84 (1975)

    Google Scholar 

  21. Eldén, L., Berntsson, F.: A stability estimate for a Cauchy problem for an elliptic partial differential equation. Inverse Prob. 21(5), 1643 (2005)

    Article  MathSciNet  Google Scholar 

  22. Fairweather, G., Karageorghis, A.: The method of fundamental solutions for elliptic boundary value problems. Adv. Comput. Math. 9(1–2), 69 (1998)

    Article  MathSciNet  Google Scholar 

  23. Franzone, P.C.: Numerical Treatment of Inverse Problems in Differential and Integral Equations, pp. 180–205. Springer, New York (1983)

    Book  Google Scholar 

  24. Hadamard, J., Morse, P.M.: Lectures on Cauchy’s problem in linear partial differential equations. Phys. Today 6, 18 (1953)

    Article  Google Scholar 

  25. Hämarik, U., Tautenhahn, U.: On the monotone error rule for parameter choice in iterative and continuous regularization methods. BIT Numer. Math. 41(5), 1029–1038 (2001)

    Article  MathSciNet  Google Scholar 

  26. Hansen, P.C.: Rank-Deficient and Discrete Ill-Posed Problems: Numerical Aspects of Linear Inversion. SIAM, Philadelphia (1998)

    Book  Google Scholar 

  27. Hansen, P.C.: The l-curve and its use in the numerical treatment of inverse problems. In: Johnston, P.R. (ed.) Computational Inverse Problems in Electrocardiology, pp. 119–142. WIT Press, Southampton (2001)

    Google Scholar 

  28. Hào, D.N., Van Duc, N., Lesnic, D.: A non-local boundary value problem method for the Cauchy problem for elliptic equations. Inverse Prob. 25(5), 055002 (2009)

    Article  MathSciNet  Google Scholar 

  29. Heinz, A.L., Engl, W.: A mann iterative regularization method for elliptic Cauchy problems. Numer. Funct. Anal. Optim. 22, 861–884 (2001)

    Article  MathSciNet  Google Scholar 

  30. Inglese, G.: An inverse problem in corrosion detection. Inverse Prob. 13(4), 977 (1997)

    Article  MathSciNet  Google Scholar 

  31. Johnson, J.L.: Numerical simulation and optimal control in plasma physics: with applications to tokamaks (jacques blum). SIAM Rev. 33(1), 147–150 (1991)

    Article  Google Scholar 

  32. Klibanov, M.V.: Carleman estimates for the regularization of ill-posed Cauchy problems. Appl. Numer. Math. 94, 46–74 (2015)

    Article  MathSciNet  Google Scholar 

  33. Kozlov, V.A., Maz’Ya, V.G., Fomin, A.V.: An iterative method for solving the Cauchy problem for elliptic equations. Comput. Math. Math. Phys. 31(1), 45–52 (1991)

    MathSciNet  MATH  Google Scholar 

  34. Lattés, R., Lions, J.L.: The Method of Quasi-Reversibility: Applications to Partial Differential Equations. Elsevier, New York (1969)

    MATH  Google Scholar 

  35. Morozov, V.A.: On the solution of functional equations by the method of regularization. Dokl. Math. 7, 414–417 (1966)

    MathSciNet  MATH  Google Scholar 

  36. Qin, H., Wei, T.: Two regularization methods for the Cauchy problems of the Helmholtz equation. Appl. Math. Model. 34(4), 947–967 (2010)

    Article  MathSciNet  Google Scholar 

  37. Quarteroni, A., Valli, A.: Domain Decomposition Methods for Partial Differential Equations. Oxford University Press, Oxford (1999)

    MATH  Google Scholar 

  38. Tikhonov, A.N., Arsenin, V.I., John, F.: Solutions of Iill-Posed Problems, vol. 14. Winston, Washington, DC (1977)

    Google Scholar 

  39. Wahba, G.: Practical approximate solutions to linear operator equations when the data are noisy. SIAM J. Numer. Anal. 14(4), 651–667 (1977)

    Article  MathSciNet  Google Scholar 

  40. Wei, T., Zhou, D.: Convergence analysis for the Cauchy problem of Laplace’s equation by a regularized method of fundamental solutions. Adv. Comput. Math. 33(4), 491–510 (2010)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Laboratoire de Mathématiques et Applications, Faculté des Sciences et Techniques, Université Sultan Moulay Slimane, B.P.523, Béni-Mellal, Morocco

    Hamid Ouaissa, Abdelkrim Chakib & Mourad Nachaoui

  2. Laboratoire de Mathématiques Jean Leray UMR6629 CNRS / Université de Nantes 2 rue de la Houssinière, BP92208, 44322, Nantes, France

    Abdeljalil Nachaoui

Authors
  1. Hamid Ouaissa
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abdelkrim Chakib
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Abdeljalil Nachaoui
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mourad Nachaoui
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ouaissa, H., Chakib, A., Nachaoui, A. et al. On Numerical Approaches for Solving an Inverse Cauchy Stokes Problem. Appl Math Optim 85, 3 (2022). https://doi.org/10.1007/s00245-022-09833-8

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00245-022-09833-8

Keywords

Mathematics Subject Classification

Search

Navigation