Measurement of local tissue perfusion through a minimally invasive heating bead

Abstract

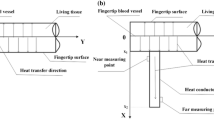
A minimally invasive approach was proposed to measure local blood perfusion rate in living tissues, based on the well-known Pennes bioheat equation. The measuring probe consists of a heater covered with conductive epoxy and temperature sensor deposited on the probe–tissue interface. By monitoring the probe–tissue interface’s temperature response before and after employing the constant heat flux, the tissue blood perfusion rate can be obtained. A theoretical model was developed to describe the measurement system. In vivo experiments were performed on the rabbit’s thighs to validate this method. At last, uncertainties implied in the temperature measurement and voltage across the heater was evaluated. The results point out the way to improve the accuracy of the present method and its appropriate application occasion.

Appendix: Temperature response of living tissue subjected to constant surface flux heating

To analyze the transient temperature response of living tissues subjected to the constant surface flux heating, the theoretical model was established as follows,

$$ \frac{1}{r}\frac{{\partial ^{2} (rT)}}{{\partial r^{2}}} + W_{b} C_{b} (T_{a} - T)/K + \frac{{Q_{m} (t)}}{K} = \frac{1}{\alpha}\frac{{\partial T}}{{\partial t}} $$

(A1)

At the distant site far from the bead, the constant heating almost has no influence on the temperature there. In order to solve the question, at r =  R′ =  0.02 m is assumed as the distant site far from the bead. The equilibrium temperature under a constant voltage is the initial temperature at the following heating process. Then boundary and initial conditions to Eq. (A1) can then be expressed as:

$$ - K \cdot \frac{{\partial T}}{{\partial r}}\bigg| {_{{r = R_{0}}}} = q_{0}, \quad r = R_{0} $$

(A2a)

$$ \frac{{\partial T}}{{\partial r}} = 0, \quad r = {R}^{\prime} $$

(A2b)

$$ T(r,0) = T_{0}, \quad t = 0 $$

(A2c)

Assuming

$$ T{\left({r,t} \right)} = T_{0} + \psi {\left({r,t} \right)}\exp {\left({- \frac{{W_{b} C_{b}}}{{\rho C}}t} \right)} $$

(A3)

Then Eqs. (A1–A2a, b, c) are transformed into

$$ \frac{{\partial ^{2} \psi}}{{\partial r^{2}}} + \frac{2}{r}\frac{{\partial \psi}}{{\partial r}} = \frac{1}{\alpha}\frac{{\partial \psi}}{{\partial t}} $$

(A4)

$$ - K \cdot \frac{{\partial \psi}}{{\partial r}}\bigg| {_{{r = R_{0}}}} = q_{0} \exp {\left({\frac{{W_{b} C_{b}}}{{\rho C}}t} \right)}, \quad r = R_{0} $$

(A5a)

$$ \frac{{\partial \psi}}{{\partial r}} = 0,\quad r = {R}^{\prime} $$

(A5b)

$$\psi (r,0) = 0,\quad t = 0 $$

(A5c)

If the Green’s function for the above Eq. (A4) is obtained, the transient tissue temperature can easily be constructed [20]. Through introducing an auxiliary problem corresponding to Eq. (A4), the Green’s function can finally be obtained as (detailed derivation is omitted here)

$$ \begin{aligned} G{\left({\left. {r,t} \right|{r}^{\prime},\tau} \right)} &= 3/{{\left({b^{3} - a^{3}} \right)}} + \frac{1}{{r \cdot {r}^{\prime}}}{\sum\limits_{m = 1}^{\infty} {e^{{- \alpha \beta ^{2}_{m} {\left({t - \tau} \right)}}} {\big\{{\beta_{m} \cos {\left[ {\beta_{m} {\left({r - R_{0}} \right)}} \right]} + {\sin {\left[ {\beta_{m} {\left({r - R_{0}} \right)}} \right]}} /{R_{0}}} \big\}}}} \\ & \quad \times \frac{2}{{{\left({\beta ^{2}_{m} + 1/{R^{2}_{0}}} \right)}{\left[ {{\left({{R}^{\prime} - R_{0}} \right)} - 1/ {{\left[ {{R}^{\prime}{\left({\beta ^{2}_{m} + 1 / {{R}^{\prime 2}}} \right)}} \right]}}} \right]} + 1/ {R_{0}}}} \\ & \quad \times {\big\{{\beta_{m} \cos {\left[ {\beta_{m} {\left({{r}^{\prime} - R_{0}} \right)}} \right]} + {\sin {\left[ {\beta_{m} {\left({{r}^{\prime} - R_{0}} \right)}} \right]}} /{R_{0}}} \big\}} \\ \end{aligned} $$

(A6)

where β _m ’s are the positive roots of

$$ \tan \beta_{m} {\left({{R}^{\prime} - R_{0}} \right)} = \frac{{\beta_{m} {\left({1 / {R_{0}} + 1 /{{R}^{\prime}}} \right)}}}{{\beta ^{2}_{m} - 1 /{{R}^{\prime}R_{0}}}} $$

(A7)

Then the tissue temperature field could be constructed with Eq. (A3)

$$ \begin{aligned} T{\left({r,t} \right)} &= T_{0} + \frac{{3\alpha R^{2}_{0} q_{0} {\left({1 - e^{{- {\left({{W_{b} C_{b}} /{\rho C}} \right)}t}}} \right)}}}{{K{\left({{R}^{\prime 3}- R^{3}_{0}} \right)}{\left({{W_{b} C_{b}}/ {\rho C}} \right)}}} + \frac{{\alpha R_{0}}}{{Kr}}{\sum\limits_{m = 1}^{\infty} {\frac{{\beta_{m}}}{{{W_{b} C_{b}} / {\rho C + \alpha \beta ^{2}_{m}}}}}}{\left({1 - e^{{- {\left({{W_{b} C_{b}} / {\rho C + \alpha \beta ^{2}_{m}}} \right)}t}}} \right)} \\ & \quad \times {\big\{{\beta_{m} \cos {\left[ {\beta_{m} {\left({r - R_{0}} \right)}} \right]} + {\sin {\left[ {\beta_{m} {\left({r - R_{0}} \right)}} \right]}} /{R_{0}}} \big\}} \\ &\quad \times \frac{2} {\left(\beta^2_m+1/R^2_0\right)\left[(R^{\prime}-R_0) -1/\left[R^{\prime}\left(\beta^2_m+1/R^{\prime 2}\right)\right]\right]+1/R_0} \end{aligned} $$

(A8)

The final solution for bead-tissue interface temperature T(R ₀, t ) is therefore in the form of

$$ \begin{aligned} T{\left({R_{0}, t} \right)} &= T_{0} + \frac{{3\alpha R^{2}_{0} q_{0} {\left({1 - e^{{- {W_{b} C_{b} t} /{\rho C}}}} \right)}}}{{K{\left({{R}^{\prime 3} - R^{3}_{0}} \right)}{\left({{W_{b} C_{b}} /{\rho C}} \right)}}} + \frac{\alpha}{K}{\sum\limits_{m = 1}^{\infty} {\frac{{2\beta ^{2}_{m}}}{{{W_{b} C_{b}}/ {\rho C + \alpha \beta ^{2}_{m}}}}}}{\left({1 - e^{{- {\left({{W_{b} C_{b}}/ {\rho C + \alpha \beta ^{2}_{m}}} \right)}t}}} \right)} \\ & \quad \times \frac{{\hbox{1}}}{{{\left({\beta ^{2}_{m} + 1 /{R^{2}_{0}}} \right)}{\left[ {{\left({{R}^{\prime} - R_{0}} \right)} - 1/ {{\left[ {{R}^{\prime}{\left({\beta ^{2}_{m} + 1/ {{R}^{\prime 2}}} \right)}} \right]}}} \right]} + 1/{R_{0}}}} \\ \end{aligned} $$

(A9)