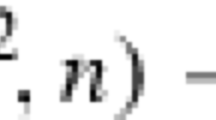Abstract
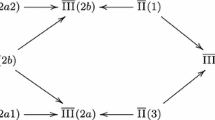
The spaces of point configurations on the projective line up to the action of \({{\rm SL}(2,\mathbb{K})}\) and its maximal torus are canonically compactified by the Grothdieck–Knudsen and Losev–Manin moduli spaces \({\overline{M}_{0,n}}\) and \({\overline{L}_n}\) respectively. We examine the configuration space up to the action of the maximal unipotent group \({\mathbb{G}_a \subseteq {\rm SL}(2,,\mathbb{K})}\) and define an analogous compactification. For this we first assign a canonical quotient to the action of a unipotent group on a projective variety. Moreover, we show that similar to \({\overline{M}_{0,n}}\) and \({\overline{L}_n}\) this quotient arises in a sequence of blow-ups from a product of projective spaces.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Arzhantsev, I., Derenthal, U., Hausen, J., Laface, A.: Cox rings. arXiv:1003.4229v2 [math.AG], (2011)
Arzhantsev I.V., Celik D., Hausen J.: Factorial algebraic group actions and categorical quotients. J. Algebra 387, 87–98 (2013)
Arzhantsev I.V., Hausen J.: Geometric invariant theory via cox rings. J. Pure Appl. Algebr. 213, 154–172 (2009)
Bäker, H.: On the Cox ring of blowing up the diagonal. preprint: arXiv:1402.5509
Bäker, H., Hausen, J., Keicher, S.: On Chow quotients of torus actions. preprint: arXiv:1203.3759
Berchtold F., Hausen J.: GIT-equivalence beyond the ample cone. Mich. Math. J. 54(3), 483–515 (2006)
Cox D.: The homogeneous coordinate ring of a toric variety. J. Algebr. Geom. 4(1), 17–50 (1995)
Dolgachev I.V., Hu Y.: Variation of geometric invariant theory quotients. Publications Mathematiques De L Ihes 87, 5–51 (1998)
Doran, B., Kirwan, F.: Towards non-reductive geometric invariant theory. Pure Appl. Math. Q., 3 (1, part 3):61–105, (2007)
Feichtner E.-M., Kozlov D.N.: Incidence combinatorics of resolutions. Selecta Math. (N.S.) 10(1), 37–60 (2004)
Gelfand, I.M., Kapranov, M.M., Zelevinsky, A.V.: Discriminants, Resultants And Multidimensional Determinants. Modern Birkhäuser Classics. Birkhäuser Boston Inc., Boston, MA. Reprint of the 1994 edition, (2008).
Hausen, J.: Geometric invariant theory based on Weil divisors. Compos. Math. 140(6):1518–1536, (2004). MR2098400.
Hausen J.: Cox rings and combinatorics II. Moscow Math. J. 8(4), 711–757 (2008)
Huggenberger, E.: Fano Varieties with Torus Action of Complexity One. Doctoral Thesis http://nbn-resolving.de/urn:nbn:de:bsz:21-opus-69570
Kapranov M., Sturmfels B., Zelevinsky A.: Quotients of toric varieties. Mathematische Annalen 290(4), 643–655 (1991)
Kapranov, M.M.: Chow quotients of Grassmannians. I. In I. M. Gel’fand Seminar, volume 16 of Adv. Soviet Math., pp. 29–110. Am. Math. Soc., Providence, RI (1993). MR1237834
Losev, A., Manin, Y.: New moduli spaces of pointed curves and pencils of flat connections. Michigan Math. J., 48, 443–472 (2000). Dedicated to William Fulton on the occasion of his 60th birthday.
Shmelkin D.A.: First fundamental theorem for covariants of classical groups. Adv. Math. 167(2), 175–194 (2002)
Speyer D., Sturmfels B.: The tropical Grassmannian. Adv. Geom. 4(3), 389–411 (2004)
Tevelev J.: Compactifications of subvarieties of tori. Am. J. Math. 129(4), 1087–1104 (2007)
